空间形式Sn+p(1)中平均曲率与纯量曲率成线性关系的完备非紧子流形

王 琪

(贵阳学院数学系，中国 贵阳 550005)

文献[1～2]首先研究了正曲率空间形式中紧致闭子流形为全脐或有全脐乘积分解的一种充分条件．随后，文献[3～4]等对此作了进一步研究．之后，文献[5]研究了空间形式中常纯量曲率的完备非紧子流形．

定理1[5]设Mn是空间形式Sn+p(1)中连通的完备非紧等距浸入子流形且单位平均曲率向量在法丛中平行．若Mn有常数纯量曲率R且R≥n(n-1)，则有如下结论：

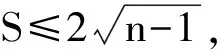
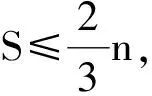
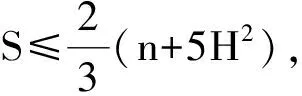
本文进一步得到如下定理A，定理A推广并改进了定理1的结论．

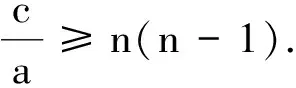
1 准备知识和若干引理

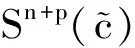
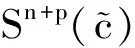
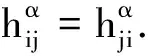
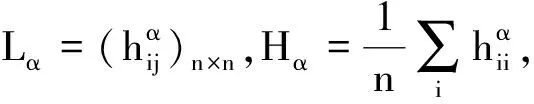
Mn的黎曼曲率张量Rijkl，法曲率张量Rαβij及纯量曲率R有如下关系[1-13]

(1)

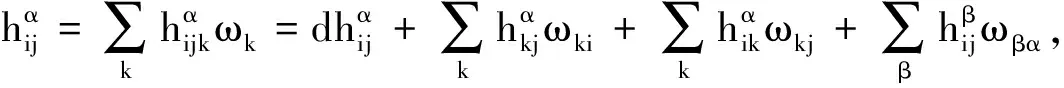
(2)

(3)

且有下列关系

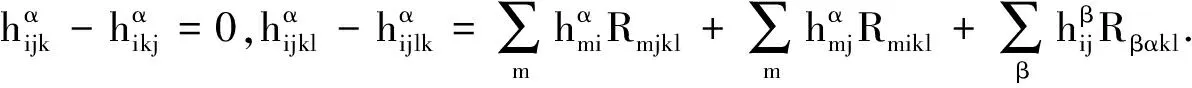
(4)

我们需要考虑Mn上二阶微分算子如下

(5)

其中fij是f在Mn上的二阶协变微分．

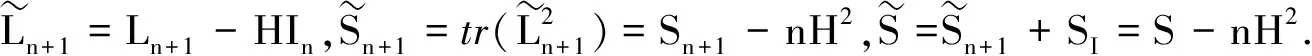
(6)

本文需要用如下一些基本引理．

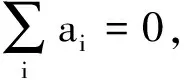
引理3当n≥3时，有

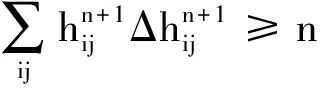
引理4当n≥2且p≥1时，有

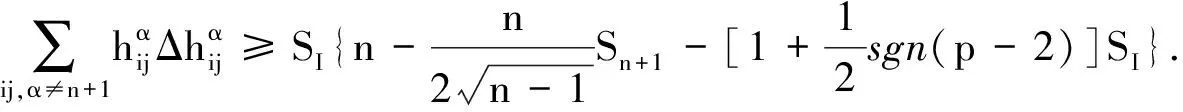
引理5当n≥8时，或当n≥3且p≤2时，有

证p≤2意味着诸Lα可以同时对角化.由此易得．

引理6当n=2,p≥1时，有

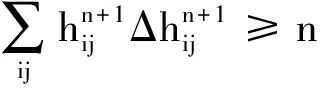
证n=2时，由引理4的不等式右边第4项小括号中可以算出．

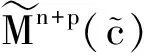
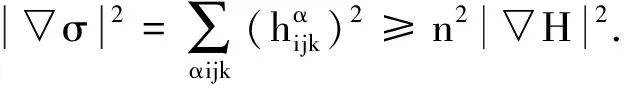
(7)

并且有如下结论．

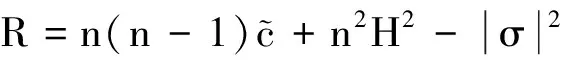
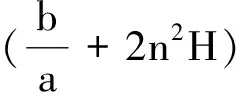
(8)

(8)式两边取模长平方并用(7)式和Schwartz不等式，可得

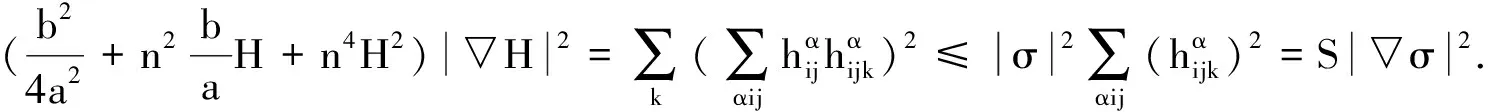
(9)

由(9)式和aR+bH=c可得

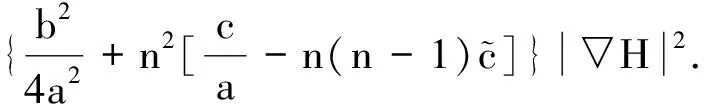
(10)

2 定理A的证明

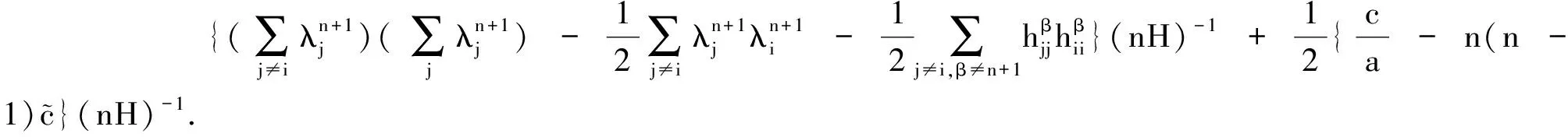
(11)

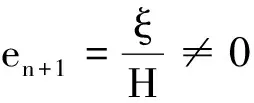
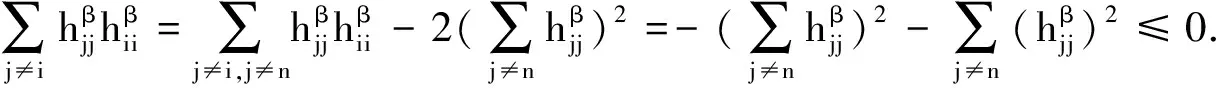
(12)

由式(11)和(12)有

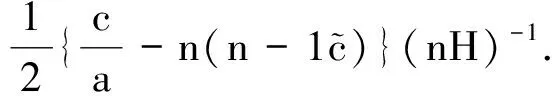
(13)

下面分3种情况讨论．

(1)当n≥8,p≥1时，或者当n≥3,p≤2时，由引理3和5易得

(2)当3≤n≤7时，由引理3和4易得

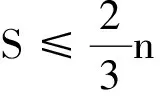
(3) 当n=2,p≤2时，由引理7和8易得

当n=2,p≥3时，由引理6和7易得

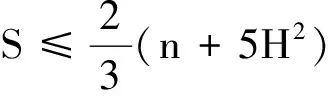
参考文献：

[1] YAU S T. Sub-manifolds with parallel mean curvature I [J].Amer J Math, 1974,96(2):346-366.

[2] YAU S T. Sub-manifolds with parallel mean curvature II [J].Amer J Math, 1975,97(1):76-100.

[3] SHEN Y B. Sub-manifolds with nonnegative sectional curvature [J].China Ann Math, 1984,5B(4):625-632.

[4] 林森春. 纯量曲率和平均曲率成线性关系的完备超曲面[J].数学年刊，1989，10A(3):333-344.

[5] ZHONG H H. Sub-manifolds of constant scalar curvature in a space form[J].Kyun Math J, 1998,38(3):438-458.

[6] LI A M, LI J M. An intrinsic rigidity theorem for minimal submanifolds in a sphere[J].Ark Math, 1992,58(3):582-594.

[7] LI H Z. Global rigidity theorems of hypersurfaces [J].Ark Math, 1997,35(2):327-351.

[8] CHERN B L, ZHU X P. Complete Riemannian manifolds with point-wise pinched curvature [J].Math Ann, 2003,327(1):1-23.

[9] BAO D, CHERN S S, CHERN Z. An introduction to Riemannian-Finsler geometry[M].New York: Springer-Verlag, 2000.

[10] ANDERSON M T, SCHOEN R. Positive harmonic functions on complete manifolds of negative curvature[J].Ann Math, 1985,121(3):429-461.

[11] CHENG S Y. On the upper estimate of the heat kernel of a complete Riemannian manifold[J].J Math, 1981,103(5):1021-1063.

[12] SPERB R P. Maximum principles and their applications[M].New York:Academic Press, 1981.

[13] GILBARG G, TRUDINGER N S. Elliptic partial differential equations of second order[M].2nd Ed. New York:Springer-Verlag, 1983.