关于可列非齐次马氏链的广义C-强遍历性

张艳,杨卫国

(江苏大学理学院,江苏 镇江 212013)

关于可列非齐次马氏链的广义C-强遍历性

张艳,杨卫国

(江苏大学理学院,江苏 镇江 212013)

马氏链遍历性理论在生物,数值计算,信息理论,自动控制,近代物理和公用事业中的服务系统等众多领域都有着广泛的应用,马氏链的C-强遍历性是马氏链遍历性理论的重要内容.本文给出了马氏链C-强遍历性的一个推广,首先给出了在可列状态空间取值的非齐次马氏链的广义C-强遍历性和广义一致C-强遍历性的概念,然后研究这两种遍历性成立的充分条件.

可列非齐次马氏链;广义C-强遍历性;广义一致C-强遍历性

1 引言

一个不可约周期为 d(d≥1)的随机矩阵 P把状态空间 S分为 d个不相交的子空间 C0,C1,…,Cd−1,并且 Pd派生出 d个随机矩阵 {Tl,0≤l≤d−1},其中 Tl定义在 Cl上.如果不可约周期矩阵 P有限,那么每一个 Tl都是强遍历的,如果不可约周期矩阵 P是可列的,Tl的强遍历性不能保证.我们只考虑不可约周期为 d的随机矩阵 P,对 l=0,1,…,d−1,Tl都是强遍历的,这样的随机矩阵称为周期性强遍历的(参见文献[3]).

关于马氏链遍历性有一些研究.文献[1]有很多关于强遍历和C-强遍历的研究.文献[2]研究了非齐次马氏链的Ces`aro平均收敛和收敛速率.文献[3]研究了可列非齐次马氏链在一致C-强遍历条件下的强大数定律.文献[4]研究了可列非齐次马氏链C-强遍历和一致C-强遍历.文献[5]研究了在一般状态空间取值的齐次马氏链的各种遍历性问题.文献[6]研究了可列非齐次马氏链在Ces`aro收敛条件下的一系列强极限定理.

本文的目的是研究可列非齐次马氏链广义C-强遍历和广义一致C-强遍历的充分条件.

2 主要结果

本节研究非齐次马氏链的广义C-强遍历和广义一致C-强遍历的充分条件.

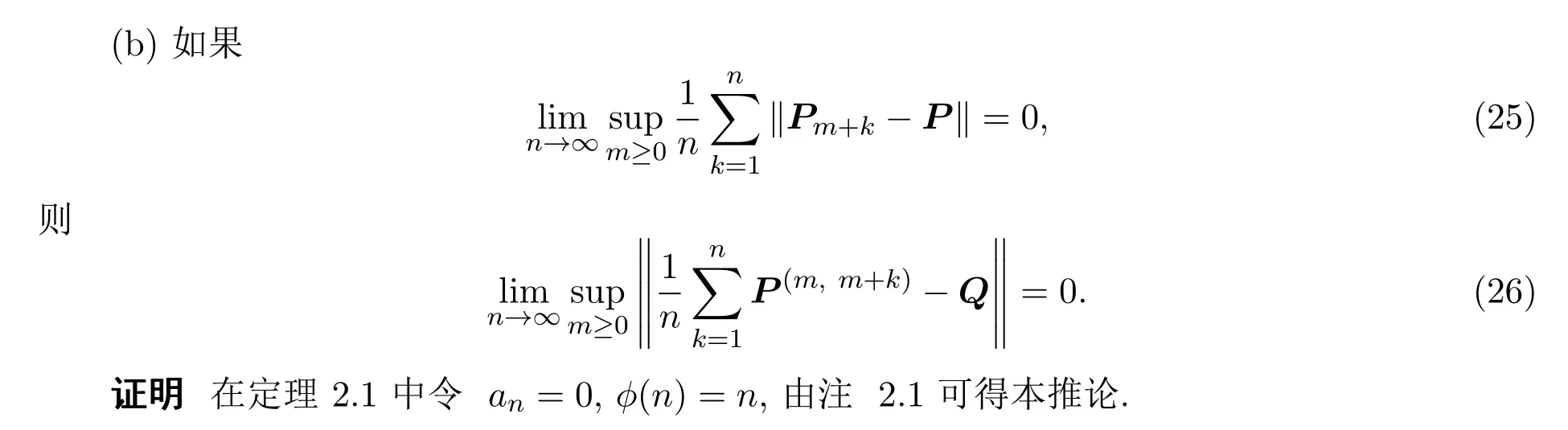
[1]Isaacson D,Madsen R.Markov Chains Theory and Application[M].New York:Wiley,1976.

[2]Yang W G.Strong law of large numbers for countable nonhomogeneous Markov chains[J].Linear Algebra Appl.,2009,430:3008-3018.

[3]Bowerman B,David H T,Isaacson D.The convergence of Ces`aro averages for certain nonstationary Markov chains[J],Stochastic Process.Appl.,1977,5:221-230.

[4]Yang W G.Covergence in the Cesaro sense and strong law of large numbers for nonhomogeneous markov chains[J].Linear Algebra Appl.,2002,354:275-288.

[5]Jarner S F,Roberts G O.Polynomial convergence rates of Markov chains[J].Ann.Appl.Probab., 2002,12:224-247.

[6]张弘知,郝瑞丽,叶中行,等.可列非齐次马氏链的强极限定理[J].应用概率统计,2016,32(1):62-68.

The generalized C-strong ergodicity for countable nonhomogeneous Markov chain

Zhang Yan,Yang Weiguo
(Faculty of science,Jiangsu University,Zhenjiang 212013,China)

The ergodicity for Markov has been widely used in the areas of biology,numerical calculate,information theory,automatic control,modern physics and service system of public enterprise.The C-strong ergodicity for Markov is an important part.This paper presents a generalization of C-strong ergodicity.We give the de fi nition of the generalized C-strong ergodicity and the generalized uniform C-strong ergodicity for countable nonhomogeneous Markov chain,and then we study the sufficient conditions of the two ergodicity.

countable nonhomogeneous Markov chains,generalized C-strong ergodicity,generalized Uniform C-strong ergodicity

O211.62

A

1008-5513(2017)01-0037-07

10.3969/j.issn.1008-5513.2017.01.005

2016-07-07.

国家自然科学基金(11571142).

张艳(1990-),硕士生,研究方向:马氏链极限理论.

2010 MSC:60F15