一类非线性三阶边值问题解的存在性
蒋志丽,杜 娟
(哈尔滨师范大学)
一类非线性三阶边值问题解的存在性
蒋志丽,杜 娟
(哈尔滨师范大学)
通过一个构造的方法来研究一类非线性三阶微分方程解的存在性,并且提出了在再生核空间中计算方程近似解的一种迭代方法,通过数值算例可以证明,此迭代方法是具有高精度的.
存在性;非线性三阶边值问题;再生核空间
0 引言
非线性三阶边值问题在物理学,工程学,生物学等各个领域起着重要作用. 例如参考文献[1-4].
在该文中,考虑如下一般的模型:

(1)
该文给出了一个存在性定理和一个简单的迭代方法,用来在再生核空间中计算方程(1)的近似解.
1 预备知识


其内积为:
(2)
(3)
易证以下两个引理.
引理1.1 对任意的正整数i,j.
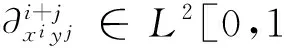
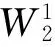
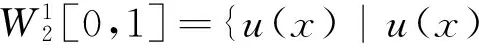
其内积为:

(4)
2 准备工作

(5)

(6)
其中βik是正交化系数.
由文献[5]知以下两个引理:
3 一种迭代方法
构造迭代序列un(x),令
(7)

证明 由上极限的定义可知,对∀ε>0,∃N>0,以及序列nk,使得当n>N时,





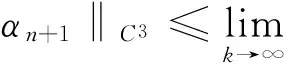
定理3.2 若对∀x∈[0,1],y,z,w∈


由(7)可得

vn+1‴(x)有界.
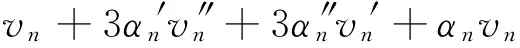

f (x,u(x),u′(x),u″(x)),n→∞

4 算例
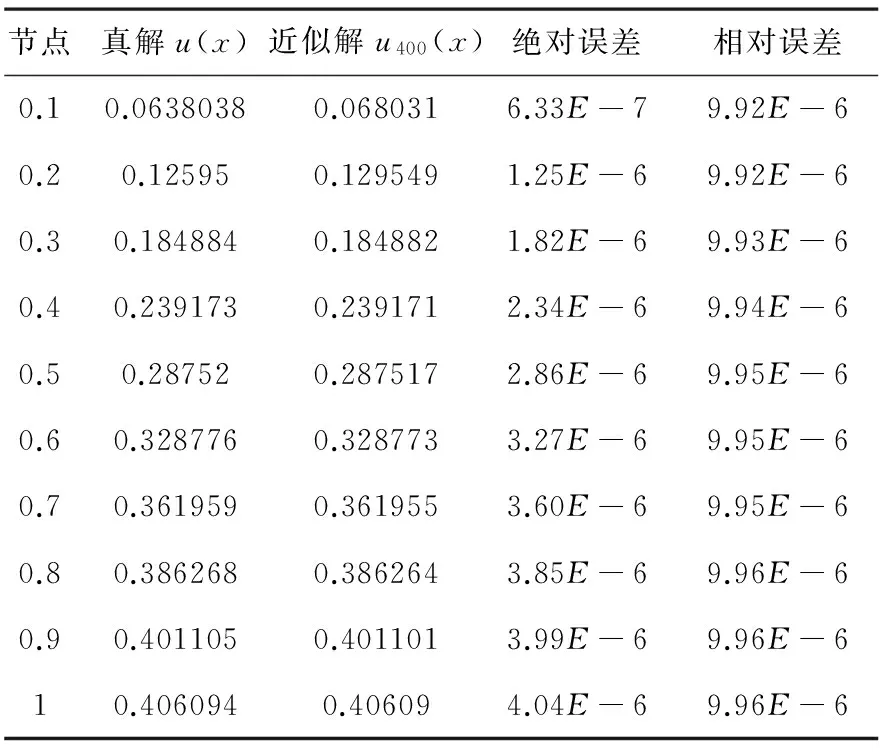
表1
[1] Aftabizadeh A R. Existence and uniquness theorems for fourth-order boundary value problems[J]. Math Anal Appl,1986, 116:415-426.
[2] 李敏.非线性二阶积分-微分解的构造性逼近[J].哈尔滨师范大学学报:自然科学版,2014,30(6):23-26.
[3] Gupta C P. Existence and uniquenness theorems for a bending of an elastic beam equation,Appl Anal 1988,26:289-304.
[4] Del Pino M A, Manasevich R F. Existence for a fourth-order nonlin-ear boundary value problem under a two parameter nonresonance condi-tion,Proc Amer Math Soc,1991, 112:81-86.
[5] Cui M G, Lin Y Z. Nonlinear numerical Analysis in the Reproducing kernel space.Nova Science Publisher,New York, 2008.
(责任编辑:于达)
Existence Results for a Third-order Nonlinear Boundary Value Problems
Jiang Zhili, Du Juan
(Harbin Normal University)
In this work, the existence of solution to a third-order nonlinear differential equations is investigated by a constructive method. In the meantime, a iterative method of computing approximate solution in the reproducing kernel space is presented. It’s demonstrated by numerical examples that this method is of high precision.
Existence; Third-order nonlinear boundary value problems; Reproducing kernel space
2016-01-06
O175
A
1000-5617(2016)02-0016-03

