一种高双折射低限制性损耗光子晶体光纤设计

赵丽娟，梁若愚，赵海英，徐志钮*

一种高双折射低限制性损耗光子晶体光纤设计

赵丽娟1,2,3，梁若愚1，赵海英1，徐志钮1*

1华北电力大学电气与电子工程学院，河北 保定 071003；2华北电力大学河北省电力物联网技术重点实验室，河北 保定 071003；3华北电力大学保定市光纤传感与光通信技术重点实验室，河北 保定 071003

本文设计了一种适用于长距离光纤通信的新型光子晶体光纤。该光纤包层内椭圆形和圆形空气孔呈交错排列，纤芯两侧为两个小椭圆空气孔。利用有限元分析方法对所设计光纤的传输特性进行分析并对其结构进行了优化，确定了最佳结构。结果表明，波长为1550 nm时，此新型光子晶体光纤在最佳结构下可提供高达3.51×10-2的高双折射和低至1.5×10-9dB/m的限制性损耗。与现存的引入椭圆形空气孔的光子晶体光纤相比，本文中的光子晶体光纤的双折射系数有较大提高，限制性损耗系数降低了5个数量级。另外，本文还详细研究了光子晶体光纤的色散随光子晶体光纤结构的变化以及其布里渊增益特性，并分析了其可制造性。基于其高双折射和低限制性损耗特性，此种光纤可应用于长距离光纤通信系统。

光子晶体光纤；高双折射；低限制性损耗；有限元分析法

1 引 言

光子晶体光纤(Photonic crystal fiber, PCF)是一种空气孔沿轴向周期性排列的，端面呈二维周期性分布的光子晶体结构[1]，又称为多孔光纤或微结构光纤，具有许多传统光纤无法实现的性能，近几年在行业内引起了极大的关注[2-4]。

与传统的光纤相比，光子晶体光纤具有许多优异特性，例如大负色散、非线性特性、限制性损耗和高双折射等。同时，双折射特性、限制性损耗以及色散特性是长距离光纤通信系统中三个重要的衡量标准。当光子晶体光纤的两个偏振轴之间的不对称程度越大，其双折射系数越大，传输过程中两个偏振轴之间的模式耦合程度会减小，有助于提升光信号的传输距离。同时，较低的限制性损耗也有助于提升光信号的传输距离。另外，色散会引起光脉冲的展宽，极大地限制了传输信道的容量和光纤的带宽[5]。因此，利用大负色散光纤进行色散补偿在高速度长距离光纤通信系统中也十分必要。

2011年，Yang等人[6]引入椭圆形空气孔，使得光子晶体光纤的双折射达到了0.87×10-2，限制性损耗为0.01 dB/m。2016年，Wu等人[7]设计的光子晶体光纤的双折射系数达到2.21×10-2。2017年，Liao等人[8]通过在纤芯引入椭圆空气孔得到了高达3.41×10-2的双折射系数和低至-399.98 ps·km-1·nm-1的色散值。2019年，Liu等人[9]设计的光子晶体光纤的限制性损耗有了新的突破，达到了10-6dB/m，其数量级有明显降低。刘旭安等人[10]提出了一种基于双空气孔单元四角晶格排列的光子晶体光纤，其双折射为10-2量级，限制性损耗为10-6dB/m量级。2020年，Agbemabiese等人[11]提出一种包层含圆形和椭圆形空气孔的光子晶体光纤，其双折射系数为2.018×10-2，限制性损耗为10-5dB/m量级。在目前现存的光子晶体光纤的设计中，空气孔的分布较为单一，大大降低其偏振轴之间的不对称性，影响双折射系数的进一步提高以及其他传输特性的优化，因此，已无法满足当今通信系统高速增长的需求。

本文设计的光子晶体光纤与已有光纤最明显的区别是本文光纤横截面内圆形与椭圆形空气孔交错排列。利用有限元方法系统地进行数值分析之后，得出了所设计光纤最优的结构参数，并且分析了其传输特性和布里渊增益谱特性。研究结果表明，在1550 nm处，此光纤在最佳结构下的双折射系数可达3.51×10-2，限制性损耗低至1.5×10-9dB/m，偏振轴具有平坦色散，偏振轴色散值最低可达到-500 ps·km-1·nm-1。偏振轴和偏振轴的布里渊频移分别为10.15 GHz和10.4 GHz。本文提出的光纤在光纤传感，制作保偏光纤以及长距离光纤通信系统等领域具有一定的应用价值。

2 光纤设计及基本理论

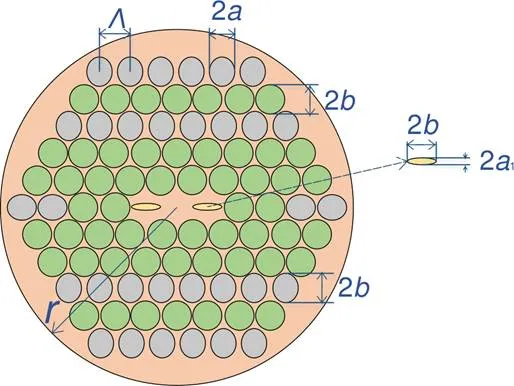
光纤的横截面示意图如图1所示。椭圆形空气孔与圆形空气孔呈交错排列，孔间距为。图中灰色区域为椭圆形空气孔，短半轴和长半轴分别用和表示；绿色区域为半径为的圆形空气孔；围绕纤芯的黄色区域为两个小椭圆空气孔，其短半轴和长半轴分别为1和，椭圆率用=1/表示；光纤半径为。

图1 光纤横截面

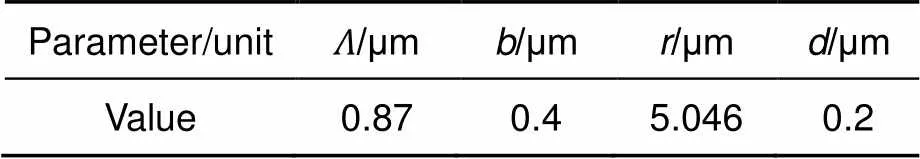
表1 光纤结构及完美匹配层参数

在利用有限元分析法对建立的模型进行计算时，添加了一层厚度为的完美匹配层(perfectly matched layer，PML)作为边界吸收条件，使计算结果更加精确。经过系统地数据分析，最终确定的部分结构参数如表1所示。

3 光纤特性分析

本文仿真模拟基于表1中的光纤结构参数。在本小节中取1=0.35 μm，=0.2。图2为该结构下光纤和偏振轴的模场分布。从图2可以看出，偏振轴和偏振轴的模场分布分别呈现出椭圆形和矩形形状，其模式电场关于轴和轴的分布失去了极轴对称性，导致了该光子晶体光纤高双折射系数的产生，不对称性越高，光纤模式双折射越高。

3.1 双折射系数

有效折射率(eff)是一个定量描述波导中单位长度相位延迟的量，随着波长的增加，光束的场分布逐渐扩展到包层区域，有效折射率随之减小，如图3所示。

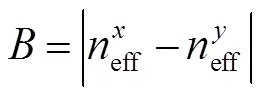
当发生变化时，双折射系数也会随之发生改变，其变化趋势如图4所示。

如图3、图4所示，当小于0.4 μm，波长小于1800 nm时，同波长下偏振轴有效折射率和双折射系数的差异较小。图3中子图部分为波长在1600 nm~2000 nm，在0.2 μm~0.35 μm范围内变化时光纤、偏振轴有效折射率的标准差，从图中可以看出，在1800 nm后光纤偏振轴的有效折射率出现了一定程度的变化，而偏振轴差异较小，因此双折射系数差异增大。而当=0.4 μm时，光纤双折射系数有明显下降，是因为此时椭圆孔全部呈现圆形，降低了偏振轴和偏振轴的非对称性。

当小于0.4 μm时其值的大小对光纤特性影响较小，而偏大时，其制造难度较低。计算表明在其他参数保持最优的情况下，=0.35 μm接近最优值。接下来我们仅优化，PCF的其他参数如表1所示。

图5显示了在典型值=0.2、0.6和0.8时双折射系数随波长的变化情况，其中1分别对应于0.08 μm，0.24 μm和0.32 μm。此外，在波长为1550 nm处标注了参考文献[8，12-15]中典型PCF的双折射系数值以与本文提出的光纤结构双折射系数值进行对比。如图5所示，双折射系数随着的减小而增加，当波长为1550 nm，=0.2时获得了3.51×10-2的高双折射。同时从图5中可以看出，与文献[8，12-15]中设计的光子晶体光纤相比，本文所提出的PCF结构在=0.2时具有最高的双折射系数，这是因为所提出的空气孔交错分布结构大大提高了两偏振轴之间的不对称性，高双折射光子晶体光纤可以有效减少光信号传输过程中两个偏振轴之间的能量耦合，有助于增加光纤通信系统的传输距离[16-18]。

图2 η=0.2时光纤LP01模式模场分布和能量等值线分布图。(a), (b) x偏振轴；(c), (d) y偏振轴

图3 光纤有效折射率随a的变化

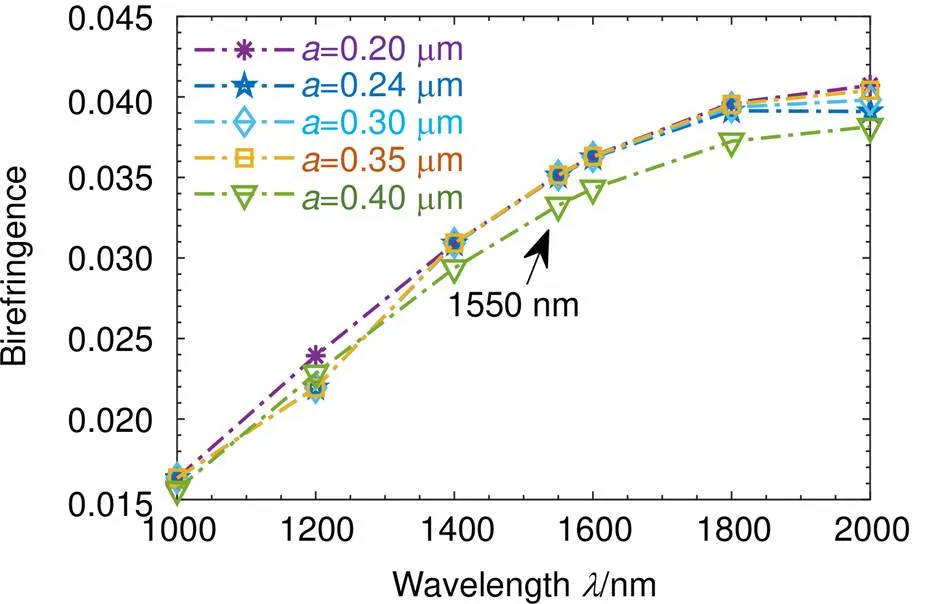
图4 光纤双折射系数随a的变化

图5 不同η下PCF的双折射系数 随波长的变化

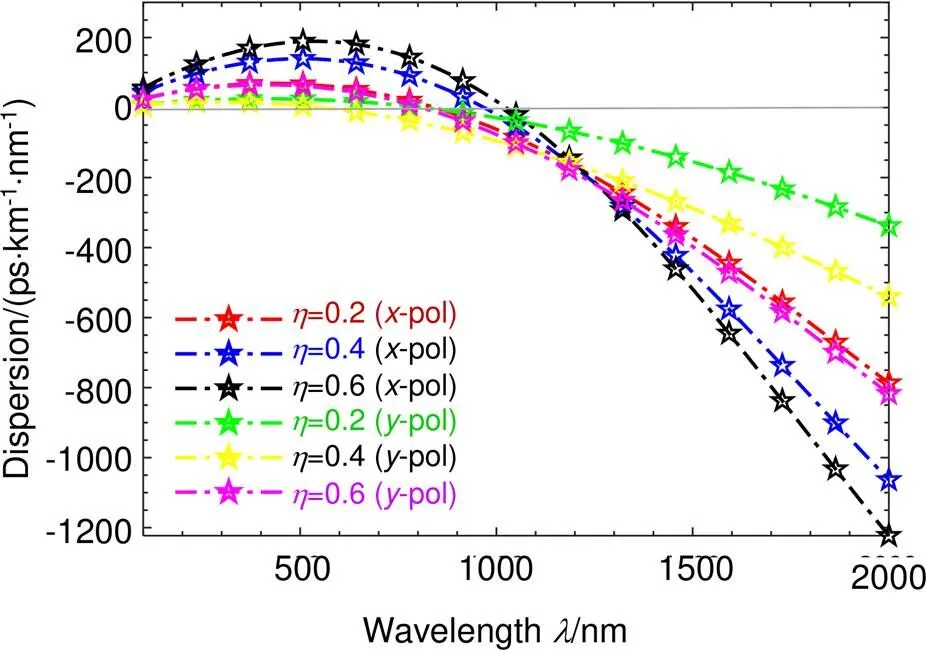
图6 光纤色散随波长的变化

3.2 色散

PCF的色散包括材料色散和波导色散[19]。在有限元计算过程中已经包括了材料色散，因此本文仅计算波导色散，其计算式如下[20]：

其中：是波长，是真空中的光速，Re(eff)是模式有效折射率的实部。本文设计的PCF色散在不同下随波长变化情况如图6所示。

在光纤通信系统中，光纤色散会引起传输信号的畸变，使得通信质量下降，限制通信容量和通信距离，在通信系统中加入适当长度具有较低负色散的色散补偿光纤可以改善色散对通信系统的影响；而色散平坦在超连续谱产生中发挥着重要作用，因此在光通信传输中的光纤设计可以综合考虑色散平坦与负色散两种特性。图6是根据仿真计算结果得到的色散随波长变化的曲线，当在0.2~0.6范围内变化时，其、偏振轴的零色散波长大概在600 nm~1100 nm范围内变化。当＝0.2时，偏振方向的色散值可低至约-500 ps·km-1·nm-1；在偏振方向也可以提供相对平坦的负色散，同时具有零色散平坦的特性，近零平坦色散有利于实现非线性光学中的相位匹配、孤子脉冲的产生和传输以及超宽且平坦超连续谱的产生[5]；当＝0.4时，其具有比＝0.2时更低的色散，但是其色散平坦度较差，不具有零色散平坦的特性；当＝0.6时，其色散平坦度也较差。因此，本文设计的PCF在＝0.2时同时有大负色散以及零色散平坦的特性，可用作高速率长距离传输系统中的色散补偿光纤元件，在超连续谱产生方面也具有重要的应用价值。

3.3 限制性损耗

限制性损耗是由PCF结构引起的，在光信号传输过程中，光并不能完全束缚在纤芯进行传输。因此，限制性损耗可认为是由光的泄漏引起，表示为

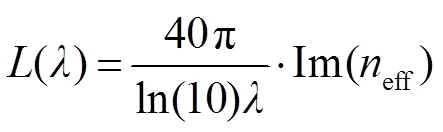
光纤限制性损耗的大小直接影响传输距离以及中继站间隔距离的远近。从图7可以看出，当波长一定时，限制性损耗随的增加而增大。在1550 nm处，=0.2时，偏振轴的限制性损耗约为1.5×10-9dB/m，偏振轴的限制性损耗约为4×10-9dB/m。与文献[16]相比降低了5个数量级，因此，它具有更优异的传输性能，可以延长通信系统传输距离。

从图4至图7可知，当=0.2时，双折射系数最高，负色散较为平坦且限制损耗最小，具有更好的性能。另外，制造难度随着减小而增加。因此，我们可以确定=0.2为光纤包层中小椭圆空气孔的最佳结构。

3.4 布里渊增益

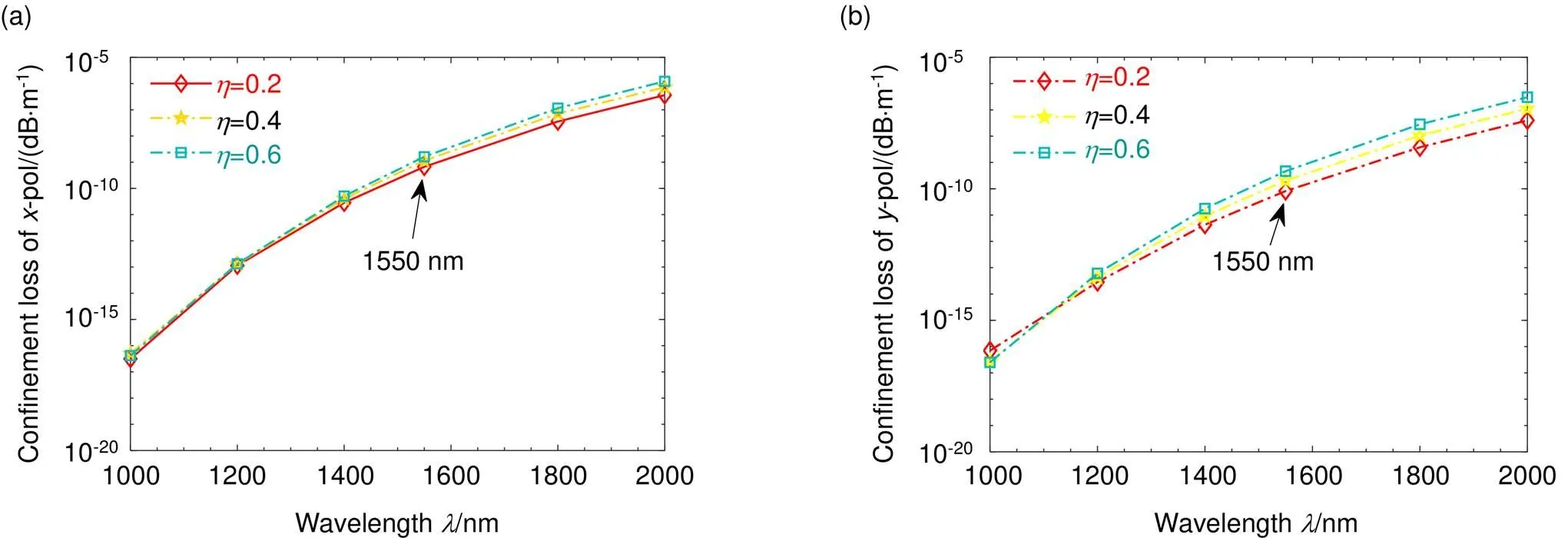
图7 光纤限制性损耗随波长的变化。(a) x偏振轴；(b) y偏振轴

图8 光子晶体光纤LP01模式的声场分布

图9 光子晶体光纤的布里渊增益谱

基于确定的光纤最佳结构，分析光子晶体光纤的布里渊增益频谱特性。每个声学模式对应一个布里渊峰。布里渊增益与声学模式的关系可定义为[22]

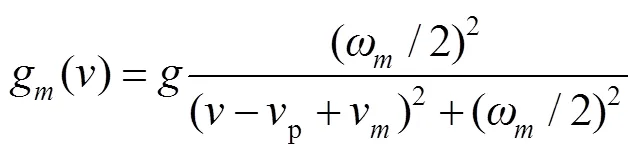
其中：和p分别是散射光和入射光的频率；v和ω分别是布里渊散射谱中第个峰的布里渊频移和线宽；是第个布里渊增益谱的峰值。

图8为LP01模式的声场分布，从图中可以看到声波的能量集中于纤芯分布，由于光场和声场之间有相互作用，从而可以形成布里渊增益谱中的峰。

图9为本文设计的光子晶体光纤的布里渊增益谱。从图9中可以看出，偏振方向的布里渊频移小于偏振方向的布里渊频移，其布里渊频移分别为10.15 GHz和10.4 GHz。在实际应用当中，根据布里渊频移可直接算出声速，由声速可以算出弹性常数，由声速的变化可得到关于声速的各向异性、弛豫过程和相变的信息等；由线宽可以研究声衰减过程。另外，在分布式光纤传感系统中，通过测量布里渊频移的变化能实现对光纤所处环境温度与应变的传感[23-26]，本节为所设计的PCF的进一步应用提供了理论基础。

3.5 与现有光子晶体光纤的性能比较

表2为所提出的PCF与已有设计之间的双折射系数()，色散()和限制损耗(())的比较。结果表明，本文提出的PCF性能有显著提高，在高速率和长距离传输系统领域中具有潜在的应用。

3.6 制造可行性分析

近年来，在光子晶体光纤制造[29]方面，Sol-gel浇铸法已变得越来越流行，它可以灵活地改变包层中空气孔的形状、大小和间距，而不会产生空隙。该方法先将熔融石英浇注到根据包层结构设计的模具中来制造纤维预制件，然后将预制件放入光纤拉伸器中进行拉制[30]。此方法为我们提出的结构的制造带来了解决方案。然而，在拉制过程中圆形气孔直径仍不可避免地会有1%~2%的误差。图10显示了制造偏差对光纤双折射系数的影响。从图中可以看出，圆形气孔直径的变化对所设计光纤的双折射系数的影响很小，因此降低了对制造精度的要求，大大提高了其可制造性。

4 结 论

本文设计了一种新型光子晶体光纤结构，包层中大椭圆形和圆形气孔序列交错分布，纤芯周围有两个小椭圆形气孔。通过有限元方法系统地研究了其传输特性。基于详细的数值分析讨论了小椭圆空气孔椭圆率对光纤性能的影响，得到了PCF的最佳参数。本文的结论如下：

1) 当波长为1550 nm时，基于最佳参数的光纤双折射系数和限制性损耗分别为3.51×10-2和1.5×10-9dB/m。与现有研究结果相比，所提出的PCF的双折射增加了0.97×10-2，限制性损耗降低了大约5个数量级，大大提高了光信号在通信系统中的传输距离。

2)=0.2时，PCF的偏振轴可以提供较平坦的大负色散，偏振轴的色散可低至-500 ps·km-1·nm-1，可用于制造色散补偿元件，有效降低脉冲展宽对信道容量及带宽的限制。

3) 最佳结构下，光子晶体光纤和偏振方向的布里渊频移分别约为10.15 GHz和10.4 GHz。

综上所述，本文设计的PCF可以用于高速率长距离传输通信系统领域，并在光纤传感领域具有潜在的应用前景。

表2 与现有光子晶体光纤的性能比较

图10 当圆空气孔直径(2b)有1%~2%的误差时 双折射系数随波长的变化

[1] Steel M J, Osgood R M. Polarization and dispersive properties of elliptical-hole photonic crystal fibers[J]., 2001, 19(4): 495–503.

[2] Wang X Y, Cui Y Z, Bi W H,. Research on control method of Graphene layers grown in air holes of photonic crystal fiber based on Raman spectroscopy[J]., 2020, 40(12): 3659–3664. 王晓愚, 崔永兆, 毕卫红, 等. 基于拉曼光谱的光子晶体光纤孔内生长石墨烯层数控制方法的研究[J]. 光谱学与光谱分析, 2020, 40(12): 3659–3664.

[3] Robert P, Fourcade-Dutin C, Dauliat R,. Spectral correlation of four-wave mixing generated in a photonic crystal fiber pumped by a chirped pulse[J]., 2020, 45(15): 4148–4151.

[4] Wei F H, Zhang X J, Tang S F. Design and analysis of photonic crystal fiber refractive index sensor based on surface Plasmon resonance[J]., 2020, 41(1): 35–38, 43. 魏方皓, 张祥军, 唐守锋. 基于表面等离子体共振的光子晶体光纤折射率传感器的设计与分析[J]. 半导体光电, 2020, 41(1): 35–38, 43.

[5] Wei H Y, Pei X N. Design of near-infrared zero-dispersion flattened all-solid microstructured optical fiber[J]., 2018, 42(10): 41–45. 魏红彦, 裴小娜. 近红外零色散平坦全固态微结构光纤的设计[J]. 光通信技术, 2018, 42(10): 41–45.

[6] Yang K Y, Chau Y F, Huang Y W,. Design of high birefringence and low confinement loss photonic crystal fibers with five rings hexagonal and octagonal symmetry air-holes in fiber cladding[J]., 2011, 109(9): 093103.

[7] Wu L M, Song P, Wang J,. A squeezed lattice high negative dispersion and high birefringence photonic crystal fiber[J]., 2016, 45(S1): S120001. 武丽敏, 宋朋, 王静, 等. 一种高双折射高负平坦色散压缩型光子晶体光纤[J]. 红外与激光工程, 2016, 45(S1): S120001.

[8] Liao J F, Huang T Y, Xiong Z Z,. Design and analysis of an ultrahigh birefringent nonlinear spiral photonic crystal fiber with large negative flattened dispersion[J]., 2017, 135: 42–49.

[9] Liu Q, Liu Q Y, Sun Y D,. A high-birefringent photonic quasi-crystal fiber with two elliptical air holes[J]., 2019, 184: 10–15.

[10] Liu X A, Cheng H P, Jiao Z. Properties of regular-lattice photonic crystal fiber based on a double-hole unit[J]., 2019, 43(1): 48–52. 刘旭安, 程和平, 焦铮. 双孔单元四边形晶格光子晶体光纤特性的研究[J]. 激光技术, 2019, 43(1): 48–52.

[11] Agbemabiese P A, Akowuah E K. Numerical analysis of photonic crystal fiber of ultra-high birefringence and high nonlinearity[J]., 2020, 10(1): 21182.

[12] Liu M, Hou J Y, Yang X,. Design of photonic crystal fiber with elliptical air-holes to achieve simultaneous high birefringence and nonlinearity[J]., 2018, 27(1): 014206.

[13] Yang T Y, Wang E L, Jiang H M,. High birefringence photonic crystal fiber with high nonlinearity and low confinement loss[J]., 2015, 23(7): 8329–8337.

[14] Sonne A, Ouchar A, Sonne K. Improving of high birefringence with negative dispersion using double octagonal lattice photonic crystal fiber[J]., 2016, 127(1): 8–10.

[15] Gao Y, Sima C, Cheng J,. Highly-birefringent and ultra-wideband low-loss photonic crystal fiber with rhombic and elliptical holes[J]., 2019, 450: 172–175.

[16] Prajapati Y K, Kumar R, Singh V. Design of a photonic crystal Fiber for dispersion compensation and sensing applications using modified air holes of the cladding[J]., 2019, 49(5): 745–751.

[17] Li Y Q, Zhang L X, Fan H B,. A self-heterodyne detection Rayleigh Brillouin optical time domain analysis system[J]., 2018, 427: 190–195.

[18] Rong G H, Yi X S. Investigation on a new high birefringence photonic crystal fiber[J]., 2018, 39(2): 211–215. 荣耕辉, 伊小素. 一种新型高双折射光子晶体光纤的特性研究[J]. 半导体光电, 2018, 39(2): 211–215.

[19] Zhang X D, Yuan M M, Chang M,. Characteristics in square air hole structure photonic crystal fiber[J]., 2018, 45(5): 170633. 张学典, 袁曼曼, 常敏, 等. 正方形空气孔光子晶体光纤特性分析[J]. 光电工程, 2018, 45(5): 170633.

[20] Chen N, Zhang X D, Nie F K,. Dispersion-compensating photonic crystal fiber with wavelength tunability based on a modified dual concentric core structure[J]., 2018, 65(12): 1459–1465.

[21] Yang T Y, Jiang H M, Wang E L,. Photonic crystal fibers with large birefringence and high nonlinearity in near-infrared band[J]., 2016, 35(3): 350–354.杨天宇, 姜海明, 王二垒, 等. 一种近红外波段的高双折射高非线性光子晶体光纤[J]. 红外与毫米波学报, 2016, 35(3): 350–354.

[22] Pan Y H, Lu Y G, Pen J Q,. Brillouin gain spectrum characteristics of photonic crystal fibers[J]., 2019, 39(6): 0619001. 潘宇航, 路元刚, 彭楗钦, 等. 光子晶体光纤的布里渊增益谱特性[J]. 光学学报, 2019, 39(6): 0619001.

[23] Bao X Y, Chen L. Recent progress in distributed fiber optic sensors[J]., 2012, 12(7): 8601–8639.

[24] Xu Z N, Hu Y H, Zhao L J,Fast and highly accurate Brillouin frequency shift extracted algorithm based on modified quadratic polynomial fit[J]., 2020, 40(3): 842–848. 徐志钮, 胡宇航, 赵丽娟, 等. 基于改进二次多项式拟合的布里渊频移快速高精度提取算法[J]. 光谱学与光谱分析, 2020, 40(3): 842–848.

[25] Xu Z N, Hu Y H, Zhao L J,. Rapid temperature and strain measurement method for optic-electric composite submarine cable based on slope-assisted method[J]., 2020, 40(5): 202–208. 徐志钮, 胡宇航, 赵丽娟, 等. 基于单斜坡法的光电复合海缆温度、应变快速测量方法[J]. 电力自动化设备, 2020, 40(5): 202–208.

[26] Sheng Y, Lu J, Yao G F,. Analysis of high birefringent and confinement loss of elliptical air-holes rectangular photonic crystal fiber[J]., 2014, 43(S1): 0106008. 盛勇, 陆驹, 姚高峰, 等. 椭圆空气孔矩形结构光子晶体光纤的高双折射及限制损耗分析[J]. 光子学报, 2014, 43(S1): 0106008.

[27] Zhang W, Li S G, Bao Y J,. A design for single-polarization single-mode photonic crystal fiber with rectangular lattice[J]., 2016, 359: 448–454.

[28] Pan C, Zhou J P, Ni H B. Colloidal photonic crystal modified optical fiber and relative humidity detection application[J]., 2018, 45(9): 180168. 潘超, 周俊萍, 倪海滨. 胶体光子晶体修饰光纤及相对湿度检测应用[J]. 光电工程, 2018, 45(9): 180168.

[29] Sun C Y, Wang W C, Jia H Z. A squeezed photonic crystal fiber for residual dispersion compensation with high birefringence over S+C+L+U wavelength bands[J]., 2020, 458: 124757.

Design of a photonic crystal fiber with low confinement loss and high birefringence

Zhao Lijuan1,2,3, Liang Ruoyu1, Zhao Haiying1, Xu Zhiniu1*

1School of Electrical and Electronic Engineering, North China Electric Power University, Baoding, Hebei 071003, China;2Hebei Key Laboratory of Power Internet of Things Technology, North China Electric Power University, Baoding, Hebei 071003, China;3Baoding Key Laboratory of Optical Fiber Sensing and Optical Communication Technology, North China Electric Power University, Baoding, Hebei 071003, China

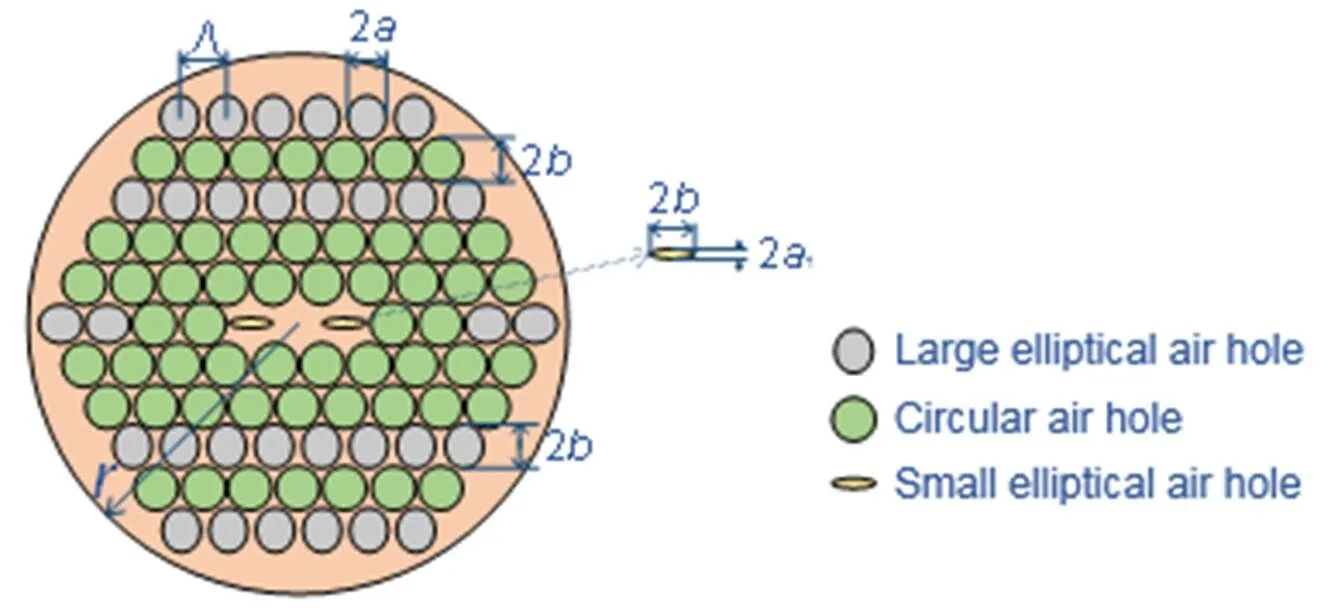
ection of the proposed PCF

Overview:Optical fiber communication is a system in which the light waves are used as the information carriers and the optical fibers are used as the transmission media. Optical fiber is more excellent than the transmission of cable and the microwave communication due to its wide transmission band, high anti-interference ability and low confinement loss, and has become the main transmission method. At the same time, with the development of communication technology, optical fiber communication systems have higher requirements for the performance of optical fibers. Traditional single-mode fibers can no longer meet the demands. Compared with conventional fibers, photonic crystal fibers (PCFs) have many unique characteristics, including no cut-off single mode transmission, highly tunable dispersion, excellent nonlinear effect, birefringence effect and so on. Therefore, PCFs have attracted considerable interests in recent years. In 2011, K. Yang proposed a PCF with elliptical air holes distributing on the inner rings. The birefringence of this PCF achieves 0.87×10-2, and the confinement loss is 0.01 dB/m. In 2016, the birefringence of the PCF designed by L. Wu reaches 2.21×10-2. In 2017, by introducing the elliptical air holes in the core, a birefringence of 3.41×10-2and a dispersion of -608.93 ps·km-1·nm-1was obtained by J. Liao. In 2019, the birefringence of the PCF with two elliptical air holes in the core proposed by Q. Liu is 1.4207×10-2, and the order of the confinement loss achieves 10-4dB/m. However, the existing studies with simple arrangement of air holes in the cladding show poor asymmetry, affecting the further improvement of the properties such as the birefringence and the confinement loss, so it could not meet the rapidly growing demands for optical fiber communication.

To fix the above problems, we proposed a novel PCF for long distance communication with crossly distributed elliptical and circular air holes sequences in the cross-section in this paper. The birefringence and confinement loss of the proposed PCF were systematically analyzed by using of the full vector finite element method. Then, we obtained the optical structure parameters by systematically numerical analysis and explored the Brillouin gain spectrum characteristics of the PCF. The results reveal that the proposed PCF offers an ultrahigh birefringence of 3.51×10-2with the confinement loss as low as 1.5×10-9dB/m for the optimal structure of the PCF at the wavelength of 1550 nm, and the Brillouin frequency shift of- and-polarization are about 10.15 GHz and 10.4 GHz respectively. In addition, the PCF proposed in this work may be helpful for applications in the field of fiber optical sensing, the polarization-maintaining fiber, and the long-distance transmission of optical signal.

Zhao L J, Liang R Y, Zhao H Y,Design of a photonic crystal fiber with low confinement loss and high birefringence[J]., 2021, 48(3): 200368; DOI:10.12086/oee.2021.200368

Design of a photonic crystal fiber with low confinement loss and high birefringence

Zhao Lijuan1,2,3, Liang Ruoyu1, Zhao Haiying1, Xu Zhiniu1*

1School of Electrical and Electronic Engineering, North China Electric Power University, Baoding, Hebei 071003, China;2Hebei Key Laboratory of Power Internet of Things Technology, North China Electric Power University, Baoding, Hebei 071003, China;3Baoding Key Laboratory of Optical Fiber Sensing and Optical Communication Technology, North China Electric Power University, Baoding, Hebei 071003, China

A photonic crystal fiber (PCF) for long distance communication was proposed in this paper. The circular and elliptical air holes distribute in the cladding, and there are two small elliptical air holes around the core in cross section of the PCF. The characteristics of the PCF were analyzed by using the finite element method (FEM) systematically. The results show that the PCF offers an ultrahigh birefringence of 3.51×10-2and the confinement loss as low as 1.5×10-9dB/m with the optimal structure at the wavelength of 1550 nm. Compared with the existing photonic crystal fibers with elliptical air holes, the birefringence has a large increase, and the confinement loss reduces by 5 orders of magnitude. Additionally, we also analyzed the relationship between the dispersion of the PCF and the wavelength, and obtained the Brillouin gain spectrum characteristics. In general, the PCF can be used in long distance communication system.

photonic crystal fiber; high birefringence; low confinement loss; finite element method

National Natural Science Foundation of China (51607066, 61775057), the Natural Science Foundation of Hebei Province (E2019502177), and the Fundamental Research Funds for the Central Universities (2019MS085)

10.12086/oee.2021.200368

TN818

A

* E-mail: wzcnjxx@sohu.com

赵丽娟，梁若愚，赵海英，等. 一种高双折射低限制性损耗光子晶体光纤设计[J]. 光电工程，2021，48(3): 200368

Zhao L J, Liang R Y, Zhao H Y,Design of a photonic crystal fiber with low confinement loss and high birefringence[J]., 2021, 48(3): 200368

2020-10-12；

2021-02-05

国家自然科学基金资助项目(51607066, 61775057)；河北省自然科学基金资助项目(E2019502177)；中央高校基本科研业务费专项资金项目(2019MS085)

赵丽娟(1981-)，女，博士，副教授，主要从事光纤传感与光纤通信的研究。E-mail：hdzlj@126.com

徐志钮(1979-)，男，博士，副教授，主要从事分布式光纤传感及其在电气设备状态监测和故障诊断中的应用的研究。E-mail：wzcnjxx@sohu.com

版权所有©2021中国科学院光电技术研究所