Wave propagation analysis for a second strain gradient rod theory

Gung ZHU, Christophe DROZ, Adelmlek ZINE, Mohmed ICHCHOU,*

a Vibroacoustics & Complex Media Research Group, LTDS - CNRS UMR 5513, Centre Lyonnais d’Acoustique CeLyA,Ecole Centrale de Lyon, Universite de Lyon, France

b Institute Camille Jordan - CNRS UMR 5208, Ecole Centrale de Lyon, France

KEYWORDS Dynamic behavior;Energy flow;Enriched rod model;Reflection and transmission;Second strain gradient theory;Wave propagation features

Abstract In this work, an enriched model describing the longitudinal wave propagation is established based on Mindlin’s Second Strain Gradient(SSG)theory,which can describe the heterogeneity caused by the micro-structure interactions in the frame of continuum mechanics.The governing equation and associated boundary conditions are derived based on Hamilton’s principle, then the dispersion relation of non-classical longitudinal wave together with the extra-waves appearing exclusively in SSG theory model are investigated. The investigations are based on the modal density,energy flow,and forced response of the rod.Wave transmission and reflection through planar interfaces based on the proposed model have been calculated. Finally, the results of the enriched model are well interpreted by comparing with the classical theory results, and some useful conclusions are derived on the SSG theory based model in the wave propagation characterization.

1. Introduction

In conventional continuum mechanics theory, the strain energy density for the material is assumed to depend only on the classical strain,and the material is modeled to be a continuous mass rather than as discrete particles.In reality,no material is an ideal continuum. Both natural and man-made materials have a complicated internal structure characterized by micro-structural details.Hence,it is not possible to describe the more complex interactions occurring in generalized continua by means of the sole Cauchy stress tensor.1This is particularly true for the long-range interactions and microstructural deformations, which leads to the failing of conventional theory in many situations.2One solution is to describe each micro-particle separately with classical continuum theory as in atomistic models,but it requires enormous time and computational resource, moreover, the numerical simulation of large structures with high mechanical and geometrical contrasts is also facing to ill-conditioned problems.

To bridge the gap between atomistic models and classical continuum mechanics, a number of theories and approaches have been developed as extensions of the conventional continuum mechanics.Among them,‘Couple Stress theory’(CS theory) was firstly suggested in 1962 by Toupin et al.3,4In CS theory, the gradient of the rotation vector is introduced into the strain energy density function. Subsequently, the ‘Strain Gradient theory’ (SG theory) was developed by introducing all the components of strain gradient into the strain energy density function.5In 1965, Mindlin6established the ‘Second Strain Gradient theory’ (SSG theory), in which the strain energy density is considered to depend on the second and third derivatives of the displacement, along with the classical strain tensor.In 2002,Modified Couple Stress(MCS)theory is developed with an additional equilibrium relation to govern the behavior of the ‘couple’.7,8Another approach to describe the micro-structural deformation effect is by increasing the degrees of freedom of the practices.9,10,11In this catalogue, the micropolar theory and the micro-stretch theory, investigated by Eringen12,13, a location vector and a rigid vector representing the inner rotation are introduced.Generalized elasticity theory formulations enrich the classical elasticity theory by means of additional higher order spatial derivatives of relevant state variables (e.g. strains, stresses). These higher order terms are usually accompanied with additional length scale parameters(or higher order constants)in order to measure the scale effects which results from the underlying micro-structure interactions.14

The generalized mechanic theory already has some successful applications, namely, being capable of capturing the scale effects of experimentally observed mechanics behaviors where the specimen characteristic length or the wavelength of the disturbance are comparable to the lengths of the micro-structure in the media.15To be specific,the enriched model can be developed with the utilization of Hamilton principle, and the derived models are employed to investigate a broad range of problems related to various beam and plate structures.16,17,18One can cite the static deflection and bending stiffness analyses,19,20fracture behaviors,21,22structural free vibration and forced vibration,23,24,25nonlinear deformation and nonlinear dynamic performances,26,27,28flexoelectricity property29and functionally graded structure property analysis30,31, etc.

Furthermore, these generalized continuum theories introduce different deformation behaviors for the particles and also more complex modal solutions. A known consequence is the existence of new wave modes which could not be observed in the classical elastic solids.Recent work by Suiker et al.31,32predicted ‘dispersive’ body waves based on the proposed second gradient micro-polar formulation. When the wavelength reaches the order the particle size, the dispersion becomes more prominent. Wave propagation characteristics are also investigated by Gopalakrishnan33using the Erigen’s Stress Gradient model and Mindlin’s Strain Gradient model, as well as by Li et al.34with nonlocal strain gradient theory.Atomistic length scale parameters are brought into the continuum governing equations. These scale parameters are proven to significantly affect the wave propagation referring to not only the dispersion relation,but also the escape frequency,phase speeds and group speeds in the structures,and the prediction results34 match very well with those predicted using atomistic simulation.

The micro-structure effect not only lead to different body wave modes in the solid but also cause different waves reflection and transmission on a discontinuous surface of generalized continua. In the context of generalized continua, Placidi et al.35studied compression and shear wave propagation in second gradient continua. They noticed that the effect of the second gradient parameter are important for the reflection and transmission coefficients at surfaces of discontinuity. Dell’Isola et al.36deduced the mechanical energy equilibrium for second gradient material, based on which they estimated that the reflection and transmission coefficients at plane displacement discontinuity surfaces are significantly frequencydependent. According to the research of Li et al.,37,38the reflection and transmission coefficients at the interface of two generalized medias are not only dependent of the microstructure’s parameters, but also of the incident angular frequency.This phenomenon is owing to the dispersive nature of the reflected and transmitted waves, and also to the additional surface waves resulting from the micro-structure effect,and this phenomenon only becomes pronounced when the incident wavelength is close to the characteristic length of the micro-structure.

Mindlin’s SSG theory is considered as one of the most effective gradient elasticity theories. Even though the lack of available experimental measurement techniques for second gradient elastic moduli and being short of the physical interpretation of the higher order constants yield some criticisms,one can note that the CS theory and SG theory are particular cases of the SSG theory with less high order constants.Therefore,this theory is more general than the CS and SG theories.Furthermore, the research works involving both the SSG theory and wave propagation are seldom in the published literature. The most detailed wave propagation features such as the modal density, the energy flux, have not been discussed,and the reflection and transmission waves based on SSG theory at an interface have not reported so far.

To study the wave propagation features of rod structure having heterogeneity caused by inner micro-structure interactions,in the meantime avoid the enormous time and computational resource that atomistic simulation method requires,in the present work,we resort to SSG theory to describe the heterogeneity caused by the micro-structure interactions in the frame of continuum mechanics. The governing equation and associated boundary conditions for the enriched model are derived based on Hamilton’s principle in Section 3.1.Then the enriched wave propagation features of the structure are studied in Section 3.2.Investigations are then conducted on the modal density (Section 3.3),the energy flow(Section 3.4),and the forced response of the rod(Section 3.5).In Section 4,the wave transmission and reflection at a planar surface between two waveguides of second gradient material are analyzed for the considered model.Numerical applications of the above are discussed in Section 5 and conclusions are drawn in Section 6.This work not only aims to contribute to the analysis of wave propagation in SSG theory based media,but also aims at providing an original solution to analyze the wave dispersion characteristics of complex media with micro-scale periodicity.

2. Overview of the SSG theory

According to Mindlin’s research,6in SSG theory,the potential energy density u-is assumed to be a function of three tensors ε,η and ξ, in which ε is the classical symmetric strain tensor, η and ξ are respectively the second gradient of the displacement vector and the third gradient of the displacement vector,

3. Analysis of wave dispersion characteristics, energy flow and modal density

3.1. The derivation of governing equation and boundary conditions

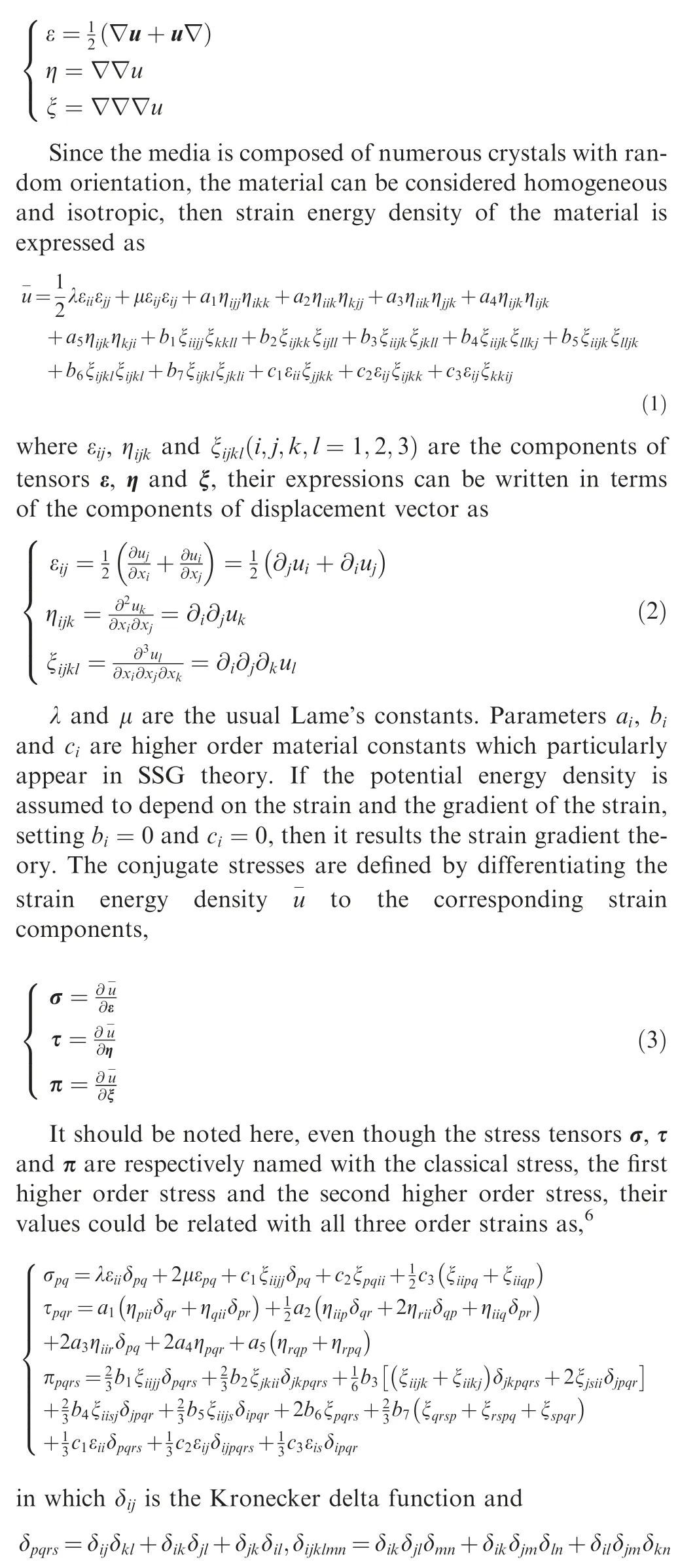
The multi-scale modeling begins with establishing the motion equation of the rod for free vibration. The coordinate system and kinematic parameters of the model are illustrated in Fig. 1. The rod is assumed to be uniform, homogeneous and initially straight along the x-direction with length L.r denotes the radius of the circular cross section, and q（x ，t） denotes the resultant of external forces acting on the rod in x direction as force per unit axial length.

The displacement field in a rod model is written as,

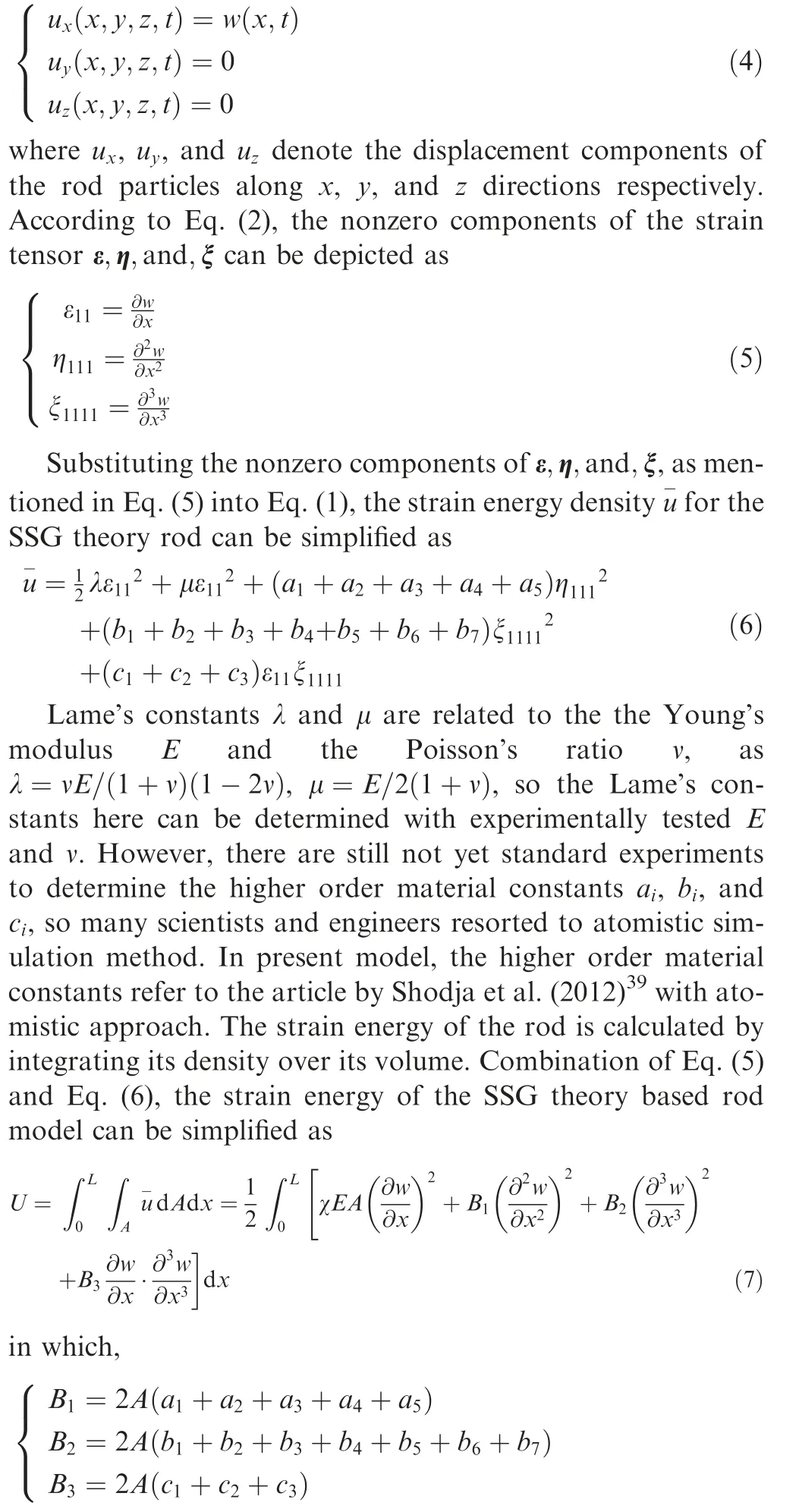
with parameter A as the area of the rod cross section, and χ= （1 -υ）/（ 1 +υ）（1 -2υ）. To avoid the Poisson effect, the results in the present work will be calculated with setting χ=1.

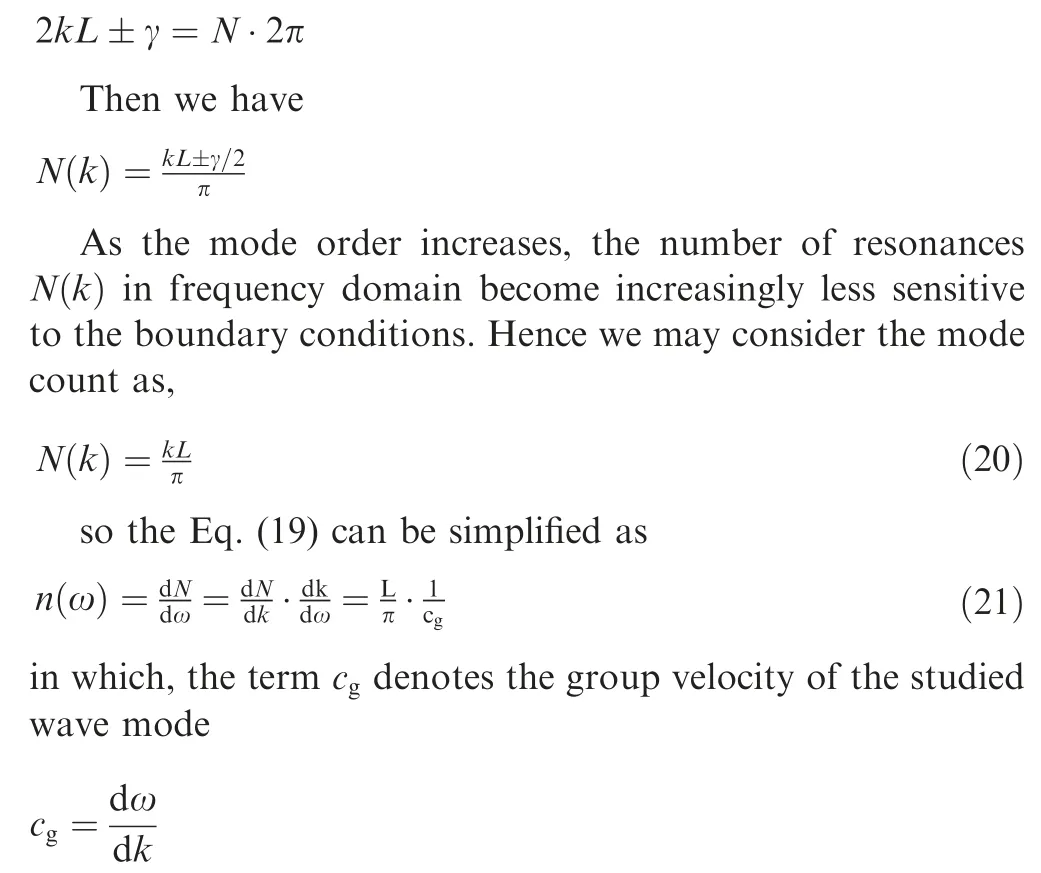
Fig. 1 Coordinate system and kinematic parameters of rod.

the term ωkis defined as

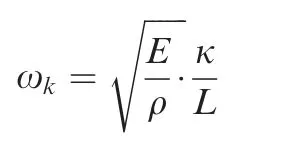
which is the normalization parameter for angular frequency ω.In this case,it is set to be one nature frequency of the structure.κ is a constant determined by the boundary conditions of the model.In the presented model,κ is set to be π.The normalization parameter for time t is expressed as

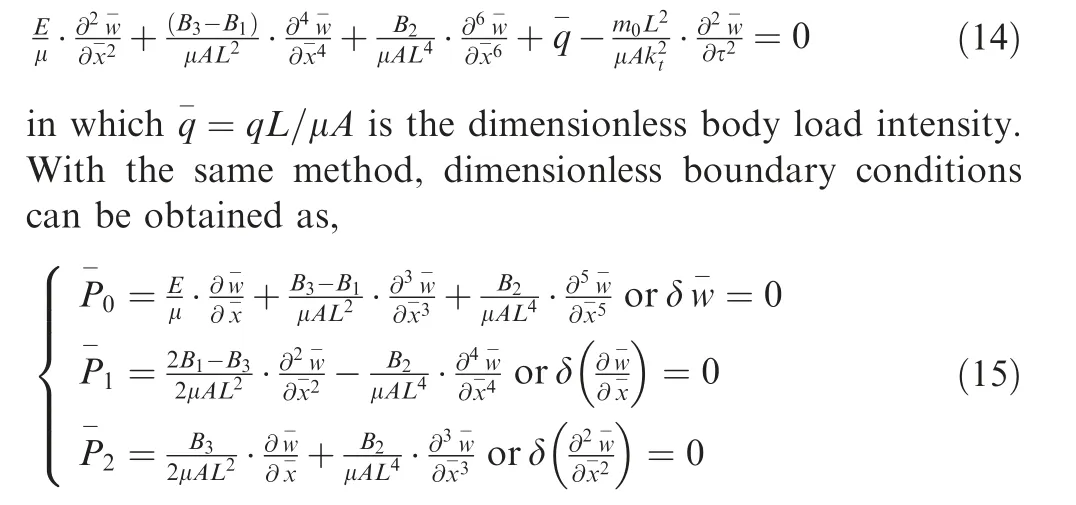
Substituting the newly defined variables into the governing equation and boundary conditions, we can have the normalized model as

3.2. Dispersion relation

For the material with heterogeneity due to micro-structures effects, long range interaction has great influences on the deformation process, especially when the wavelength is of the same order with the heterogeneity,and the classical elasticity theory is clearly not capable of describing these physical properties. In the following section, the dispersion relation of the waves propagating in the rod structure will be investigated based on the proposed model.

Assuming the external loading q-=0, the free wave propagation modes in the proposed enriched model can be achieved by injecting the general exponential form of wave propagation solution:

3.3. Modal density

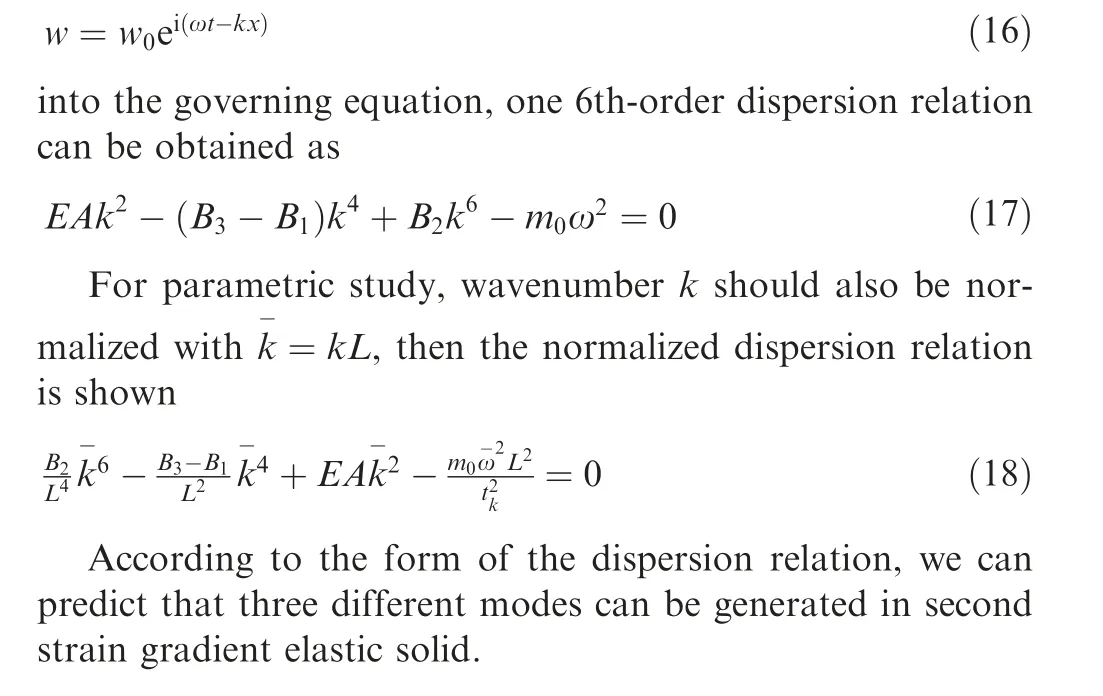
When a structure or a system is subject to excitation of high frequencies, its response may involve a large number of highorder modes. Meanwhile in high frequency range, wavelength tends to be more comparable to the length of inner microstructure,and its influence on wave propagation becomes more pronounced. The modeling of such systems leads the problem of ‘high frequency’ vibration analysis, and Statistical Energy Analysis (SEA) is one of the most common methods for high frequency vibration analysis. In this section, one important parameter in SEA method, namely ‘modal density’, is studied based on the proposed model. Modal density is one statistics based measurement representing the distribution of modal natural frequencies in the frequency domain. Modal density of a structure at frequency ω is denoted as n（ω ）, which means the number of resonant frequencies in a neighborhood Δω local to that frequency. It may also be interpreted as the expected number of natural frequencies per radiant per second as

in which the mode count N represents the number of resonant frequencies below that given frequency ω. The propagating wave at any frequency is characterized by the wavenumber k（=2π/wavelength）, which can be interpreted as the phase difference per unit distance in wave propagation direction.As the wave propagates from the left-end of the rod to the right-hand end,the total phase change is kL.Then wave is reflected by the right-hand end,and back towards the left-hand end.Phase difference will be introduced by the Wave reflection at each end,and that difference varies with different boundary conditions.Assuming γ represent the total phase change introduced by reflecting boundaries,the total phase changes as the wave travels one complete circuit around the structure can be expressed as 2kL±γ. The ‘‘phase-closure principle” states that if the total phase change is an integral number of 2π′s,the condition is satisfied for a natural mode, and the frequency at which it occurs is a natural frequency of the system. This principle can be written as

3.4. Energy flux analysis

In this section, the approach to predict the energy velocity at one point in the SSG theory based rod structure is presented.At one observation point M, the instantaneous kinetic energy density Ek（M，t）, potential energy density Es（M，t） and the instantaneous active energy flow Ep（M，t） based on the classical elasticity theory are defined as42,43

where ρ is the mass density,V is the velocity vector,and σ and ε are the classical stress and strain tensors respectively. As the strain and stress tensor are defined differently in the SSG theory, potential energy density is enriched with the higher order components as6

In the following investigations,the time will be removed by time-averaging. A physical quantity H, which represents here an energy or a power density, can generally be expressed as

where f and g are complex harmonic physical variables which denote stress,strain,or displacement in the formulation.Thus the time averaged of H is given by

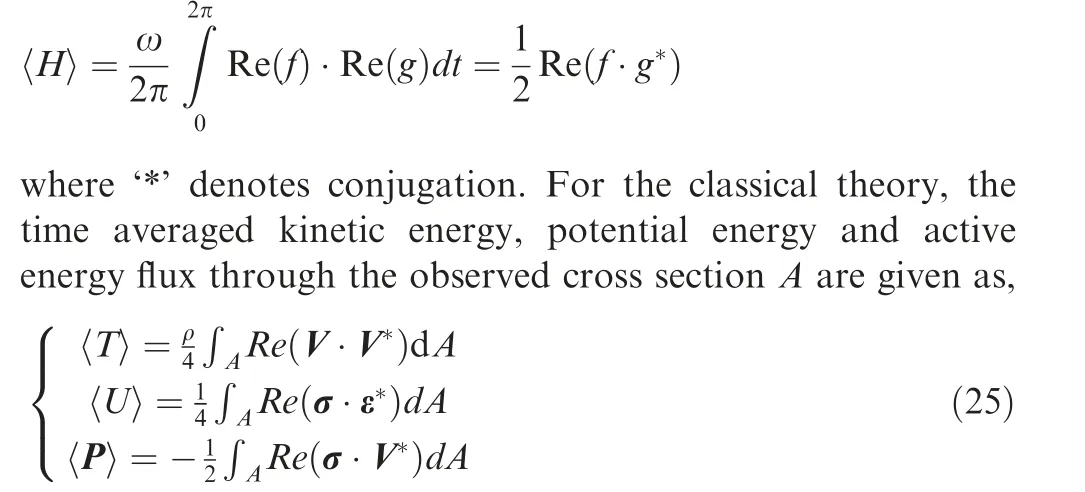
For the SSG theory, the time averaged potential energy of the observed cross section A is given as,

where P0, P1, and P2are the loads dual to the corresponding kinematic parameters, and ‘˙w’ denotes the time derivative of the displacement w.

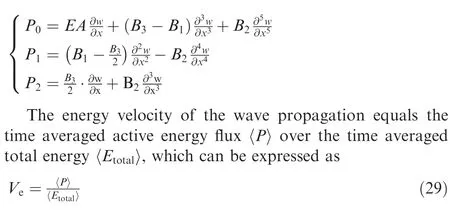
3.5. Frequency response analysis

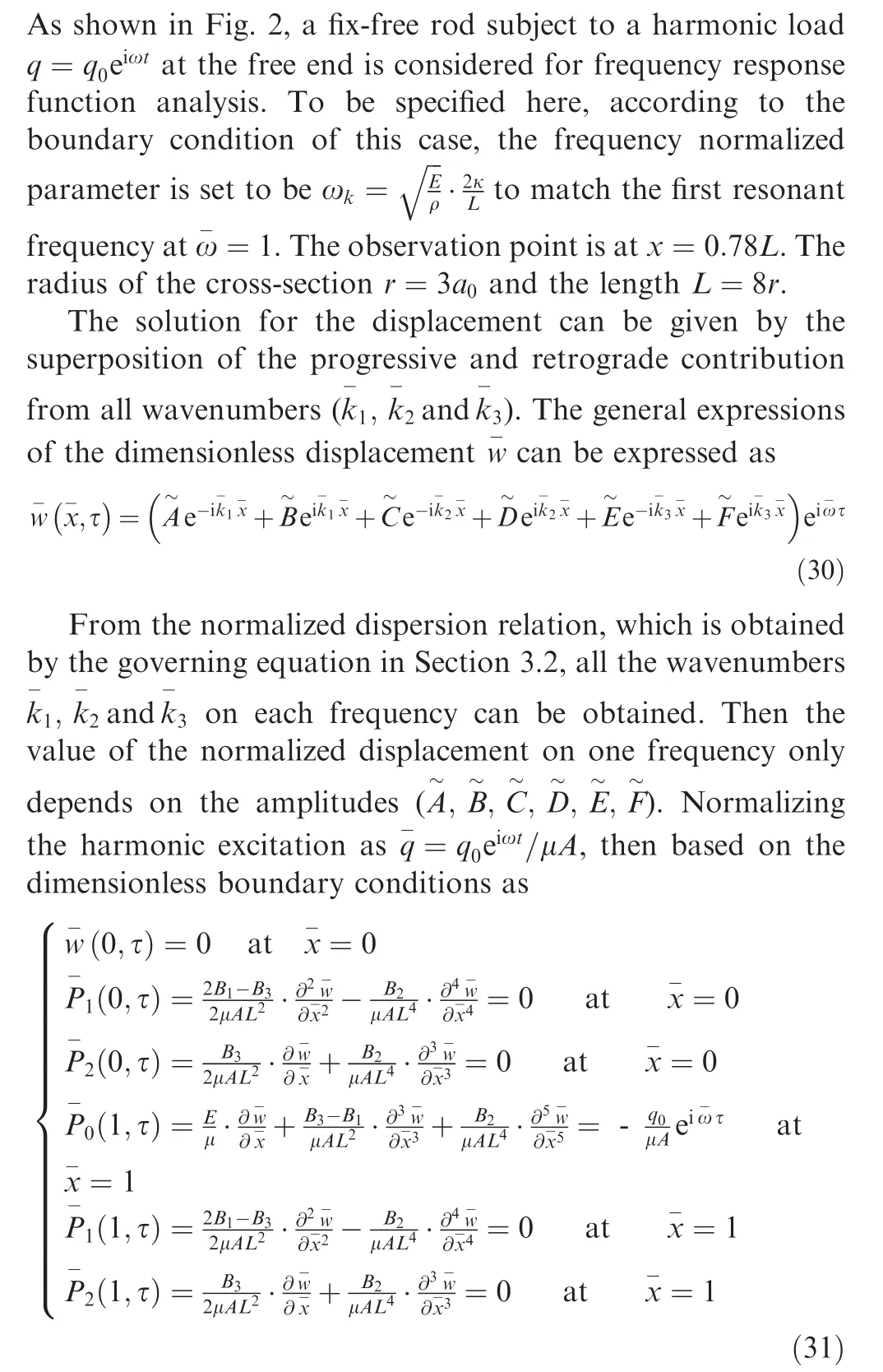
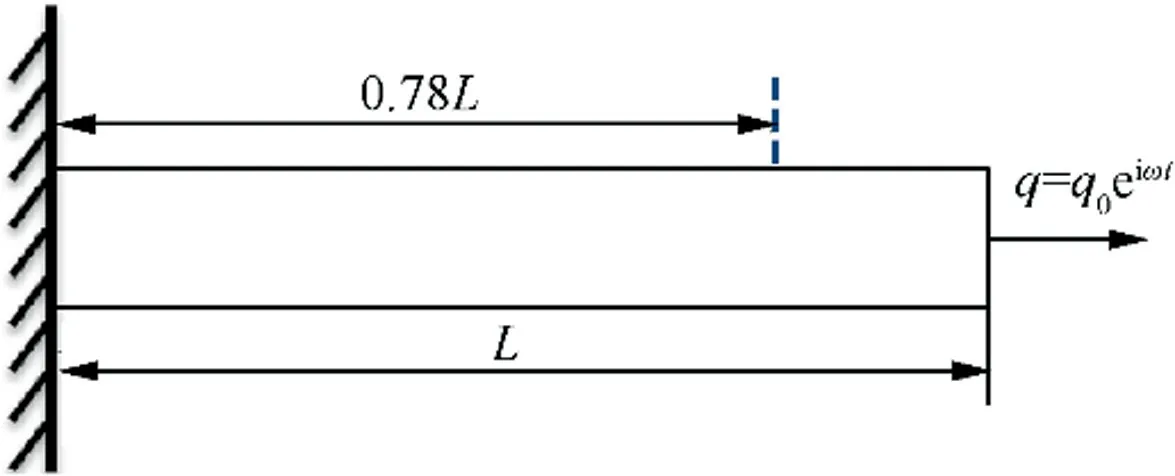
Fig. 2 A fixed-free micro-rod with loading in free end.

4.Wave reflection and the transmission through planar interface based on the enriched model

According to Section 3.2, it can be predicted that the body wave modes are different in second strain gradient continua due to the micro-structure effect, and that will lead to significant differences in elastic transmitted and reflected waves. In this section, the reflection and transmission coefficients on a discontinuous surface as well as the energy transmitted ratio are discussed based on the proposed formulation.Fig.3 shows rod 1 and rod 2, which are connected together with same circular cross section but different materials.

From the dispersion relation in Sections 3.2, we know that 3 modes can be created in second gradient continua.Assuming modes k1，k2，k3can propagate on rod 1 while k4，k5，k6propagate on rod 2, and the incident wave wave k1vertically inject on the discontinuous surface with amplitude A1, then the displacement caused by incident wave U1+can be expressed as

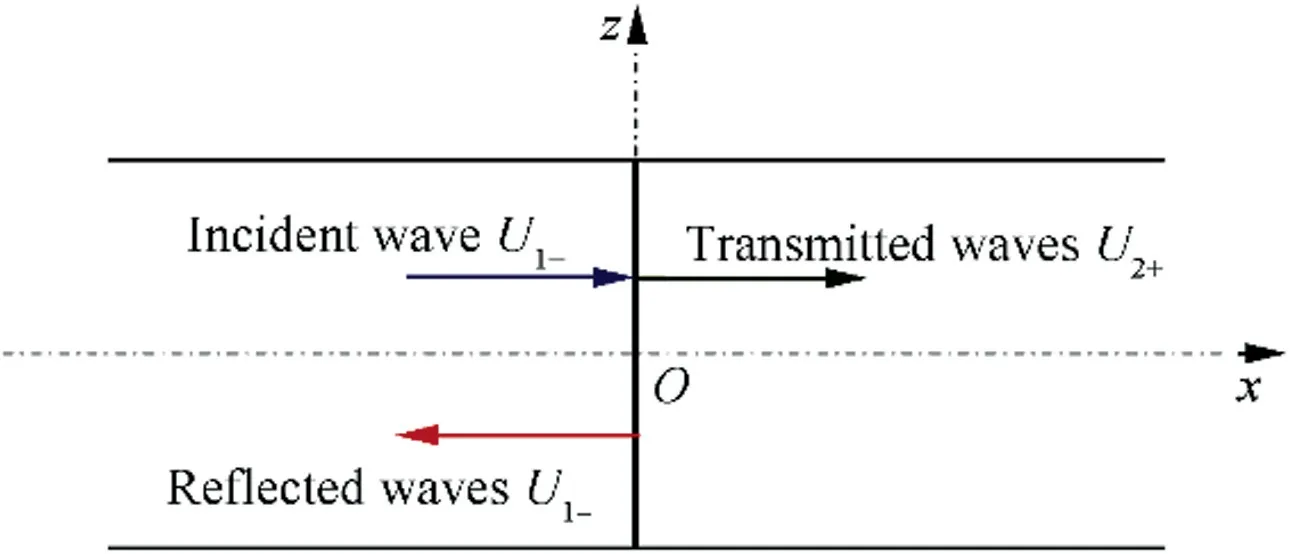
Fig. 3 Reflection and transmission of longitudinal wave.

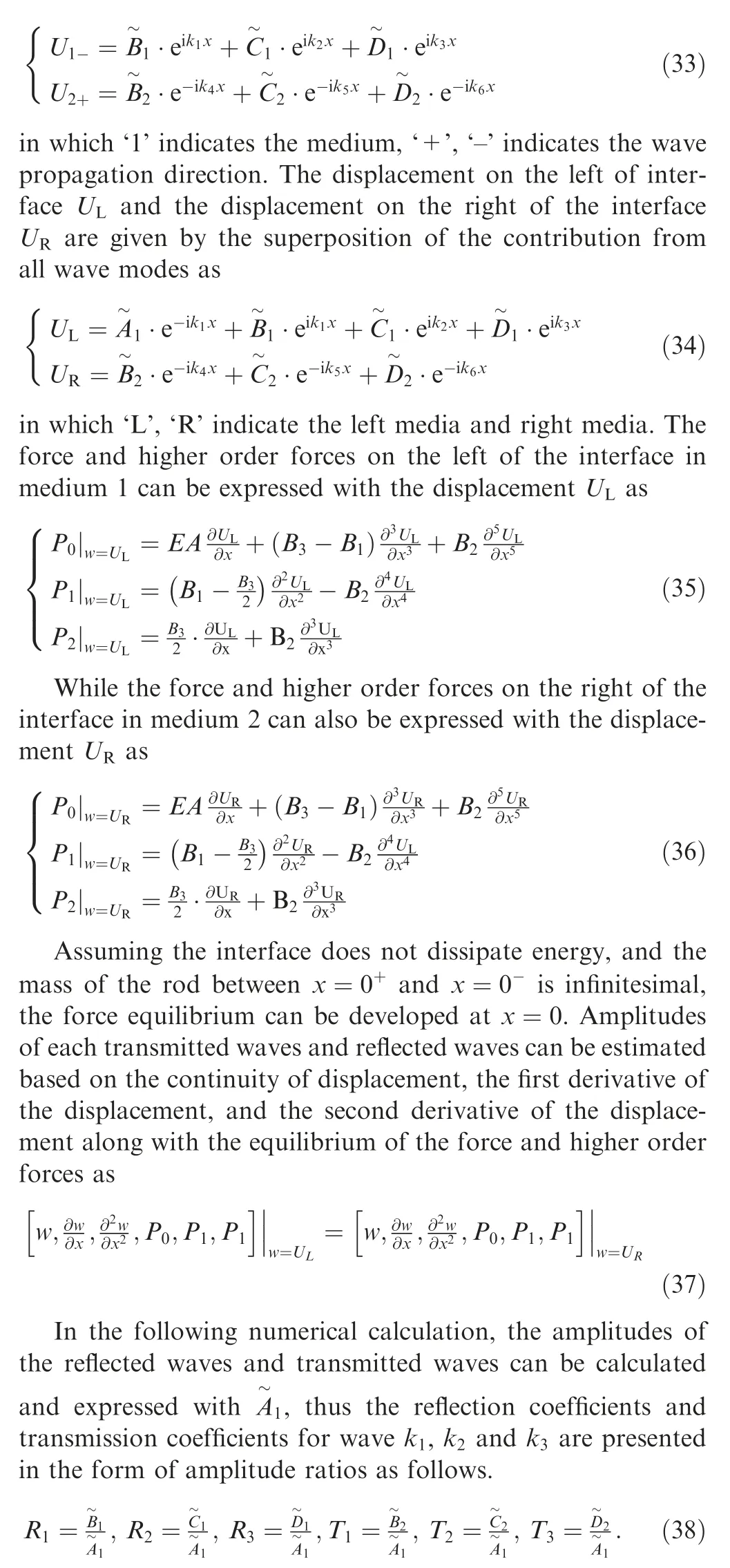
R1,R2and R3are the reflection coefficients for wave k1,k2and k3;T1,T2and T3denote the transmission coefficients for wave k4, k5and k6.

According to Section 3.4, energy flux in second strain gradient continua is coupled with the classical force and the higher order forces, hence the reflected energy flux and transmitted energy flux based on the proposed SSG model can be expressed out as

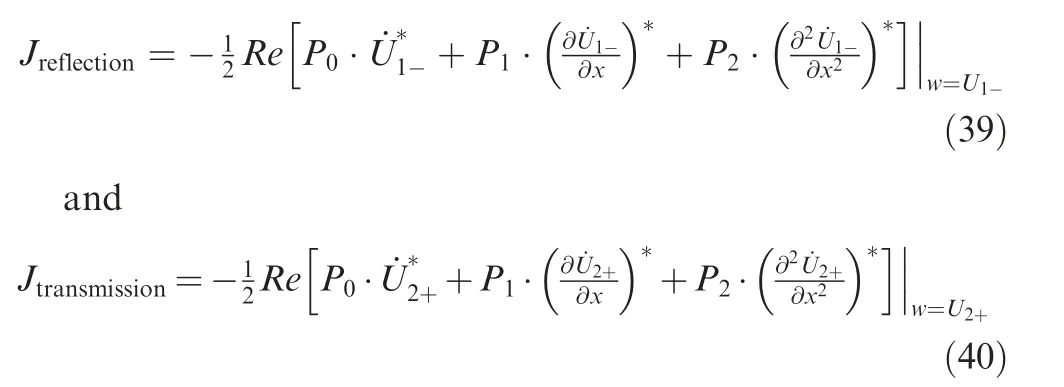
P0, P1and P2in the expressions are in dependence on the displacement in each media. The energy distributed in transmitted and reflected waves can be achieved by the calculating the ratio between the transmitted energy as well as the reflected energy with the incident energy flux.

Until here, the formulation of the governing equation and the associated boundary conditions of the SSG theory based rod model are fully established. The characterization of wave dispersion relation and modal density, the frequency response of bounded SSG theory based rod, the energy flux in second gradient continua and wave reflection and transmission coefficients on the discontinuous surface are all completed.

5. Numerical application and discussions

In this section, cases are studied to illustrate enriched wave properties based on SSG theory model. The structure of the rod is shown as in Fig.140.The material is assumed to be aluminum with μ=26 GPa, and the higher order material constant values are given in Table 1 with lattice parameter a0=4.04A. The rod has a circular cross section with radius r=3a0, and length L=8r.

5.1. Dispersion curves

Employing the parameters above for the dispersion Eq. (18),one arrives six solutions, which indicates 3 positive going modes and 3 negative going modes on each frequency. The results are shown in Fig. 4 and Fig. 5.

Fig. 4. and Fig. 5. illustrate the dispersion relation of positive going waves and negative going waves, respectively. It should be noticed here, the wavenumber k3, with negative imaginary part and negative real part is considered as a positive-going wave, and k6with positive imaginary part and positive real part as a negative-going wave. In a periodic waveguide with unit-cell’s dimension d, k is 2mπ/d -periodic,the positive- or negative-going waves can have arbitraryReal（ k） sign. The direction of propagation is defined by the Imag（k ）, which corresponds to the wave attenuation.

Table 1 High-order material constants for aluminum.40

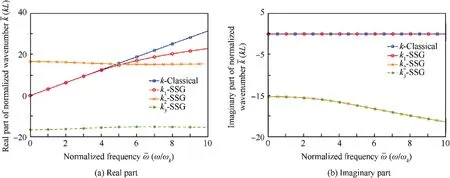
Fig. 4 Dispersion relation of positive-going waves k+（ω）.

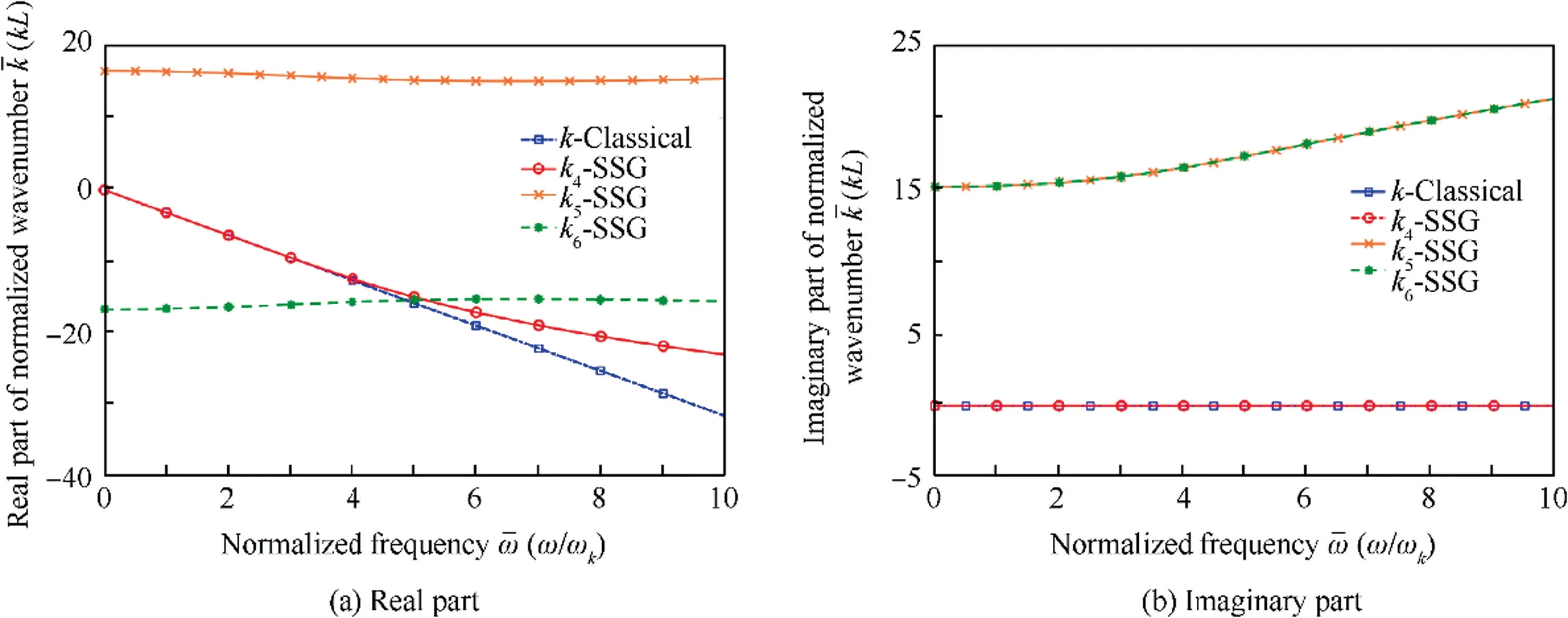
Fig. 5 Dispersion relation of negative-going waves k-（ω）.

Fig. 4 has two subfigures, Fig. 4 (a) and Fig. 4 (b), which display the real part and imaginary part of the dimensionless wavenumbers for the positive-going waves in frequency range［0，10 ωk］. Different from the classical theory results, 3 modes（k1，k2，k3） are predicted by the enriched model, in which the non-classical longitudinal wave k1is propagating in a dispersive manner. Real part of k1is smaller than classical one and the gap become wider with the increasing frequency.The other two wave k2and k3appear exclusively in SSG theory model,and they are both evanescent waves. These results agree with the Refs. 32,33,44,45.

In order to study the scale-effect of the dispersive feature,wavenumbers of mode k1propagating in rod structures of different size are investigated.The results are illustrated in Fig.6.

As dimension goes up, length to radius ratio of the structure is fixed as L=5r, hence the resulting dispersion curve of classical model is linear correlated with angular frequency.Whereas the dispersion curve of SSG theory is observed to keep changing and approaching the classical result as the radius r goes from 3a0to 8a0. When the radius increase, the structural characteristic length grows longer. Therefore, this phenomenon reflects that the influence of micro-structure interaction become weaken as the structural characteristic length goes up.

The above outcomes are all calculated without energy dissipation. However, in practical applications, there are always damping in the system. Energy dissipation can significantly influence wave propagation features. Assuming the damping is viscous,then E=52（1+iζ）GPa can be utilized with ζ indicating the loss factor of the system.To analyze the influence of damping for the proposed enriched model, the dispersion curves with ζ=0.005 is shown in Fig. 7.

In relatively low frequency range［0，2ωk］,the results of SSG model and classical model are similar. In higher frequency range ［2ωk，10ωk］, wave number is evidently influenced for the non-classical wave k1, as the absolute value of imaginary parts decrease and draw near to zero. Energy dissipation through viscous damping for non-classical longitudinal wave k1become negligible compared with the classical model.

5.2. Modal density

Fig.8 depicts modal density of non-classical wave k1compared with classical result, which keeps still in frequency domain.The influence of micro-structure effect is quite obvious as the non-classical result is size and frequency dependent. Modal density of k1decrease significantly compared with classical results. The contrast becomes more serious as the model’s radius decreasing gradually to r=3a0. The present SSG theory model can clearly capture the micro-structure effect. This phenomenon is similar to the observation in the case of wavenumber characterization.

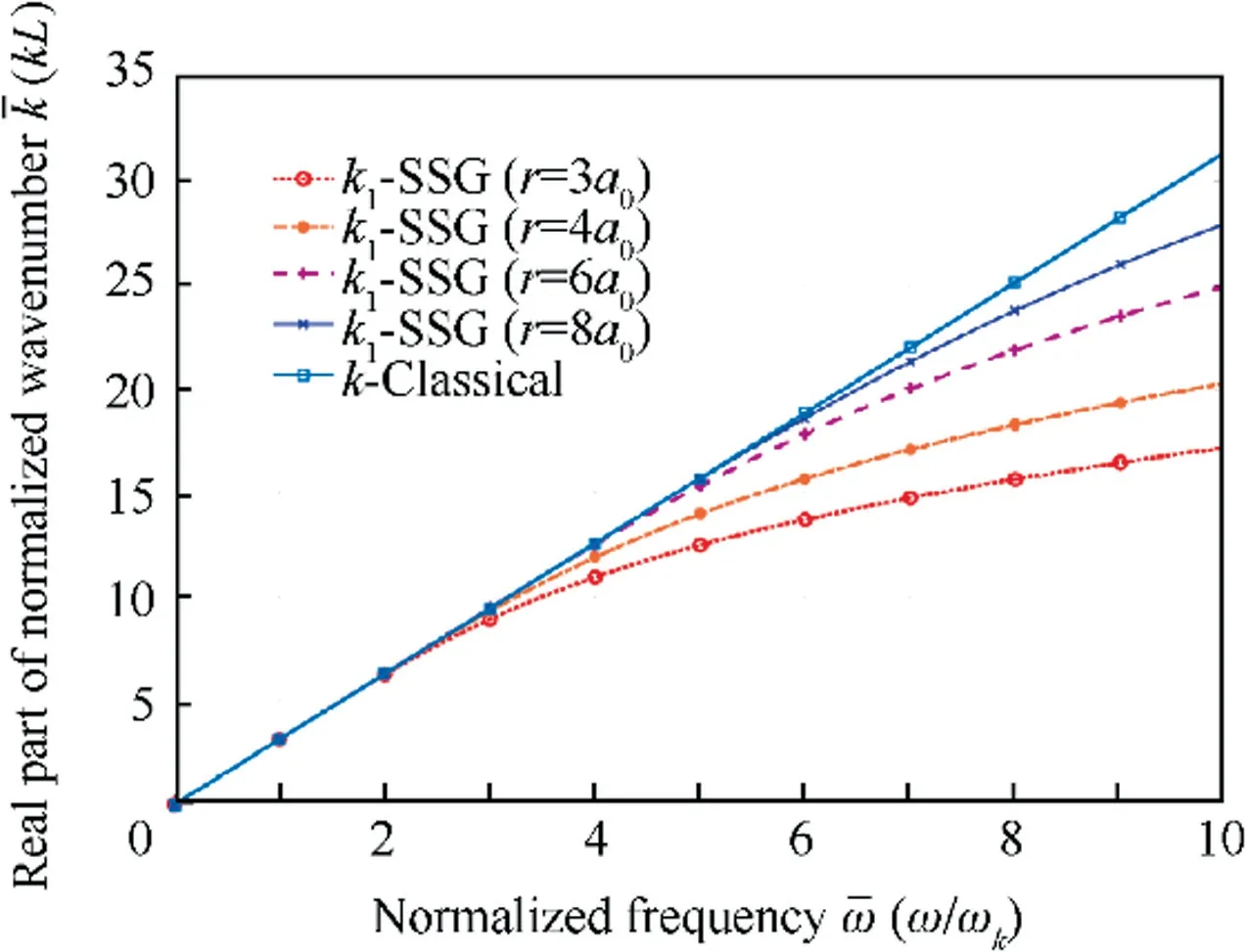
Fig. 6 Wavenumber changing with rod dimension.

5.3. Energy velocity

In Section 3.4, we established the energy flux expression for SSG theory based rod model. The validation of energy flux expression is important for the following researches which are developed from energy equilibrium. In this section, energy velocity Veand group velocity cgare illustrated for nonclassical wave k1. If the formulation Eq. (27) and Eq. (28)are validated, group velocity cgshould be identical to the energy velocity Vewithout damping in the system.

From Fig. 9, as expected, the formulation and for energy flux in SSG theory based rod model are proved to be correct with cg=Vein the whole frequency range. In addition, group velocity cgand phase velocity cphof k1in SSG theory model are clearly different from each other, which confirms the dispersive property of non-classical k1.

5.4. Frequency response function

For frequency response analysis, one harmonic force q=0.05μAeiωtis applied on free end of the structure. Based on the previous formulation, the amplitude of each mode at observation point x-=0.78 are calculated out on each frequency, and the displacement magnitudes are obtained by the superposition of each mode.

Fig. 7 Wavenumber changing with damping.

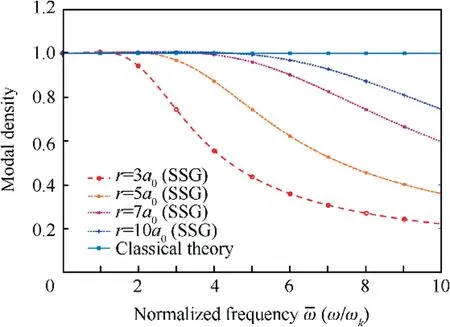
Fig. 8 Modal density.

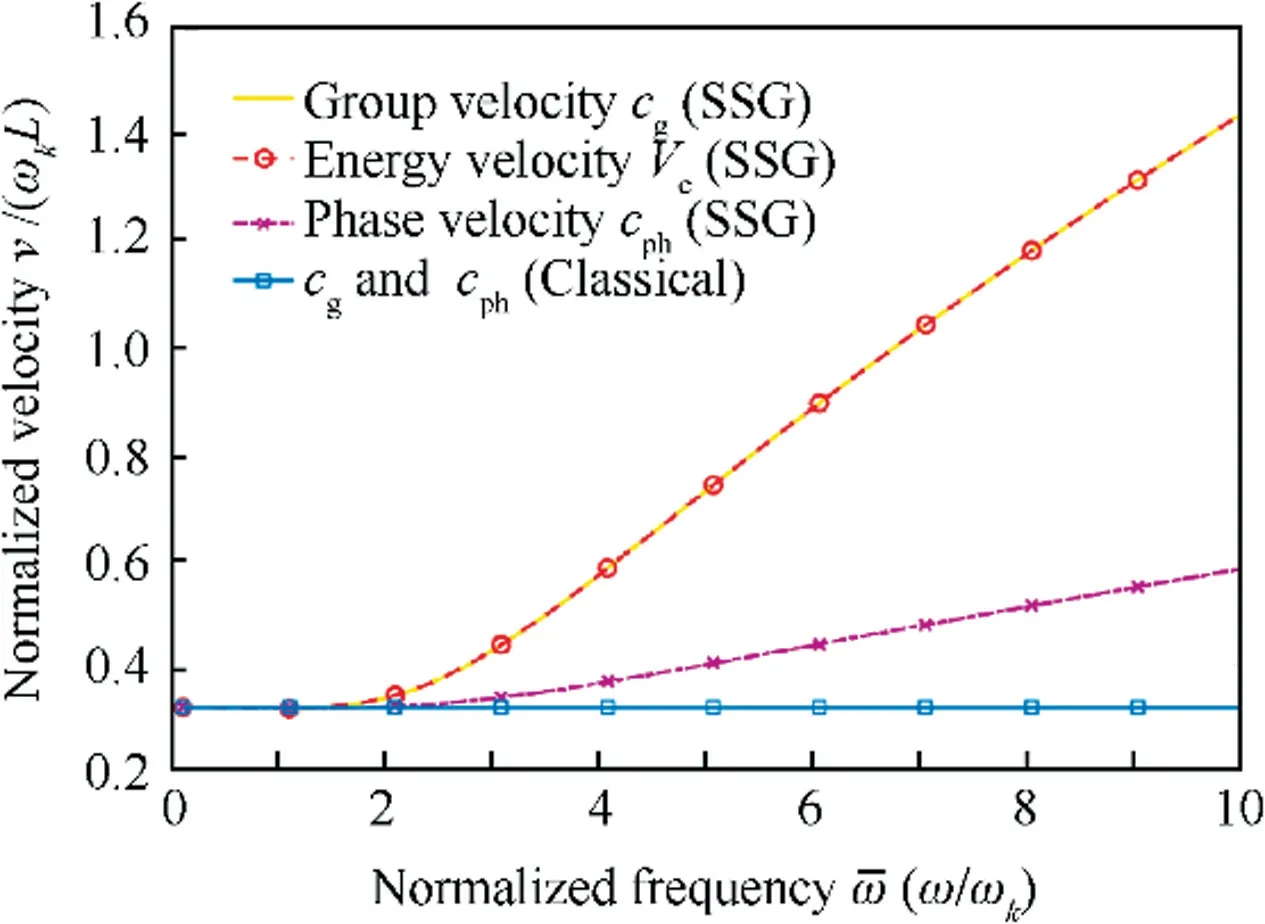
Fig. 9 Energy velocity of longitudinal wave.

Fig.10 displays the normalized displacement magnitudes at observation point based on SSG theory and classical theory.We notice that resonances can be well predicted by both models. In lower frequency range, the results of these two models math well, but in higher frequency, the values of SSG theory shift to higher frequencies compared to classical ones,and less resonance peaks are observed in frequency range ［0.1ωk，10ωk］(7 for SSG theory and 10 for classical theory).Due to the influence of micro-structure interactions, wave propagation is significantly affected, vibration energy is not only conserved in the propagating longitudinal wave, but also converted into the other two evanescent modes. This can be the explanation for the reduction of resonance peaks. In higher frequency,micro-structure effect could cause more energy conversion into the extra evanescent waves.

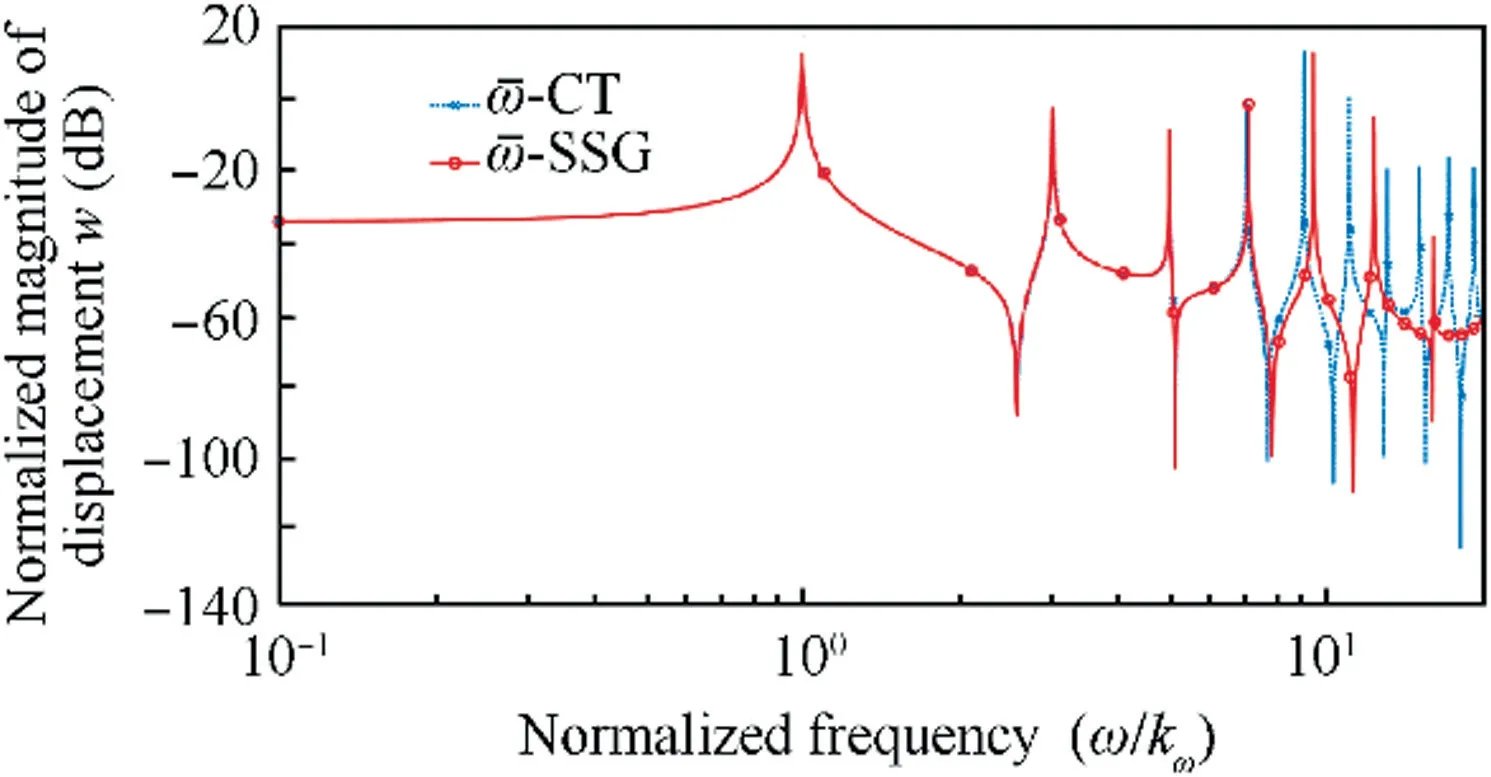
Fig. 10 Frequency response function at x=0.78L.

Table 2 High-order material constants for copper.40

5.5. Reflection and transmission coefficients

Numerical calculation for the reflected and transmitted wave at the discontinuous surface through different SSG theory based structures is conducted in this section. As shown in Fig. 3, rod 1 and rod 2 are connected with same cross section radius r=3a0. Rod 1 is made of aluminum and the material constants have been illustrated in previous section. Rod 2 is copper with μ=40GPa, and the higher order material constants are shown in Table 240.

Substitute these values into the formulations in Section 4,we can easily achieve the reflection and transmission coefficients as well as the energy transmission situation.

Fig. 11 depicts the transmission and reflection coefficients based on SSG theory and classical theory. Compared with the classical transmission coefficient Tcand reflection coefficients Rcwhich stay still in whole frequency range, the SSG theory results are frequency dependent and change significantly especially for R1. In higher frequency, R1decrease dramatically compared with the classical result, while T1changes slightly. Transmission and reflection coefficients T2， T3, R2and R3all grow up as frequency goes up.

In order to verify the size effect for the wave reflection features, reflection coefficients of wave k1propagating in these two SSG theory based rods with increasing dimensions are analyzed and the results are illustrated in Fig. 12. We can see the reflection coefficient of k1increase and approach to the classical result as the cross section radius r increasing from 5a0to 15a0.

Based on the previous formulation, it is admitted that the energy converted to the extra evanescent waves k2and k3leads to great differences in energy transmission. To achieve more information, we intended to calculate the energy conserved in all three modes separately, but it is impossible due to the strong coupling between these three modes refer to energy flux.Therefore,in order to investigate the energy transmission ratio in SSG theory model, Fig. 13 is plotted.

Fig. 11 Transmission and reflection coefficients.

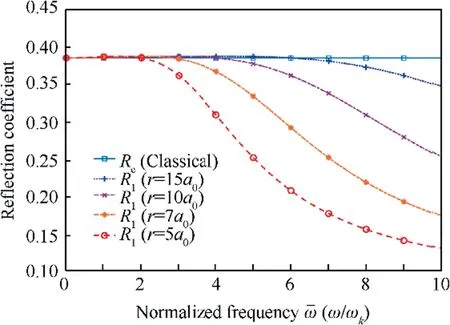
Fig. 12 Size effect of reflection coefficients.

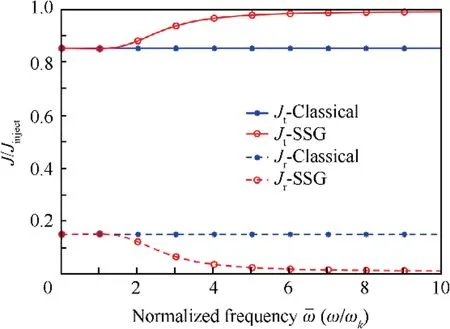
Fig. 13 Energy transmission and reflection.

Fig. 13 shows the distribution of transmitted energy and reflected energy conserved in all 3 modes. We notice that even though the energy transmitted ratio with SSG theory formulation is increasing and reflected ratio is decreasing, the summation of them is still ‘1’, which indicates the previous formulations are valid.With the enriched model,energy is predicted to transmit more and reflect less.Combined with Fig.11(a), we noted that that the transmitted energy diffuse quite large percentage to wave modes k2and k3,so T1,the transmission coefficient of wave k1is actually decreasing. Conversely,the total reflected energy decreases slightly, but with considering the energy diffusion into k2and k3,the reflection coefficient for wave k1decreases dramatically.

6. Conclusions

Wave propagation in SSG theory based media with consideration of the heterogeneity caused by micro-structure effect is different from the conventional one. In order to analyze this special behavior, we established an enriched model based on Mindlin’s Second Strain Gradient(SSG)theory.This formulation allows the micro-structure’s effect to be captured by considering the higher order strains and the heterogeneity to be described in the frame of continuum mechanics. From the numerical calculations,one can see that the proposed SSG theory based rod model is effective in predicting the non-classical dispersive behaviors in complex media, and that results are in good agreement with some literature.32,33,44,45Apart from the dispersion characteristics,this research also highlighted a number of interesting features of the modal density,the energy flux and the resulting forced response of the rod. Wave transmission and reflection between two different waveguides in second gradient material was also investigated. Some conclusions can be drawn from the numerical calculation and discussions as follows:

(1) There are three waves in second gradient rod,one is the propagating non-classical longitudinal wave k1which exhibits dispersive feature, and the dispersive behavior become more prominent in higher frequency. The other two waves k2and k3are both evanescent. In higher frequency,energy dissipation through viscous damping for wave k1become negligible compared with classical result. As dimension goes up, micro-structural effect is weaken for the reason that the length of inner heterogeneity is less comparable to the increasing structural characteristic length, and wave k1become less dispersive.

(2) The proposed formulation of the energy flux is validated, as the energy Veand group cgvelocities of wave k1are proved to be identical in all the frequency range.Energy flux is coupled with one classical force and two higher order forces,which are respectively the resultants of classical and higher order stresses through the surface of the section.

(3) The statistical value of the modal density for the nonclassical longitudinal wave k1decreases drastically compared with classical results. This is due to the influence of micro-structure. This phenomenon becomes more pronounced as the model characteristic size decreases.From the FRF analysis,the resonant frequencies are different,the values of SSG theory shift to higher frequencies compared to classical ones.These unusual behaviors can be explained with different energy transfer through the extra evanescent waves k2and k3especially in higher frequency range when the wavelength and the structural characteristic length are in the same order.

(4) Wave reflection and transmission on a discontinuous surface based on the enriched model are highly different from the classical theory ones. Vibration energy of the incident wave is predicted to transmit more and reflect less, while the transmitted and reflected energy is conserved in all three modes.Due to the influence of modes k2and k3, energy attenuation increases to some extent.Therefore, the transmission coefficient of the propagating wave k1decrease slightly and the reflection coefficient decreases drastically. The higher the frequency becomes, the greater the impact of micro-structure.

Acknowledgements

This work was supported by the LabEx CeLyA(Centre Lyonnais d’Acoustique, ANR-10-LABX-0060) of Universite´ de Lyon.