双参数C-半群

黄翠,王彩侠,张明翠,王淑莉

(中国矿业大学理学院,江苏徐州 221008)

双参数C-半群

黄翠,王彩侠,张明翠,王淑莉

(中国矿业大学理学院,江苏徐州 221008)

引入单参数C-半群和双参数C0-半群,给出了更一般的双参数C-半群和无穷小生成元的定义,应用双参数半群与单参数半群的关系和双参数半群的性质,给出了双参数C-半群的阶全微分,偏微分,指数有界性,和双参数C-半群是由C、A1和A2唯一确定的闭稠定线性算子的一个充分条件.

双参数C-半群;全微分;偏微分;指数有界;闭稠定线性算子

1 引言

满足某些条件的算子半群是对应抽象柯西问题(ACP)的解.目前算子半群已经被应用生物、物理、计算机、交通等领域.因此,研究一些算子半群的性质是非常必要的.前人对C0-半群的性质,扰动和逼近,稳定性已经做了很深入的研究,得到了很多有用的结果.对于更一般C-半群和双参数C0-半群也有一些研究,但是没有像C0-半群理论那么深入,尤其是扰动和逼近、稳定性.本文根据C-半群和双参数C0-半群,定义更一般的双参数C-半群,并对它的全微分和偏微分,指数有界性和闭稠定性做了详细的研究.

2 定义和引理

定义2.5[3]设S是R中的点集,如果点集S在每个非空的开集中都不稠密,就称S是疏朗集或称无处稠密集.

引理2.1[3]点集S是疏朗集的充要条件是在任何开区间(α,β)中存在开区间(α′,β′)⊂(α,β)在开区间(α′,β′)中没有S中的点.

引理2.2[3]疏朗集的余集一定是稠密集.

3 主要结论

证明设映射L:R2→L(x),如果存在某个线性变换L使得(s,t)∈U(0,0)点(0,0)的某个领域时有T(s,t)-T(0,0)=L(s,t)+R(s,t),其中

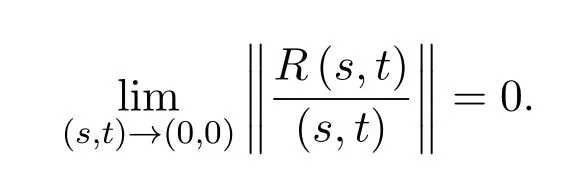
则T(s,t)作为二元函数在(0,0)处的微分dT(s,t)|(0,0)存在.

设A1、A2分别是C-半群{T(s,0)}s≥0和{T(0,t)}t≥0的无穷小生成元,令

[1]陈文忠.C-无穷小生成元的表示式[J].厦门大学学报:自然科学版,1993,32(2):135-140.

[2]Al-Sharif Sh,Khalil R.On the generator of two parameter semigroups[J].Applied Mathematics and Computation,2004,156:403-414.

[3]张鸣歧.应用泛函分析引论[M].北京:北京理工大学出版社,1989.

[4]黄永忠.算子半群与应用[M].武汉:华中科技大学出版社,2011.

[5]Li Y C,Shaw S Y.On characterization and perturbation of local C-semigroups[J].Proceedings of the American Mathematical Society,2007,1(1):1097-1106.

[6]Shaw S Y,Kuo C C.Generation of local C-semigroups and solvability of the abstract Cauchy problems[J]. Taiwanese Journal of Mathematicas,2005,9(2):291-311.

[7]陈闯,宋晓秋,李晓敏.C-半群Lyapunov方程的自伴解与稳定性[J].南京大学学报:数学半年刊,2008,25(1): 86-93.

[8]宋晓秋.应用泛函分析[M].徐州:中国矿业大学出版社,2013.

Two parameter C-semigroups

Huang Cui,Wang Caixia,Zhang Mingcui,Wang Shuli

(College of Science,China University of Mining and Technology,Xuzhou221008,China)

According to the C-semigroup and two parameter C0-semigroups,this paper gives the definition of a more general two-parameter C-semigroup,infinitesimal generator,two parameter C-semigroup′s total differential and partial differential.It is exponentially bounded.With the complement of C-semigroup′s domains lichtung setes,proof the two parameter C-semigroups is uniquely determined by the C,A1and A2,whose infinitesimal generator is densely defined and closed linear operator.

two parameter C-semigroups,total differential,partial differential,exponentially bounded, densely defined and closed linear operator

O177.2

A

1008-5513(2013)03-0299-07

10.3969/j.issn.1008-5513.2013.03.012

2012-11-06.

高水平论文专项基金(2012LWB53).

黄翠(1986-),硕士生,研究方向：泛函分析与应用泛函分析.

2010 MSC:47A62