带Brown运动的随机奇异积分的存在性
殷承元
(上海财经大学应用数学系,上海 200433)
带Brown运动的随机奇异积分的存在性
殷承元
(上海财经大学应用数学系,上海 200433)
在轨迹二阶导数具有H¨older连续的条件下,利用高阶奇异积分思想和概率极限的理论,研究了在Brown运动下的随机奇异积分.得到了以Brown运动为积分元的随机奇异积分是存在性定理.
随机奇异积分;Brown运动;随机基
1 引言
奇异积分理论和应用非常重要,文献[1-2]做过了大量开创性工作.随机积分也日益发展[3].文献[4]讨论了简单的随机奇异积分.本文在文献[5-6]基础上,探讨在Brown运动下的一类奇异积分.
设Ω是一个完备的概率空间,其中A是Ω上的一个σ-代数,P是关于这个σ-代数的概率测度.记F是满足通常条件的过滤,(ΩA,P,F)是随机基,Wt=W(t,ω),t∈R+是Brown运动.
设过滤F在时刻t的σ-代数为Ft,f(t,τ,ω)是R+×R×Ω,上的函数,对每个t≥0, f(t,τ,ω)关于σ(B[0,t]×B(R)×Ft)可测.本文将讨论下列的奇异积分:
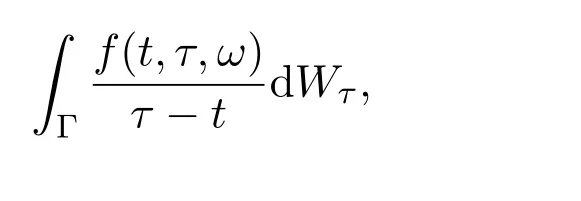
这里Γ是R+中的区间.通常情况下,

是发散.本文将利用高阶奇异积分的思想引入这个带Brown运动的奇异积分.
2 结果与证明

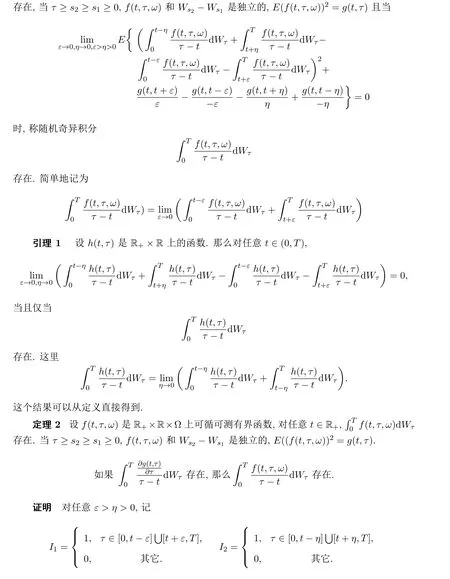

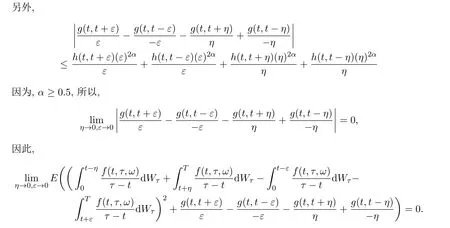
[1]路见可.解析函数边界问题[M].武汉:武汉大学出版社,2009.
[2]Du Jinyuan.Singular integral operators and singular quadrature operators associated with singular integral equations[J].Acta.Math.Sci.,1998,18(2):227-240.
[3]P´eter Medvegyev.Stochastic Integration Theory[M].Oxford:Oxford University Press,2007.
[4]王传荣.随机奇异积分的存在定理[J].福州大学学报:自然科学版,2004,32(4):393-396.
[5]殷承元.闭复超球上的普里瓦洛夫定理[J].安徽大学学报:自然科学版,1990,14(3):6-11.
[6]殷承元.第三类典型域上的Cauchy型积分[J].纯粹数学与应用数学,2002,18(1):6-20.
Existence of singular integral with Brown motion
Yin Chengyuan
(Applied Mathematics Department,Shanghai University of Finance&Economics,Shanghai200433,China)
In the note,random singular integral with Brown motion is investigated.If the second derivative of the integrand is H¨older continuous,by the theories of higher singular integral and limit with respect to probability,the random singular integral with integrator of Brown motion exists in probability limit.
random singular integral,Brown motion,stochastic basis
O211
A
1008-5513(2013)03-0221-05
10.3969/j.issn.1008-5513.2013.03.001
2013-03-15.
国家自然科学基金(11271240);上海财经大学211工程项目.
殷承元(1958-),博士,教授.研究方向:基础数学与概率.
2010 MSC:47B06,47B38

