Principles and methods for determining calendar life and corrosion tolerance of mechanical parts

Fuze ZHANG

Beijing Aeronautical Technology Research Center, Beijing 100076, China

KEYWORDS Coating calendar life;Corrosion damage tolerance;Equal-damage temperature and humidity spectrum;Metal calendar life;Total calendar life

Abstract In order to facilitate the determination of the calendar life of mechanical parts, the author summarized his relevant research papers that had studied for many years as eight distinct topics: (A). The principle and method for compiling the equal-damage temperature and humidity spectrum; (B). The principle for preparing the high concentration medium solution; (C). The principle and method for determining the metal calendar life; (D).The principle and method for determining the protective coating calendar life;(E).The principle and method for determining the total calendar life; (F). The principle of the reliability processing; (G). The principle and method for determining the corrosion damage tolerance; (H). The principle and matching design method to ensure that the total fatigue life and the total calendar life of the mechanical part were safe simultaneously. The above contents established a complete set of theoretical systems and methods for determining the calendar life.

1. Introduction

The studying of the calendar life of mechanical parts (including aircraft structures) belongs to the corrosion discipline that has been conducted for more than 500 years.However,due to too many and complicated factors affecting corrosion,it is still not possible to determine the calendar life and corrosion damage tolerance of metal parts in the service environment internationally. Long-term use of mechanical parts without calendar life control makes the corrosion damage value of the metal structure greater than the corrosion tolerance value, which has leaded to the occurrence of major accidents.For example,in 2002 and 2007,two F-15 aircrafts in the United States broke up in the air because of tail corrosion and no calendar life control. In 1981, a Boeing 747 passenger plane in Taiwan, China,broke up in the air due to corrosion of the fuselage and no calendar life control, killing 150 people. In 1980, a Chinese helicopter disintegrated in the air causing casualties owing to blade girders corroded and no calendar life control. In 1984,a chemical pipeline in India was cracked as a consequence of corrosion, killing 3000 people and seriously injured 180000 people. In 1992, a gasoline pipeline in a Mexican factory leaked for corrosion reason, killing 300 people. There are numerous similar incidents all over the world. According to international statistics, the economic loss caused by corrosion accounts for about 3% of GDP in every country in the world every year. What a huge economic loss!

The author has always been engaged in the study of aircraft fatigue life, but in order to solve the disasters and economic losses caused by corrosion,he has studied the corrosion calendar life in his spare time.After more than 20 years of research,it is now possible to use real corrosion parameters to determine the calendar life of parts in the real environment through theoretical calculations and experiments. The set of theories and methods for determining the calendar life has been validated by comparing the calculation result of a type of aircraft with the real calendar life.Because as the papers about this research have been published over a long span of time, and are scattered, it is not convenient for readers to consult. To this end,the author rearranged the published papers on calendar life according to different majors. Each paper focused on its composition principles and application methods. The formula derivation and experimental verification were not repeated in this paper.If details were needed,please refer to the references.The specific contents of the summary were as following.

2. Compiling the equal-damage temperature and humidity spectrum and preparing the high concentration medium solution

The temperature and humidity spectrum and corrosive media were the basic parameters for determining the calendar life of the parts. Without an accurate temperature and humidity spectrum and the real use media, an accurate calendar life of the mechanical part could not be ascertained.

2.1. Compiling the equal-damage temperature and humidity spectrum

2.1.1. Conversion formula

In the world, it was always impossible to equal-damage convert the original use temperature and humidity spectrum into the corrosion test temperature and humidity spectrum, which made the corrosion test results lose their authenticity. The author proposed the Eq. (1) in which the original temperature and humidity spectrum of various temperatures and humidity could be equal-damage converted into the test temperature and humidity spectrum1. Which was derived from the T-H curve and RH-H curve developed by the author in the Ref.2(T,RH and H are corrosion temperature, humidity, and time respectively). For details, please refer to the Ref.1.

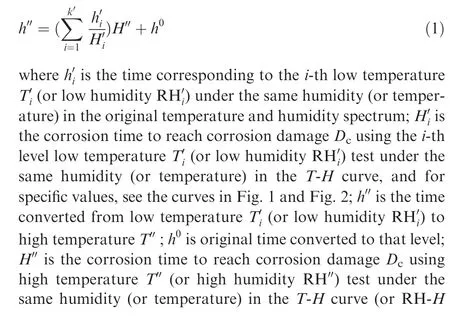
Fig. 1 T-H curve.

Fig. 2 RH-H curve.

Table 1 Original average temperature and humidity spectrum of Hainan province in one year.

Table 2 H value of T-H curve and RH-H curve of LY12CZ aluminum alloy in test (Dc = 0.1 mm).

(4) Conversion result: Through the equal-damage conversion of Eq.(1),the 7th-level humidity RH in Table 1 was converted into the 3rd-level humidity RH in Table 3.

It could be seen that the test time reduced from 10.7 months to 7.3 months.In the same way,the 6th-level temperature T in Table 1 could be converted into 1st-3rd level temperature T,which reduced the test time even more.This kind of high temperature and humidity spectrum is equivalent to the original temperature and humidity spectrum, and there is no need for equivalent conversion during application. In this way, the equivalent conversion problem that has been unable to be solved in the international corrosion field has been solved.

2.2.Preparing the high concentration corrosive medium solution

To determine the calendar life of parts,it is not only necessary to measure and count the real corrosive medium components and contents in the service environment of parts, but also to prepare the high concentration corrosive medium solution(hereinafter referred to as the high concentration medium solution)with these media and contents for the calendar life corrosion test of parts. At present, countries in the world do not have a unified principle and method of corrosive medium solution preparation,and this article gives the following principles and methods6,7.

Table 3 Equal-damage temperature and humidity spectrum converted from Table 1.

2.2.1. Basis curve

The relationship curve between the metal corrosion damage D and the corrosion solution concentration d proposed in the Ref.7, i.e., the corrosion ‘‘3 equal-line”, is shown in Fig. 3.

It can be seen from Fig. 3 that the corrosion damage value D had a linear relationship with the corrosion solution concentration d when the test temperature, humidity, and time remained unchanged. This linear relationship was the basis for preparing high concentration medium solutions.

2.2.2. Method

(1)Measure and count the components and contents of real corrosive media in the service environment of the part,and let the concentration of the corrosive media solution in this case d = 1. The contents of three media with d = 1 are shown in Table 4.

(2)In order to accelerate the corrosion test,it was necessary to increase the multiples of various media content,i.e.,increase the multiples of d.According to the D-d curve in Fig.3,D had a linear relationship with d.Therefore,on the basis of d=1,d could be increased by a factor of 10, 20 and 30 as shown in Table 4.

2.2.3. Conversion of the calendar life tested with high concentration medium solution

How to convert the metal calendar life which was calculated by using the corrosion damage value tested with high concentration medium solution back to the calendar life of the service environment (d = 1)? This was the equivalent conversion problem in the international corrosion field, and it also led to the problem of large errors in the calendar life.So far,international researchers are still looking for a solution to this problem.In the Ref.7,the author solved this problem by using the metal calendar life formula deduced by the corrosion ‘‘3 equal-line”. The calendar life calculated by this formula was the calendar life in the service environment and required no need for equivalent conversion.

Fig. 3 Metal corrosion D-d curve.

Table 4 Preparation of three kinds of high concentration solution in Hainan province.

3. Determining the metal calendar life

The metal calendar life is the main body of the total calendar life of parts. If the main body calendar life is not accurately determined, there is no accurate total calendar life of parts.But so far,in the world,no discipline can use the real corrosion parameters to give the metal calendar life through theoretical calculations and experiments. After more than 20 years of research,the author has given the following three kinds of theoretical formulas and methods for determining the metal calendar life. From the application needs, a brief description is as follows.

3.1. Determining the metal calendar life by corrosion damage

3.1.1. Calculation formula

In the study of Ref.7, the author found that the metal corrosion damage D was linearly related to the corrosion solution concentration d, as shown in Fig. 3. According to this linear line,the formula for calculating the metal calendar life by corrosion damage was given after derivation, as following.

Where: λ is the metal calendar life (year); λtis the cycle number of the temperature and humidity spectrum in the test,i.e.,the corrosion time; Dcis the known metal corrosion damage value; Dtis the corrosion damage value obtained through test (or use); dtis the solution concentration value for corrosion test.

3.1.2. Solution method

(1) Compile the equal-damage temperature and humidity spectrum.

According to the conversion principle and method given in Ref.5, compile the equal-damage temperature and humidity spectrum.

(2) Prepare high concentration medium solution (hereinafter referred to as the high concentration dt).

(3) If the part is under load, the load spectrum should be compiled for the corrosion test.

(4) According to corrosion and different stress conditions,make specimens. Three kinds of test concentrations should be considered, each of which uses 3-5 specimens.

(5) Obtain Dtand λtthrough experiments

Divide the specimens into 3 groups. Each group of specimens is subjected to corrosion tests with the 3 kinds of high solution concentrations and the compiled temperature and humidity spectrum. If the part is also under load, the load spectrum should be added. Regarding the termination time of the test, since the conclusion was reached in the Ref.8that the length of λtdid not affect the calendar life calculation,the λtcan be customized in the test.However,in order to facilitate the detection of the corrosion damage value,the stopping time λtshould be maintained until the specimen has obvious corrosion damage. After the tests are terminated, the average corrosion damage value Dtof the 3 groups of specimens under 3 kinds of concentrations is obtained by measuring the corrosion damage of each specimen. From the termination time of the tests,the cycle number of the test temperature and humidity spectrum corresponding to Dt, i.e., λt(year), can also be obtained. And from the tested solution concentration, the dtvalue can be obtained too.

(6) Obtain the metal calendar life λ

The metal calendar life can be obtained using Eq.(2)by the experiment value dt, Dt, λtand known Dc.

3.1.3. Application example

(1) Question: What was the calendar life λ of 30CrMnSiA alloy steel used in Hainan Island when the corrosion damage Dcreached 200 g/m2?

(2) Solution method and steps

①Prepared the high concentration dt,as shown in Table 4.In theory,one kind of high concentration dttest was sufficient,but in order to reduce the test error, three kinds of high concentration dttests should be used to calculate λ by taking the average value of Dt.

②Compiled the equal-damage temperature and humidity spectrum, as shown in Table 5.

③Calculated Dt, λt, dt:

Used the high concentration d in Table 4 and the temperature and humidity spectrum in Table 5 to perform a 92-days corrosion test. The test data is shown in Table 6.

From the test results in Table 6,the average corrosion damage value of the three kinds of concentrations could be calculated, Dt=(306 + 336 + 354)/3 = 332 g/m2. Since this test was conducted only for one cycle (92 days) according to the temperature and humidity spectrum in Table 5,λt=1(1 year).Also because this test was carried out with three kinds of concentrations, the average dt=(10 + 20 + 30)/3 = 20.

④Calculated the calendar life λ of 30CrMnSiA alloy steel in Hainan Island when Dc= 200 g/m2.

Substituting the obtained Dt= 332 g/m2, λt= 1, dt= 20 and known Dc= 200 g/m2into Eq.(2) to obtain λ .

3.1.4. Application notes

(1) For any metal with known corrosion damage Dc, the calendar life λ can be obtained by Eq.(2).

(2)The height of dtand length of λtin Eq.(2)have no effect on λ . Therefore, dtand λtin the test can be customized, see Ref.8for details.

(3) Because λ calculated by Eq. (2) is already for the environment of metal part (d = 1), there is no need for the international traditional ‘‘equivalent conversion”.

3.2. Determining the metal calendar life by the ‘‘corrosion constant”

The author found a‘‘corrosion constant”8,which had a similar function to the ‘‘hubble constant” discovered by astronomer Edwin Hubble. The ‘‘hubble constant” means that the expansion rate of space is constant, thus the expansion of the interstellar is linear.Similarly,the‘‘corrosion constant”means that the corrosion rate between the corrosion damage and the corrosion life(time)is constant,thus the relationship between the corrosion damage and the corrosion life (time) is linear. With this linear relationship, only the ‘‘corrosion constant” is solved, and the metal calendar life of any damage value can be obtained without corrosion tolerance.From the perspective of application, a brief description is as follows.

3.2.1. Solution principle

(1) Calculation model of the metal calendar life using the‘‘corrosion constant”

The author gave the calculation model of the metal calendar life using the ‘‘corrosion constant” and the calculation model of the‘‘corrosion constant”k8,such as Eqs.(3)and(4).

where k is the ‘‘corrosion constant”. This constant defined by the solution concentration value for corrosion test dttimes thecorrosion test time λt(years) divides by the damage value Dtobtained by the corrosion tests. This value remains constant and not affected by the dtand length of λt, as long as these 3 parameters (Dt, λt, dt) are combined in this way8. D′c is the unknown corrosion damage value.

Table 5 Equal-damage temperature and humidity spectrum of Hainan province in one year.

Table 6 Corrosion test data of 30CrMnSiA.

(2) Obtain the ‘‘corrosion constant” k using the real parameters

①Obtain Dt, λt, dtthrough tests

The method for obtaining Dt, λt, dtin this section is the same as that in Section 3.1.2.

②Calculate the ‘‘corrosion constant” k

Substitute the Dt,λt,dtobtained from the tests into the Eq.(4) to obtain the value of k.

(3) Calculate the calendar life of any corrosion damage using ‘‘corrosion constant” k

By substituting the obtained ‘‘corrosion constant” k into Eq. (3), the corresponding metal calendar life λ can be calculated through any corrosion damage value. If an accurate metal corrosion tolerance can be given,the accurate metal calendar life can be easily obtained through the ‘‘corrosion constant” k.

3.2.2. Solution method and example

(1)Question:Using the‘‘corrosion constant”k to calculate the calendar life λ of any corrosion damage of LY12CZ aluminum alloy used in Hainan Island.

(2) Solution method and steps

①Prepared the high concentration dt,as shown in Table 4.② Temperature and humidity spectrum is shown in Table 5.

③Corrosion test:The test time was one cycle block time of the temperature and humidity spectrum in Table 5,i.e.,λt=1.The test solution concentration dt= 10, 20, 30, and the test data is shown in Table 7.

④k calculation: The k value was obtained by substituting average dt= 20, average damage Dt= 0.7467, and test time λt= 1 from Table 7 into Eq. (4).

⑤Calculated the calendar life λ of any corrosion damage of LY12CZ aluminum alloy with k.

Substituted k into Eq. (3), got the Eq. (5).

Eq.(5)was the calculation equation to calculate any corrosion damage with the corrosion constant for LY12CZ aluminum alloy serviced in Hainan Island. Three kinds of corrosion damage depth were given: D1= 0.2 mm,D2= 0.5 mm, D3= 0.8 mm, and the results as follow.

3.2.3. Focus points

(1) The ‘‘corrosion constant” specific values can be obtained by real corrosion parameters, which make the metal calendar life calculation very simple and more convenient for engineering applications.

(2)For reducing the experimental error,it is suggested that three kinds of concentrations dt, and the equal-damage temperature and humidity spectrum should be used in the tests to calculate the ‘‘corrosion constant”.

3.3. Determining the metal calendar life by T-H curve

It was found that there was a power function curve for metal corrosion2, i.e., the T-H (temperature-time) curve. Based on this curve, combined with damage mechanics and fatigue strength, a theoretical formula for calculating metal calendar life was finally given through rigorous mathematical derivation. From the perspective of application, a brief description is as follows.

3.3.1. Test T-H curve

According to the principle and method given by the author in the Ref.1, the T-H curves of the metal service conditions were tested, as shown in Fig. 4. This curve family was measured by corrosion damage D1, so the calendar life calculated from thecurve family data was corresponding to the corrosion damage D1. In Fig. 4, RH1= 100%, RH2= 90%, RH3= 80%.

Table 7 Corrosion damage values of LY12CZ aluminum alloy with the Hainan temperature and humidity spectrum and high concentration dt tests.

Fig. 4 Metal service T-H curve.

3.3.2. Formula

The author researched and gave a formula for calculating the metal calendar life by the T-H curve in the Ref.2, as shown in Eq. (6).

Where: λ is the calendar life when metal corrosion damage reaches Dc; hiis the hours of the i-th temperature in the temperature and humidity spectrum, which obtained from the temperature and humidity spectrum; Hiis the corrosion time when the metal corrosion damage reaches Dcusing the i-th temperature (T) test under the service media and a certain humidity(RH),which obtained from T-H curve;t is the number of temperature levels in the temperature and humidity spectrum; m is the number of humidity levels in the temperature and humidity spectrum.

3.3.3. Method and application example

(1) Question: Calculating the calendar life λ of LY12CZ aluminum alloy serviced in Hainan Island by T-H curve when the corrosion damage D reached 0.5 mm.

(2) Solution method and steps

①Compiled the equal-damage temperature and humidity spectrum, as shown in Table 5.

②Prepared the high concentration dt,as shown in Table 4.

③According to the test principle of Ref.1,the T-H curve of LY12CZ aluminum alloy was tested with Hainan Island media solution and D = 0.5 mm. The test data could be found on pages 363 and 367 of the Ref.1, and the data related to this question were extracted, as shown in Table 8 and Table 9.

④Found the corresponding value of hiand Hiin Eq. (6)and calculated ∑hi/Hi. Finding hifrom Table 5, and finding Hifrom Table 8 and Table 9,the value of ∑hi/Hiwere calculated as shown in Table 10.

⑤Calculated λ

Substituted the calculated value of ∑hi/Hiinto Eq.(6),the calendar life λ of LY12CZ aluminum alloy serviced in Hainan Island which corresponded to D = 0.5 mm could be calculated.

3.3.4. Application notes

(1) For any metals with a T-H curve law, Eq. (6) can be used to calculate the metal calendar life.

(2) When testing the T-H curve, the test principle of Ref.1should be used to measure the T-H curve of the service environment (d = 1) to ensure that the calculated calendar life is the service calendar life.

(3) The equal-damage temperature and humidity spectrum should be compiled in years by the principle of Ref.5to ensure that the calculated calendar life is in years.

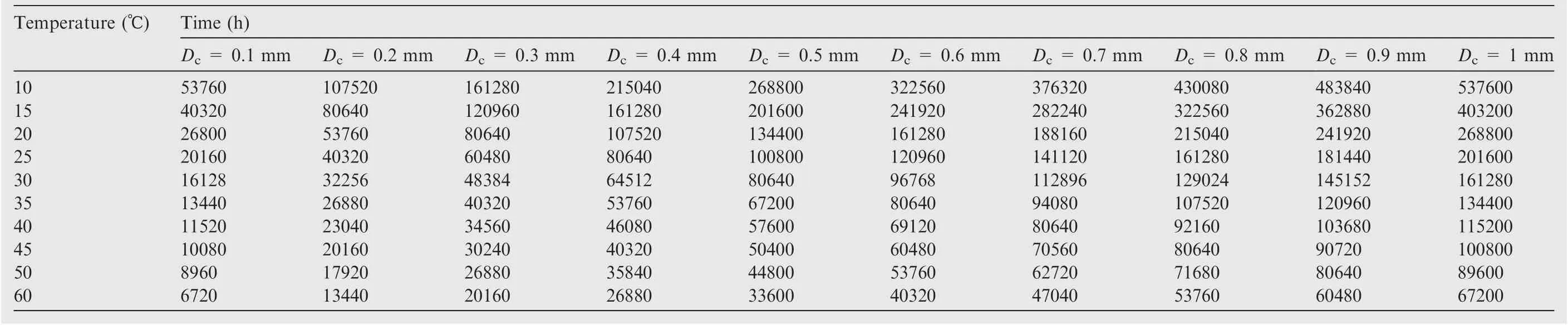
Table 8 T-H curve test data of LY12CZ aluminum alloy (RH =90%, d =1).

Table 9 T-H curve test data of LY12CZ aluminum alloy (RH =100%, d =1).

3.4.Comparison of the calendar life of LY12CZ aluminum alloy serviced in Hainan Island calculated by two theories, corrosion damage D = 0.5 mm

3.4.1.Calculated calendar life λ with the‘‘corrosion constant”k

From the application example in Section 3.2.2:

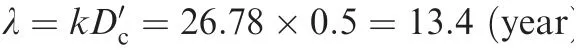
3.4.2. Calculated calendar life λ with T-H curve theory

From the application example in Section 3.3.3:

3.4.3. Comparison analysis

The results calculated by two different calculation theories had a relative error of 29%, which was acceptable in the field of international corrosion. The main source of this error was the T-H curve test. As long as the T-H curve was measured accurately, the error could be reduced.

3.4.4. Notes on equivalent conversion

Since these three metal calendar life calculation formulas in this paper obtain the calendar life of metal parts in actual use, the calendar life calculated by them does not require equivalent conversion. If the equal-damage high temperature and humidity spectrum compiled by the principles of this paper and the service concentration (d = 1) solution are used for the metal calendar life test,the results do not need equivalent conversion.In this way,the problem of metal calendar life determination and equivalent conversion in the field of international corrosion is solved.

4. Determining the protective coating calendar life

4.1. Theoretical formula and calculation method

The calendar life of the protective coating is one of the components of the total calendar life of parts. If the protective coating does not fail, the metal will not corrode, and the calendar life of parts will be extended.

However, in the field of international corrosion, the calendar life of the protective coating in the real service environment is still hard to determine.For this reason,the author developed the calculation formula and the determination method of the calendar life of the coating in the Ref.9. From the application needs, a brief description is as follows.

4.1.1. Theoretical formula

The author found that the failure time and temperature of some protective coatings also had a power function curve,which was consistent with the metal T-H curve9. According to this curve,the theoretical calculation formula of the coating calendar life was also derived, such as Eq. (7).

4.1.2. Method and application example

(1) Question: If the coating on the surface of LY12CZ aluminum alloy was used in Hainan Island,what was the calendar life of the coating failure?

(2) Solution method and steps

①Compiled the equal-damage temperature and humidity spectrum, as shown in Table 11.

②Prepared the medium solution for the service environment (d = 1) of Hainan Island, such as the medium with d = 1 in Table 4.

③Tested the T-H curve of the coating.The corrosion tests of three specimens at each temperature were carried out by using solution concentration d = 1 in Table 4 and temperatures at 25 ℃, 50 ℃and 65 ℃. The test data is shown in Table 12 and the test curve is shown in Fig. 5.

④Found the corresponding value of hiand H′′′iin Eq. (7)and calculated the ∑hi/H′′′i. Finding hifrom Table 11, andfinding the number of weekscorresponding to 40℃、50℃、60℃from Fig. 5, they were converted from weeks to hours (1 week = 168 hours), as shown in Table 13.

Table 10 hi, Hi and calculated value of ∑hi/Hi

Table 11 1-year equal-damage temperature and humidity spectrum in Hainan Island.

Table 12 Test data of T-H curve of the coating on the surface of LY12CZ aluminum alloy.

Fig. 5 T-H curve of the coating test.

⑤Calculated the calendar life λmof the coating on the surface of LY12CZ aluminum alloy

Substituted the value (0.1089) in Table 13 into Eq. (7) to calculate the λm.

4.1.3. Verification test

In order to verify the correctness and accuracy of the theoretical calculation Eq. (7) for determining the calendar life of the coating, the author also conducted a closed verification test in the Ref.9. On one hand, the calendar life of the coating obtained by Eq. (7) was 9.18 years, which kept under seal.On the other hand, another person used the same specimens and the same corrosion conditions to test and found that the calendar life of the coating failure was 12 years. Finally, at a professional conference, the theoretical calculation results and test results were announced separately and the relative error was 23.5%9. At that time, there was no theoretical formula for calculating the calendar life of coatings in the world,which stirred the surprise and interest in this theory of the participating experts.

4.1.4. Notes on equivalent conversion

Since the coating calendar life calculation formula in this article obtains the calendar life of the coating in actual use,the calculated calendar life does not require equivalent conversion.If the equal-damage high temperature and humidity spectrum compiled by the principles of this paper and the service concentration (d = 1) solution are used for the coating calendar life test, the results do not need equivalent conversion. In this way, the problem of coating calendar life determination and equivalent conversion in the field of international corrosion is solved.

4.1.5. Application notes

(1) For any coating with the T-H curve law, Eq. (7) can be used to calculate the calendar life.

(2)To test the T-H curve of the coating,a high temperature test should be used instead of a high concentration test.Different coatings have different temperature requirements.

(3) 1 year’s equal-damage temperature and humidity spectrum should be used and its temperature should be the same level as the temperature of the test T-H curve so as to calculate and determine calendar life.

5. Determining the total calendar life and the calendar overhaul period of parts

The total calendar life of the part is composed of the metal calendar life,the coating calendar life and the number of the overhaul. Metal calendar life and coating calendar life can be calculated according to this paper.The number of the overhaul can be found in the fatigue life determination.But how to combine these three parameters scientifically to give the total calendar life and the calendar overhaul period of the part is an internationally unsolvable problem. After years of research,the author finally solved this problem with a simple ‘‘graphic method”10.

5.1. Calculation formula

5.1.1. Assumption

The calendar life of each overhaul period of the part was y1,y2,...,yn,and they were equal.The total calendar life was Y.The life when the metal was corroded to the damage tolerance Dcwas λ. The protective coating had an effective life λm. The number of the overhaul was n. The validity period λmof the protective coating was equal after each overhaul. The life λ was equally distributed to each overhaul period.

5.1.2.Overall forecast diagram for determining the calendar life of parts

According to the internal relations and assumptions of the above-mentioned parameters, the Ref.10researched andobtained:the overall forecast diagram for determining the calendar life of parts, as shown in Fig. 6.

Table 13 Corresponding value of hi, H′′′i , and ∑hi/H′′′i .

Fig.6 Overall forecast diagram for determining the calendar life of parts (in the figure: η=).

5.1.3. Formula for determining the calendar life of parts

(1) The calculation formula of each overhaul period yiof parts, i.e., Eq. (8), was derived from Fig. 6.

(2)The calculation formula of total calendar life Y of parts,i.e., Eq. (9), was derived from Fig. 6.

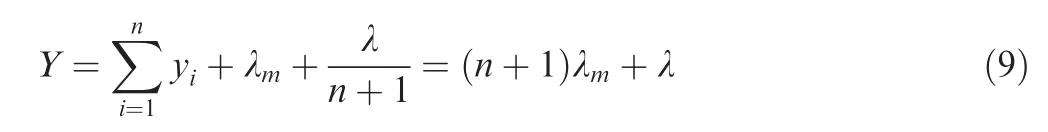
where y is the overhaul calendar life;Y is the total calendar life of the part;λ is the calendar life when the metal is corroded to the damage tolerance Dc; λmis one time effective calendar life of the protective coating.

5.2. Application example

5.2.1. Question

LY12CZ aluminum alloy part serviced in Hainan Island, its metal calendar life λ = 13.4 years, coating calendar life λm=12 years,the number of the overhaul n=3.How many years were the total calendar life Y and the overhaul calendar life y of the part?

5.2.2. Solution

(1) The overhaul calendar life y of the part

5.3. Application notes

(1) As be seen in Eq. (9), the total calendar life Y is greatly affected by the coating calendar life λm,which increases the life by a factor of(n+1).It can be seen that in order to extend Y,the best way is to increase λm.

(2)As be seen in Eq.(8)and Eq.(9),λ is an important part of Y and y.The length and accuracy of λ are controlled by the corrosion tolerance Dc.Therefore,to obtain accurate Y and y,accurate Dcmust be calculated.

6. Reliability processing of the calendar life of parts

The reliability processing of the calendar life of parts is to solve the problem of the reliability of the calendar life. The aforementioned calendar lives of metals and coatings were all calculated by theoretical calculations using damage values or test curves of corrosion tests. These tests were all carried out with the average temperature and humidity spectrum, the test results could only represent the average level, and the given calendar life was the average life too. The reliability of the average life was 50%, i.e., half of the given calendar life was reliable. Therefore, in order to improve the reliability of the calendar life of parts, reliability processing must be carried out. At present, in the field of international corrosion, there is no such article that has studied the above aspects. For this reason, the author conducted the research on the reliability of the calendar life in the Ref.11.

6.1. Scatter factor Lf of the metal calendar life

6.1.1. Relation between reliability and scatter factor

The author proposed that the scatter factor of the metal calendar life increased along with the increase of reliability through research,as shown in Table 14.Please refer to the original text of Ref.11for details.6.1.2.Method for improving the reliability of the metal calendar life

(1) The mean value of the metal calendar life was given by using the average temperature and humidity spectrum and load spectrum to test.

(2)Determined the high reliability level according to the use of parts. Different fields of parts, have different reliability requirements. For example, the reliability requirement for the aircraft structure is 99.9%, which means that structures of 999 aircrafts are guaranteed to be reliable out of 1000 aircrafts,but structures of only 1 aircraft are unreliable.This high reliability was converted into scatter factor through normal distribution curve and mathematical derivation.And high reliability meant high dispersion coefficient,as shown in Table 14.When determining the calendar life of parts, different scatter factors were directly selected to reflect the calendar life at different reliability. So different mechanical fields had different scatter factor requirements, which were reflected in their respective mechanical design specifications.

Table 14 Relationship between corrosion scatter factor Lf and reliability.

6.2. Scatter factor Lf of the protective coating calendar life

The scatter factor of the coating calendar life was studied by using the research principle and method in the Ref.11. Due to the lack of effective test data, only a scatter factor of reliability of LY12CZ aluminum alloy coating was given here.According to the test data of the coating on the surface of LY12CZ aluminum alloy in Table 12, the scatter factor value of the coating with the reliability of 99.9% was Lf= 1.2 after calculation. As the basic test data for this value were few, it was only used as a reference value.

6.3. Method for calculating the calendar life of high reliability parts

6.4. Concerns

(1)Reliability processing is an indispensable part of the calendar life determination of parts. It converts reliability into scatter factor according to probability mathematics. Lfis directly used in the determination of calendar life.

(2) The scatter factor of the calendar life of parts has not been studied in the international corrosion field,and the study in Ref.11is only the beginning. Theoretical research and engineering application research are also needed in different mechanical fields.

7. Determining the corrosion damage tolerance of the metal

Corrosion damage tolerance is the basic index for determining the calendar life of parts and corrosion damage inspection standard. Without an accurate corrosion damage tolerance,it is not only impossible to obtain an accurate calendar life,but also establish the inspection standard for corrosion damage. However, owing to the various forces on the parts and the numerous and complex corrosion factors,it was impossible to solve the corrosion damage tolerance value of the parts under the real load in the field of international machinery and corrosion.Until the publication of Ref.12in 2021,the corrosion tolerance of the parts under static load and fatigue load could be solved. Based on the research conclusion of the Ref.12,for the purpose of application,the following is selected.

7.1. Calculating the corrosion damage tolerance (Dc) of corroded parts under static load

7.1.1. Solution principle

(1)Relationship curve between stress σ and corrosion damage D

In Ref.12, the author conducted a comprehensive study of stress σ and corrosion damage D of corroded parts under static load. Finally, it was found that the stress of corroded parts under static load was approximately proportional to corrosion damage D before the middle and middle stages of corrosion,i.e., approximately linear, and exponentially distributed in the later stages, as shown in Fig. 7.

(2) Calculated the corrosion tolerance using the linear section of the curve in Fig. 7.

Set: In service tolerance stress of the critical parts was σp.

Find: In the linear section of the curve in Fig. 7, found the Dcby σp, as shown in the arrow line in Fig. 7.

7.1.2. Solution method and steps

In order to simplify the test,this section changes the"one-level high concentration test" to "service concentration test".

(1) Compiled the equal-damage high temperature and humidity spectrum for the service environment.

(2) Counted or measured the component and content of media in the service environment and prepared the service concentration (d = 1) solution.

(3)Simulated critical parts and manufactured specimens for corrosion and static loading tests.There were 4 groups of specimens, 3 in each group. If the parts for which the corrosion damage tolerance was to be obtained, and there was some damage,it should be simulated when manufacturing the parts.

(4) The corrosion test was carried out with the equaldamage high temperature and humidity spectrum and the service concentration(d=1)solution.The test time was different for materials with different corrosion rates, among which t1< t2< t3< t4. However, no matter what the specimen was, its test time t1was to ensure that the specimen had relatively obvious corrosion damage before stopping. The process was as follows:

①.For each specimen in one group, the static loading and corrosion test of t1spectrum blocks was carried out using the equal-damage high temperature and humidity spectrum and the service concentration solution. After that, the mean value of corrosion damage D1was first measured, and then the tensile test was conducted to measure the fracture stress σ1.

②.For each specimen in one group, the static loading and corrosion test of t2spectrum blocks was carried out using the equal-damage high temperature and humidity spectrum and the service concentration solution. After that, the mean value of corrosion damage D2was first measured, and then the tensile test was conducted to measure the fracture stress σ2.

Fig. 7 σ-D curve of corroded parts under static load.

③. For each specimen in one group, the static loading and corrosion test of t3spectrum blocks was carried out using the equal-damage high temperature and humidity spectrum and the service concentration solution. After that, the mean value of corrosion damage D3was first measured, and then the tensile test was conducted to measure the fracture stress σ3.

④. For each specimen in one group, the static loading and corrosion test of t4spectrum blocks was carried out using the equal-damage high temperature and humidity spectrum and the service concentration solution. After that, the mean value of corrosion damage D4was first measured, and then the tensile test was conducted to measure the fracture stress σ4.

⑤.Checking the σ-D curve,if it was not approximately linear, another test should be conducted by adjusting the corrosion time.

(5) The σ-D curve was drawn using σ1, σ2, σ3, σ4and D1,D2, D3, D4, as shown in Fig. 7.

(6) The corrosion damage tolerance Dcwas obtained by using the tolerance stress σpof the critical parts, as shown the arrow line in Fig. 7.

7.2. Calculating the corrosion damage tolerance of corroded parts under fatigue load

7.2.1. Solution principle

(1) Relationship between fatigue life N and corrosion damage D

In Ref.12, the author conducted a comprehensive study of fatigue life N and corrosion damage D of corroded parts under fatigue load. Finally, it was found that the relationship between the fatigue life N and corrosion damage D of the corroded parts under fatigue load conformed to the power function distribution curve, as shown in Fig. 8. Using this curve,the corrosion damage tolerance Dcof corroded parts under fatigue load could be obtained.

(2) Calculated the corrosion damage tolerance Dc

Set: The total fatigue life Npof the critical parts had been given by fatigue tests. Np=N-/Lf, N-is the mean test life. Lfis the scatter factor.

Find: In Fig. 8, found the Dcby Np.

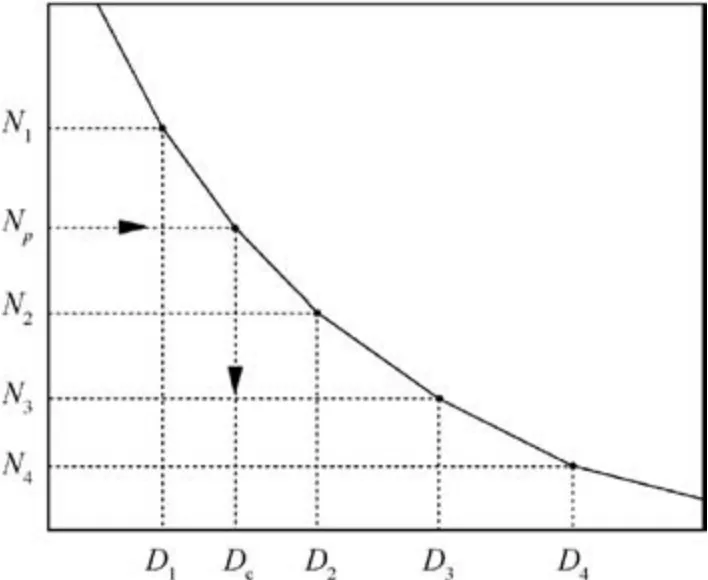
Fig. 8 N-D curve.

7.2.2. Solution method and steps

In order to simplify the test,this section changes the"one-level high concentration test" to "service concentration test".

(1) Compiled the equal-damage high temperature and humidity spectrum for the service environment.

(2) Counted or measured the component and content of media in the service environment and prepared the service concentration (d = 1) solution.

(3) Compiled fatigue load spectrum of the fatigue critical parts. The load spectrum for determining the fatigue life of parts could be used,but it should be matched with the temperature and humidity spectrum (One year’s temperature and humidity spectrum was matched with one year’s fatigue load spectrum).

(4) Simulated fatigue critical parts and manufactured specimens for corrosion and fatigue tests.If the parts for which the corrosion damage tolerance was to be obtained,and there was some initial damage,it should be simulated when manufacturing the parts.

(5)Calculated the corrosion damage of the service environment (d = 1):

①.For a group of 3 specimens,the t1spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t1was also used in the tests, and the fatigue tests were carried out at 0.5 t1. After that, the mean corrosion damage D1was measured.

②.For a group of 3 specimens,the t2spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t2was also used in the tests, and the fatigue tests were carried out at 0.5 t2. After that, the mean corrosion damage D2was measured.

③.For a group of 3 specimens,the t3spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t3was also used in the tests, and the fatigue tests were carried out at 0.5 t3. After that, the mean corrosion damage D3was measured.

④.For a group of 3 specimens,the t4spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t4was also used in the tests, and the fatigue tests were carried out at 0.5 t4. After that, the mean corrosion damage D4was measured.

(6) Determined the fatigue life N1, N2, N3and N4corresponding to D1, D2, D3and D4:

①.For a group of 5 specimens,the t1spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t1was also used in the tests, and the fatigue tests were carried out at 0.5 t1.Then,the fatigue life N1was measured by fatigue tests with the same load spectrum.

②.For a group of 5 specimens,the t2spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t2was also used in the tests, and the fatigue tests were carried out at 0.5 t2.Then,the fatigue life N2was measured by fatigue tests with the same load spectrum.

③.For a group of 5 specimens,the t3spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t3was also used in the tests, and the fatigue tests were carried out at 0.5 t3.Then,the fatigue life N3was measured by fatigue tests with the same load spectrum.

④.For a group of 5 specimens,the t4spectrum blocks corrosion tests were carried out by using the equal-damage high temperature and humidity spectrum and the service concentration solution. The fatigue load spectrum matched with t4was also used in the tests, and the fatigue tests were carried out at 0.5 t4.Then,the fatigue life N4was measured by fatigue tests with the same load spectrum.

Note:The corrosion test time t1

(7) The measured N1, N2, N3, N4, D1, D2, D3and D4were used to draw the N-D curve, as shown in Fig. 8.

(8)The total fatigue life Npof the critical parts was used to determine the corrosion damage tolerance Dc, as shown the arrow line in Fig. 8. Since the N-D curve was measured by the equal-damage high temperature and humidity spectrum and the service concentration (d = 1) solution, the damage in the test was consistent with the actual service damage, so the Dcobtained from the N-D curve did not require equivalent conversion.

7.2.3.Method for calculating corrosion tolerance Dcof the parts without total fatigue life Npby calendar life index

(1) The method in Section 7.2.2 of this paper was used to calculate the ‘corrosion + fatigue’ damage D1, D2, D3and D4under the condition of d = 1, and then the corresponding test time H1,H2,H3and H4was used to draw the H-D curve of‘corrosion + fatigue’7, as shown in Fig. 9.

(2)Used calendar life index Y,or looked for retired similar parts with basically the same service environment and stress status, and used its service calendar year Y.

Fig. 9 H-D curve of ‘corrosion + fatigue’

(3) On the H-D curve in Fig.9, the Dcwas calculated by Y(as shown by the arrow line). Although this Dcwas not an accurate value, it had reference value.

Note: The above method is also suitable for the approximate determination of corrosion tolerance of pure corrosion(without load) parts, but the fatigue load should be removed in the H-D curve testing.

7.3. Method for calculating corrosion damage tolerance of the parts under static load and fatigue load simultaneously

Originally, this problem is a very complex and unsolvable problem,but with the above research conclusions in this paper,this problem has been solved:

(1) The corrosion damage tolerance Dc1(Dc2) of the same part under static load (fatigue load) was calculated respectively. The specific method was as aforementioned, and was not repeated here.

(2)Dc1and Dc2were compared,and the smaller one was the corrosion damage tolerance value of the part.

8.To ensure that both the total fatigue life and the total calendar life of the part were all safe

8.1. Principle

The part works not only under fatigue load but also under corrosive environment,so it has both fatigue life and calendar life.These two kinds of life are given by fatigue field and corrosion field respectively.How to ensure the different life given by different fields is all safe? This is an international problem that the user department is looking forward to solving for a long time, the design department is pursuing to solve, and the research department is exploring to solve.

This paper solved this problem by studying corrosion damage tolerance of parts.Its principle was that the corrosion damage tolerance Dcof the parts was determined by the total fatigue life,and then the total calendar life of the parts was calculated by using this Dcand the corresponding calculation formula.Both the total calendar life and the total fatigue life were all safe.

8.2. Method and steps

(1)The total fatigue life Npof the part was obtained by fatigue tests with the real load spectrum.

(2) Calculated the total calendar life Y:

①The N-D curve was drawn through corrosion test and fatigue test by using the real load spectrum and temperature and humidity spectrum, as shown in Fig. 8.

②The total fatigue life Npwas used to calculate the corrosion damage tolerance Dcthrough the N-D curve,as shown the arrow in Fig. 8.

③Used Dcand Eq. (2) to calculate the calendar time λ of the pure metal part.

④Use λ and Eq.(9)to calculate the total calendar life Y of the part.

(3) Analysis of both Npand Y safety

According to Eq. (9), λmwas the effective calendar life of the protective coating, meaning that the metal was noncorrosive during the effective life λm. If the metal did not corrode,the total fatigue life Npwould not be affected.Therefore,(n + 1)λmonly affected the total calendar life Y of parts, and did not affect the total fatigue life Npof parts. As a result, in the total calendar life Y, only the pure metal corrosion calendar life λ was associated with the total fatigue life Np, as long as λ was safe, Npwas safe.

Since the λ in the total calendar life Y was calculated by the metal corrosion damage tolerance Dcand Eq. (2), and the Dcwas determined by the total fatigue life Npand N-D curves,Dcwas the intermediate ‘‘coordinator” between λ and Np. As long as the metal corrosion damage value Dtof the part in use was less than or equal to Dc, both λ and Npwere safe,i.e., both Y and Npwere safe. This reflected the scientificity and superiority of using Npto determine Dc.

9. Matching design, in-service and determining sequence of the total fatigue life Np and the total calendar life Y of the same part

9.1. Np and Y matching design

9.1.1. Npanalysis

When the material,structure and fatigue load of the parts were unchanged, the total fatigue life Npof the parts given by the test was not allowed to be changed without life extension.That was to say, the total fatigue life Np(hours) was a constant value.

9.1.2. Y analysis

According to the calculation Eq.(9)of the total calendar life,λ was the corrosion time (year) when the pure metal of the part was corroded to the damage tolerance Dc. In the case of the corrosive environment and structure unchanged, generally λ was a fixed value.In the(n+1)λmof Eq.(9),λmwas one time effective calendar life(year)of the protective coating,n was the overhaul number of the part.λmwas variable in the part overhaul process, and the total calendar life Y could be increased or decreased by increasing or decreasing λm. That was, the total calendar life Y of the part was adjustable.

9.1.3. Npand Y matching design and use

Theoretically speaking, Npwas a fixed value, which could be converted into the total service life Y0by the average use intensity (hours of use per year). In the design of the total calendar life Y of the part, by adjusting the λmin the Eq. (9), made Y = Y0, so as to realize the matching design of the total fatigue life Npand the total calendar life Y of the same part.

The service life of the total fatigue life Npwas related to the service strength of the parts. High using intensity, low service life.Otherwise,low using intensity,high service life.It led to a mismatch between the service life of the total fatigue life Npand the total calendar life Y. For this reason, when the part was in use,attention should be paid to adjusting the use intensity, so that the service life of Npmatched with Y as much as possible.

9.2. Determining sequence of Np and Y

As for the sequential problem of Npand Y determination of the same part, based on the study of metal corrosion damage tolerance in this paper, the sequence of their determination was as follows: first,the Npwas determined, and then the corrosion damage tolerance Dcwas determined according to Npand N-D curve; Secondly, according to the Dc, calculated the metal corrosion time λ; finally, the total calendar life Y could be calculated with λ, one time effective calendar life λmof the protective coating, and the overhaul number n. That’s the sequence from Npto Y.

10. General principles for determining the calendar life of the aircraft

(1) The total calendar life of the aircraft is determined by the total calendar life of each critical parts, and the smallest calendar life value is selected as the total calendar life of the aircraft.

(2) The calendar life of non-critical parts with corrosion does not need to be given by the method of determining the calendar life of critical parts. As long as the corrosion protection and partial repairs are used to ensure that there is no corrosion or slight corrosion but do not affect the total calendar life during an overhaul period.

(3) For non-critical parts without corrosion,follow normal corrosion protection and repair.

11. Conclusion

In order to facilitate the application, the author summarized his papers on calendar life research for many years and came to the following conclusions.

(1) By using the conversion principle and method of equal corrosion damage developed in Ref.1, the temperature and humidity spectrum of equal corrosion damage was compiled.

(2)According to the principle that corrosion damage D was proportional to concentration d in Ref.7,a high concentration d solution was prepared for the test.

(3) Using the theoretical formula developed in Ref.7, the calendar life of the metal contained a certain corrosion damage was calculated.

(4) The ‘‘corrosion constant” developed in Ref.8was used to calculate the calendar life of any corrosion damage.

(5) The theoretical formula and T-H curve developed in Ref.2were used to calculate the calendar life of the metal contained a certain corrosion damage.

(6)Using the theoretical formula and T-H curve developed in Ref.9, the calendar life of the protective coating failure was calculated.

(7) The total calendar life and overhaul calendar life were calculated by using the theoretical formula developed in Ref.10.

(8) The scatter factor studied in Ref.11was used to obtain the calendar life with high reliability.

(9)The solution principle and method of corrosion damage tolerance developed in Ref.12were used to determine the corrosion damage tolerance value under different loads.

(10) Based on the principle studied in Ref.12, the principle and matching design method that the total fatigue life and the total calendar life of the same part were both safe were given.

(11) General principles for determining the calendar life of the aircraft were given.

(12) This set of theories and methods is suitable for determining the corrosion tolerance and calendar life of corrosive machinery and equipment used in the atmosphere, marine and soil environments,and has been successfully used in determining the calendar life of an aircraft.

This set of calendar life determination system, its various tests and theoretical calculations are carried out with the corrosion parameters and loads of the real service environment,and finally give a real and reliable calendar life.Using this calendar life to control the service time of machinery and equipment can eliminate corrosion disasters and economic losses that have been unable to solve internationally.This is the goal that this system can achieve. In order to achieve this goal, in addition to providing this new theory and method to the international corrosion field,the author also provides free consultation to application units and individuals.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.