一类扩散食物链模型正解的多重性和唯一性
李海侠
(宝鸡文理学院数学与信息科学学院,陕西 宝鸡 721013)
一类扩散食物链模型正解的多重性和唯一性
李海侠
(宝鸡文理学院数学与信息科学学院,陕西 宝鸡 721013)
研究了一类具有Crowley-Martin反应函数的食物链模型。首先利用全局分歧理论和度理论讨论了正解的存在性和多重性,得到了正解分歧的基本形状。接着运用扰动理论给出了正解的唯一性和稳定性条件,结果表明当d充分大时系统在一定条件下存在唯一且稳定的正解。
Crowley-Martin反应函数;分歧;扰动;多重性;唯一性
本文在齐次Robin 边界条件下讨论如下具有C-M反应函数的食物链模型
其中 Ω∈RN是带有光滑边界∂Ω的有界区域。系统(1)是食物链模型,其中u是食饵,v是以u为食物的捕食者,w是以v为食物的捕食者。r1和r3是食饵u和捕食者w的增长率。r2是捕食者v的死亡率。a1,a2,c和d分别代表了捕食者v的捕获率、转化率、处理时间和捕食者间的干扰度。u0(x),

(1)
当系统(1)没有捕食者w且d=0时,系统(1)变为具有Holling-II反应函数的两物种捕食-食饵模型。文献[12-14]在Neumann边界条件下运用分歧理论研究了非常数正解的不存在性和存在性以及周期解的存在性。如果系统(1)没有捕食者w,则系统(1)变为具有C-M反应函数的两物种捕食-食饵模型。文献[15]在Neumann边界条件下考察了系统平衡态正解的图灵不稳定性和Hopf分歧。
本文将重点考虑系统(1)对应的平衡态系统
(2)
正解的存在性、多重性、稳定性和唯一性。
令

为了得到重要结论,这节最后给出一些预备知识。

则λ1(q)连续依赖q,λ1(q)是简单的。而且,如果q1≤q2,q1≢q2,则λ1(q1)<λ1(q2)。为了简单起见,记λ1(0)为λ1,相应于λ1的主特征函数记为 ϑ1,ϑ1>0且‖ϑ1‖∞=1。
考虑如下非线性问题
-Δu=u(r-u),x∈Ω;

(3)
众所周知,若r>λ1,则(3)有唯一正解。定义唯一正解为Θr。特别地,Θr≤r且Θr连续依赖r。
1 正解的存在性和多重性
本小节应用不动点指数理论和分歧理论讨论系统(2)正解的存在性和多重性条件。首先类似文献[17]定理2的证明给出系统(2)共存解的先验估计。
引理1 如果a2r1>r2(1+r1c),则系统(2)的任意共存解(u,v,w)有先验估计
u(x)≤r1,v(x)≤Q,w(x)≤r3+b2
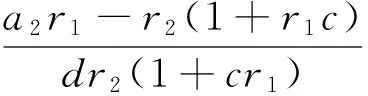
注1 下面总假设a2>max{r2(1+r1c)/r1,cλ1}。
引理2 如果系统(2)有共存解,则r1>λ1,r2<Λ,r3+b2>λ1。
利用引理2和上下解方法易得



接下来,以r2为分歧参数研究系统(2)关于强半平凡解(Θr1,0,Θr3)的分歧。为了应用分歧理论引入如下空间:
令U=Θr1-u,V=v,W=w-Θr3,则U,V,W>0满足
(4)
其中
G1(U,V,W)=U2+(Θr1-U)·

G2(U,V,W)=
G3(U,V,W)=

令
G(U,V,W)=
(G1(U,V,W),G2(U,V,W),G3(U,V,W))
显然,G(0,0,0)=0且G连续。而且,Fréchet导数D(U,V,W)G(0,0,0)=0。令K是带有Robin边界条件的-Δ的逆,则问题(4)成为
定义T:R+×E→E为
T(r2,U,V,W)=
KG1(U,V,W),
则T(r2,U,V,W)是可微紧算子。令F=I-T。显然,F是C1函数且F(r2,0,0,0)=0。而且,F(r2,U,V,W)=0当且仅当(r2,Θr1-U,V,Θr3+W)是系统(2)的非负解。

‖φ1‖2=1,
接着,将局部分歧延拓为全局分歧。
令r2,i(μ)(μ≥1)是如下特征值问题的特征值







-ΔU=r1U,x∈Ω;

x∈Ω;


下面应用不动点指数理论讨论系统(2)共存解的多重性。为此,记



W=K⊕K⊕K,
D={(u,v,w)∈W|u(x) v(x) 定义算子At:D→W为 At(u,v,w)=(-Δ+q)-1· 接下来运用类似文献[19]的方法给出算子A在平凡解和半平凡解处的不动点指数,在此省略其证明。 引理4 (i) indexW(A,D)=1; (ii) 若r1≠λ1,r3>λ1,则indexW(A,(0,0,0))=0; (iii) 设r1>λ1。若r2<Λ,r3>λ1,则indexW(A,(Θr1,0,0))=0; (iv) 设r3>λ1。若r1>λ1,则indexW(A,(0,0,Θr3))=0; 下面计算A在P1的指数。结合引理4用类似于文献[19]的方法有 引理5 若r1>λ1,r2<Λ,r3+b2>λ1, 则indexW(A,P1)=0。 由引理4、引理5和度的可加性得系统(2)正解的存在条件。 这里ν∈[0,1]。显然系统(2)有非负解当且仅当A1在D中有不动点。 令D1=DDδ。由引理1可知Aν在∂D1上没有不动点。于是根据不动点指数的同伦不变性可知indexW(A1,D1)=indexW(A0,D1)。显然A0在D1内有不动点(0,0,0),(Θr1,0,0),(0,0,Θr3)。用Dancer指数定理易证得在已知条件下A0在平凡解和半平凡解处的指数都为0。于是,indexW(A1,D1)=indexW(A0,D1)=1。最后结合引理4和引理5得 indexW(A1,D1)= indexW(A1,(0,0,0))+indexW(A1,(Θr1,0,0))+ indexW(A1,(0,0,Θr3))+indexW(A1,P1) 这表明系统(2)在DDδ内至少有一个正解。 图1 系统(2)正解可能的分歧曲线(a) 定理2条件下;(b) 定理4条件下Fig.1 Possible bifurcation diagrams of positive solutions to system (2)(a) under the conditions of Theorem 2;(b) under the conditions of Theorem 4 这一节考察当d充分大时系统(2)正解的稳定性和唯一性。我们注意到当d充分大时,系统(2) 的正解只有一种类型。也就是说,如果(u,v,w)是系统(2)的任意正解,则对于充分大的d,(u,dv,w) 接近于如下问题的正解: (6) 如果r1>λ1,则问题(6)等价于 (7) 首先,我们给出系统(7)正解非退化和线性稳定的条件。 证明假设χ是系统(7)的正解,则由系统(7)可得 (8) 接下来,证明系统(7)任意正解的稳定性。假设χ是系统(7)的任意正解。考虑如下特征值问题 x∈Ω; (9) 由(9)得 于是唯一性得证。 又因为wi≥Θr3, 所以考虑如下两种情况: (i) 如果v≡0。则(ui,vi,wi) 接近于(Θr1,0,Θr3),与假设矛盾; (ii)如果v≥0,≢0,w>0。由Harnack不等式得v>0。于是h=0,v≡0。同情况(i)与假设矛盾。 这表明 ‖u-Θr1‖C1+‖v‖C1+‖w-Θr3‖C1≤σ 成立。 x∈Ω; (10) 设divi=χi。则χi满足 (11) x∈Ω; (12) ; 最后给出系统(2)正解的稳定性和唯一性条件。 (13) (14) (15) 由式(15)的第一和第三个方程得ξ≡0,ζ≡0。如果η≢0,则由式(15)的第二个方程可得 此矛盾表明系统(2)的任意共存解(如果存在) 是非退化和线性稳定的。 1=indexW(A,D)= 这表明系统(2)存在唯一正解。 本文在齐次Robin边界条件下考察了一类扩散食物链模型共存解的存在性、多解性、稳定性和唯一性。研究结果表明当捕食者间的相互干扰度不强且物种v的死亡率在一定范围内时,三物种出现多重共存态;当捕食者间的相互干扰度非常强且物种v的死亡率不超过某临界值时,三物种出现唯一的稳定共存态。 [1] WANG M, WU Q. Positive solutions of a prey-predator model with predator saturation and competition [J]. J Math Anal Appl, 2008, 345(2): 708-718. [2] WEI M H, WU J H, GUO G H. The effect of predator competition on positive solutions for a predator-prey model with diffusion [J]. Nonlinear Analysis, 2012, 75(13): 5053-5068. [3] DONG Q L, MA W B, SUN M J. The asymptotic behavior of a Chemostat model with Crowley-Martin type functional response and time delays [J]. Journal of Mathematical Chemistry, 2013, 51(5): 1231-1248. [4] LIU X Q, ZHONG S M, TIAN B D, et al. Asymptotic properties of a stochastic predator-prey model with Crowley-Martin functional response [J]. Journal of Applied Mathematics and Computing, 2013, 43(1/2): 479-490. [5] 李海侠, 李艳玲. 一类带有Crowley-Martin反应函数的捕食-食饵模型的定性分析[J]. 中山大学学报(自然科学版), 2014, 53(5): 66-72. LI H X, LI Y L. Qualitative analysis for a predator-prey system with Crowley-Martin functional response [J]. Acta Scientiarum Naturalium Universitatis Sunyatseni, 2014, 53(5): 66-72. [6] MENG X Y, HUO H F, XIANG H, et al. Stability in a predator-prey model with Crowley-Martin function and stage structure for prey [J]. Applied Mathematics and Computation, 2014, 232(3): 810-819. [7] LI H X. Asymptotic behavior and multiplicity for a diffusive Leslie-Gower predator-prey system with Crowley-Martin functional response [J]. Computers and Mathematics with Applications, 2014, 68: 693-705. [8] ZHOU J. Qualitative analysis of a modified Leslie-Gower predator-prey model with Crowley-Martin functional responses [J]. Communications on Pure and Applied Analysis, 2015, 14(3): 1127-1145. [9] LI S B, WU J H, DONG Y Y. Uniqueness and stability of a predator-prey model with C-M functional response [J]. Computers and Mathematics with Applications, 2015, 69(10): 1080-1095. [10] DONG Y Y, LI S B, LI Y L. Multiplicity and uniqueness of positive solutions for a predator-prey model with C-M functional response [J]. Acta Applicandae Mathematicae, 2015, 139(1): 187-206. [11] TRIPATHI J P, TYAGI S, ABBAS S. Global analysis of a delayed density dependent predator-prey model with Crowley-Martin functional response [J].Communications in Nonlinear Science and Numerical Simulation, 2016, 30(1/2/3): 45-69. [12] WONLYUL K, KIMUN R. Qualitative analysis of a predator-prey model with Holling type II functional response incorporating a prey refuge [J]. J Differential Equations, 2006, 231(231): 534-550. [13] YI F Q, WEI J J, SHI J P. Bifurcation and spatiotemporal patterns in a homogeneous diffusive predator-prey system [J]. J Differential Equations, 2009, 246(5): 1944-1977. [14] PENG R, SHI J P. Non-existence of non-constant positive steady states of two Holling type-II predator-prey systems: Strong interaction case [J]. J Differential Equations, 2009, 247(3): 866-886. [15] SAMBATH M, GNANAVEL S, BALACHANDRAN K. Stability and Hopf bifurcation of a diffusive predator-prey model with predator saturation and competition [J]. Applicable Analysis, 2013, 92(12): 2439-2456. [16] KUANG Y, BERETTA E. Global qualitative analysis of a ratio-dependent predator-prey system [J]. J Math Biol, 1998, 36(4): 389-406. [17] 姜洪领. 一类蜘蛛-昆虫模平衡态正解的存在性[J]. 中山大学学报(自然科学版), 2016, 55(3): 64-67. JIANG H L. The existence of steady-state positive solutions for a spider-insect model [J]. Acta Scientiarum Naturalium Universitatis Sunyatseni, 2016, 55(3): 64-67. [18] WU J H. Global bifurcation of coexistence state for the competition model in the chemostat [J].Nonlinear Analysis, 2000, 39(7): 817-835. [19] LI H X, LI Y L, YANG W B. Existence and asymptotic behavior of positive solutions for a one-prey and two-competing-predators system with diffusion [J]. Nonlinear Analysis: Real World Applications, 2016, 27: 261-282. Multiplicityanduniquenessofpositivesolutionstoadiffusivefood-chainmodel LIHaixia (Institute of Mathematics and Information Sciences, Baoji University of Arts and Sciences, Baoji 721013, China) A food-chain system with Crowley-Martin functional response is studied. By making use of the global bifurcation theory and degree theory, the existence and multiplicity of positive solutions are discussed and the basic shape of bifurcation curve of positive solutions is obtained. Moreover, the conditions of the uniqueness and stability of positive solutions are given by means of perturbation theory. It turns out that there exists a unique asymptotically stable positive solution under certain conditions whendis sufficiently large. Crowley-Martin functional response;bifurcation; perturbation;multiplicity;uniqueness O175.26 A 0529-6579(2017)05-0051-09 10.13471/j.cnki.acta.snus.2017.05.007 2016-09-19 国家自然科学基金(11501496,11401356);陕西省教育厅专项科研计划(16JK1046);陕西省自然科学基础研究计划(2014JQ2-1003) 李海侠(1977年生), 女;研究方向偏微分方程计算及其可视化;E-mail: xiami0820@163.com








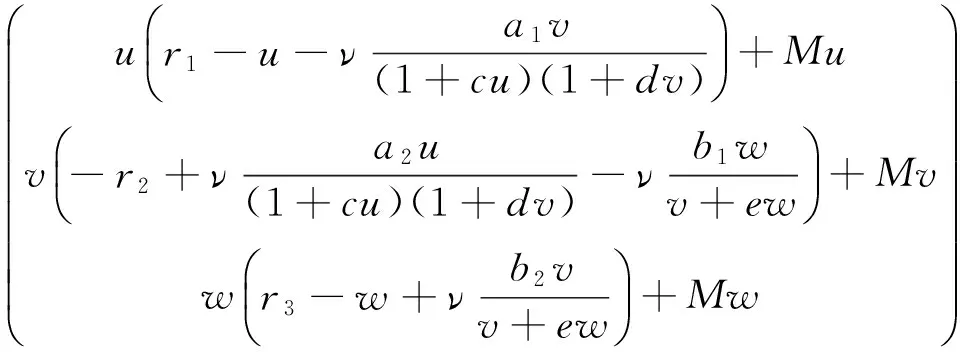



2 正解的唯一性和稳定性



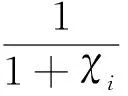






















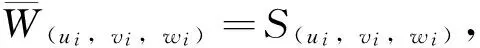

3 结 论

