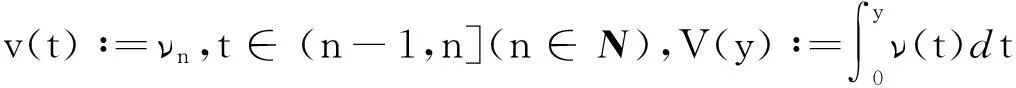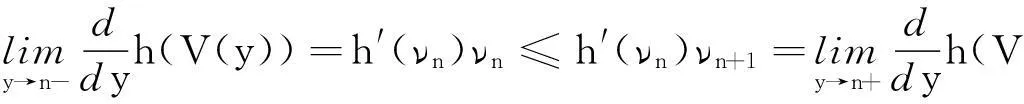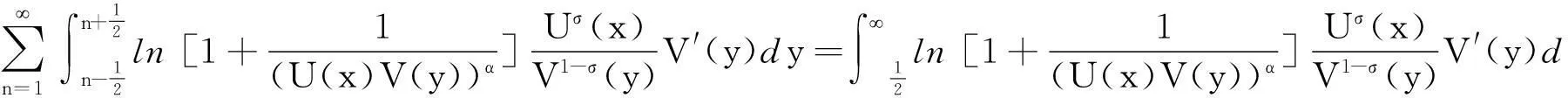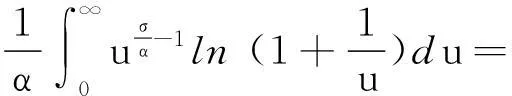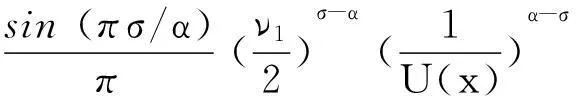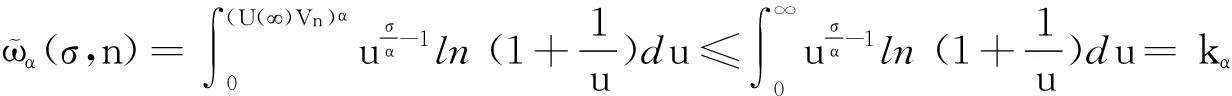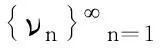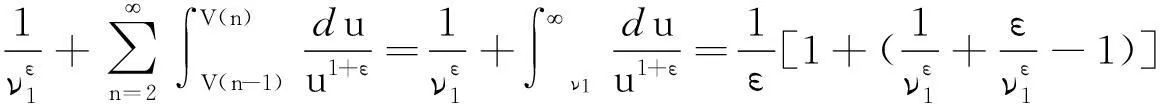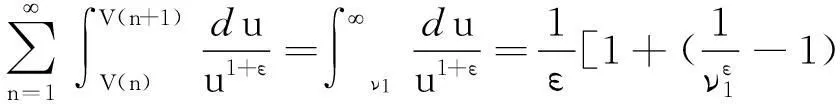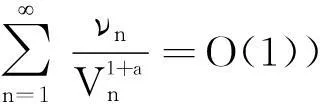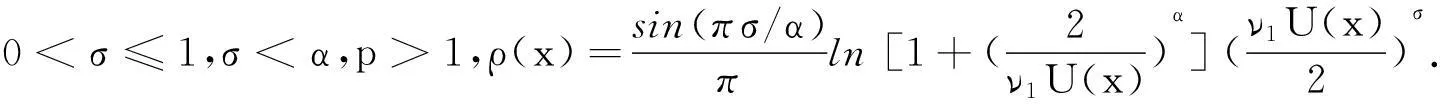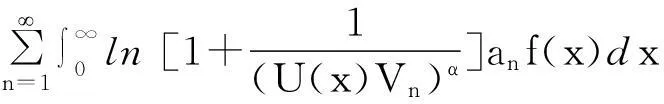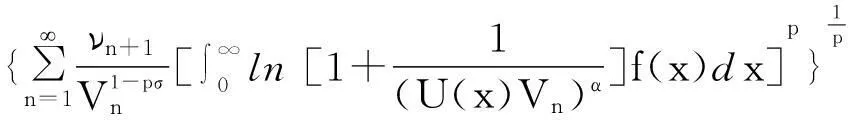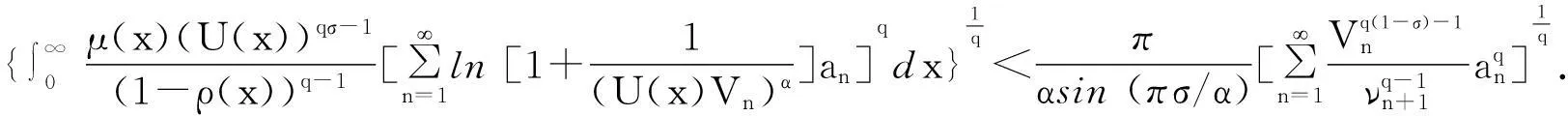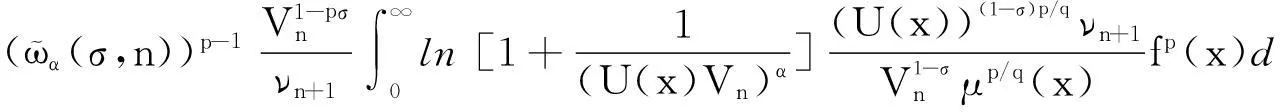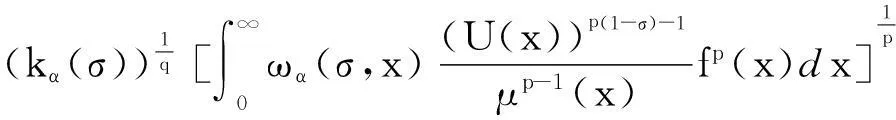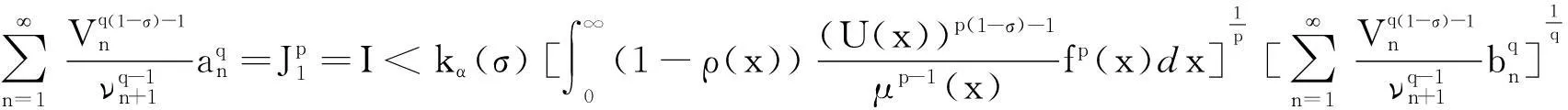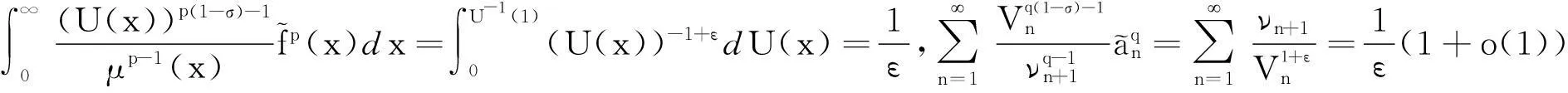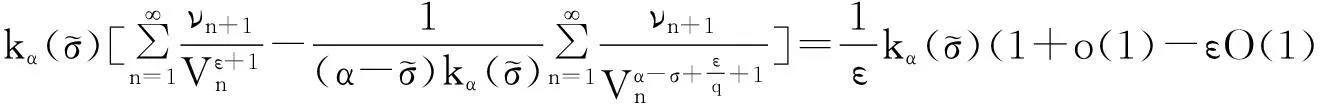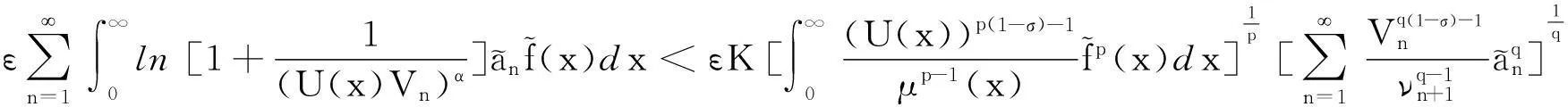一个半离散非齐次核Hardy-Hilbert型不等式的加强

(广东第二师范学院 数学系, 广东 广州 510303)



一个半离散非齐次核Hardy-Hilbert型不等式的加强

黄启亮, 杨必成

(广东第二师范学院 数学系, 广东 广州 510303)

摘要:引入独立参数, 应用权函数的方法及Hermite-Hadamard不等式, 建立一个具有最佳常数因子的加强的半离散非齐次核Hardy-Hilbert型不等式,还考虑了其等价式.

关键词:Hardy-Hilbert型不等式;参数;权函数;等价式;加强

0引言

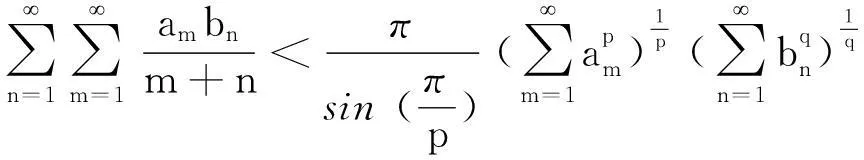
(1)

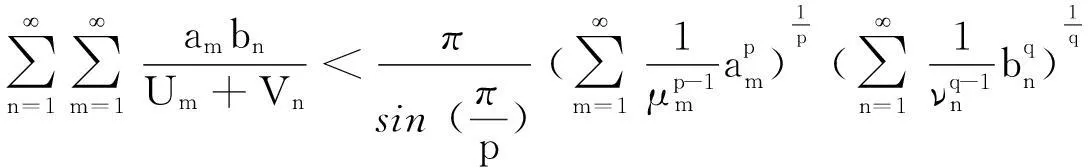
(2)

当μi=νi=1(i=1,2,…)时,式(2)变为式(1).

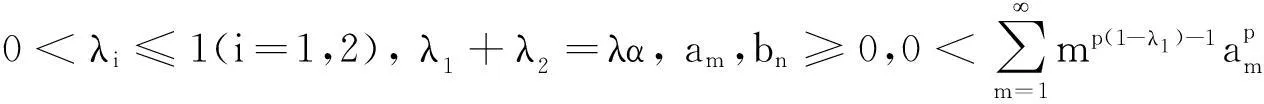
(3)

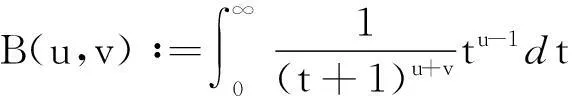
(4)

2009—2011年,杨必成在文[5-6]中论述了引入参量的、离散的Hilbert型不等式理论. 2015年,在类似条件下,文[7]给出如下一个式(2)及式(3)的具有最佳常数因子的推广式:

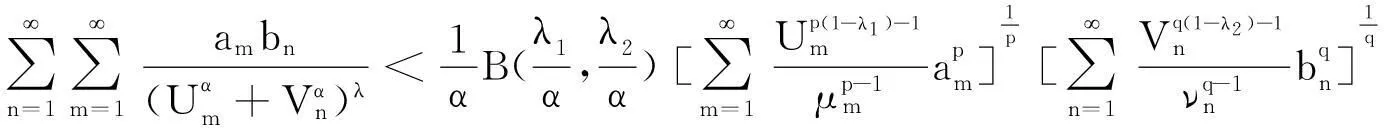
(5)

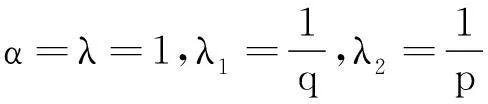
关于半离散及全平面Hilbert不等式的一些最新结果,可参阅文[8-11].

本文引入独立参数,应用权函数的方法及Hermite-Hadamard不等式,建立一个类似于式(5)的具有最佳常数因子的加强的半离散非齐次核Hardy-Hilbert型式,还考虑了其等价式.

1 一些引理

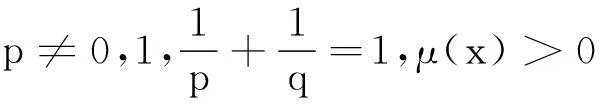
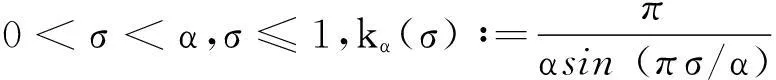
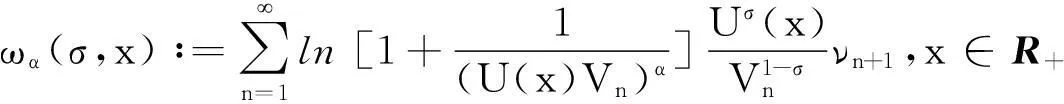
(6)

(7)

则有不等式:

ωα(σ,x)

(8)

ϖα(σ,n)≤kα(σ)(n∈N;0<σ<α).

(9)

对上式作变换u=(U(x)V(y))α,有

我们还有

(10)

(11)

2等价不等式及其最佳常数因子

(12)

(13)

(14)

证明配方,并由带权的Hölder不等式[12],有

(15)

由式(9),即有

(16)

再由式(8),即有式(13). 配方,由Hölder不等式[12],有

(17)

故式(13)成立且与式(12)等价.

同理可证式(14)成立且与式(12)等价.故式(12),式(13)与式(14)齐等价.证毕.

若有正常数K≤kα(σ),使取代式(12)的常数因子kα(σ)后仍成立,则特别有

(18)且由定理2的证明知式(18)的常数因子也是最佳值.

参考文献：

[1]HARDYGH.NoteonatheoremofHilbertconcerningseriesofpositiveterms[J].ProceedingsLondonMathSoc, 1925, 23(2):RecordsofProc.xlv-xlvi.

[2]HARDYGH,LITTLEWOODJE,POLYAG.Inequalities[M].Cambridge:CambridgeUnivPress, 1952.

[3]YANGBi-cheng.OnbestextensionsofHardy-Hilbert’sinequalitywithtwoparameters[J].JournalofInequalitiesinPureandAppliedMathematics, 2005, 6(3):Article81.

[4] 王竹溪,郭敦仁.特殊函数论[M].北京:科学出版社,1979.

[5] 杨必成.算子范数与Hilbert型不等式[M].北京:科学出版社,2009.

[6]YANGBi-cheng.DiscreteHilbert-typeinequalities[M].Sharjah,TheUnitedArabEmirates:BenthamSciencePublishersLtd, 2011.

[7] 杨必成. 一个推广的Hardy-Hilbert型不等式[J].广东第二师范学院学报,2015,35(3):1-7.

[8] 杨必成,陈强. 一个含对数核半离散的Hilbert型不等式[J].上海大学学报(自然科学版),2014,20(6): 726-732.

[9]HUANGQi-liang,WANGAi-zhen,YANGBi-cheng.Amoreaccuratehalf-discreteHilbert-typeinequalitywithageneralnon-homogeneouskernelandoperatorexpressions[J].MathematicalInequalitiesandApplications, 2014,17(1):367-388.

[10]WANGAi-zhen,YANGBi-cheng.Amoreaccuratereversehalf-discreteHilbert-typeInequality[J].JournalofInequalitiesandApplications, 2015:85.

[11]GUZhao-hui,YANGBi-cheng.AHilbert-typeintegralinequalityinthewholeplanewithanon-homogeneouskernelandafewparameters[J].JournalofInequalitiesandApplications, 2015:314.

[12] 匡继昌.常用不等式[M].济南:山东科技出版社,2004.

收稿日期：2015-12-09

基金项目:国家自然科学基金资助项目(61370186)

作者简介：黄启亮,男,广西桂林人,广东第二师范学院数学系教授.

中图分类号：O 178

文献标识码：A

文章编号：2095-3798(2016)03-0008-05

A Strengthened Version of a Half-Discrete Hardy-Hilbert-Type Inequality with a Non-Homogeneous Kernel

HUANG Qi-liang, YANG Bi-cheng

(Department of Mathematics, Guangdong University of Education,Guangzhou, Guangdong， 510303, P. R. China)

Abstract:By applying the way of weight functions and Hermite-Hadamard’s inequality, a strengthened version of a half-discrete Hardy-Hilbert-type inequality with a non-homogeneous kernel and a best possible constant factor is provided. Furthermore, the strengthened equivalent forms are considered.

Key words:Hardy-Hilbert-type inequality; parameter; weight function; equivalent form; strengthened version