两个Gamma商函数的对数完全单调性

刘媛，王连堂

（西北大学数学学院，陕西 西安　710127）

两个Gamma商函数的对数完全单调性

刘媛，王连堂

（西北大学数学学院，陕西 西安710127）

主要研究了Gamma商函数的对数完全单调性.通过引入一个新的辅助函数证明了一类Gamma商函数是严格对数完全单调的，对此类函数的系数进行了推广并证明了推广后的这类Gamma商函数也是严格对数完全单调的.

Gamma函数；Psi（digamma）函数；Polygamma函数；对数完全单调性

1　引言

Gamma函数定义为：

lnΓ（x）的导函数称为psi函数（digamma函数）记为ψ（x），即

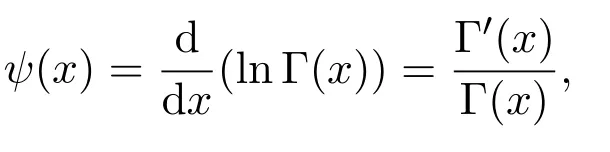
ψ（x）的n阶导函数ψ（n）（x）称为polygamma函数.

所谓Gamma商函数是指关于某些Gamma函数的商，关于Gamma商函数的完全单调性的研究是非常有意义的课题.文献［1］证明了函数在区间（0，+∞）上为严格对数凹的.文献［2］推广了这一结果，证明了G（x）的倒数函数G-1（x）在区间（0，+∞）上是严格对数完全单调的.文献［3］证明了函数在区间（0，+∞）上是对数完全单调的，其中m≥2是整数.

对数完全单调概念的提出，为研究函数的完全单调性提供了简便的方法.本文通过引入一个新的辅助函数证明了当m为大于1的正实数时，F（x）是严格对数完全单调的，从而推广了文献［3］中的结果.另外，也证明了关于F（x）的一个推广的函数（即定理3.2中的h（x））是严格对数完全单调的.

2　定义及引理

定义 2.1［4］函数f在区间I上称为是完全单调的，若函数f在区间I上有任意阶导数，并且对于任意x∈I以及n=0，1，2，···，满足（-1）nf（n）（x）≥0；若此不等式是严格的，则称f是严格完全单调的.

定义 2.2［5］正函数 f在区间 I上称为是对数完全单调的，若对于任意 x∈I以及n=0，1，2，···，满足（-1）n［lnf（x）］（n）≥0；若此不等式是严格的，则称f是严格对数完全单调的.

文献［5］中证明了（严格）对数完全单调函数一定是（严格）完全单调的.

引理2.1［6］当x∈（0，+∞）时，有

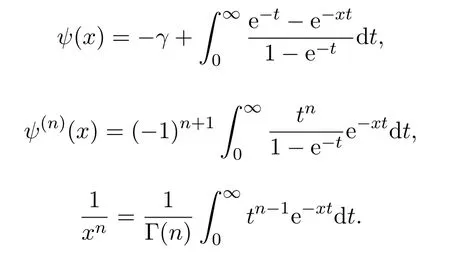
引理 2.2［6］函数 f（x）在区间 （0，+∞）上完全单调的充要条件是存在单调递增的函数α（t），使得f（x）=∫∞0e-xtdα（t）.

引理 2.3［7］（Laplace变换的卷积定理）设函数fi（t）（i=1，2）在任意有限区间上是分段连续的，包括（0，+∞）.如果存在常数Mi＞0和ci≥0，有|fi（t）|≤Miecit（i=1，2），那么

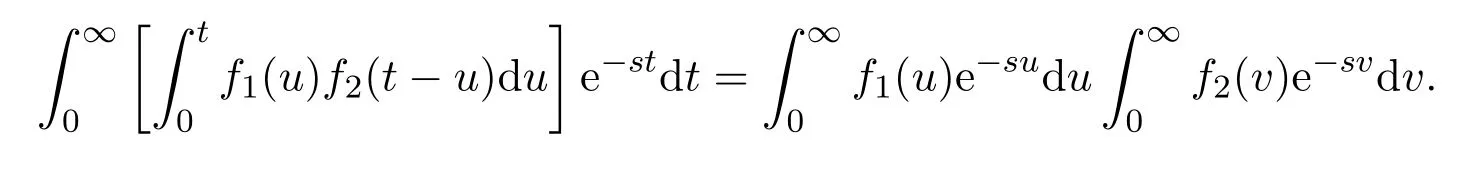
3　主要定理及其证明

4　小结

文章通过引入一个新的辅助函数（即定理证明中的ϕ（x））证明了两类Gamma商函数的严格对数完全单调性，这推广了文献［3］中的结果，并且此种证明方法更加简单直接.

［1］Merkle M.On log-convexity of a ratio of gamma functions［J］.Univ.Beograd.Publ.Elektrotehn.Fak.Ser.Mat.，1997，8：114-119.

［2］Chen Chaoping.Complete monotonicity properties for a ratio of gamma functions［J］.Univ.Beograd.Publ.Elektrotehn.Fak.Ser.Mat.，2005，16：26-28.

［3］Li Aijun，Chen Chaoping.Logarithmically complete monotonicity and Shur-convexity for some ratios of gamma functions［J］.Univ.Beograd.Publ.Elektrotehn.Fak.Ser.Mat.，2006，17：88-92.

［4］Qi Feng，Guo Senlin.Complete monotonicities of functions involving the gamma and digamma functions［J］.Applied Mathematics and Computation，2004，7（1）：63-72.

［5］Qi Feng，Chen Chaoping.A complete monotonicity property of the gamma function［J］.Journal of Mathematical Analysis and Applications，2004，296（2）：603-607.

［6］Chen Chaoping，Qi Feng.Logarithmically completely monotonic functions relating to the gamma function［J］.Journal of Mathematical Analysis and Applications，2006，321（1）：405-411.

［7］Guo Senlin，Qi Feng，Srivastava H M.A class of logarithmically completely monotonic functions related to the gamma function with applications［J］.Integral Transforms and Special Functions，2012，23（8）：557-566.

［8］Necdet Batir.On some properties of digamma and polygamma functions［J］.Journal of Mathematical Analysis and Applications，2007，328（1）：452-465.

［9］Qi Feng，Christian Berg.Complete monotonicity of a difference between the exponential and trigamma functions and properties related to a modified bessel functions［J］.Mediterranean Journal of Mathematics，2013，10（4）：1685-1696.

［10］王竹溪，郭敦仁.特殊函数概论［M］.北京：北京大学出版社，2000.

Logarithmically complete monotonicity of two classes of functions for the ratio of Gamma function

Liu Yuan，Wang Liantang

（College of Mathematics，Northwest University，Xi′an710127，China）

In this article，logarithmically complete monotonicity for the ratio of gamma functions is presented.By introducing a new function，we prove that the ratio of gamma function is strictly logarithmically completely monotonic.We also prove that a generalized form of the function is strictly logarithmically completely monotonic.

Gamma function，Psi（digamma）function，Polygamma function，logarithmically complete monotonicity

O174.6

A

1008-5513（2015）03-0291-05

10.3969/j.issn.1008-5513.2015.03.010

2014-12-03.

陕西省自然科学基金（2010JM1017）.

刘媛（1990-），硕士生，研究方向：特殊函数论.

2010 MSC：26A48