关于Bernstein-Durrmeyer-Bézier算子在Orlicz空间内的逼近

邓雪莉，吴嘎日迪

（内蒙古师范大学数学科学学院，呼和浩特 内蒙古　010022）

关于Bernstein-Durrmeyer-Bézier算子在Orlicz空间内的逼近

邓雪莉，吴嘎日迪

（内蒙古师范大学数学科学学院，呼和浩特 内蒙古010022）

在连续函数空间和Lp空间内研究算子逼近方法的基础上，利用一阶Ditzian-Totik积分模与不等式技巧研究了Bernstein-Durrmeyer-Bézier算子在Orlicz空间内的逼近性质.得到了Bernstein-Durrmeyer-Bézier算子在Orlicz空间内的逼近正定理和逼近等价定理.由于Orlicz空间比连续函数空间和Lp空间都“大”，其拓扑结构也比Lp空间复杂得多，所以本文的结果具有一定的拓展意义.

Bezier型算子；逼近正定理；等价定理；K-泛函；光滑模

1　引言

Bernstein-Durrmeyer-Bézier算子的定义为［1］：

其中

显然，Dn，α（f，x）是正线性算子并且Dn，α（1，x）=1.当α=1时，Dn，α（f，x）就是Durrmeyer算子

本文将利用Ditzian-Totik模研究算子Dn，α（f，x）在Orlicz空间内的逼近正定理及逼近等价定理.

M（u）和N（v）表示互余的N函数，关于N函数的定义及其性质见文献［2］.由N函数M（u）生成的Orlicz空间L∗M［0，1］是指具有有限Orlicz范数：

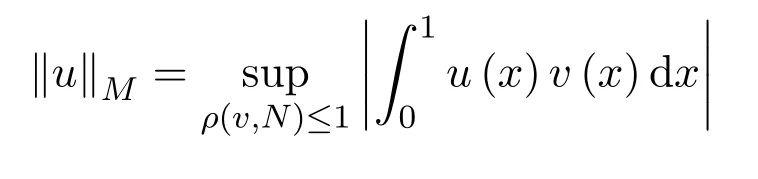
的可测函数全体｛u（x）｝，其中

是v（x）关于N（v）的模.

由文献［2］知，Orlicz范数其等价形式为：

在下文中用L∗M［0，1］表示带有Orlicz范数的Orlicz空间.对于f∈L∗M［0，1］，定义其光滑模和K-泛函如下：

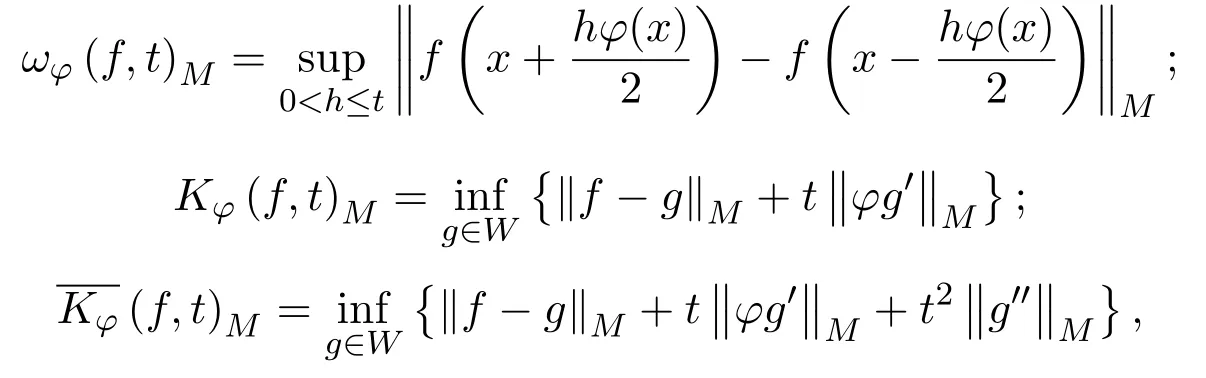
其中

由文献［3-4］知

这里a～b的含义是存在常数C＞0，使得C-1a≤b≤Ca.

用C表示与n，x无关的正常数，但在不同处可以表示不同的数值.

2　正定理

首先列出一些将要用到的性质，这些性质都可以通过简单计算得到.

为了证明正定理，首先证明几个引理.

引理 2.1对于f∈L∗M［0，1］，有

证明由（2.5）式和文献［5］中的引理1.1.1的结论，有

引理得证.

与文献［6］中相应结果的证明完全相仿，有

引理2.2对Pm∈Πm（定义在［0，1］上次数不超过m的代数多项式全体），k∈N，有

下面给出逼近正定理

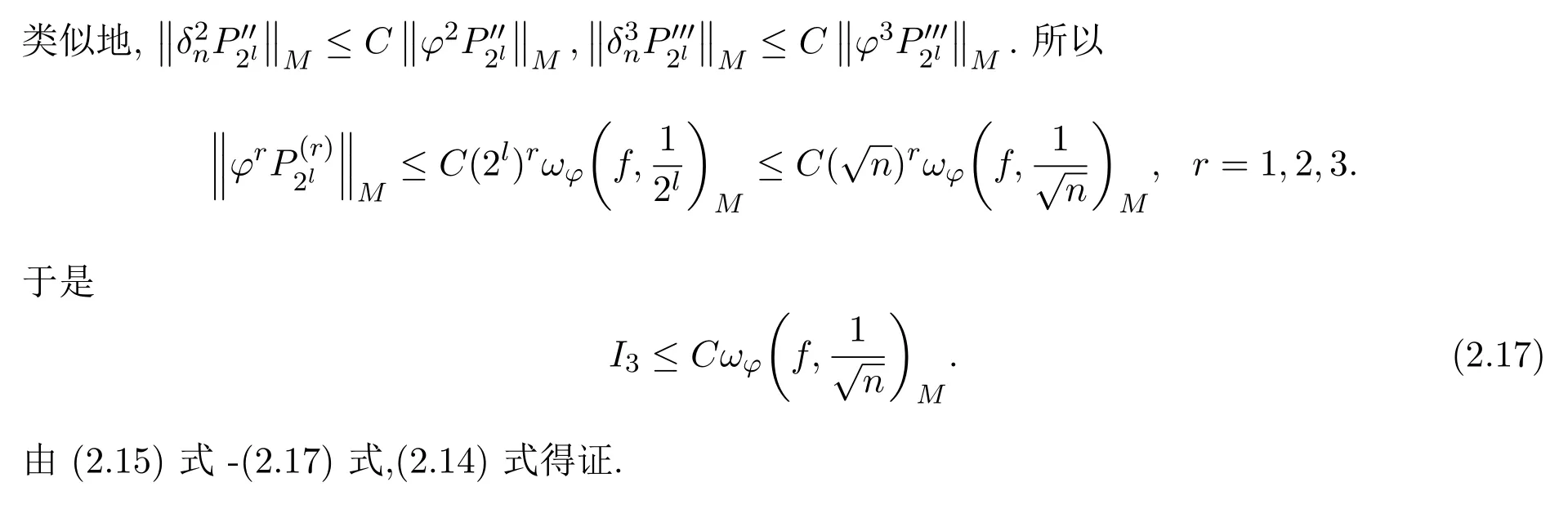
3　等价定理

为了证明等价定理，首先给出两个引理.

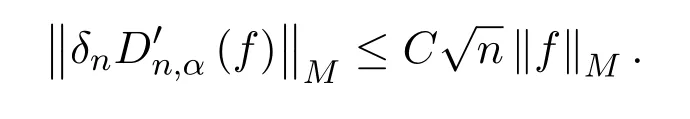
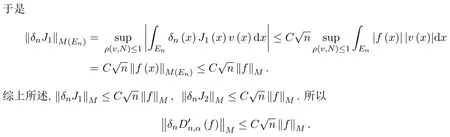
定理3.1 设

［1］Chang G.Generalized Bernstein-Bézier polynomial［J］.J.Comput.Math.，1983，1（4）：322-327.

［2］王廷辅.奥尔里奇空间及其应用［M］.哈尔滨：黑龙江科学技术出版社，1983.

［3］Wu Garidi.On Approximation by polynomials in Orlicz spaces［J］.A.T.A.，1991，7（3）：97-110.

［4］Ditzian Z，Totik V.Mouli of Smoothness［M］.NewYork：Spring-Verlag，1987.

［5］顾春贺.Orlicz空间中几个逼近问题的研究［D］.内蒙古师范大学：图书馆，2009.

［6］郭顺生，刘国芳，宋占杰.关于Bernstein-Durrmeyer-Bézier算子在Lp空间中的逼近［J］.数学物理学报：A辑，2010，30（6）：1424-1434.

［7］Zeng X，Chen W.On the rate of convergence of the generalized Durrmeyer type operators for function of bounded variation［J］.J.Approx.Theory，2000，102：1-12.

［8］陈广荣，吴嘎日迪.Orlicz空间中的联合最佳逼近［J］.纯粹数学与应用数学，1992，8（1）：102-104.

On approximation of Bernstein-Durrmeyer-Bézier operators in Orlicz spaces

Deng Xueli，Wu Garidi

（college of mathematics Science，Inner Mongolia Normal University，Hohhot010022，China）

In this paper we investigate the approximation problem of Bernstein-Durrmeyer-Bézier operators in Orlicz spaces based on the methods of studying the operator approximation in continuous function space and Lpspace，and used the tools of Ditzian-Totik integral modulus，with the help of the inequality techniques，and obtain the results of direct theorem and equivalence theorem on approximation of Bernstein-Durrmeyer-Bézier operators in Orlicz spaces.Because the Orlicz space is“big”than continuous function space and Lpspace，and its topological structure is more complicated than Lpspace，the results of this paper have certain expansion significance.

Bézier-type operator，direct theorem，equivalence theorem，K-functional，modulus of smoothness，elementary method，conjecture

O174.41

A

1008-5513（2015）03-0307-11

10.3969/j.issn.1008-5513.2015.03.012

2012-11-13.

国家自然科学基金（11161033）；内蒙古师范大学人才工程基金（RCPY-2-2012-K-036）.

邓雪莉（1986-），硕士生，研究方向：函数逼近论.

吴嘎日迪（1962-），硕士，教授，研究方向：函数逼近论.

2010 MSC：26A15