拟周期平面振子平衡点的稳定性

邢秀梅,任秀芳

(1.伊犁师范学院 数学与统计学院,新疆 伊宁 835000;2.南京农业大学 理学院数学系,南京 210095)



拟周期平面振子平衡点的稳定性

邢秀梅1,任秀芳2

(1.伊犁师范学院 数学与统计学院,新疆 伊宁 835000;2.南京农业大学 理学院数学系,南京 210095)

利用主积分方法,将周期系统平衡点的稳定性判据推广到拟周期情形,即证明拟周期二阶微分方程x″+h(t)x′+a(t)x2n+1+e(t,x)=0(n≥1)平衡点x=x′=0的稳定性,其中h(t),a(t),e(t,x)是拟周期系数,其频率向量满足Diophantine条件,且在x=x′=0附近,|e(t,x)|=O(x2n+2).结果表明,具有变号阻尼项拟周期振子的平衡点在一定条件下具有稳定性.

拟周期;Diophantine条件;平衡点稳定性

0 引 言

近年来,对拟周期微分方程的研究受到人们广泛关注.关于周期微分方程平衡点稳定性的研究已有许多结果[1-9].储继峰等[1]考虑具有一个半自由度的阻尼震荡系统：

(1)

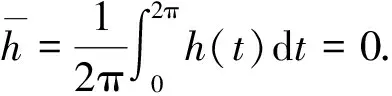
(2)

本文将刘期怀等[2]的相关结果推广到拟周期微分方程：即在方程(2)中,要求e(t,x)在x=0附近满足|e(t,x)|=O(x2n+2),h(t),a(t),e(t,x)关于t,x是实解析的,并且关于t是拟周期函数,相应的频率向量(ω1,ω2,…,ωm)满足Diophantine条件：即存在常数γ>0和τ>m-1,使得对一切k=(k1,k2,…,km)≠0,都有

(3)

其中|k|=|k1|+|k2|+…+|km|.

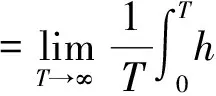
1)方程(2)的平衡点x=x′=0是稳定的;

1 典则变换

(4)

相应的Hamiltonian函数为

(5)

(6)

(7)

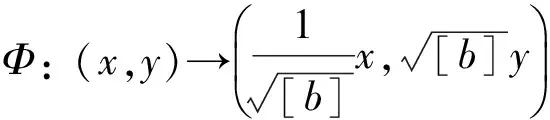
(8)

考虑辅助系统

(9)

令c=|[c]|[b]n+1.记(C(t),S(t))是方程(9)的满足初始条件(C(0),S(0))=(1,0)的周期解.令T>0为其最小正周期,则这些函数满足下列条件:

(10)

2 不稳定性的证明

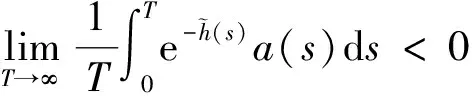
1)首先,引进典则变换:

则Hamiltonian函数(8)变为

(11)

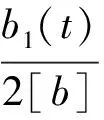
其次,定义一个与时间相关的典则变换:

其中

(12)

它关于t是拟周期的.则变换后的Hamiltonian函数(11)具有如下形式:

其中

(13)

令

(14)

利用式(12)和2β>1,得

(15)

2)不稳定性的证明.考虑关于变量λ,φ的动力系统

(16)

首先,证明存在一个φ*和0<υ<1,使得ψ(φ*)=0,并且当|φ-φ*|≤υ时,下述结论成立:

(17)

事实上,由式(10)有

并且

记m=min{|ψ(φ*+υ)|,|ψ(φ*-υ)|}.对于系统(16),存在常数r0>0,使得当|λ|≤r0时,下述不等式成立：

(19)

其次,定义角形区域Sε={(λ,φ)||λ|≤ε,|φ-φ*|≤υ},则必存在一点(λ0,φ0)∈Sε和某一时刻t*<0,使得λ(t*,λ0,ψ0)≥r0.

事实上,否则方程(16)的负向解属于集合

(20)

3 稳定性的证明

1)由于[c]>0,所以典则变换Φ1将Hamiltonian函数(8)变为

(21)

其中：

显然f1(t,θ)关于t的均值为零、关于θ是1周期的.

2)利用典则变换Φ2,使变换后的Hamiltonian函数(21)具有如下形式:

其中

(22)

(23)

(24)

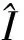
(25)

可得

(26)

对于固定的t,解λ,φ在每一时刻t关于φ0连续,相应的积分曲线形成了t轴的管状领域.由解的存在唯一性知,该管状内出发的解永远位于管状领域内.由于该管状领域大小由ε控制,而且ε可任意小,因此得到系统(23)的不动点λ=0是稳定的.

参考文献

[1] CHU Jifeng,DING Jinhong,JIANG Yongxin.Lyapunov Stability of Elliptic Periodic Solutions of Nonlinear Damped Equations [J].J Math Anal Appl,2012,396(1):294-301.

[2] LIU Qihuai,QIAN Dingbian,WANG Zhiguo.The Stability of the Equilibrium of the Damped Oscillator with Damping Changing Sign [J].Nonlinear Anal:Theory,Methods &Appl,2010,73(7):2071-2077.

[3] Chetayev N G.The Stability of Motion [M].New York:Pergamon Press,1961.

[4] Dieckerhoff R,Zehnder E.Boundedness of Solutions via the Twist-Theorem [J].Ann Scuola Norm Sup Pisa Cl Sci,1987,14(1):79-95.

[5] LIU Bin.The Stability of the Equilibrium of Planar Hamiltonian and Reversible Systems [J].J Dyn Differ Equ,2006,18(4):975-990.

[6] Bibikov Yu N.On the Stability of the Zero Solution of Essentially Nonlinear Hamiltonian System and Reversible Systems with One Degree of Freedom [J].Differ Equ,2002,38(5):579-584.

[7] LIU Bin.The Stability of Equilibrium of Quasi-periodic Planar Hamiltonian and Reversible Systems [J].Science in China Series A:Mathematics,2010,53(1):125-136.

[8] LIU Bingwen.Global Exponential Stability of Positive Periodic Solutions for a Delayed Nicholson’s Blowflies Model [J].J Math Anal Appl,2014,412(1):212-221.

[9] LI Lin,LIU Zhicheng.Existence of Periodic Solutions and Stability of Zero Solution of a Mathematical Model of Schistosomiasis [J/OL].J Appl Math,2014-02-13.http://dx.doi.org/10.1155/2014/765498.

[10] WU Yunchao,WANG Yiqian.The Stability of the Elliptic Equilibrium of Planar Quasi-periodic Hamiltonian Systems [J].Acta Math Sin:Engl Ser,2012,28(4):801-816.

[11] Pöschel J.A Lecture on the Classical KAM Theorem [J].Proc Sympos Pure Math,2001,69(2):707-732.

(责任编辑：赵立芹)

StabilityoftheEquilibriumofQuasi-periodicPlanarOscillator

XING Xiumei1,REN Xiufang2

(1.SchoolofMathematicsandStatistics,YiliNormalUniversity,Yining835000,XinjiangUygurAutonomousRegion,China;2.DepartmentofMathematics,CollegeofScience,NanjingAgriculturalUniversity,Nanjing210095,China)

We generalized the stability criteria for the equilibrium of the periodic system to those for that of quasi-periodic system,applying the method of main integration.Concretely,we showed the stability for the equilibriumx=x′=0 of the quasi-periodic second order differential equationx″+h(t)x′+a(t)x2n+1+e(t,x)=0,n≥1,whereh(t),a(t),e(t,x)are quasi-periodic coefficients,whose frequency vectors meet the requirements proposed by Diophantine.And moreover,|e(t,x)|=O(x2n+2)nearx=x′=0.The results we obtained also imply that,under some conditions,the equilibrium of the quasi-periodic oscillator with damping changing sign can still be stable.

quasi-periodic;Diophantine condition;stability of the equilibrium

10.13413/j.cnki.jdxblxb.2015.03.07

2014-10-27.< class="emphasis_bold">网络出版时间

时间：2015-02-11.

邢秀梅(1973—),女,汉族,博士,讲师,从事Hamiltonian系统的研究,E-mail:xingxm09@163.com.通信作者:任秀芳(1982—),女,汉族,博士,讲师,从事拟周期动力系统的研究,E-mail:xiufangren@gmail.com.

国家自然科学基金(批准号:21364016)、新疆维吾尔自治区自然科学基金(批准号:20122111328)和新疆维吾尔自治区重点学科项目(批准号:2012ZDXK13).

http://www.cnki.net/kcms/detail/22.1340.O.20150211.1126.001.html.

O175.13

：A

：1671-5489(2015)03-0383-06