具有抑制作用和离散时滞的捕食系统的Hopf分岔分析

王新慧，刘海鸿

(云南师范大学数学学院，云南昆明650092)

具有抑制作用和离散时滞的捕食系统的Hopf分岔分析

王新慧，刘海鸿

(云南师范大学数学学院，云南昆明650092)

研究了一类具有抑制作用和离散时滞的捕食－食饵模型，通过分析该模型在正平衡点的线性化方程及其相应的特征方程，研究了正平衡点渐近稳定性并证明了Hopf分岔的存在.通过应用规范型理论和中心流形定理，得到了确定Hopf分岔方向和分岔周期解的稳定性计算公式.最后，利用数值模拟验证了研究结果.

Lotka－Volterra捕食－食饵系统;离散时滞;稳定性;Hopf分岔;周期解;抑制作用

当F(s)，G(s)取不同形式时，很多学者已经做了广泛的研究［3－11］，例如，F(s)=G(s)=δ(s)时(δ为Dirac delta函数)，Chen［3］，Zhang［11］等研究指出系统(1)正平衡点的存在性暗示了它的全局稳定性.1973年May［12］首次提出并讨论了当系统(1)F(s)=δ(s－τ)，G(s)=δ(s)时的带有单个离散时滞的捕食－食饵模型:

Song和Wei［6］研究了系统(2)把τ作为分岔参数时τ对动力系统(2)的影响.F(s)=δ(s－τ)，(τ≥0)，G(s)=δ(s－η)(η≥0)时，(1)转化为如下形式:

Faria［4］，Ruan［5］，Yan和Chu［8］，He［13］，Lu和Wang［14］分别对(3)做了不同的研究.

其中，x(t)，y(t)分别表示食饵、捕食者种群的密度;k1＞0，k2＞0为食饵的增长率和捕食动物的死亡率，参数α1，α2，β12，β21，γ1均为正常数.本文研究了时滞τ对系统(4)的影响.通过研究发现时滞能导致捕食－食饵系统产生震荡.所以，由产生毒素而出现的捕食时滞会影响不同捕食模型的种群密度，从而影响季节繁殖、生长.因此对此问题的研究具有重要的意义.经过查阅大量文献还没发现从此角度考虑的研究.

1 正平衡点的稳定性和Hopf分岔的存在性

通过计算，当条件H1):k1α2＞k2α1成立时，式(4)存在唯一正平衡点E(x*，y*)，其中，

模袋混凝土的充灌是整个施工过程中的关键工序，要做到混凝土搅拌、运送、泵送、充灌成形一条龙作业，把握好充灌速度。所需混凝土必须严格按配合比搅拌，保证坍落度、强度等指标。充灌混凝土的顺序采取自下而上逐排口逐仓充灌 (每4 m为1个灌口)，每排的充灌顺序为：由模袋搭接的一侧开始向另一侧逐口充灌，采用这样的充灌顺序实际上是几条模袋轮流交替充灌。与同一次连续充满一条模袋后再充灌下一条模袋的顺序相比，这样的顺序有以下优点：

其中，M=－α1x*－γ1x*y*，N=－β12x*，Q=－γ1(x*)2，a11=－(α1+γ1y*)，a12=－β12，，a13=－2γ1x*，a14=－γ1，D=α2y*，E=－β21y*，b11=－β21，b12=α2.显然，通过变量变换，(4)的正平衡点E(x*，y*)转化为(5)的平衡点E*(0，0).易计算系统(5)在平衡点E*(0，0)性化系统的特征方程为:

其中，a1=－(M+E)，a0=ME－DN，b0=－DQ.

1)当τ=0时，(6)转化为λ2+a1λ+a0+b0=0.通过计算，易得a1＞0，a1(a0+b0)＞0，由Routh－Hurwitz定理可知式(6)的2个根均具有负实部，因此当τ=0时，正平衡点是局部渐近稳定的.

2)当τ≠0时，设λ=iω(ω＞0)是式(6)的根，则－ω2+ia1ω+a0+b0(cos(ωτ)－i sin(ωτ))= 0.分离实部和虚部得－ω2+a0+b0cos(ωτ)=0，a1ω－b0sin(ωτ)=0，把上式两端平方相加得

定理1如果系统(4)中系数ki，αi(i=1，2)满足条件H1)，ai(i=0，1)，b0满足H2)，则当τ∈［0，τ0)时，式(4)的正平衡点是渐近稳定点;当τ＞τ0时，式(4)的正平衡点是不稳定点;当τ=τj(j=0，1，2，…)时，式(4)在正平衡点附近出现Hopf分岔.

2 Hopf分岔的性质

运用Hassard在文献［19］中介绍的方法，讨论式(4)正平衡点附近分岔周期解的稳定性及Hopf分岔方向.在系统(5)中，令x－k(t)=xk(τt)，为了书写简便，记x－k(t)为xk(t)，则系统(5)转化为如下模型

定义线性算子L(μ):→R2和非线性算子f(·，μ):C→R2

其中δ表示狄拉克函数对于φ∈C1(［－1，0］，R2)，定义

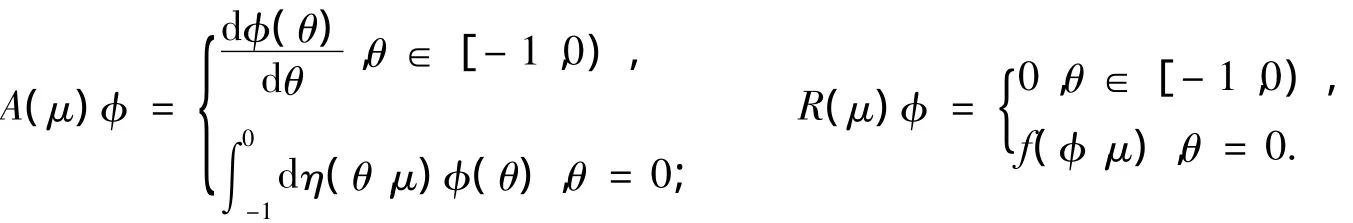
则(8)等价于x.t=A(μ)xt+R(μ)xt.对于ψ∈C1(［0，1］，(R2)*)定义

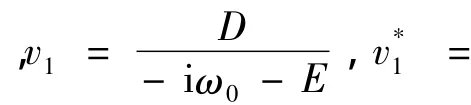
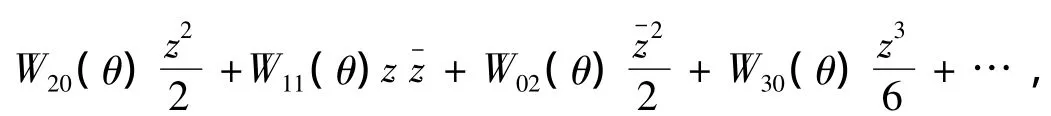
详细计算过程参看文献［19］.通过上面的讨论，可以得到下面结果.

定理1μ2决定了Hopf分岔的方向;如果μ2＞0(＜0)，则Hopf分岔是超临界(亚临界)的;β2决定了分岔的稳定性;如果β2＞0(＜0)，分岔周期解是不稳定(稳定)的;T2决定了分岔周期解的周期;如果T2＞0(＜0)，周期是增加(减少)的.

3 数值模拟及分析

利用数学计算软件Matlab和Xppant对前面的讨论结果进行数值模拟.考虑系统，

经计算E(1.046 8，0.2561 8)，ω0=0.263 88，τ0=9.455 78.由定理1可知:当τ＜τ0时，正平衡点E(1.046 8，0.256 1)是局部渐近稳定点，数值仿真如图1所示;当τ比τ0稍大时，系统(4)在正平衡点E附近产生Hopf分岔周期解，对应的数值仿真如图1所示.

由图1(a)可见当τ=9.2＜τ0，α=1时，系统(13)的数值仿真图正平衡点E(1.046 8，0.256 1)是渐近稳定的.由图1(b)可见当τ=9.8＞τ0，α=1时，系统(13)的数值仿真图正平衡点E(1.046 8，0.256 1)不稳定并且一个稳定的周期解从E(1.046 8，0.256 1)处分岔出来.

4 结语

论文讨论了式(4)正平衡点的稳定性以及Hopf分岔的存在性，并应用中心流形定理和规范型理论讨论正平衡点分岔的方向以及分岔周期解的稳定性，最后，通过数值模拟对相应理论结果加以验证.

［1］VOLTERRA V.Lecons sur la th'eorie math'ematique de la lutte pour la vie［M］.Paris:Gauthier－viuars，1931.

［2］BRELOT M.Sur le probl`eme biologique h'er'editaire de deux esp`eces d'evorante et d'evor'e［J］.Ann Mate Pura Appl，1931，9:58－74.

［3］CHEN L.Mathematical models and methods in ecology［M］.Beijing:Science Press，1988.

［4］FARIA T.Stability and bifurcation for a delayed predator－prey model and the effect of diffusion［J］.J Math Anal Appl，2001，254(2):433－463.

［5］RUAN S.Absolute stability，conditional stability and bifurcation in Kolmogorov－type predator－prey systemwith discrete delays［J］.Quart Appl Math，2001，59(1):159－172.

［6］SONG Y，WEI J.Local Hopf bifurcation and global periodic solutions in a delayed predator－prey system［J］.J Math Anal Appl，2005，301(1):1－21.

［7］SONG Y，YUAN S.Bifurcation analysis in a predator－prey system with time delay［J］.Nonlinear Anal:Real World Appl，2006，7(2):265－284.

［8］YAN X P，CHU Y D.Stability and bifurcation analysis for a delayed Lotka－Volterra predator－prey system［J］.Comput Appl Math，2006，196(1):198－210.

［9］YAN X P，LI W T.Hopf bifurcation and global periodic solutions in a delayed predator－prey system［J］.Appl Math Comput，2006，177(1):427－445.

［10］YAN X P，ZHANG C H.Hopf bifurcation in a delayed Lokta－Volterra predator－prey system［J］.Nonlinear Anal，RWA 2008，9(1):114－127.

［11］ZHANG J，FENG B.Geometric theory and bifurcation problems of ordinary differential equations［M］.Beijing:Beijing University Press，2000.

［12］MAY R M.Time delay versus stability in population models with two and three trophic levels［J］.Ecology，1973，54(2):315–325.

［13］HE X Z.Stability and delays in a predator－prey system［J］.J Math Anal Appl，1996，198(2):355－370.

［14］LU Z，WANG W.Global stability for two－species Lotka－Volterra systems with delay［J］.J Math Anal Appl，1997，208(1): 277－280.

［15］RICE E L.Allelopathy［M］.New York:Academic Press，1984.

［16］MAYNARD SMITH J.Models in ecology［M］.Cambridge:Cambridge University，1974.

［17］CHATTOPADHYAY J.Effects of toxic substance on a two－species competitive system［J］.Ecol Modl，1996，84(1/2/3):287－289.

［18］MUKHOPADHYAY A，CHATTOPADHYAY J，TAPASWI P K.A delay differential equation model of plankton allelopathy［J］.Mathematical Biosciences，1998，149(2):167－189.

［19］HASSARD B D，KAZARINOFF N D，WAN Y H.Theory and applications of Hopf bifurcation［M］.Cambridge:Cambridge Univ Press，1981.

［20］徐雪娇，林怡平.一类具有时滞的Lotka－volterra系统的Hopf分支与混沌控制［J］.云南师范大学学报:自然科学版，2010，30(4):28－33.

［21］张俊丽，陈斯养.一类具有时滞的广义造血模型的Hopf分支［J］.云南师范大学学报:自然科学版，2010，30(6):34－38.

(责任编辑梁志茂)

Analysis of Hopf Bifurcation in a Predator－Prey System of Population Allelopathy with a Discrete Delay

WANG Xin-hui，LIU Hai-hong
(Department of Mathematics，Yunnan Normal University，Kunming 650092，China)

A Lotka－Volterra two－species predator－prey system of population allelopathy with a discrete delay is studied.By linearizing the system at the positive equilibrium and analyzing the associated characteristic equation，the asymptotic stability of the positive equilibrium is investigated and Hopf bifurcations are demonstrated.Furthermore，the direction of Hopf bifurcation and the stability of the bifurcating periodic solutions are determined by the normal form theory and the center manifold theorem for functional differential equations.Finally，some numerical simulations are carried out for illustrating the theoretical results.

Lotka－Volterra predator－prey system;discrete delay;stability;Hopf bifurcation;periodic solution; allelopathy

O 175.13;O 193

A

1672－8513(2012)04－0286－06

10.3969/j.issn.1672－8513.2012.04.014

2011－12－19.

云南省自然科学基金(2011FZ086).

王新慧(1984－)，女，硕士.主要研究方向:微分方程与动力系统.

刘海鸿(1977－)，男，博士，副教授.主要研究方向:微分方程与动力系统.