Optical pulling force on nanoparticle clusters with gain due to Fano-like resonance

Jiangnan Ma(马江南), Feng Lv(冯侣), Guofu Wang(王国富), Zhifang Lin(林志方),Hongxia Zheng(郑红霞),,†, and Huajin Chen(陈华金),,4,‡

1School of Automation,Guangxi University of Science and Technology,Liuzhou 545006,China

2School of Electronic Engineering,Guangxi University of Science and Technology,Liuzhou 545006,China

3State Key Laboratory of Surface Physics and Department of Physics,Fudan University,Shanghai 200433,China

4Guangxi Earthmoving Machinery Collaborative Innovation Center,Liuzhou 545006,China

Keywords: optical pulling force,nanoparticle clusters,Fano-like resonance

1. Introduction

Optical manipulation based on the optical force has been a subject of intensive research after the pioneering works in optical trapping (well-known as optical tweezers nowadays)by Ashkin and collaborators.[1,2]Optical tweezers together with other optical manipulation techniques have become indispensable tools in various research fields such as biology,[3]chemistry[4,5]as well as physics.[6,7]Optical tweezers make use of the optical gradient force to stably trap particles,generally confining particles around the maximum of light intensity.[1,8,9]The optical scattering force,counterpart of the gradient force,however,usually propels[2,10–12]or pulls[13–16]objects, achieving particle transportation, even along curved trajectories.[12]The optical pulling force,[17,18]a member of the optical scattering force family, has attracted increasing attention in the last decade since it offers an opportunity to present a counterintuitive phenomenon: pull particles against light propagation.In contrast to the traditional ability of an optical force to trap or propel particles,optical pulling force can pull particles toward the source and accelerate particles over a long distance without an equilibrium position defined. In the past decade, some theoretical and experimental scenarios have been proposed to achieve optical pulling of complex particles or structures by various structured light beams besides conventional homogeneous polystyrene sphere,such as particles with chiral, gain, hyperbolic materials via nondiffractive Bessel beam,Airy beams,solenoid beam,interference of multiple beams and, even, a single plane wave.[13–18]However,these studies are mostly limited to a single particle or dimer,optical pulling of cluster has yet been observed,although cluster is extremely critical in many subjects including condensed matter physics, chemistry, biology and so on, in which the cluster can be used as a model to study properties of extended systems such as crystals and proteins.[19]

In this paper, we focus on exhibiting the optical pulling forces exerted on the nanoparticle clusters with gain. Clusters discussed here are composed of three,four,or five dipolar nanoparticles which make up three different configurations. It is found that, under the illumination of a simple single plane wave,optical pulling force can be achieved for all three clusters via exciting the Fano-like resonance. The Fano-like resonance describing the asymmetric line shape is an extended notion of Fano resonance, which originates from the original work of Fano, characterizing the coupling of a discrete atomic state with a continuum.[20]Recently,the Fano-like resonance has found its application in inducing optical force,but these researches limit in single nanostructure[21–23]or plasmonic dimer.[24–26]However, its effect on tailoring the optical force on clusters,especially optical pulling of clusters,has yet to be explored. Here, based on the full-wave calculation which combines the generalized Lorenz–Mie theory,[27]multiple scattering theory[28]as well as the Maxwell stress tensor approach,[29]we put forward the optical pulling of clusters at its Fano-like resonance. Our sufficient numerical results indicate that the optical pulling forces can be exactly attributed to the interaction between electric and magnetic dipoles excited in the nanoparticle clusters, which suppresses the extinction force giving rise to optical pushing force and,finally,pull clusters against light propagation direction. In addition, besides the adjustable force amplitude through the material gain, the resonant frequencies for clusters with different particle sizes present obvious shift,rendering its possible application in optical sorting of different clusters.

2. Results and discussion

Three different configurations illuminated by a single linearly polarized plane wave are schematically demonstrated in Fig.1,which are considered as examples to present the Fanolike resonance-induced optical pulling forces in nanoparticle clusters. The cluster composed of three spheres in Fig. 1(a)forms an isosceles triangle with a sphere adhered to another two spheres,whose Cartesian coordinates are(300,350,0)nm,(0,0,0)nm,and(0,700,0)nm,respectively. The rest two clusters presented in Figs. 1(b) and 1(c) are both symmetric regardingxaxis with spheres attached to each other. Each component particle in clusters is silicon sphere sharing the same radiusR=200 nm and permittivity. In general,the permittivity of material can be described asεr=+and its imaginary part＜0 denotes the material gain.[30,31]The gain is given by[32–34]=-(λ/4π)Nδe, whereλis the illuminating wavelength,δeis the emission cross section andNis the concentration ofErions.As a proof-of-concept demonstration of the optical pulling,we takeεr=12.6096-0.5i for different wavelengths in our theoretical calculations,whose gain can be kept constant by adjusting theδeandNin experiments. In this paper, we assume that the irradiance of the plane wave isI0=1.0 mW/μm2and the background is vacuum. Based on the generalized Lorenz–Mie theory,[27]multiple scattering theory,[28]and Maxwell stress tensor approach,[29]one can obtain the optical forces exerted on clusters composed of nanospheres with arbitrary configurations. Let us recapitulate the expression of optical force exerted on a cluster ofNparticles. In our cases, we focus on the longitudinal component of the optical forceFzalong the light propagation direction,which reads[28,35,36]

wherekandε0are the wave number and permittivity in background, respectively.E0is the amplitude of the electric field, which can be derived by the irradiance throughI0=|E0|2/(2Z0),withZ0being the wave impedance of background. The constant coefficientsc1andc2are given by

while thef1andf2dependent on the scatters and incident optical field can be formulated as

The superscript* denotes the complex conjugate.andare the scattering coefficients forj-th particle,whose relationship between the expansion coefficients of the total optical field imping on thej-th particle(and)presented later,can be bridged by the Mie scattering coefficientsandof thej-th particle[37]

with

where[x f(x)]′denotes the derivative with respect tox.μ(μj)is the relative permeability of background (particlej).xj=kjRjis the size parameter of particlejwithRjbeing the radius of particlej.mj=kj/kindicates the relative refractive index of thej-th particle.jnandare, respectively, the spherical Bessel function of the first and third kinds.andin Eqs. (3) and (4) are the expansion coefficients of the total optical field imping on thej-th particle in terms of vector spherical wave function.[37]The total optical field imping on thej-th particle consists of two distinct parts: firstly the initial incident wave striking the whole cluster,and secondly the sum of the scattered wave from all other particles except the considered particlej. The expansion coefficients can thus be given by[38]

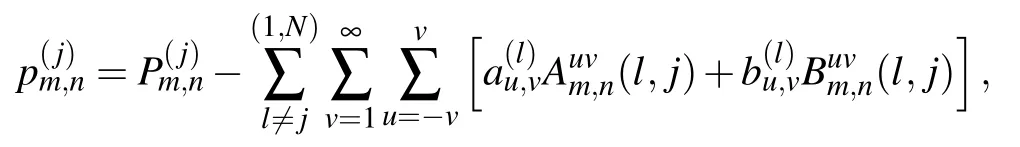
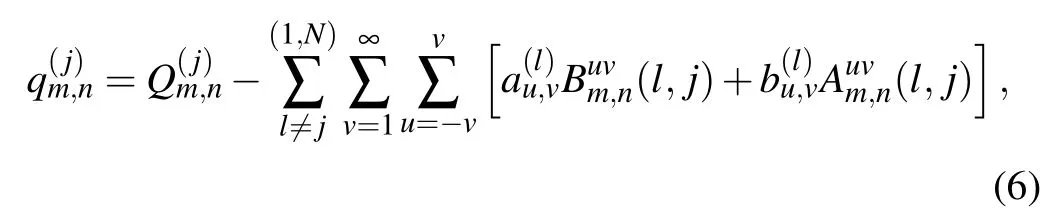
whereanddenote the expansion coefficients of the initial incident wave,[39]while the rest represent all the scattered fields from all other particles except particlej.(l,j)and(l,j) are the translation coefficients,[39]referring to the mathematical process that translate the expansion center of each particlelto that of the considered particlej.

Fig.1. Schematic illustration of clusters composed of three(a),four(b),and five (c) nanoparticles, respectively. The incident optical field is a linearlypolarized plane wave propagating along the positive z direction. Clusters are located in the xoy plane with radius R=200 nm for each constituent particle.

We next calculate theFzexerted on clusters demonstrated in Fig.1 based on the force formulations presented in Eqs.(1)–(6). The results are shown in Figs.2(a)–2(c)for different clusters corresponding to those in Figs. 1(a)–1(c), respectively.For all three cases, one can see that the Fano-like resonances come into play roughly aroundλ=1500 nm in the range denoted by the two dashed reference lines, which results in remarkably enhanced optical pulling forces withFz ＜0. The asymmetric Fano-like resonance line shapes are mimicked by the spectrum of total extinction efficienciesQext,as presented by the red lines in Figs.2(a)–2(c). For clusters illuminated by a single plane wave,theQextcan be evaluated according to[40]

with

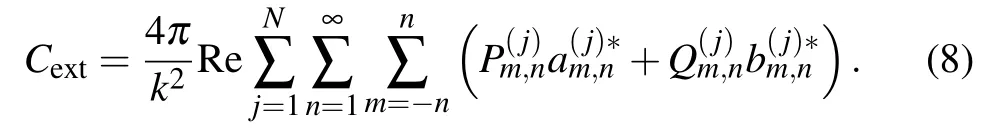
Also, we see from Figs. 1(a)–1(c) that the optical force on the cluster composed of four particles is the weakest by comparing with the ones of three and five particles. This is because the four-particle structure has a smallest cross sectionCpr,[41]to which the magnitude of the optical force in the direction of light propagation is proportional. In addition, one can roughly find that the structures of the three clusters generating the optical pulling force share the following same characteristics.First,these structures can exhibit a Fano-like in the illuminating wavelength. Second,all particles constituting the structures lie in the same plane that is perpendicular to the direction of light propagation and they do not have a crossover in the propagation direction. Third, the structures are symmetric with respect to the axis normal to the direction of both the light propagation and the electric field polarization. These properties can be treated as a reference to generate the optical pulling force on other clusters.

To trace the physical origin of the optical pulling force,we decompose the optical force into the extinction forceIFzand the recoil forceRFz,which can be expressed as[13]

with

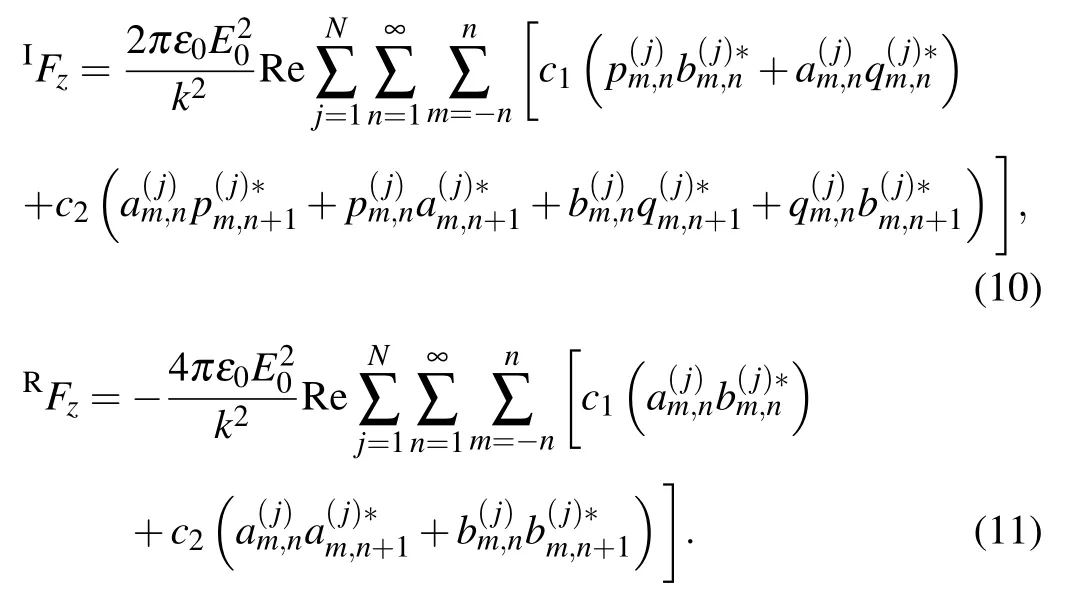
The extinction forceIFzis proportional to the polarizability,stemming from the coupling between the multipoles excited in particles and the incident field. It can be physically understood from the process that particles in the light path will intercept the light and then an extinction force will be exerted on the particles due to the optical momentum transfer. The recoil forceRFz, however, has quadratic dependence on the polarizability,arising from the coupling between various multipoles excited in particles with the physical picture that recoil will occur by light re-emitted by the oscillating multipoles.The expressions of extinction forceIFzand the recoil forceRFzcan thus be easily derived by, respectively, splitting the linear and quadratic terms of Mie coefficients in Eqs.(1)–(3).Figures 2(d)–2(f) present the decomposed resultsIFz(orange line) andRFz(pink line) for different clusters in Figs. 1(a)–1(c),whose optical forces have been shown in Figs.2(a)–2(c)and reproduced in Figs. 2(d)–2(f) by black lines for clarity.One can observe that, for all three clusters considered in this paper,the extinction forcesIFzare always along the propagation direction withIFz ＞0. At the Fano-like resonance, the recoil forcesRFzsurpass the extinction forcesIFz, leading to the optical pulling withFz ＜0. It is worth noting that the Fano-like resonance-induced optical pulling force is the result of the collective interaction of all particles in clusters,whereas it can not occur for an individual particle due toIFz ＞RFzin our case.

Fig.2. The z component of the optical forces and the extinction spectra for different clusters(a)–(c)corresponding to those in Figs.1(a)–1(c),respectively.(d)–(f)The z component of decomposed optical forces corresponding to panels(a)–(c).

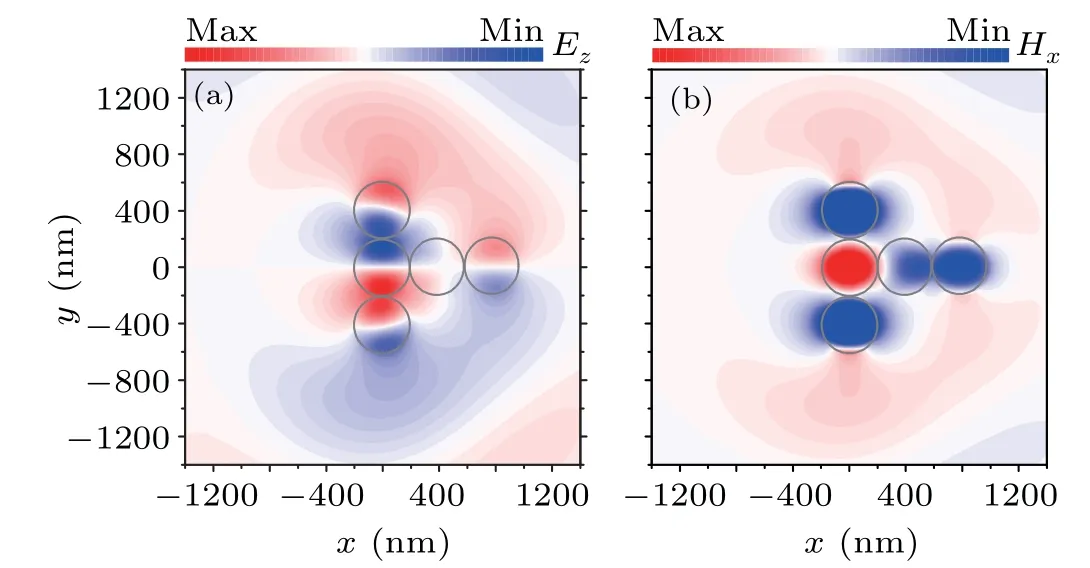
Fig.3. The near-field plots of the z component of the electric field and the x component of the magnetic field for the cluster composed of five particles at the wavelength λ =1525 nm. All the other parameters are the same as those in Fig.1(c).

A more deep understanding about the underlying physics of the optical pulling can be achieved through distinguishing the coupling between various multipoles, termed as different coupling channels here. After careful investigation, we find that the recoil forces can be thoroughly traced to the lowest coupling channel from the interaction between electric and magnetic dipoles, viz.Fpm, as indicated by the overlap between the cyan line and pink line in Figs. 2(d)–2(f). Contributions from all other coupling channels referring high-order multipoles,viz.Fotherpresented by the green line in Figs.2(d)–2(f), however, can be ignored. The formulations forFpmandFotherare expressed as

An intuitive demonstration of the excitation of the electric and magnetic dipoles can be visualized by the near-field patterns as shown in Fig. 3, where the cluster composed of five particles is just given as an example. One can roughly observe that thezcomponent of the electric field and thexcomponent of the magnetic field suggest the excitation of the electric and magnetic dipoles in the cluster due to the collective contribution from each particle.

The Fano-like resonance-induced optical pulling force can be agilely tailored owing to the flexible adjustability of the Fano-like resonance through particle properties. The particle size and material gain of each constituent particle for cluster composed of five particles shown in Fig.1(c)are considered as typical examples here. Figures 4(a)and 4(b)demonstrate the spectra of the optical forces as well as extinction efficiencies for various clusters with different particle sizes. It can be observed in Fig.4(a)that the optical pulling forces exhibit a red shift as the particle size increases,following shift tendency of the resonance of the extinction efficiencies shown in Fig.4(b).The crucial role of the Fano-like resonance in inducing optical pulling is thus suggested, in agreement with the results in Fig. 2. It should be also noticed that the maximum of the optical pulling forces always occurs aroundλ/R=7.6, one can therefore selectively pull clusters of different particle sizes with an appropriate operating wavelength. Furthermore, the optical forces and the corresponding extinction efficiencies for the cluster composed of five nanoparticles with different gains are also calculated in Fig.5. One can see that the emergence of the optical pulling forces for different gains lies always in the range around the Fano-like resonance. With increasing the gain from-0.4 to-0.55, the pulling force is significantly enhanced, while the optical pushing forces exhibit nearly no graphically discernible difference, as illustrated in Fig. 5(a).It is therefore concluded that the Fano-like resonance-induced optical pulling force can be flexibly tailored through both the particle size and gain, providing alternative degrees of freedom to manipulate particle clusters.

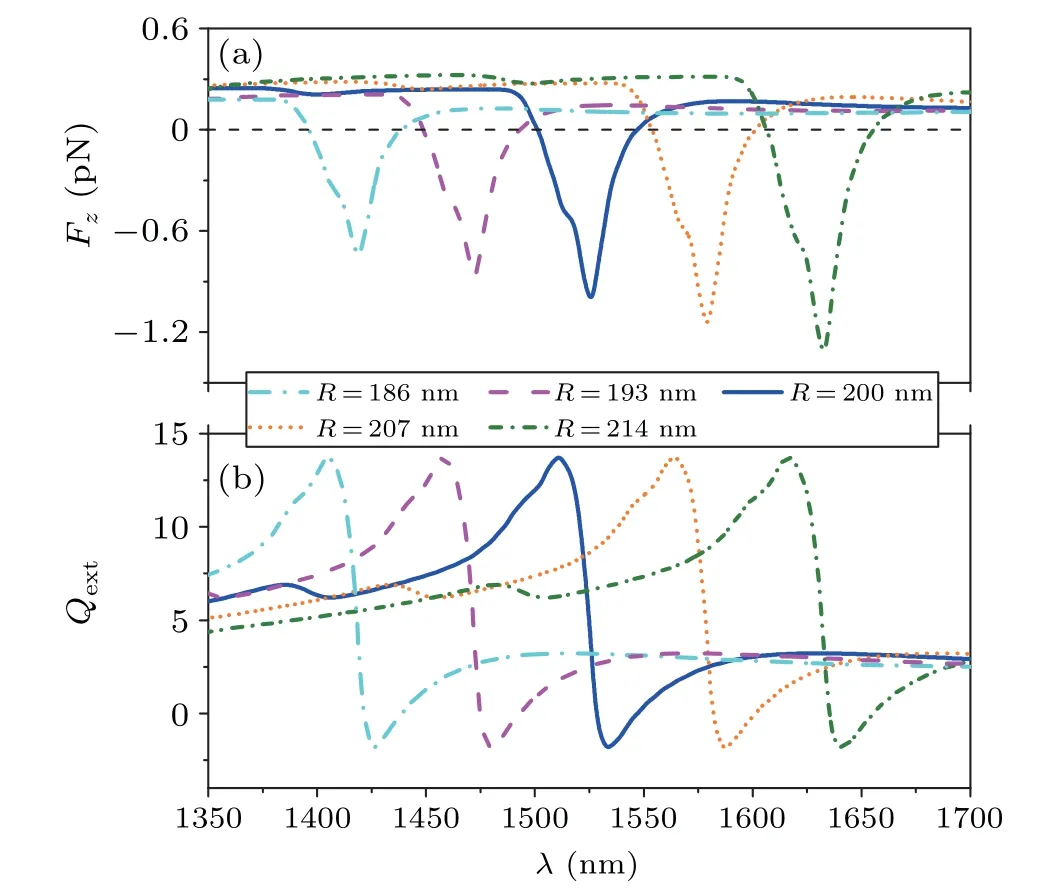
Fig.4. The z component of the optical forces(a)and the extinction spectra(b) for the cluster composed of five nanoparticles with different radii. All other parameters are the same as those in Fig.1(c).

Fig.5. The z component of the optical forces(a)and the extinction spectra(b) for the cluster composed of five nanoparticles with different gains. All other parameters are the same as those in Fig.1(c).

3. Conclusions

We have demonstrated that, using the full-wave calculation, the Fano-like resonance can induce the optical pulling forces on different nanoparticle clusters with gain immersed in a plane wave with linear polarization. It is revealed that the Fano-like resonance-induced optical pulling force comes entirely from the recoil force,which is mostly dominated by the interaction between electric and magnetic dipoles while other higher-order coupling channels have a negligible contribution.Besides,the Fano-like resonance-induced optical pulling force can be flexibly tailored by both the particle size and gain, it thus may find application in optical sorting and separation of particle clusters. As Fano or Fano-like resonances are widely occurred in various systems such as plasmonic structures and photonic devices,[42–44]the Fano-like resonance-induced optical pulling force is expected to be universal for other systems exhibiting Fano or Fano-like resonances.

Data availability statement

The data that support the findings of this study are openly available in Science Data Bank at https://www.doi.org/10.57760/sciencedb.j00113.00087.

Acknowledgments

Project supported by the Natural Science Foundation of Guangxi Province of China (Grant No. 2021GXNSFDA196001), the National Natural Science Foundation of China (Grant Nos. 12174076, 12074084, and 12204117), Guangxi Science and Technology Project (Grant Nos. AD22080042 and AB21220052), and Open Project of State Key Laboratory of Surface Physics in Fudan University(Grant No.KF202215).