Optimization and verification of wind tunnel free-flight similarity law for separation of cluster munition

Fei XUE, Jun TANG, Huqing WANG, Zenghui JIANG,Yuho WANG, Hn QIN, Peng BAI

a China Academy of Aerospace Aerodynamics, Beijing 100074, China

b Hubei Aerospace Flight Vehicle Institute, Wuhan 430048, China

c Beijing Institute of Control Engineering, Beijing 100036, China

KEYWORDS Cluster munition;Ejection separation;Free-flight wind tunnel test;Multi-body separation;Similarity-law derivation

Abstract In view of the separation form of the separator from the back of the carrier upward and from the side of the carrier outward,separation-safety research is carried out by taking the separation of a cluster munition as an example.In previous wind tunnel free-flight tests,the similarity law of vertical,downward,moving submunition was used to design submunitions at different positions in different initial-velocity directions,which resulted in large discrepancies between wind tunnel test results and real flight. In a wind tunnel test, each submunition has an independent time-reduction ratio with respect to the dispenser.Even if the separation trajectory of a single submunition is accurate,there will be errors in the position of each submunition at a given time.Therefore,it is necessary to determine the time-reduction ratio between submunitions, and to modify the test results later. In order to ensure the accuracy of wind tunnel test results, the similarity law of a freeflight test in a wind tunnel is derived in this paper. The time-correction scheme to ensure motion similarity between submunitions is solved. Numerical simulation is used to simulate the separation of a wind tunnel test and real aircraft, and the motion parameters of different submunitions are solved. The results show that the new similarity laws derived for different types of submunitions can greatly reduce the errors caused by previous similarity laws.In addition to the case for the separation of a cluster munition, the similarity law can also be applied to the free-flight test design of wind tunnels for vertical separation and horizontal separation of other kinds of aircraft.

1. Introduction

In the process of separation, most separators move downward from the belly of an aircraft. However, with the more extensive use of aircraft, the separation forms of stores have become diverse.There are forms of separation from the back of the carrier upwards,from the side of the carrier outwards,and from the rear of the carrier backwards. For example, Lockheed Martin’s D-21,using the M-12 as the carrier,adds a bracket on the back of the fuselage behind the master aircraft,carries the D-21 on the back and releases the D-21 when the flight speed reaches Ma=3.25. During the development of the D-21, many accidents occurred. Notable, in the fourth separation in 1966, a D-21 collided with the mother aircraft after separation, resulting in the disintegration of an M-12 aircraft and the drowning of the pilot in the sea. Compared with downward separation, the displacement caused by gravity during upward separation and lateral separation no longer increases distance.The displacement caused by gravity is more likely to cause collision between the store and the carrier, leading to separation failure. In addition to the D-21, the separation-cluster munition is also a common aircraft,with both upward and lateral separation.Avoiding collision is a focus of research.

The cluster munition has high lethality, and submunitions are ejected during the flight of a dispenser, which can damage a large area.1,2Much research has been carried out concerning different perspectives on the separation of a cluster munition.The cluster munition, with smaller submunitions, is mainly used for ground attack and defense interception at high altitude, such as kinetic kill vehicle.3-5With the aid of a highspeed flying kinetic missile, submunitions have great kinetic energy and can directly destroy a target. Large submunitions can have automatic guidance, and can independently carry out air-to-air or air-to-ground attack tasks after separation from the dispenser.6,7

Dietz et al.carried out an experiment on the influence of aerodynamic force on the separation of cluster munition with rudder surface under the condition of Ma=1.9,at sea level,and studied the possibility of collision between submunitions and dispenser, as well as the possibility of collision between submunitions during the separation process.8Wang and Tao of Nanjing University of Technology analyzed the multi-body flow characteristics and the aerodynamic interference characteristics of submunitions under sequential separation. Variation in the flow characteristics and the aerodynamic interference characteristics of submunitions with different separation styles was shown, and the interaction process of aerodynamic interference between submunitions in different separation stages was revealed.9,10Using wind tunnel testing and numerical simulation,Panneerselvam et al. researched the aerodynamic characteristics of submunitions and the separation process.11-13

In addition to the above research methods, wind tunnel free-flight testing is an unsteady method, which is different from other test methods. Its main feature is that the model has no support interference, making it the same as the case of real aircraft, so it can truly match aircraft motion.14,15Because of its unique advantages, the wind tunnel free-flight test method can not only be used to study the dynamic aerodynamic characteristics of a single aircraft,but also the aerodynamic interference of multiple objects in the separation process, for example, the interference of carrier and missile,the safety of external store separation, the separation of two-stage aircraft, and the separation of submunitions. In recent years, Xue carried out a number of studies on separation similarity laws,such as the store separation from aircraft.14,16,17Some progress has been made in solving the problem of large errors in similarity law free-flight testing in these kinds of wind tunnels. However, for the separation problem of submunitions and the dispenser, no relevant research has been conducted, and no one has carried out the derivation of a high-precision similarity law. The existing method still uses the general similarity law of previous free-flight tests, and test error remains large.

In previous wind tunnel free-flight testing of the separation of cluster munition, the parameters of vertical downwardmoving submunitions are designed by using the general similarity law14; these parameters are then used for all other submunitions. The test error of this method is very large.Firstly, the error in the former vertical downward separation similarity law is itself very large,and the latest vertical separation wind tunnel free-flight test similarity law has only been studied in the last year.Secondly,the former free-flight similarity law of submunitions in a wind tunnel is not designed according to the actual characteristics of separation submunitions. Previously, the similarity laws of submunitions did not consider the motion characteristics of the submunitions in a circular direction, which resulted in significant test error. For example, submunitions that move vertically upward and horizontally must be different from submunitions that move vertically downward.According to the existing similarity theory(Fr similarity),gravity is an important factor in the motion of submunitions,and the initial motion of each submunition and the influence of gravity on each submunition are different. Therefore, it is obviously incorrect to use the design method of vertical downward submunitions to design all submunition parameters, and previous wind tunnel free-flight test errors have, therefore, been large, resulting in large error in the final test.In consideration of the above,the previous similarity laws of free-flight testing of submunitions need to be derived again.

According to previous studies on the similarity law of store separation from aircraft,14,16,17if an independent derivation of the similarity law can be carried out for each submunition at different positions on the circumference,the error inherent to wind tunnel testing can be reduced, guaranteeing its accuracy. Time similarity is also an important similarity index in the derivation of the similarity law in free-flight testing of the previous wind tunnel test.In other separation studies,for example,with respect to store separation from aircraft, there are only two objects in the separation process, i.e., carrier and separator, so it is easier to ensure the time similarity between them. The separation of submunitions will become more complex because it is impossible to separate only one submunition. More than one submunition will lead to each submunition’s own time-reduction ratio with respect to the dispenser, and will also lead to the test results of separation characteristics different from the real aircraft. If the former similarity law is used to ignore this problem,the test error arises. Therefore, the test results need time-scaling correction to obtain the correct relative-position relationship between submunitions, so as to judge whether the separation of submunition is safe.It is very important to solve the time-scaling relationship between different submunitions and to determine the correction scheme for different submunitions test data, both of which are very difficult.

In this paper,the above two points are taken as the focus of the work.Not only should the similarity laws of free-flight testing in a wind tunnel for submunitions at different positions be derived,but also the time-correction scheme,to ensure motion similarity between submunitions.The model in this paper uses a high-altitude, high-speed, high-mass submunition, such as that of a long-range air-to-air missile.18-20In this kind of flight process, the separation of submunitions under the high-speed flight of the dispenser can very easily cause a collision between submunitions and the dispenser,as well as between the submunitions themselves.In this paper,for typical cross-shaped submunitions, the derivation of the similarity law for free-flight testing of submunitions separation wind tunnel is carried out. Through reasonable simplification, the similarity law and time-correction scheme of free-flight testing in a wind tunnel with strong practicability and high accuracy are solved.CFD technology is used to simulate separation of the real submunition,the separation characteristics of the wind tunnel test obtained by the previous similarity law,the separation characteristics of the wind tunnel test obtained by the previous ideal design method, and the separation characteristics of the wind tunnel test designed using the similarity law derived in this paper, to verify the accuracy of the new similarity law derived in this paper.

2. Derivation of similarity law of submunition motion

In the separation of cluster munition,there are three representative types of submunition: vertical downward moving submunitions: No. 1; horizontal lateral moving submunitions:No. 2 and No. 4; and vertical upward moving submunitions:No. 3; as shown in Fig. 1.

This paper is arranged as follows:firstly,the wind tunnel test conditions for these three kinds of submunitions are deduced to improve the test accuracy of free flight in the wind tunnel. Secondly, to ensure the test accuracy of each submunition (relative to the movement of the dispenser), the time-correction scheme to ensure the movement similarity between submunitions are solved, so as to ensure the movement between submunitions is accurate.This section focuses on the first work,and the third section focuses on the second work.

2.1. Submunitions moving vertically downward

shrinkage ratio, kl, a preliminary model is designed using three-dimensional-model-design software. According to Eq.(1), the mass, mm, and inertia, Im, of the designed wind tunnel test model are iterated, and the mass and inertia values of the model are determined. In previous research, the design of experimental-model-separation speed was also simple. The method adopted ensures that the kinetic energy of the separation in the wind tunnel test is proportional to the work done by the reference length of the wind tunnel inflow.

Let kmbe the mass ratio of the wind tunnel submunition to the real submunition,kv0the separation-velocity ratio of the wind tunnel submunition to the real submunition, and kq∞the free-stream dynamic pressure ratio of the wind tunnel to a real flight. Careful study reveals that Eq. (2) lacks a real physical basis, so the accuracy of the separation speed needs to be verified.The traditional light-model method and the traditional heavy-model method cannot be realized, meaning there is a lack of solutions, so we can only use the methods of Eqs. (1) and (2) for approximation testing. Previous experiments are not so much similar as approximate,so it is conceivable that the test errors of previous methods are large.

An improved derivation of the similarity law is in Ref. 17 and will not be covered in detail here. In this paper, the similarity laws of horizontal and vertical upward moving submunitions are derived. The following are the results of the law of similitude for vertically downward moving submunitions:

The vertical downward moving submunition is a conventional separation method.The previous test similarity law is very simple. Firstly, using a wind tunnel to determine the model-

Subscript 1 indicates the relevant parameters of submunition 1. Let km1be the mass ratio of the wind tunnel submunition 1 to the real submunition 1,klthe length ratio of the wind tunnel submunition to the real submunition, kρthe fluiddensity ratio of the wind tunnel submunition to the real submunition, kv01the separation-velocity ratio of the wind tunnel submunition 1 to the real submunition 1. n1is the displacement-correction parameter of submunition 1.

2.2. Submunitions moving horizontally and laterally

In the literature, there is no special similarity-law derivation for horizontal lateral motion submunitions, which will be carried out in this paper. The biggest difference between vertical movement and downward movement is that the vertical movement of the submunition completely depends on the acceleration due to gravity to produce displacement. At the same time, there is obvious horizontal motion in the lateral motion submunition. These findings are significantly different from those of previous wind tunnel free-flight tests. In order to match motion similarity between a wind tunnel test and realaircraft horizontal submunition, the similarity law should be derived again based on this feature.

Based on previous findings, the similarity law of vertical downward submunitions ignores lateral motion with small displacement. In lateral separation, there is an initial velocity which cannot be ignored during lateral movement of the submunition,so lateral displacement cannot be ignored and needs to be considered.At the same time,in supersonic flow,aerodynamic pressure is large, and aerodynamic resistance is often larger than the submunition’s own gravity. Therefore, the displacement along the air flow direction cannot be ignored. On the contrary, in the vertical direction, because the vertical displacement is the second power of time, and the time is very short,usually less than 1 s,the vertical displacement of the lateral moving submunition is small. In order to simplify the derivation process, the vertical displacement of the lateral moving submunition can be ignored. The real-flight displacement is as follows:

Subscript 2 indicates the relevant parameters of submunition 2.Let xsbe the horizontal displacement of the real submunition,zsthe lateral displacement of the real submunition,q∞sthe free-stream dynamic pressure of the real submunition, Ssthe reference area of the real submunition, lsthe reference length of the real submunition,msthe mass of the real submunition, v0sthe separation velocity of the real submunition, tsthe motion time of the real store, and g gravitational acceleration.

The displacement of a wind tunnel test is as follows:

Let xmbe the horizontal displacement of the wind tunnel submunition, zmthe lateral displacement of the wind tunnel submunition, q∞mthe free-stream dynamic pressure of the wind tunnel,Smthe reference area of the wind tunnel submunition, lmthe reference length of the wind tunnel submunition,mmthe mass of the wind tunnel submunition,v0mthe separation velocity of the wind tunnel submunition, and tmthe motion time of the wind tunnel store.

According to the trajectory equation:

After simplification:

Let ktbe the motion-time ratio of the wind tunnel submunition to the real submunition.

Because the linear displacement must meet a similar sizereduction ratio:

Insert Eqs. (6) and (8) into (11) to obtain:

Inserting Eq. (12) into (10) gives:

For the horizontal lateral motion submunition to satisfy the motion similarity, the former must satisfy Eq. (13). There is a strange phenomenon.Ref. 14 studies the similarity law of vertical downward separation,while this part of this paper studies the similarity law of horizontal moving submunition. The objects of the two studies are different. However, Eq. (13) in this paper is the same as Eq. (5) in Ref. 14.

Eq.(5)in Ref.14 is the similarity-law equation of the previous wind tunnel test. Due to a lack of rigorous derivation of the former similarity law, the former wind tunnel test has a large error,which is an abandoned similarity law.As described in Ref. 14, Eq. (5) is derived from Eq. (4). By comparing Eq.(4) in Ref. 14 with Eq. (9) in this paper, we can see that they have different forms, and the solutions of the two equations are also different, but we get the same form of solution. To sum up, it should be a coincidence that the two are the same.After all,regarding the initial velocities of the two subjects,one is horizontal and one is vertical downward, and the starting points of the two studies are different. Therefore, we cannot deny the innovation of this section because the new similarity law (Eq. (13))derived in this paper is the same as the previous abandoned wind tunnel test similarity law of vertical downward separation, which was abandoned.

2.3. Submunitions moving vertically upward

In the past wind tunnel free-flight test, Fr,similarity is usually considered,and the main consideration is how to make up for the lack of gravitational acceleration during motion of the separated object with an initial downward ejection velocity.Previously,no matter how small the separation speed was,even if it was 0,the separated object could be separated from the projec-

tile under the action of gravity. The gravity of the separated matter moving upward is not negligible. If the initial upward velocity is not large enough, or the lift is not large enough, a collision can easily occur between the separated object and the dispenser,which is also a unique feature of this paper.Previous wind tunnel free-flight tests did not consider how to conduct the test if the initial ejection velocity of the separated object was upward. It is obviously wrong to use uniform downward moving submunition parameters for design. To solve this problem, this paper uses a method similar to the method of derivation of the similarity law of the vertically upward moving submunition and the method of trajectory similarity, specifically as follows:

Subscript 3 indicates the relevant parameters of submunition 3. Let ymbe the vertical displacement of the wind tunnel submunition, and ysthe vertical displacement of the real submunition.

The displacements of the free-flight-test model in the wind tunnel are as follows:

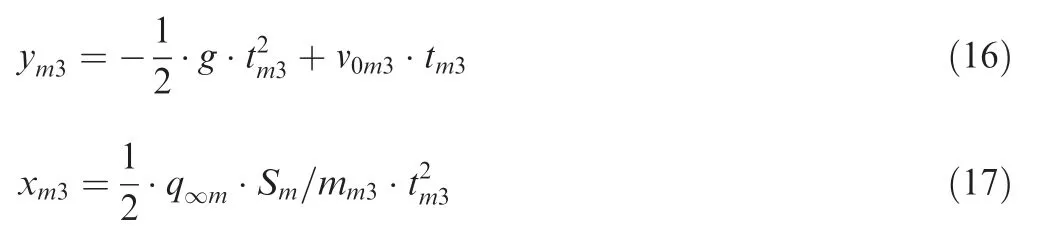
It can be seen from the equation that the displacement caused by the acceleration of gravity is negative,which is consistent with physical knowledge. According to the trajectory equation:

The free-flight-test time of the wind tunnel is short, so the quadratic term of time can be ignored:

In the real-flight scenario of Eq.(16),let ys3=l0;solve ts3at this time, and then let:

The numerical value of n3can then be solved. n3is the displacement-correction parameter of submunition 3. Eq.(18) is simplified as:

In Eq. (11) of Ref. 14, the time-scaling relationship is obtained:

Therefore, the relationship between the initial separation velocities of vertical upward submunitions can be obtained as follows:

Eq. (23) shows that the similarity law of vertical upward submunitions is similar to that of Eq. (4) (of vertical downward submunitions).It is not difficult to understand this point.In Ref. 17, a simplified equation of the similarity law of vertical downward separation is proposed, that is, Eq. (2) in this paper. In this section, the submunition moving vertically upward is studied. This specific detail is considered in Eqs.(12) and (16) established for this physical feature. Therefore,although Eq. (23) is similar to Eq. (4), there is a large difference between the numerical values of n3and n1. Therefore, in the design of free-flight testing in a wind tunnel, the model parameters of vertically downward moving submunition are designed according to Eq. (4). According to Eq. (13), the model parameters of lateral moving submunitions are designed; and according to Eq. (23), the model parameters of vertical upward moving submunitions are designed.

3. Derivation of time-correction scheme

The starting point of this paper is to ensure that the trajectory of each submunition relative to the dispenser is accurate and similar to the real cluster munition.This means that the movement of a single submunition is accurate, but also, proceeding from this starting point, that a proportional relationship or other forms of constraints between the time scales of submunitions may occur. Therefore, in order to ensure that the locations of submunitions are accurate, it is necessary to solve the time-scaling relationship between submunitions. In a later stage, the time of the test data is modified to obtain the realposition relationship between submunitions; time similarity is relative. Based on the time between the downward moving submunition and the dispenser, the time relationship between the vertical downward moving submunition and the vertical upward moving submunition, and the time relationship between the vertical downward moving submunition and the lateral moving submunition are solved respectively.

3.1.Time relationship between submunition 1 and submunition 3

Eq.(22)is the time scaling kt3of the vertical upward submunition.In Ref. 14,the time scaling kt1,of the vertical downward submunition is obtained:

Eq. (25) shows that as long as the mass m1of the vertical downward submunition is equal to the mass m3of the vertical upward submunition, the time of the two submunitions is always similar.

However, in order to ensure similar angular displacement,it is necessary to meet the following requirements:

Therefore,as long as the model is designed according to Eq.(26):

3.2.Time relationship between submunition 1 and submunition 2

Eq. (12) gives the time relationship between the side moving submunition and the dispenser.

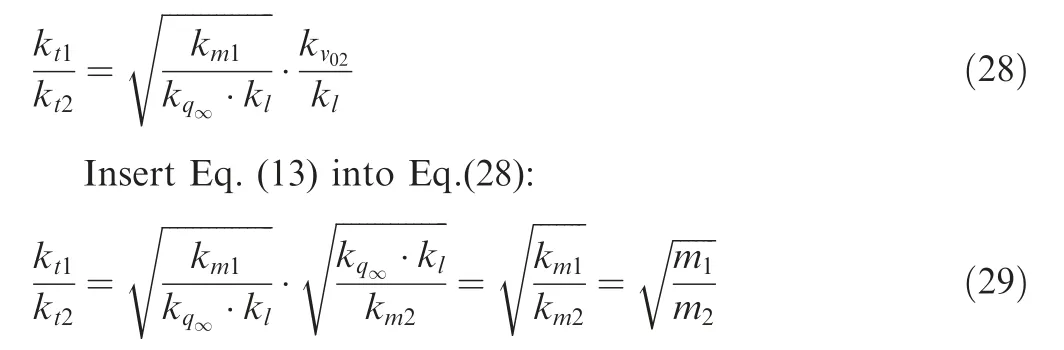
In the same way,as long as the mass m2of the lateral moving submunition is equal to the mass m1of the vertical downward submunition, it can be ensured that:

Therefore,as long as the mass characteristics of the submunitions are the same,the time between the submunitions can be constrained to be equal,and time correction is not necessary.If the mass of each submunition is not equal, it is necessary to modify the times of submunitions in different positions according to Eqs. (25) and (29).

4. Verification

After theoretical derivation,in order to verify the feasibility of the new similarity law, a numerical simulation of different states is carried out. Symbol Ssdata are obtained by CFD;the separation parameters satisfy those of a real aircraft.Symbol Sfordata are obtained by CFD; the separation parameters satisfy those of the previous wind tunnel-test method. Symbol Soptdata are obtained by CFD;the separation parameters satisfy the new similarity law. The separation curves of each method are obtained and the data are compared. Verification of the effect of this optimization follows.

4.1. Model used in verification process

The model used in this verification is the model in Ref. 18, as shown in Fig. 2.

Fig. 3 is the three-dimensional diagram and coordinatesystem description of the model used in this verification.

To realize the relative motion of multiple bodies,the dynamic overset unstructured grid method is used. In the solver, the unsteady compressible Reynolds-averaged Navier-Stokes equations are solved using the unstructured grid finite-volume method for spatial discretization and the implicit LU-SGS-based dual time-stepping scheme for temporal discretization.For separation problems,the trajectories of bodies can be obtained by coupling the six-degree-of-freedom motion equations.

4.2. State description

The real submunition parameters and wind tunnel-test model parameters are shown in Table 1.The mass of the wind tunnel model is calculated according to Eq. (26).

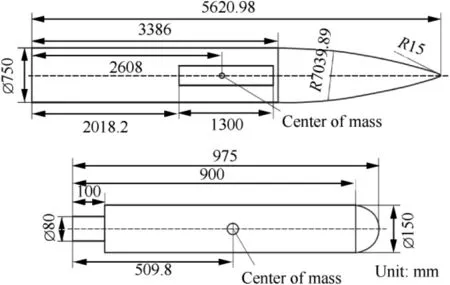
Fig. 2 Shape parameters of dispenser and submunition.

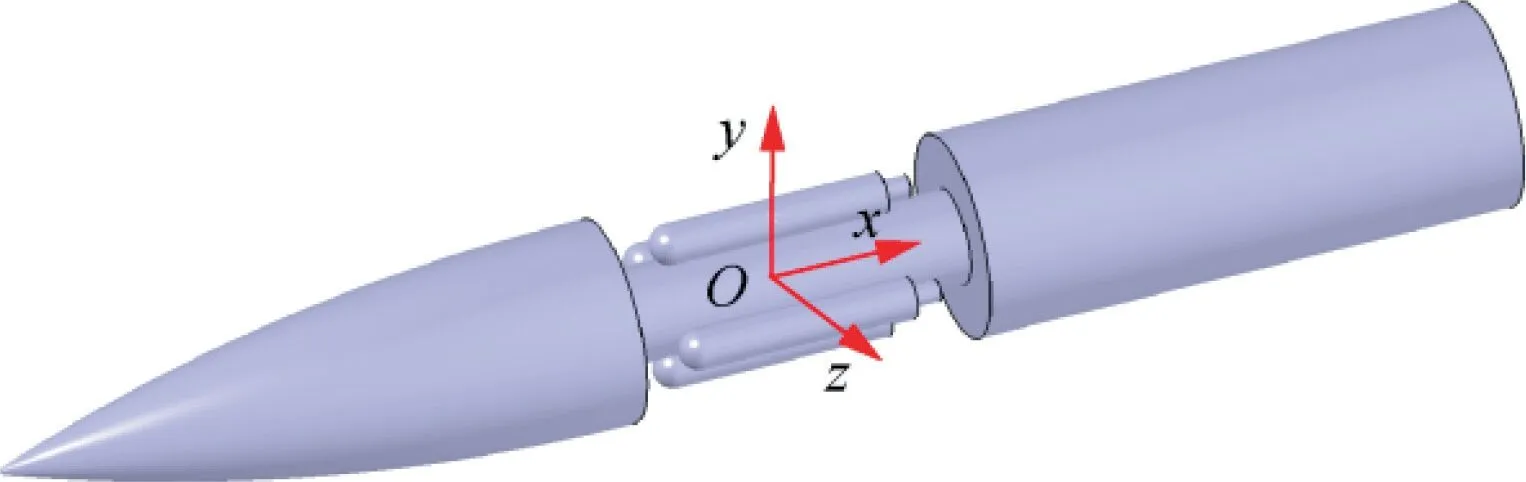
Fig. 3 Coordinate-system description.

The wind tunnel test(Sm)adopts the flow parameters of the FD12 wind tunnel, see Table 2 for details.

Table 3 shows the status of this simulation. The real-flight conditions (Ss) of the model are not scaled down, and the airflow parameters use the atmospheric-environment parameters,Ma=2.5,H=8 km.The angle reference value α0=15°,and the separation attack angle α=0°. The real aircraft scale is 1:1, and the wind tunnel state is 1:15.

5. Data analysis

5.1. Verification of trajectory similarity law of submunitions

Fig. 4 shows a data comparison between the test and the real vehicle for submunition 1 when the separation speed of the real submunition is v0s=4.5 m/s, and the reference length l0s=1500 mm. There is a difference between the trajectory of the vertical downward submunition obtained by the previous test similarity law and the trajectory of the real submunition. The new similarity law a good match to the real submunition trajectory, which is more real.

Fig. 5 shows a data comparison between the test and the real vehicle for submunition 2 when the separation speed of the real submunition is v0s=4.5 m/s. With respect to the derivation of the similarity law of horizontal submunitions,the new similarity law is the same as the previous similarity law, so the simulation results are consistent. The difference between the trajectory of horizontal lateral submunition obtained by the similarity law of previous experiments and that of the real submunition is small.

Fig. 6 shows a data comparison between the test and the real vehicle for submunition 3 when the separation speed of the real submunition is v0s=4.5 m/s. There is a difference between the trajectory of the vertical upward submunition obtained by the similarity law of previous experiments and that of the real submunition. The new similarity law a good match to the real submunition trajectory, and is more real.

Table 1 Real-submunition and model-submunition parameters.

Fig. 7 shows a data comparison between the test and the real vehicle for submunition 1 when the separation speed of the real submunition is v0s=7.0 m/s. There is a difference between the trajectory of the vertical downward submunition obtained by the previous test similarity law and the trajectory of the real submunition. The new similarity law a good match to the real submunition trajectory, and is more real.

Fig. 8 shows a data comparison between the test and the real vehicle for submunition 2 when the separation speed of the real submunition is v0s=7.0 m/s. It is consistent with v0s=7.0 m/s; the new similarity law is the same as the previous similarity law, so the simulation results are consistent.The difference between the trajectory of horizontal lateral submunition obtained by the similarity law of previous experiments and that of the real submunition is small.

Fig. 9 shows a data comparison between the test and the real vehicle for submunition 3 when the separation speed of the real submunition is v0s=7.0 m/s. There is a difference between the trajectory of the vertical upward submunition obtained by the similarity law of previous experiments and that of the real submunition. The new similarity law a good match to the real submunition trajectory.

When comparing the trajectories of a single submunition,it is found that the new similarity law has higher simulation accuracy for submunitions with different initial-motion directions, and can solve the problem of all submunitions designed by a previous similar law which leads to a large test error.

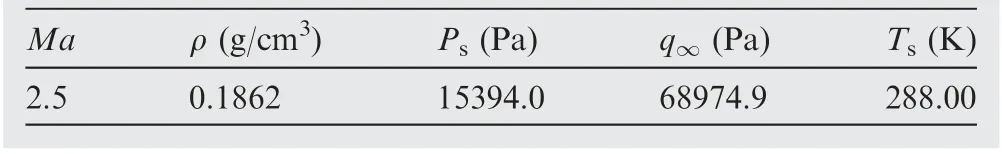
Table 2 FD12 wind tunnel parameters.

5.2. Verification of time correspondence between submunitions

After verifying that the new similarity law can better simulate the movement of a single submunition,the next step is to verify the time correspondence between submunitions. The new similarity law calculates the time-conversion relationship between submunitions. When the masses are the same, no time correction is needed. The reference time is defined as the time when the vertically downward moving submunition reaches the reference-length position. The reference time of v0s=4.5 m/s is t01, and the reference time of v0s=7.0 m/s is t02.

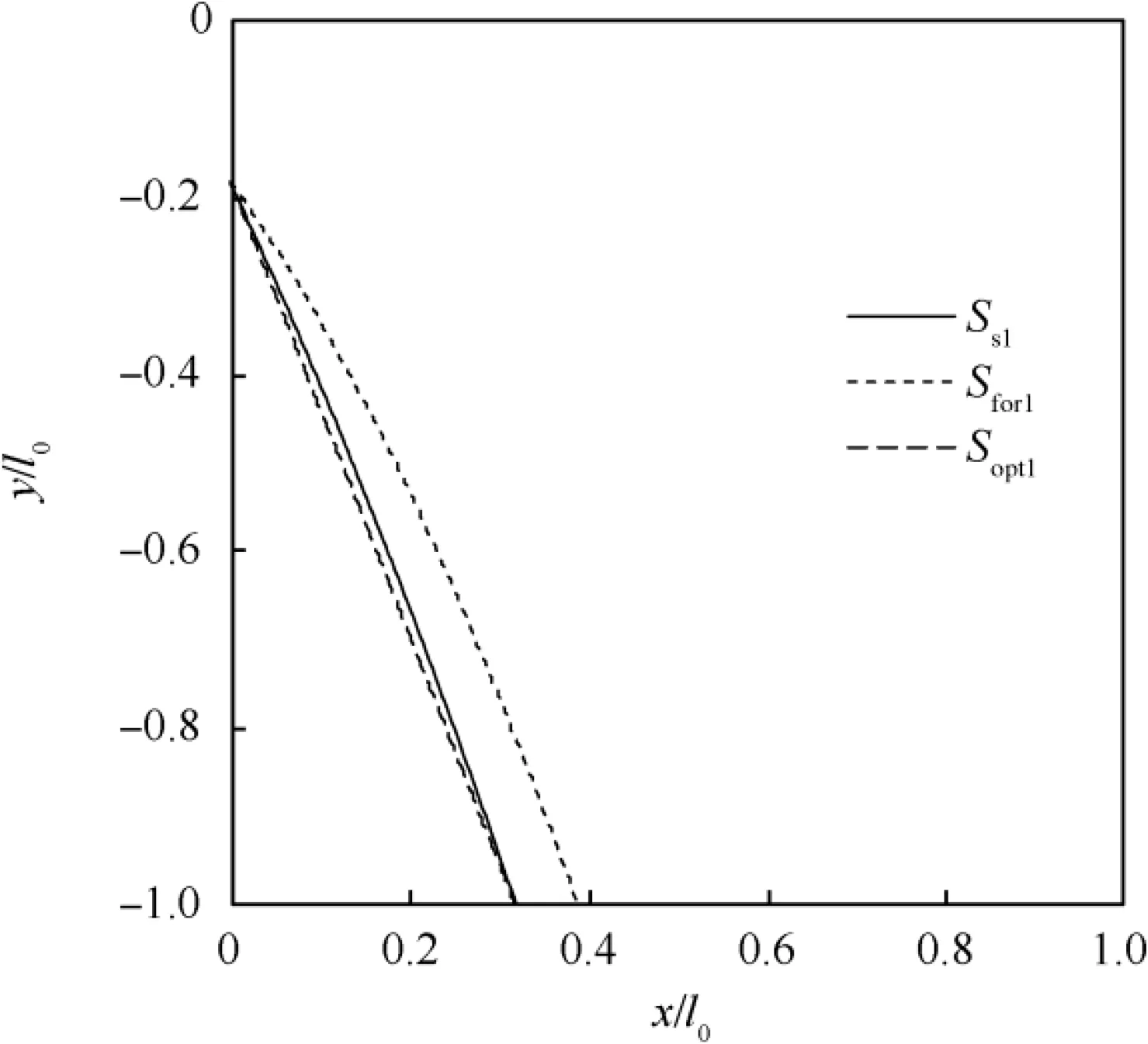
Fig. 4 Trajectory of initial vertical downward motion submunition, v0s=4.5 m/s.

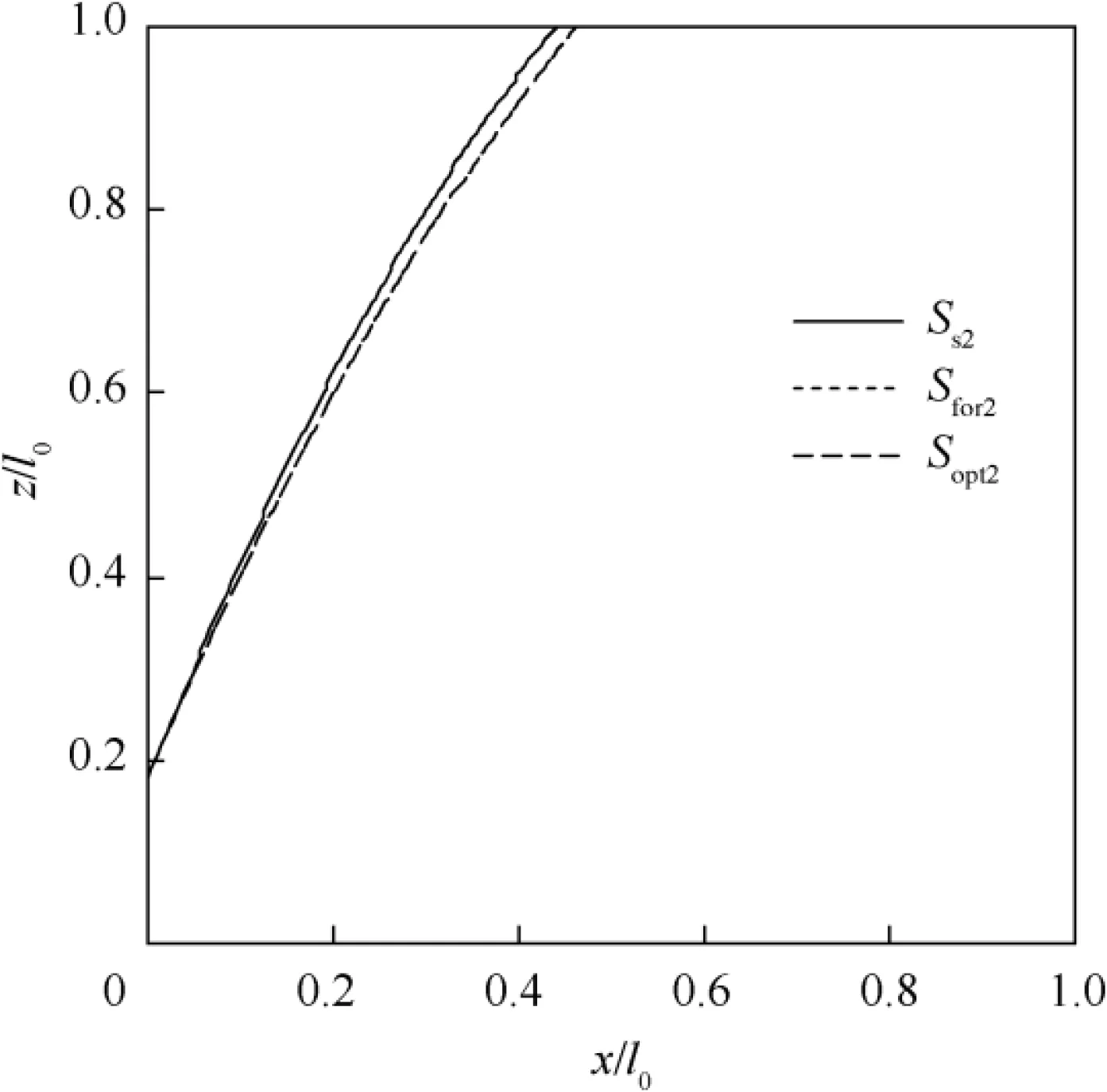
Fig. 5 Trajectory of initial horizontal lateral motion submunition, v0s=4.5 m/s.

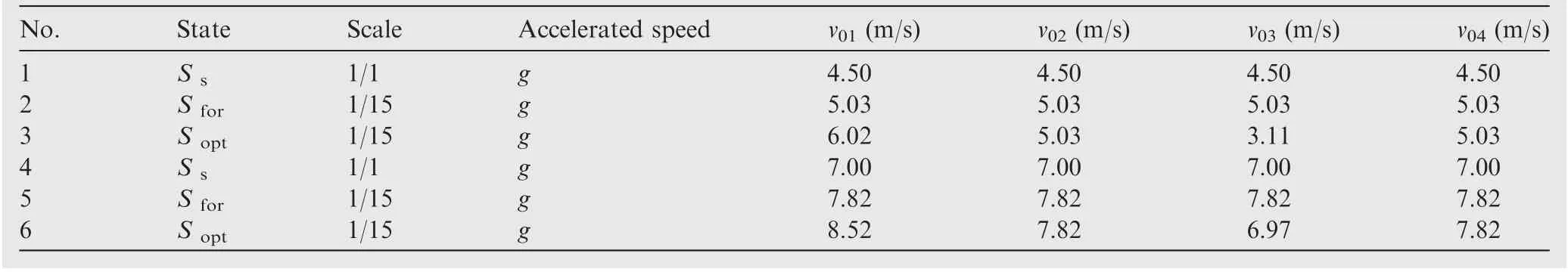
Table 3 Parameters of state arnd model.

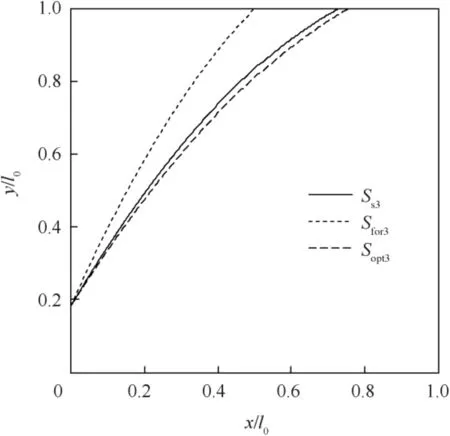
Fig.6 Trajectory of initial vertical upward motion submunition,v0s=4.5 m/s.

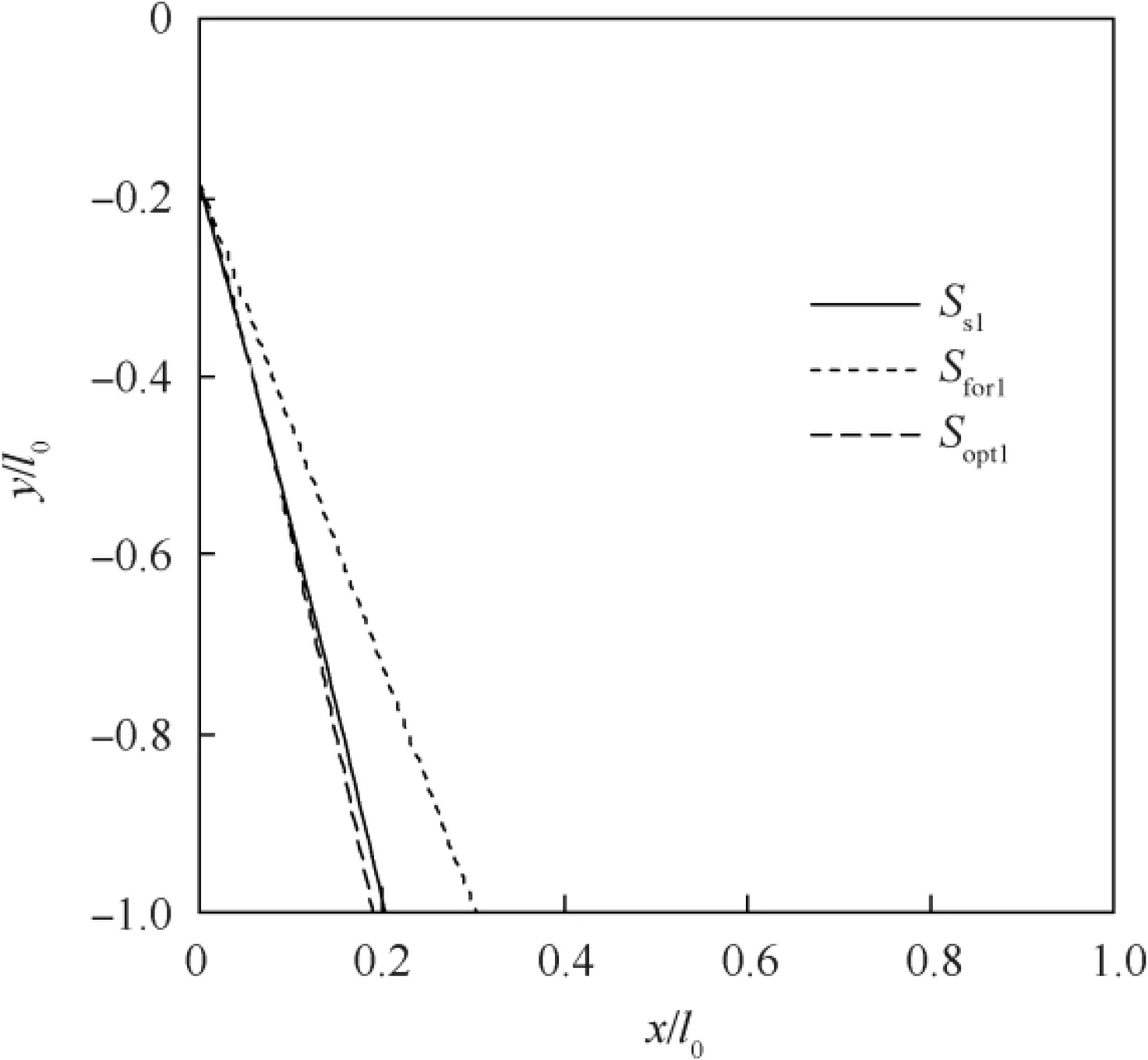
Fig. 7 Trajectory of initial vertical downward motion submunition, v0s=7.0 m/s.

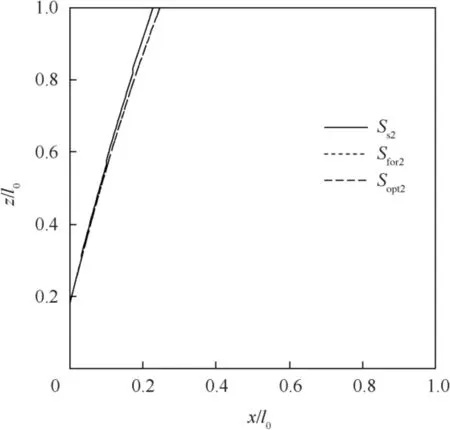
Fig. 8 Trajectory of initial horizontal lateral motion submunition, v0s=7.0 m/s.

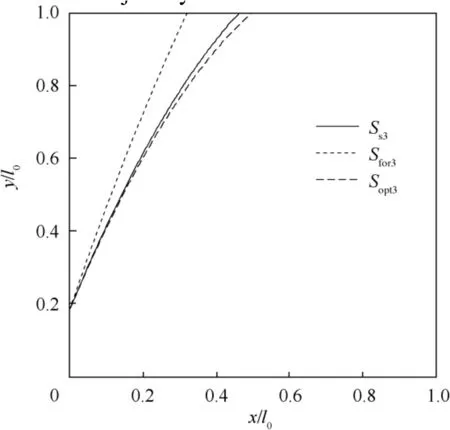
Fig.9 Trajectory of initial vertical upward motion submunition,v0s=7.0 m/s.

Fig.10 shows a comparison of submunition positions when v0s=4.5 m/s and t/t01=1. The submunition position obtained by the previous similarity law has a certain error.The horizontal submunition has little difference, but the vertical upward and vertical downward submunitions have large errors. The difference between the submunition position obtained by the improved similarity law and the real submunition position is small,and the error is small.The reason for the difference between the position and the corresponding value of the similarity law in previous tests is not due to an error in time scaling, but due to the similarity law used in previous tests.

Fig. 10 Comparison of submunition positions, v0s=4.5 m/s, t/t01=1.

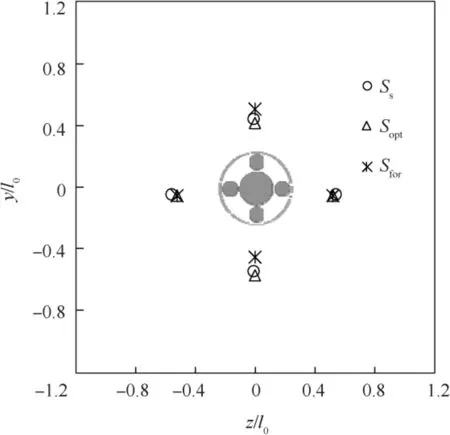
Fig. 11 Comparison of submunition positions, v0s=4.5 m/s, t/t01=0.5.

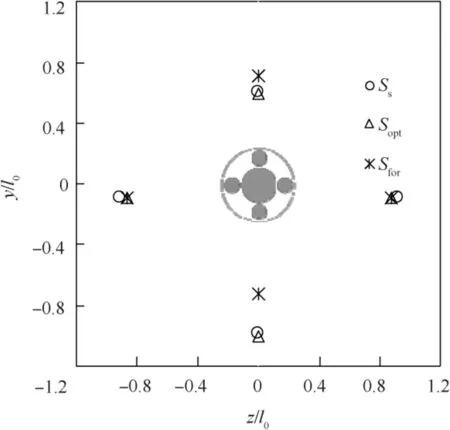
Fig. 12 Comparison of submunition positions, v0s=7.0 m/s, t/t02=1.

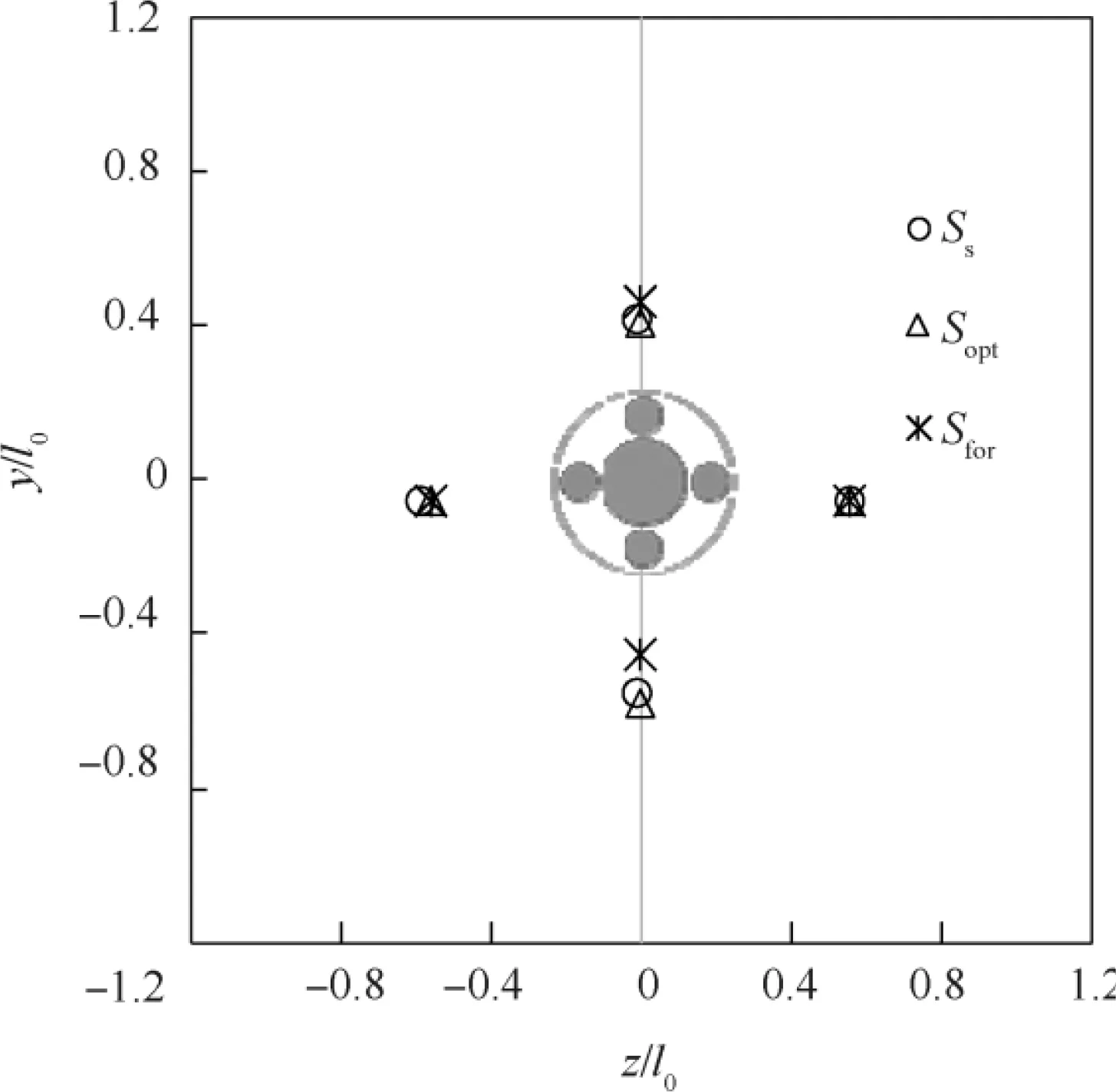
Fig. 13 Comparison of submunition positions, v0s=7.0 m/s, t/t02=0.5.

Table 4 Position error of each submunition, v0s=4.5 m/s.

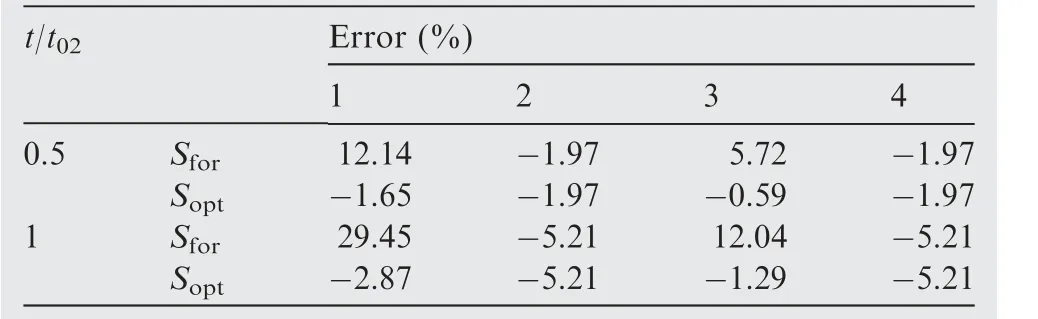
Table 5 Position error of each submunition, v0s=7.0 m/s.

Fig.11 shows a comparison of submunition positions when v0s=4.5 m/s and t/t01=0.5. From this, we can see similar problems with t/t01=1. Fig. 12 shows a comparison of submunition positions when v0s=7.0 m/s and t/t02=1. The position error of the submunition obtained by the previous similarity law is relatively large. The horizontal submunitions have some errors.The error of submunitions moving vertically upward and vertically downward is large. The difference between the submunition position obtained by the improved similarity law and the real aircraft position is small.The reason for the large, corresponding difference of position of the similarity law in previous experiments is due to the similarity law used in those experiments.

Fig.13 shows a comparison of submunition positions when v0s=7.0 m/s and t/t01=0.5. From this, we can see similar problems with t/t02=1.

The following is an analysis of the error.Table 4 shows the position error of each submunition for v0s=4.5 m/s. The difference between the data of Sforand Soptand that of Ssis calculated.

Table 4 shows that the wind tunnel test designed with the new similarity law can greatly reduce the error caused by the previous similarity law,and that the new similarity law is effective for many kinds of submunitions. The positions between submunitions in the wind tunnel test at the same time a good match to that of the real submunition, and the error is small.

Table 5 shows the position error of each submunition for v0s=7.0 m/s.When the separation speed of the real submunition increases,the wind tunnel test designed with the new similarity law greatly reduces the error caused by the previous similarity law; the new similarity law is effective for many kinds of submunitions. The positions between submunitions in the wind tunnel test at the same time a good match to the real aircraft, and the error is small.

Through a comparison of the data from different numerical simulations, the new similarity law can effectively guide the free-flight testing of a wind tunnel and improve the accuracy of wind tunnel testing.By comparing the trajectory of a single submunition, it is found that using the new similarity law of vertical upward moving submunition ensures that a wind tunnel test matches the real trajectory, and solves the problem of using a similarity law to design all submunitions, which may result in a large test error. In this paper, a new understanding of the separation physical process of horizontal lateral submunitions have been studied.By comparing the relative positions of the submunitions at a given time, it is found that the position relationships between the submunitions obtained by the new similarity law match those of the real submunition.

6. Conclusions

In this paper,a new similarity law for free flight in a wind tunnel is studied, which is aimed at solving the problem of different submunition parameters designed by one method in previous free-flight testing. The previous method leads to a large test error and the problem of time reduction between submunitions in wind tunnel testing. The new similarity law calculates the conditions for the motion similarity of a single submunition and the time-scaling correction-method between submunitions.Using a typical cluster munition,the separation of wind tunnel testing and real aircraft is simulated by numerical simulation,and the motion parameters of different submunitions are solved. The research shows that the design of wind tunnel testing using the new similarity law not only anticipates the real trajectory of a single submunition, but also the real,relative position of each submunition, at the same time, providing wind tunnel-testing accuracy. In addition to clustermunition separation, the similarity law can also be applied to the free-flight-test design of wind tunnels for vertical separation and horizontal separation of other kinds of aircraft.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgement

This work was supported by the Advanced Research Fund for Weapons and Equipment Development of China.