Finite-time composite guidance law with input constraint and dynamics compensation

Meijun DUAN, Di ZHOU

School of Astronautics, Harbin Institute of Technology, Harbin 150001, China

KEYWORDS Dynamics;Finite-time convergence;Guidance;Hysteresis-band switching;Near space interceptor;Output constraint

Abstract Guidance laws are proposed for a near space interceptor with on-off type thrust as output. The target-interceptor engagement kinematics is integrated with the first-order dynamics of thruster to design guidance laws.The bang-bang type guidance law with linear sliding mode is proposed to deal with thruster dynamics,and the sufficient condition for finite time convergence is rigorously proved based on the finite-time convergence theory.According to the sufficient condition,a sliding mode guidance law with hysteresis-band switching is introduced to reduce the switching frequency of thruster. Then two guidance laws are combined into a composite guidance law to improve the guidance performance. Simulation results show that the line-of-sight angular rate can converge to a neighborhood of the equilibrium point before the final time of the guidance process, and the composite guidance law has better performance than typical guidance laws.

1. Introduction

Near space hypersonic vehicle has exceeded the interception capability of traditional air defense missile for fast-moving and continuous-maneuvering, such as X-51A.1Kinetic Interceptor2(KI) can destroy targets via hit-to-kill technology,and there are following two control strategies. The Thruster Vector Control(TVC)is realized by utilizing gravity for accelerating interceptor in exo-atmospheric interception. The Divert and Attitude Control System (DACS) is used in the Terminal High-Altitude Area Defense (THAAD) system, and the system has the capability to intercept the hypersonic vehicles in near space. Due to the strong target maneuvers and large relative velocity, it’s necessary to study the robust guidance law with fast convergence.3Meanwhile,since the thruster on KI can only output an on-off type thrust with a lag,4pulsewidth modulation5and dynamics6should be considered in the design of terminal guidance law.

Finite-time control7is introduced to design the guidance law for fast convergence and strong robustness. Zhou et al.8designed finite-time guidance laws based on the sufficient condition for finite time stability of the Line-of-Sight(LOS)angular rate. Zhao et al.9developed an impact angle guidance law with finite time convergence.Zhang et al.10presented composite guidance laws based on finite time feedback control and nonlinear disturbance observer technique. But the above proposed guidance laws have neglected the dynamics which would cause bad influence on the guidance precision.

To compensate the bad influence of dynamics,11Sun et al.12designed a finite-time guidance law considering the first-order dynamics.However,its implementation is not easy for the existence of second-order derivative of LOS angle. Moreover,Golestani et al.13derived the finite time convergent guidance law considering the first-order dynamics by introducing finite-time integral back-stepping method. Qu and Zhou14designed a dimension reduction observer-based guidance law accounting for the second-order dynamics using the dynamics surface control method. But the guidance laws are difficult to be implemented when the guidance system of KI must output continuous acceleration.

Since the thrust produced by the thrusters on KI is approximately a constant with the form of discrete switches,15it’s necessary to design a jet logic for the thrusters according to a guidance law. Duan and Zhou16proposed a bang-bang type guidance law using the pulse thrusters on KI, but it’s not easy to implement any guidance law with continuous variations of acceleration. Zhou et al.17used pulse width pulse frequency (PWPF) modulator to approximately implement PN guidance law, but it may arouse the limit cycle and additional delay. Guo et al.18designed a PWPF modulator with variable dead zone, but switching frequency and fuel consumption are increased in the last phase of guidance process. Moreover, the guidance laws16-18have not considered the dynamics and are not finite time convergent.

With the above analysis,no result about finite-time convergent guidance law considering thruster dynamics described by a lag and on-off style is available in the literature.Herein aiming at the interception of hypersonic vehicle in near space, a composite guidance law is proposed. The main contributions are as follows:

(1) A finite-time guidance law considering thruster dynamics is proposed, and the sufficient condition for finitetime convergence is strictly proven. The guidance law has no derivatives of the LOS angular rate and is easy to be implemented by the thrusters on KI.

(2) Based on the sufficient condition for finite-time convergence, a variable hysteresis-band switching scheme is presented to attenuate the possible zigzag motion of the LOS angular rate. In this scheme the sensitivity of pulse switching is increased with the decreasing of the relative range.

2. Formulation

The geometry of planar interception is depicted in Fig. 1,respectively. The differential equation of LOS angular rate7is

where R and q denote the relative range and the LOS angle,respectively; aTand aIdenote the accelerations of the target and interceptor normal to the LOS, respectively.

The dynamics of thruster on KI can be approximately described as

where τ and u denote the time constant and guidance command, respectively. In particular, the value of aIis a constant or zero.

Define the LOS angular rate as x1= ˙q, and Eq. (1) can be written as

where

Define x2=-agx1-bgaI, and there is

Differentiating x2with respect to time,it follows from Eqs.(2) and (5) that

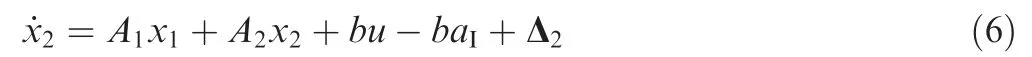
where

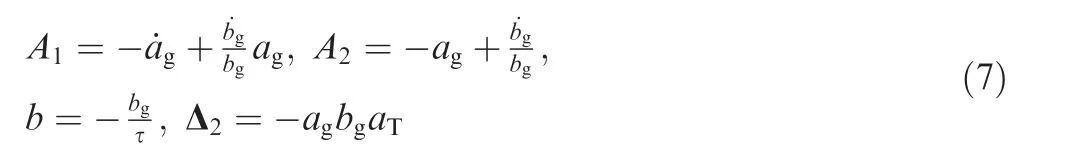
Combining Eqs. (3) and (6) gives the math model of the guidance system considering the first-order dynamics of thruster

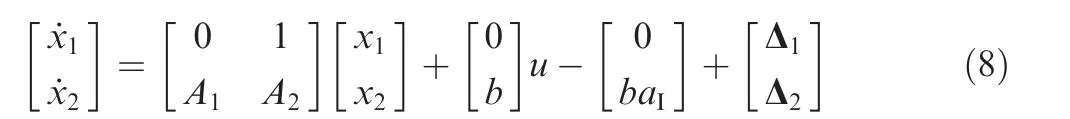
where the starting time of the guidance process is taken to be zero(i.e., t=0). The initial values of the state variables in the guidance system are denoted asR（0 ）,,x1（0）and x2（0）.At time t, they are denoted as R（t）, ˙R（t）, x1（t） and x2（t）.

Assumption 1. The target-to-interceptor relative velocity is approximately a constant, i.e., ˙R=-c， c=const.>0.

If Assumption 1 holds, Eq. (4) can be rewritten as

Substituting Eq. (9) into Eq. (7) yields

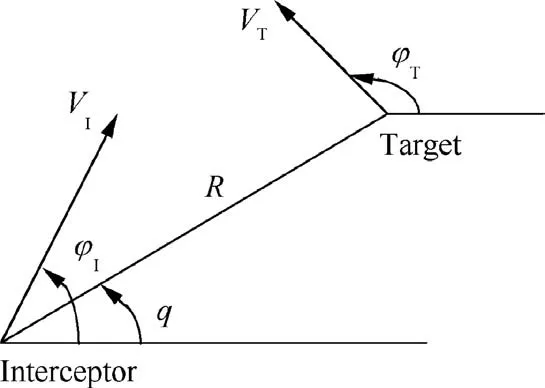
Fig. 1 Planar interception geometry.

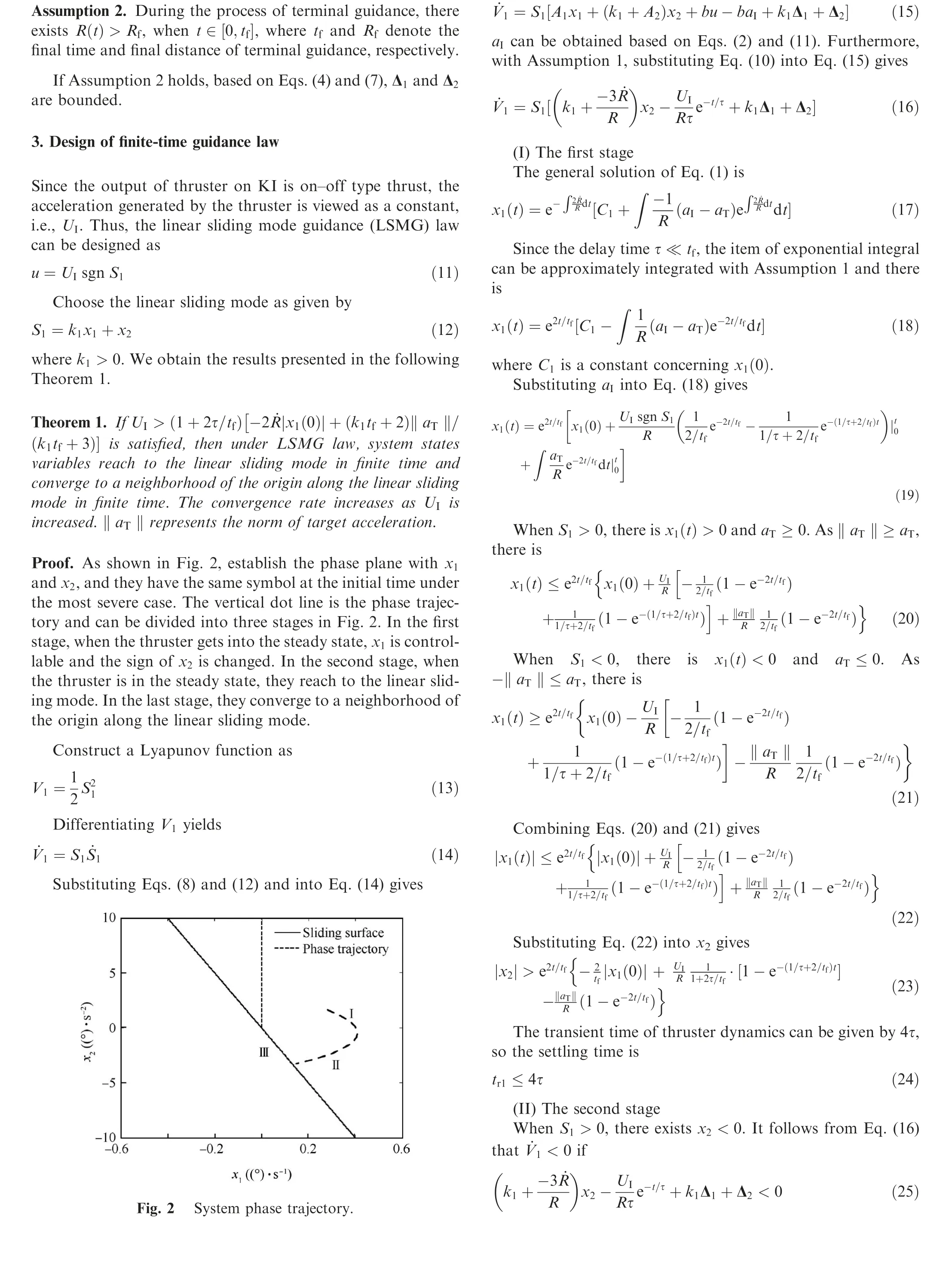
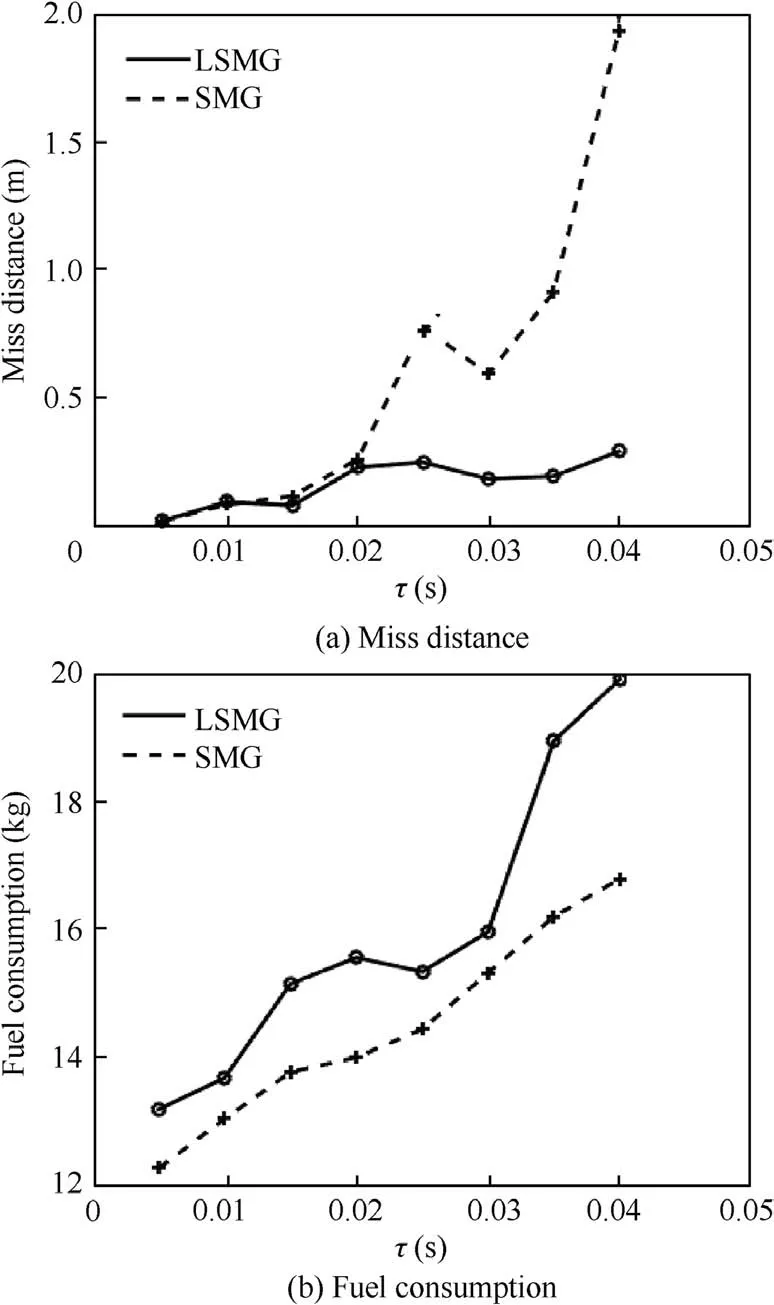
Fig. 4 Miss distance and fuel consumption for dynamics.

5.1. Influence of dynamics lag

To verify the importance of considering the dynamics lag,LSMG law and SMG law are studied under a group of delay time constants. The miss distance and fuel consumption are shown in Fig. 4, respectively. For contrast, both LSMG and SMG law limit the LOS angular rate within 0.02（◦）/s. So the parameters of LSMG law are chosen as k1=50 and 1（◦）/s when τ ≤0.01 s; k1=33 and 0.66（◦）/s when τ ≤0.02 s;k1=28 and 0.56（◦）/s when τ ≤0.03 s; k1=16 and 0.32（◦）/s when τ = 0.035 s; k1=11 and 0.22（◦）/s when τ = 0.04 s.

KI can’t destroy the target when the miss distance is larger than 0.3 m. From Fig. 4(a), it is seen that the miss distance under SMG law significantly increases withτincreased, and KI misses X-51A when τ>0.02 s. The miss distance under LSMG law can be reduced by adjusting parameters of sliding mode surface in guidance law Eq.(11).From Fig.4(b),the fuel consumption is increased asτis increased.

5.2. Sufficient condition for finite-time convergence

The sufficient condition for finite-time convergence in Theorem 1 consists of two aspects. One is the initial condition of terminal guidance, and the other one is the acceleration of thruster. Three kinds of flight-path angle are chosen, such as in θI0= 8◦;in θI0= 12◦;in θI0= 16◦.The LOS angular rates are plotted in Fig. 5(a). Three kinds of output thrust are chosen,such as in F=4800 N;in F=3840 N;in F=2800 N.And the corresponding accelerations are 5g，4g，3g, respectively.The LOS angular rates are plotted in Fig. 5(b).

It is clear from Fig.5 that LOS angular rates converge to a neighborhood of the origin in finite time.When the flight-path angles are increased,both the initial LOS angular rates and the setting time increase in Fig. 5(a).The convergence rates in Fig. 5(b) decrease as the output thrust are decreased. The settling time increases with the increasing of the initial LOS angular rate and the decreasing of the thruster acceleration. It can be obtained that the LOS angular rate would diverge when the sufficient condition isn’t satisfied.

5.3. Composite guidance law

The proposed LSMG law causes the zigzag motion of the LOS angular rate around the threshold,such that a pair of thrusters have to be frequently switched. Therefore, a sliding mode guidance law with hysteresis-band switching is employed to lower the switching frequency of thruster. CG,SMG, PN and DGG are studied in the same condition. The simulation results of miss distance and fuel consumption are summarized in Table 1.The variations of fuel consumption and LOS angular rates are plotted in Figs.6 and 7.The longitudinal acceleration are plotted in Fig. 8.

Fig. 5 LOS angular rate under different initial condition and output thrust.

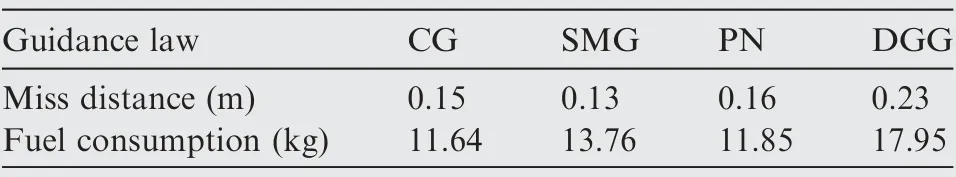
Table 1 Simulation results.

Fig. 6 Fuel consumption for guidance laws.

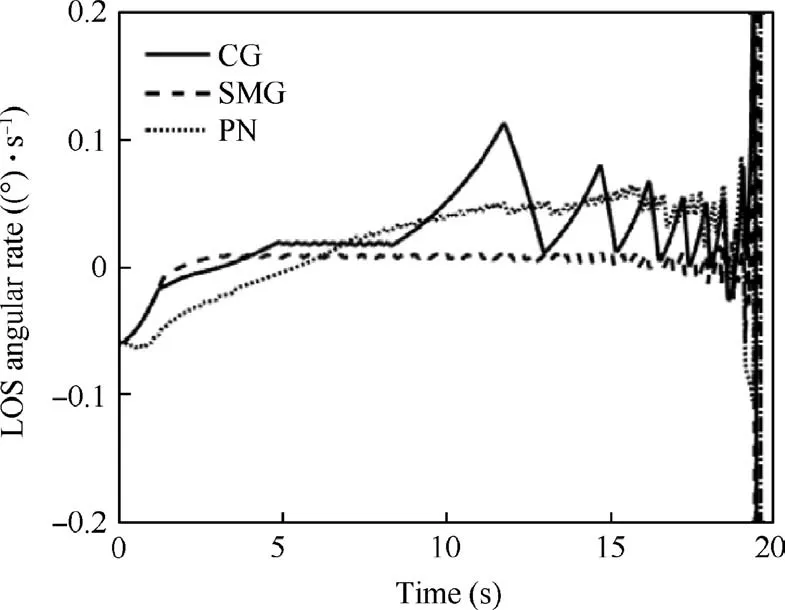
Fig. 7 LOS angular rate for guidance laws.

According to Table 1, the miss distances of guidance laws are all less than 0.3 m. From Fig. 6, the fuel consumption under DGG and SMG law is much more than the PN guidance and CG law. From Fig. 7, the LOS angular rate under CG converges to a neighborhood of the origin,and then stays between the opening threshold and the closing threshold. The LOS angular rate under SMG law converges to the dead-zone threshold,but gradually vibrates with the decreasing of relative range. The LOS angular rate under PN can’t converge to the origin while encountering the maneuvering target,and its convergence rate is the slowest one.

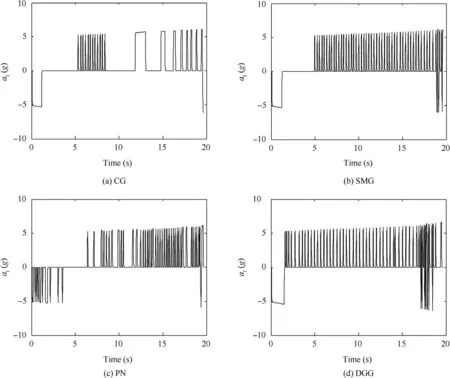
Fig. 8 Acceleration under different guidance law.

From Fig.8(b),the dead-zone in SMG law has reduced the chattering,but the switching frequency is high.A pair of thrusters work alternatively with the decreasing of relative range for the threshold is a constant.From Fig.8(c), under PN law,the opening time of thruster is short and the switching frequency is high due to PWPF modulator. From Fig. 8(d), under DGG law, the opening time of thruster in the beginning of terminal guidance is long to reduce the zero miss distance,but then the switching frequency is high and the fuel consumption always increases. From Fig. 8(a), under CG law, the opening time of thruster is long,so the convergence rate of LOS angular rate is quick. Moreover, the switching frequency is the lowest one for employing the variable hysteresis-band switching.

6. Conclusions

In this paper, a composite guidance law considering thruster dynamics is proposed for a near space interceptor. The main conclusions are as follows:

(1) The bang-bang type guidance law with linear sliding mode can ensure the guidance precision in the presence of thruster lag,and the LOS angular rate converges to a neighborhood of the equilibrium point in finite time.It is robust to target maneuvers and apt to be implemented by the on-off type thruster.

(2) The variable hysteresis-band switching scheme can effectively avoid the zigzag motion of the LOS angular rate around the threshold.

(3) Numerical simulation results show that, compared to the typical guidance laws, the proposed guidance laws not only send the LOS angular rate to the origin more rapidly but also ensure less fuel consumption and switching frequency of the thruster. Thus, the proposed guidance laws are potentially useful in practical applications.

Acknowledgement

This study was supported by the National Natural Science Foundation of China (No. 61773142).