两类非线性三阶四点边值问题解的存在性

林东海，裴明鹤

(北华大学数学与统计学院，吉林 吉林　132013)



两类非线性三阶四点边值问题解的存在性

林东海，裴明鹤

(北华大学数学与统计学院，吉林 吉林132013)

利用Leray-Schauder度理论，得到了非线性三阶微分方程x‴=f(t，x，x′，x″)，t∈[0，1]分别满足下列四点边界条件x(0)=0，x′(0)=αx′(ξ)，x′(1)=βx′(η)和x′(0)=αx′(ξ)，x(1)=0，x′(1)=βx′(η)的两类边值问题解的存在性，并且作为应用给出了一个例子.

Leray-Schauder度理论；Nagumo条件；四点边值问题；存在性

【引用格式】林东海，裴明鹤.两类非线性三阶四点边值问题解的存在性[J].北华大学学报(自然科学版)，2016，17(5)：572-576.

1　引　　言

本文考虑两类非线性三阶四点边值问题，即非线性三阶微分方程

x‴=f(t，x，x′，x″)， t∈[0，1]，

(1)

逐一满足下列四点边界条件

x(0)=0， x′(0)=αx′(ξ)， x′(1)=βx′(η)，

(2)

x′(0)=αx′(ξ)， x(1)=0， x′(1)=βx′(η)

(3)

的边值问题解的存在性，这里f(t，x0，x1，x2)在[0，1]×3上连续，ξ，η∈(0，1)，0<α≤1，0<β≤1，并且α+β≠2.

三阶微分方程出现于应用数学和物理学的许多领域，例如挠度具有常数或横断面发生变化的弯曲梁，三层梁以及电磁波或重力驱动流等[1-2].因此，三阶边值问题受到微分方程学者的广泛关注[1,3-17].而上述提到的成果大多是关于两点或三点边界条件的，而关于四点及其以上边界条件的成果较少见到[1，7，12-13].本文的目的是利用Leray-Schauder度理论，建立非线性三阶四点边值问题(1)-(2)和(1)-(3)的解的存在性结果.

2　主要结果

首先利用Leray-Schauder度理论建立非线性三阶四点边值问题(1)-(2)的解的存在性定理.

定理1假设

(ⅰ)f(t，x0，x1，x2)∈C([0，1]×3)，并且对每一个固定的(t，x1，x2)∈[0，1]×2，f关于x0单调递减；

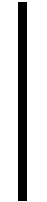
xf(t，x，x，0)>0；

(ⅲ)f(t，x0，x1，x2)满足Nagumo条件，即存在一个定义于[0，+)上的正值连续函数h(s)，使得∀(t，x0，x1，x2)∈[0，1]×[-M，M]2×，有

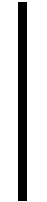
则三阶四点边值问题(1)-(2)至少存在一个解x=x(t)满足

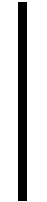
证明：首先验证下面的边值问题族

x‴=λf(t，x，x′，x″)，t∈[0，1]，λ∈[0，1]，

(4)

x(0)=0，x′(0)=αx′(ξ)，x′(1)=βx′(η)，

(5)

在C2[0，1]中先验有界.为此，设x(t)是BVP(4)-(5)的任意一个解.我们将证明

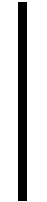
(6)

以及

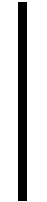
(7)

首先证明

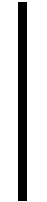
(8)

注意到，如果在方程(4)中λ=0，则BVP(4)-(5)只有平凡解，从而式(6)和(7)成立.因此可设λ∈(0，1].假设式(8)不成立，则存在t*∈[0，1]，使得

x′(t*)>M或x′(t*)<-M.

x′(t0)x‴(t0)=λx′(t0)f(t0，x(t0)，x′(t0)，0) ≥λx′(t0)f(t0，x′(t0)，x′(t0)，0)>0，

从而x‴(t0)>0，这与x′(t)在t=t0处达到其正的最大值以及x″(t0)=0矛盾，故式(8)成立.

于是由式(8)和边界条件(5)，有

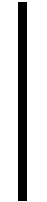
综上，不等式(6)成立.

兹断定存在t1∈(0，1)，使得

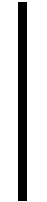
因此x″(t)在(t3，t4)内恒正或恒负.于是由假设条件(ⅲ)和N的定义，可得下面的矛盾：

故不等式(7)成立.于是由式(6)和(7)，有

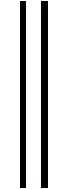
(9)

最后，我们将证明BVP(1)-(2)的解的存在性.为此，定义线性映射L：D(L)⊂C2[0，1]→C[0，1]如下：

(Lx)(t)=x‴(t)，x∈D(L)，

这里D(L)={x∈C3[0，1]：x(t)满足(5)}，则L是一对一映射.又定义非线性映射N：C2[0，1]→C[0，1]如下：

(Nx)(t)=f(t，x(t)，x′(t)，x″(t))，x∈C2[0，1].

则N是有界连续映射.再定义线性映射K：C[0，1]→C2[0，1]如下：

这里G(t，s)是x‴(t)=0满足边界条件(5)的格林函数.则易见，LKx=x，∀x∈C[0，1]，并且KLx=x，∀x∈D(L).更进一步，由Arzela-Ascoli定理可知，K将C[0，1]中的有界集映成C2[0，1]中的相对紧致集.因此KN：C2[0，1]→C2[0，1]是全连续的.

注意到x∈C3[0，1]是BVP(4)-(5)的解当且仅当x∈C2[0，1]是算子方程

Lx=λNx

的解.而算子方程Lx=λNx等价于算子方程

[I-λKN]x=0，

这里I：C2[0，1]→C2[0，1]是恒同映射.

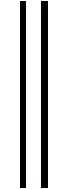
degLS(I-KN，Br，0)=degLS(I-λKN，Br，0)=degLS(I，Br，0)=1.

故KN在Br内有不动点x(t).易见，此不动点x=x(t)即为BVP(1)-(2)的解，并且满足

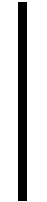
证毕.

对于三阶四点边值问题(1)-(3)，类似于定理1证明，可得如下结果：

定理2假设

(ⅰ) f(t，x0，x1，x2)∈C([0，1]×3)，并且对每一个固定的(t，x1，x2)∈[0，1]×2， f关于x0单调递增；

xf(t，x，-x，0)<0；

(ⅲ) f(t，x0，x1，x2)满足Nagumo条件，即存在一个定义于[0，+)上的正值连续函数h(s)，使得∀(t，x0，x1，x2)∈[0，1]×[-M，M]2×，有

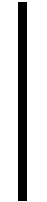
则三阶四点边值问题(1)-(3)至少存在一个解x=x(t)满足

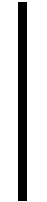
这里N与定理1中的相同.

3　例　　子

考虑三阶四点边值问题

x‴=-tx3+x′ex′2+x″2， t∈[0，1]，

(10)

x(0)=0， x′(0)=αx′(ξ)， x′(1)=βx′(η)，

(11)

这里ξ，η∈(0，1)，0<α≤1，0<β≤1，并且α+β≠2.

令

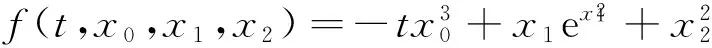
xf(t，x，x，0)=-tx4+x2ex2≥-x4+x2ex2.

而

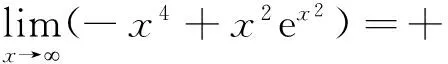
xf(t，x，x，0)>0.

此外，易见函数f(t，x0，x1，x2)满足定理1的假设条件(ⅰ)和(ⅲ).因此由定理1，三阶四点边值问题(10)-(11)至少存在一个解.

[1] H H Alsulami，S K Ntouyas，SA Al Mezel.A study of third-order single-valued and multi-valued problems with integral boundary conditions[J].Boundary Value Problems，2015,2015：25.

[2] M Gregus.Third order linear differential equations[M].Dordrecht：Reidel Publishing Co，1987.

[3] R P Agarwal.Boundary value problems for higher order differential equations[M].Singapore：World Scientific，1986.

[4] A Boucherif，N Al-Malki.Nonlinear three-point third-order boundary value problems[J].Appl Math Comput，2007，190：1168-1177.

[5] A Cabada，M R Grossinho，F M Minhós.On the solvability of some discontinuous third order nonlinear differential equations with two point boundary conditions[J].J Math Anal Appl，2003，285：174-190.

[6] C P Gupta，V Lakshmikantham.Existence and uniqueness theorems for a third-order three-point boundary value problem[J].Nonlinear Anal，1991，16：949-957.

[7] Z Du，X Lin，W Ge.On a third-order multi-point boundary value problem at resonance[J].J Math Anal Appl，2005，302：217-229.

[8] J R Graef，J Henderson，R Luca，etal.Boundary value problems for third-order Lipschitz ordinary differential equations[J].Proceedings of the Edinburgh Mathematical Society，2015，58：183-197.

[9] M R Grossinho，F M Minhós，A I Santos.Existence result for a third-order ODE with nonlinear boundary conditions in presence of a sign-type Nagumo control[J].J Math Anal Appl，2005，309：271-283.

[10] J Henderson.Best interval lengths for third order Lipschitz equations[J].SIAM J Math Anal，1987，18：293-305.

[11] B Hopkins，N Kosmatov.Third-order boundary value problems with sign-changing solutions[J].Nonlinear Anal，2007，67：126-137.[12] W Jiang，F Li.Several existence theorems of monotone positive solutions for third-order multipoint boundary value problems[J].Boundary Value Problems，2007,2007：1-9.[13] S Jin，S Lu.Existence of solutions for a third-order multipoint boundary value problem with p-Laplacian[J].Journal of the Franklin Institute，2010，347：599-606.

[14] R Ma.Multiplicity results for a third order boundary value problem at resonance[J].Nonlinear Anal，1998，32：493-499.

[15] M Pei，S K Chang.Existence and uniqueness of solutions for third-order nonlinear boundary value problems[J].J Math Anal Appl，2007，327：23-35.

[16] M Pei，S K Chang.Solvability of nth-order Lipschitz equations with nonlinear three-point boundary conditions[J].Boundary Value Problems，2014,2014：239.

[17] H Shi，M Pei，L Wang.Solvability of a third-order three-point boundary value problem on a half-line[J].Bull Malays Math Sci Soc，2015，38：909-926.

【责任编辑：陈丽华】

Existence of Solutions for Two Classes of Nonlinear Third-Order Four-Point Boundary Value Problems

Lin Donghai，Pei Minghe

(School of Mathematics and Statistics，Beihua University，Jilin 132013，China)

By using the Leray-Schauder degree theory，we obtained the existence of solutions for nonlinear third-order differentialx‴=f(t，x，x′，x″)，t∈[0，1]with one of the following sets of four-point boundary conditionsx(0)=0，x′(0)=αx′(ξ)，x′(1)=βx′(η)；x′(0)=αx′(ξ)，x(1)=0，x′(1)=βx′(η).Meanwhile，as an application of our results，an example is given.

Leray-Schauder degree theory；Nagumo condition；four-point boundary value problem；existence

1009-4822(2016)05-0572-05

10.11713/j.issn.1009-4822.2016.05.003

2016-06-15

吉林省教育厅科学技术研究项目(2016-45).

林东海(1982-)，男，硕士研究生，主要从事微分方程边值问题研究，E-mail：limdonghae@163.com；通信作者：裴明鹤(1963-)，男，博士，教授，主要从事微分方程定性理论研究，E-mail：peiminghe@163.com.

O175.8

A