Almost Fuzzy Compactness in L-fuzzy Topological Spaces
Li Hong-YananD Cui Wei
(1.School of Mathematics and Information Science,Shandong Institute of Business and Technology,Yantai,Shandong,264005)
(2.Department of Modern Science and Technology,Shenyang Municipal Party Committee Party School,Shenyang,110036)
Communicated by Lei Feng-chun
Almost Fuzzy Compactness in L-fuzzy Topological Spaces
Li Hong-Yan1anD Cui Wei2
(1.School of Mathematics and Information Science,Shandong Institute of Business and Technology,Yantai,Shandong,264005)
(2.Department of Modern Science and Technology,Shenyang Municipal Party Committee Party School,Shenyang,110036)
Communicated by Lei Feng-chun
In this paper,the notion of almost fuzzy compactness is defined in L-fuzzy topological spaces by means of inequality,where L is a completely distributive DeMorgan algebra.Its properties are discussed and many characterizations of it are presented.
L-fuzzy topological space,L-fuzzy almost compactness,L-fuzzy compactness,almost fuzzy compactness
2010 MR subject classification:54A40,54D30,03E72
Document code:A
Article ID:1674-5647(2015)03-0267-07
1 Introduction
Almost compactness is a very important concept.Many researchers have tried successfully to generalize the compactness theory of general topology to L-topology(see[1-9]).Recently,Shi[10]introduced new definitions of almost fuzzy compactness in L-topological spaces with the help of inequality,where L is a completely distributive DeMorgan algebra.The aim of this paper is to generalize the notion of almost compactness in[10]to L-fuzzy topological spaces,thus some properties and characterizations are researched.
2 Preliminaries
In this paper,(L,∨,∧,′)is a completely distributive DeMorgan algebra(i.e.,completely distributive lattice with order-reversing involution,see[11]).The largest element and thesmallest element in L are denoted by⊤and⊥,respectively.
Definition 2.1[12]An L-fuzzy topology on a set X is a map τ:LX→L such that


For a subfamily Φ⊆LX,2(Φ)denotes the set of all finite subfamilies of Φ.
Definition 2.2[13]is an L-fuzzy inclusion on X,it is defined asFor simplicity,it is denoted by[A~⊂B]instead of~⊂(A,B),
3 Definitions and Properties of L-fuzzy Almost Compactness
Definition 3.1Let(X,τ)be an L-fuzzy topological space and A∈LX.For all r∈L,

is called the r-interiors of A with respect to τ.The r-closures of A with respect to τ is defined as


An L-topology T can be regarded as a map χT:LX→L defined by
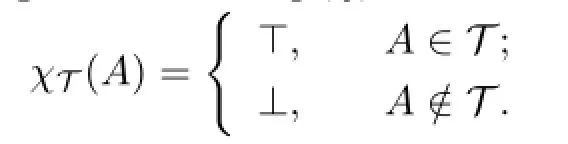
In this way,(X,χT)is a special L-fuzzy topological space and

This shows that Definition 3.1 can be regarded as the generalization in L-fuzzy topological space of the interiors and closures in L-topological space.
By Definition 3.1,we have the following theorem.
Theorem 3.1Let(X,T)be an L-topological space and A∈LX.Then,for all r,s∈L,
(6)A∈τr⇔τ(A)≥r.
Definition 3.2Let(X,τ)be an L-fuzzy topological space.G ∈LXis called L-fuzzy almost compact,if U⊆LX,it follows that


In an L-topological space(X,T),G∈LXis almost fuzzy compact(see[10])if and only if for all U⊆T,the inequality

is satisfied.
At the same time,(X,χT)is a special L-fuzzy topological space,and for all U⊆T,we have r=χT(U)=⊤.So,G∈LXis almost fuzzy compactness if and only if for all U⊆T,it follows that

Thus,the following theorem can be obtained.
Theorem 3.2Let(X,T)be an L-topological space and G∈LX.Then G is almost fuzzy compact in(X,T)if and only if G is L-fuzzy almost compact in(X,χT).
The definition of L-fuzzy compactness(see[14])in an L-fuzzy topological space can be described as follows:Let(X,τ)be an L-fuzzy topological space.G∈LXis called L-fuzzy compact if U⊆LX,then

In this way,the proposition“L-fuzzy compactness⇒L-fuzzy almost compactness”can be proved by Theorem 3.1.
By Definitions 2.1,2.2,3.1,3.2 and Theorem 3.1,we can prove the following theorem.
Theorem 3.3Let(X,τ)be an L-fuzzy topological space and G∈LX.Then G is L-fuzzy almost compact if and only if for all P⊆LX,one has

Theorem 3.4Let(X,τ)be an L-fuzzy topological space and G∈LX.Then G is L-fuzzy almost compact if and only if for all r∈L and for τ(U)≤r,U⊆τr,one has

Proof.(1)Necessity.Suppose that G is L-fuzzy almost compact,and for all r∈L and for τ(U)≤r,U⊆τr.As U⊆τr,by Theorem 3.1,we have τ(A)≥r for all A∈U.Then we get τ(U)≥r.Therefore,τ(U)=r.By L-fuzzy almost compactness of G,we have

(2)Sufficiency.Suppose that for all r∈L and for τ(U)≤r,U⊆τr.We have
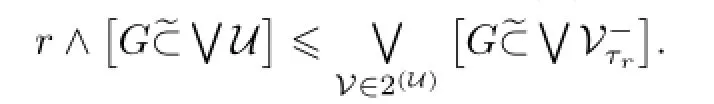
For all U⊆LX,let r=τ(U).Then for all A∈U,τ(A)≥r.So we get U⊆τrby Theorem 3.1.Thus

Therefore,G is L-fuzzy almost compact.
Theorem 3.5Let(X,τ)be an L-fuzzy topological space and G∈LX.If both G and H are L-fuzzy almost compact,then G∨H is L-fuzzy almost compact.
Proof.For all U⊆LX,let r=τ(U).Since G and H are L-fuzzy almost compact,we have
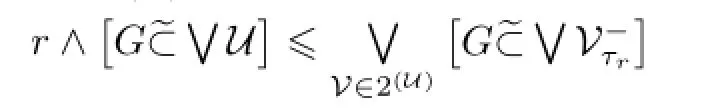
and

By the facts

and

we know that

Therefore,G∨H is L-fuzzy almost compact.
Theorem 3.6Let(X,τ)be an L-fuzzy topological space and G∈LX.If G is L-fuzzy almost compact,and H ∈LXwith τ(H)=τ∗(H)=⊤,then G∧H is L-fuzzy almost compact.
Proof.For all U⊆LX,let r=τ(U),W=U∪H′.Then we get W ⊆LX.It is easy to check



Then we obtain

from the following facts
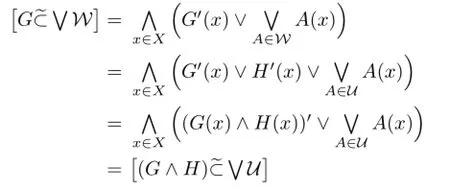
and

Therefore,G∧H is L-fuzzy almost compact.
4 Characterizations of L-fuzzy Almost Compactness
Definition 4.1Let(X,τ)be an L-fuzzy topological space,G∈LXand Ω⊆LX.Then
(1)Ω is r-cover(see[9],r-shading in[15])of G if and only if

(2)Ω is r+-cover(see[9],strong r-shading in[15])of G if and only if

(3)Ω is almost r-cover of G with respect to τ if and only if

(4)Ω is almost r+-cover of G with respect to τ if and only if

(5)Ω is an r-remote family(see[15])of G if and only if

(6)Ω is a strong r-remote family(see[15])of G if and only if

By Definitions 3.2,4.1 and Theorem 3.3,we can get the following theorem.
Theorem 4.1Let(X,τ)be an L-fuzzy topological space and G∈LX.Then the following conditions are equivalent:
(1)G is L-fuzzy almost compact;
(2)For any r∈L{⊤},each r+-cover U of G with τ(U)≰r has a finite subfamily V which is an almost r+-cover of G;
(3)For any r∈L{⊤},each r+-cover of G with τ(U)≰r has a finite subfamily which is an almost r-cover of G;
(4)For any r∈P(L),each r+-cover of G with τ(U)≰r has a finite subfamily which is an almost r-cover(r+-cover)of G;
(5)For any r∈P(L),each r+-cover U of G with τ(U)≰r has b∈α∗(r)and a finite subfamily V such that V is an almost b-cover of G;
(6)For any r∈L{⊤},each strong r-remote family P of G with τ∗(P)≰r′has a finite subfamily Q such thatis a strong r-remote family of G;
(7)For any r∈L{⊤},each strong r-remote family P of G with τ∗(P)≰r′has a finite subfamily Q such thatis a r-remote family of G;
(8)For any r∈M(L),each strong r-remote family P of G with τ∗(P)≰r′has a finite subfamily Q and b∈β∗(r)such thatis a strong b-remote family of G;
(9)For any r∈M(L),each strong r-remote family P of G with τ∗(P)≰r′has a finite subfamily Q and b∈β∗(r)such thatis a b-remote family of G.
Definition 4.2[15]Let(X,τ)be an L-fuzzy topological space,G∈LXand Ω⊆LX.We define that



It is easy to prove the following theorem.
Theorem 4.2Let(X,τ)be an L-fuzzy topological space and G∈LX.Then the following conditions are equivalent:
(1)G is L-fuzzy almost compact;
(2)For any r∈L{⊥}(r∈M(L)),each strong βr-cover U of G with r∈β(τ(U))has a finite subfamily V such thatis a(strong)βa-cover of G;
(3)For any r∈L{⊥}(r∈M(L)),each strong βr-cover U of G with r∈β(τ(U))has a finite subfamily V of U and b∈L(b∈M(L))with a∈β(b)such thatis a(strong) βb-cover of G;
(4)For any r∈L{⊥}(r∈M(L))and any b∈β∗(r),each Qr-cover U of G with τ(A)≥r for any A∈U has a finite subfamily V such thatis a Qb-cover of G;
(5)For any r∈L{⊥}(r∈M(L))and any b∈β∗(r),each Qr-cover U of G with τ(A)≥r for any A∈U has a finite subfamily V such thatis a(strong)βb-cover of G.
References
[1]Chen S L.Almost F-compactness in L-fuzzy topological spaces.Northeastern Math.J.,1991,7(4):428-432.
[2]Concilio A D,Gerla G.Almost compactness in fuzzy topological spaces.Fuzzy Sets and Systems,1984,13:187-192.
[3]Haydar Es A.Almost compactness and near compactness in fuzzy topological spaces.Fuzzy Sets and Systems,1987,22:289-295.
[4]Kudri S R T,Warner M W.Some good L-fuzzy compactness-related concepts and their properties I.Fuzzy Sets and Systems,1995,76:141-155.
[5]Kaur C,Sharfuddin A.On almost compactness in the fuzzy setting.Fuzzy Sets and Systems,2002,125:163-165.
[6]Meng H,Meng G W.Almost N-compact sets in L-fuzzy topological spaces.Fuzzy Sets and Systems,1997,91:115-122.
[7]Mukherjee M N,Sinha S P.Almost compact fuzzy sets in fuzzy topological spaces.Fuzzy Sets and Systems,1990,38:389-396.
[8]Mukherjee M N,Chakraborty R P.On fuzzy almost compact spaces.Fuzzy Sets and Systems,1998,98:207-210.
[9]Wen G F,Shi F G,Li H Y.Almost S∗-compactness in L-topological spaces.Iran.J.Fuzzy Syst.,2008,5(3):31-44.
[10]Shi F G.A new approach to almost fuzzy compactness.Proyecciones,2009,28(1):75-87.
[11]Liu Y M,Luo M K.Fuzzy Topology,Singapore:World Scientific Publishing,1997.
[12]H¨ohle U,Rodabaugh S E eds.Mathematics of Fuzzy Sets:Logic,Topology,and Measure Theory,The Handbooks of Fuzzy Sets Series,vol.3.Boston-Dordrecht-London:Kluwer Academic Publishers,1999.
[13]˘Sostak A P.On Compactness and Connectedness Degrees of Fuzzy Sets in Fuzzy Topological Spaces.in:General Topology and its Relations to Modern Analysis and Algebra,Berlin:Heldermann Verlag,1988:519-532.
[14]Shi F G,Li R X.Compactness in L-fuzzy topological spaces.Hacet.J.Math.Stat.,2011,40(6):767-774.
[15]Shi F G.A new definition of fuzzy compactness.Fuzzy Sets and Systems,2007,158:1486-1495.
10.13447/j.1674-5647.2015.03.09
date:Oct.28,2014.
The NSF(11471297)of China.
E-mail address:lihongyan@sdibt.edu.cn(Li H Y).
 Communications in Mathematical Research2015年3期
Communications in Mathematical Research2015年3期
- Communications in Mathematical Research的其它文章
- A Class of Schur Convex Functions and Several Geometric Inequalities
- Exact Controllability for a Class of Nonlinear Evolution Control Systems
- A Note on Weighted Composition Operators on the Fock Space
- Global Dynamics of a Predator-prey Model
- A Note on Zinbiel Algebras
- Multilinear Fractional Integral Operators on Morrey Spaces with Variable Exponent on Bounded Domain
