A Riesz Product Type Measure on the Cantor Group∗

SHI QI-YAN

(Department of Mathematics,Fuzhou University,Fuzhou,350108)

A Riesz Product Type Measure on the Cantor Group∗

SHI QI-YAN

(Department of Mathematics,Fuzhou University,Fuzhou,350108)

A Riesz type product as

Riesz product,Cantor group,weak topology,singularity of measure

1 Introduction

The Riesz product is a kind of lacunary series of trigonometric.It is an important topic in the field of harmonic analysis.The classical Riesz product measure is first introduced on the circle group T=R/Z by Riesz,and later generalized by Zygmund[1]as the weak limit of finite Riesz products

as N tends to in fi nity,where an’s are bounded by 1 and the integers λn’s are lacunary in the sense λn+1/λn≥3.In other words,there is a Radon measureµsuch that

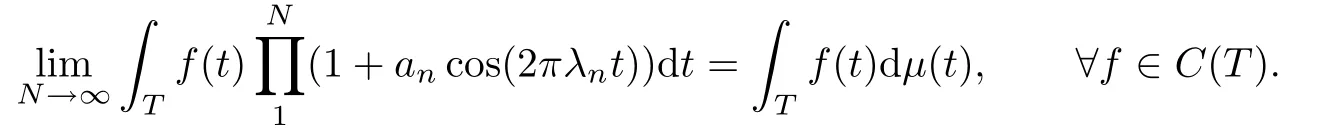
Moreover,this measure is continuous,that is,

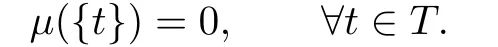
Later,Hewitt and Zuckerman[2]de fi ned Riesz products on a general non-discrete compact abelian group.A short description of their approach is as follows.

Let G be a nondiscrete compact abelian group with discrete dual groupΓ,Λbe a subset ofΓ,and W(Λ)be the set of all elements γ∈Γin the form of

where ǫk∈{−1,1}and λkare distinct elements ofΛ.Suppose thatΛsatis fies the requirement that each element of W(Λ)has a unique representation of the form(1.1)up to the order of the factors,and let α be any complex function onΛbounded by 1.For any finite setΦ⊂Λ,de fi ne a Riesz product on G as follows:

Hewitt and Zuckerman[2]showed that there exists a unique continuous probability measure µα,λon G which is the weak limit of P(Φ,α)dm in the topology of M(G),where M(G)is the convolution algebra of all Radon measures on G and m is the normalized Haar measure on G.A famous theorem of Kakutani[3]says thatµα,λis either absolutely continuous or singular with respect to the Lebesgue-Haar measure on G,according to whether α∈l2(Λ) or not.

The Riesz product is proved to be a source of powerful idea that can be used to produce concrete examples of measures with desired properties,such as singularity and multifractal structure.For the latter topic,refer to Peyriere[4]and Fan[5].

In this paper,we study a Riesz product type measure on the Cantor group.Throughout this paper,let

be the cartesian product with all factors equal to

Ωis well known as an abelian group under the operation of pointwise product.With the discrete topology on each factor,the product topology onΩmakes it a compact abelian group,the so-called Cantor group.This topology can also be induced by a metric that the distance between two elements ε=(εn)n∈N,δ=(δn)n∈NinΩequals to

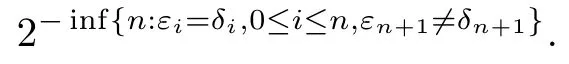
Denote the projection ωn:Ω→{−1,1}by

Elements in the dual groupΓofΩ,which are continuous group homomorphisms fromΩinto the multiplicative group of complex numbers of modulus 1,are provided by the projection functions.Precisely,let

Then each nontrivial element ofΓcan be uniquely written as

Note that for the normalized Haar measure m onΩ,{ωj}may be viewed as independent random variables taking values in{−1,1}with equal probability.We write dm as dω,and the Haar measure onΩj={−1,1}by dωjin the sequel.

Let M(Ω)be the convolution algebra of all Radon measure onΩ.As usual,we de fi ne the Fourier transform ofµ∈M(Ω)by

The following result due to Lvy is needed in the next section.

Theorem 1.1LetGbe a nondiscrete metrizable compact abelian group with discrete dual group Γ and let{µn}be a sequence of probability measures onG.Ifconverges everywhere in Γ and de fi nes a limit functionf,thenµnconverges weakly to a probability measureµonG,andf=.

The classical Riesz product measure onΩis of the form

As we have known,it is a continuous probability measure,and is either absolutely continuous or singular with respect to the normalized Haar measure m onΩaccording to whether{aj} is square summable or not.Moreover,if ajare all constants,the dimension and multifractal structure ofµare completely known(see[6]).Now it is natural to consider the following products:

where aj,bjare real numbers and

They are generalization of classical Riesz product measure onΩand give birth to essentially different properties compared with the classical ones.

2 A Measure

Consider the finite products onΩ

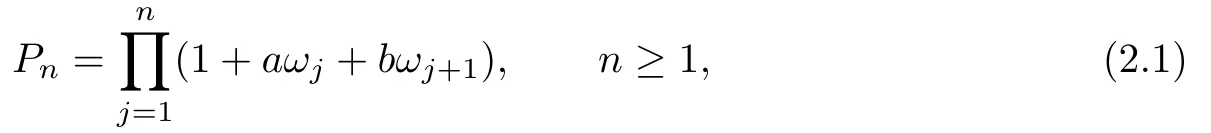
where a,b are two real numbers with

where uk,vkare real numbers independent of ω,and satisfy the following relations:

which can be seen from the formula(2.7),though they are not de fi ned in the beginning of this section.

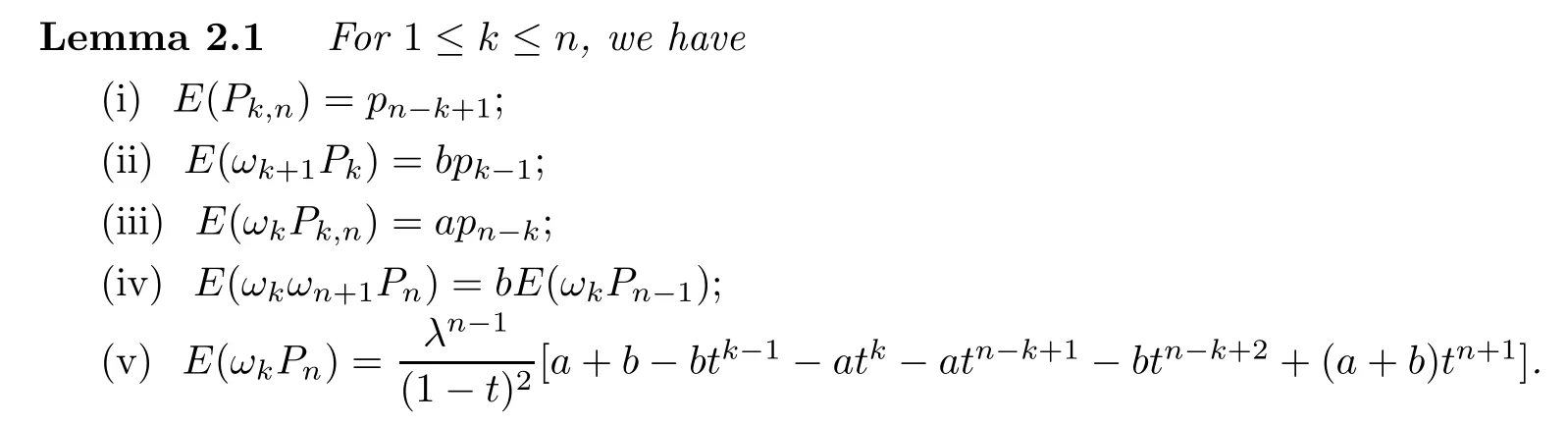
Proof.(i)–(iv)These four formulas are easy to be established.

(v)For convenience,we denote

Substituting(2.7)into the right hand side of the above equation and using the equation

we have the desired result.

By this lemma,we have

Proof.We prove thatˆµnconverges everywhere inΓand then apply Lvy theorem.Noticing that

for some probability measureµ∈M(Ω),and

Moreover,we have the following result.

Proposition 2.2µis a continuous measure.

Proof.Notice thatµis continuous if and only if

We only consider the case of ab0 because,in the case a or b is zero the corresponding measure is continuous,which has been discussed in Section 1.

If a,b have the same signs.Since

the right hand side in(2.8)tends to 0 as n→∞.

If a,b have the different signs,without loss of generality,we assume that

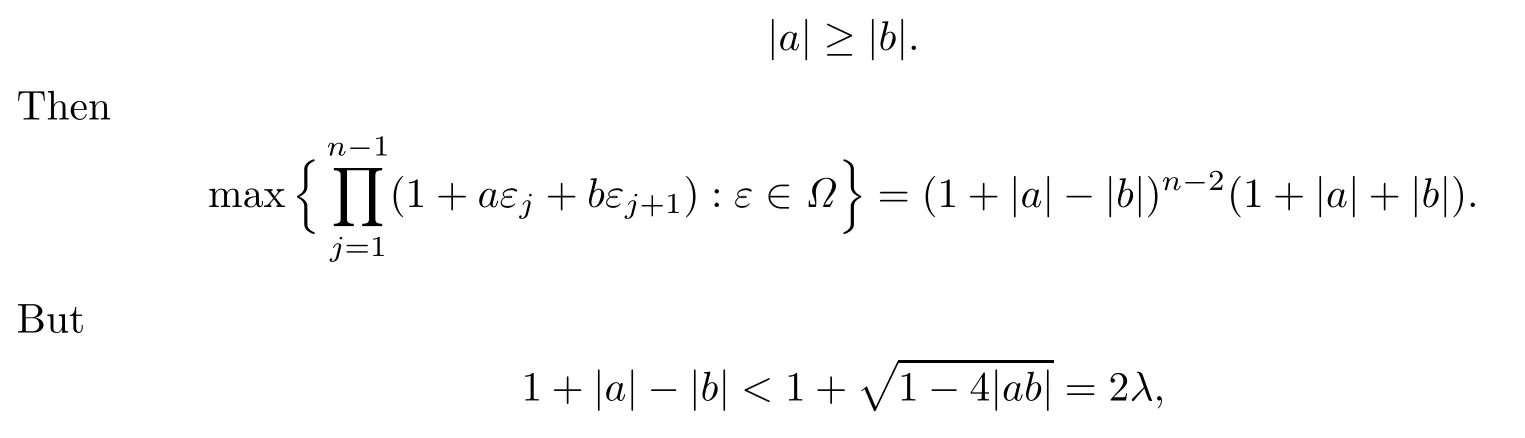
and thus the right hand side in(2.8)also tends to 0 as n→∞.This completes the proof.

3 Singularity

In this section,we show thatµde fi ned in the previous section is singular with respect to the normalized Haar measure m onΩin case a+b0.

Proposition 3.1Ifa+b0,the measuresµand the normalized Haar measuremonΩ are mutually singular.

4 Discussions

(i)By formula(2.8)we know thatµis a quasi-Bernoulli measure onΩ.The fractal analysis and the validity of multifractal formalism of such a measure were studied extensively by Brownet al.[7].

(ii)Our approach may be applied to the products

which can have even more items in the bracket,where a,b,c are real numbers with

(iii)For the general case of

[1]Zygmund,A.,Trigonometric Series,Vols.I,II,Cambridge University Press,Cambridge,1959. [2]Hewitt,E.and Zuckerman,H.,Singular measures with absolutely continuous convolution aquares,Proc.Cambridge.Philos.Soc.,62(1966),399–420.

[3]Kakutani,S.,On equivalence of in finite product measures,Ann.Math.,49(1948),214–224.

[4]Peyriere,J.,Etude de quelques proprietes des produits de Riesz,Ann.Inst.Fourier(Grenoble), 25(1975),127–169.

[5]Fan,A.H.,Quelques proprietes des produits de Riesz,Bull.Sci.Math.,117(1993),421–439.

[6]Shi,Q.,Riesz Product Type Measures on the Cantor Group,Lecture Notes of Seminario Interdisciplinare di Matematica IV,S.I.M.Dep.Mat.Univ.Basilicata,Potenza,2005,73–77.

[7]Brown,G.,Michon,G.and Peyriere,J.,On the multifractal analysis of measures,J.Statist. Phys.,66(1992),775–790.

Communicated by Ji You-qing

42A55,28A33

A

1674-5647(2010)01-0007-10

date:Nov.6,2007.