CFD simulation of hydrodynamics and mixing performance in dual shaft eccentric mixers

Songsong Wang,Xia Xiong,Peiqiao Liu,Qiongzhi Zhang,Qian Zhang,Changyuan Tao,Yundong Wang,Zuohua Liu,

1 School of Chemistry and Chemical Engineering, Chongqing University, Chongqing 400044, China

2 State Key Laboratory of Coal Mine Disaster Dynamics and Control, Chongqing 400044, China

3 School of Mechanical and Transportation Engineering, Chongqing University, Chongqing 400044, China

4 Department of Chemical Engineering, Tsinghua University, Beijing 100084, China

ABSTRACT This work aims to systematically study hydrodynamics and mixing characteristics of non-Newtonian fluid (carboxyl methyl cellulose,CMC) in dual shaft eccentric mixer.Fluid rheology was described by the power law rheological model.Computational fluid dynamics was employed to simulate the velocity field and shear rate inside the stirred tank.The influence mechanism of the rotational modes,height difference between impellers,impeller eccentricities,and impeller types on the flow field have been well investigated.We studied the performance of different dual-shaft eccentric mixers at the constant power input with its fluid velocity profiles,average shear strain rate,mixing time and mixing energy.The counter-rotation mode shows better mixing performance than co-rotation mode,and greater eccentricity can shorten mixing time on the basis of same stirred condition.To intensify the hydrodynamic interaction between impellers and enhance the overall mixing performance of the dual shaft eccentric mixers,it is critical to have a reasonable combination of impellers and an appropriate spatial position of impellers.

1.Introduction

The mixing operations of non-Newtonian fluid are commonly encountered in the chemical industry,which is extensively applied in many industries such as paint,food,cosmetic and pharmaceutical.The viscosity in different regions of the stirred tank is varied on account of the unique shear-thinning properties.This results in special mixing characteristics [1–3] and related issues,such as low heat transfer and high mixing time,formed ‘‘cavern effects”in the tank [4–9].The existence of these issues significantly reduces the efficiency of non-Newtonian fluid mixing process with the product quality influenced.Thus,it is extremely necessary to achieve efficient mixing and eliminate cavern effects by proper selection and reasonable optimization of the agitated system.

Many studies have been carried out on optimizing impeller structure to achieve efficient mixing of highly viscous and non-Newtonian fluids.Liuet al.[10] explored the chaotic mixing characteristics of high viscosity fluids with a rigid-flexible impeller in the tank.Compared with the traditional rigid impeller,the rigid-flexible impeller could improve the largest Lyapunov exponents(LLE)of the system by about 10.29%under the same working condition.Motleyet al.[11] studied the hydrodynamic properties of rigid and flexible impellers.They found that the flexible impeller can provide better hydrodynamic performance by utilizing its selfadaptive deformation characteristics in comparison to the rigid impeller.Muleet al.[12] investigated the mixing performance of fractal impeller and conventional impeller.They found the mixing time of fractal impeller was shorter than conventional impeller under the same stirring conditions.

Hybrid properties of highly viscous fluids or non-Newtonian fluids from the optimization and design of the stirring system have also been investigated.The fluids with complex rheological properties are efficiently mixed by the more advanced mixing system,such as the coaxial mixers [13–15],the planetary mixers [16,17],and dual shaft mixers[18,19].Pakzadet al.[20–22]systematically studied the mixing performance of non-Newtonian fluids with a coaxial mixer.They found that coaxial mixer was more conducive to achieving the mixing of non-Newtonian fluids compared with the traditional agitated tank.Liuet al.[23]explored the power consumption of a coaxial mixer consisting of an outer anchor and different inner impellers by stirring high viscosity fluidexperiments.Results showed that inner impeller has an obvious effect on the power consumption of outer impeller,and the power consumption of co-rotation mode was still superior to counterrotation mode.In addition,Liuet al.[24] also investigated power consumption and flow field characteristics of a coaxial mixer with a double inner impeller,they concluded that compared with single rotation of inner combined impeller,the velocity,shear rate and mass flow improve significantly under the double-shaft mixing.Tanguyet al.[25] concluded that the dual off-centered impellers were more likely to destroy the separation area and shorten the mixing time compared with the single-center impeller.Cabaretet al.[26] used dual off-centered impellers to research the hydrodynamic properties of high viscosity Newtonian fluids.The results indicated that dual off-centered shaft mixer was more energy efficient than single shaft mixer.

Fig.1. Schematic diagrams of stirred experimental system: (a) experimental apparatus,(b) dual shaft eccentric mixer,(c) three impellers.1–data collection and control system;2–frequency modulator;3–baffles;4–shafts;5–impeller;6–electromagnetic speed regulating motor;7–rotary torque sensor;8–tank.

Table1 Three impeller combinations

Table2 Dimensions of various parts in the stirred tank

Table3 The rheological parameter of CMC solution

Fig.2. Velocity distributions at axial direction with different mesh numbers(N=300 r∙min-1, r/R=0).

In our previous work [27],the hydrodynamic performance of three mixers (single shaft central mixer,single shaft off-centred mixer,dual shaft off-centred mixer) was investigated in the laminar regime.We found that dual shaft eccentric mixer has the advantage of energy saving.The above works clearly indicated that optimized impeller structures and advanced stirred systems are significant to promote the mixing efficiency of stirred tank with highly viscous or non-Newtonian fluids.So far,there are few studies on optimal impeller position,mixing performance and impellers interaction mechanism in non-Newtonian fluid system driven by dual shaft eccentric mixer.Here,we present a systematic study to investigate the effect of the operation mode,impeller type on power consumption and chaotic mixing performance in dual shaft eccentric mixer.Furthermore,the influences of impeller spacing,impeller eccentricity on mixing performance and interactionbetween impellers were also discussed based on the optimized operation mode.It is expected to provide a theoretical basis for the application of dual shaft eccentric mixers.

Fig.3. Computational grids of stirred tank: (a) stirred tank,(b) three impellers.

Fig.4. Comparison of CFD and experimental power values at different speeds.

Fig.5. The location diagram of tracer feed point and five monitoring points.

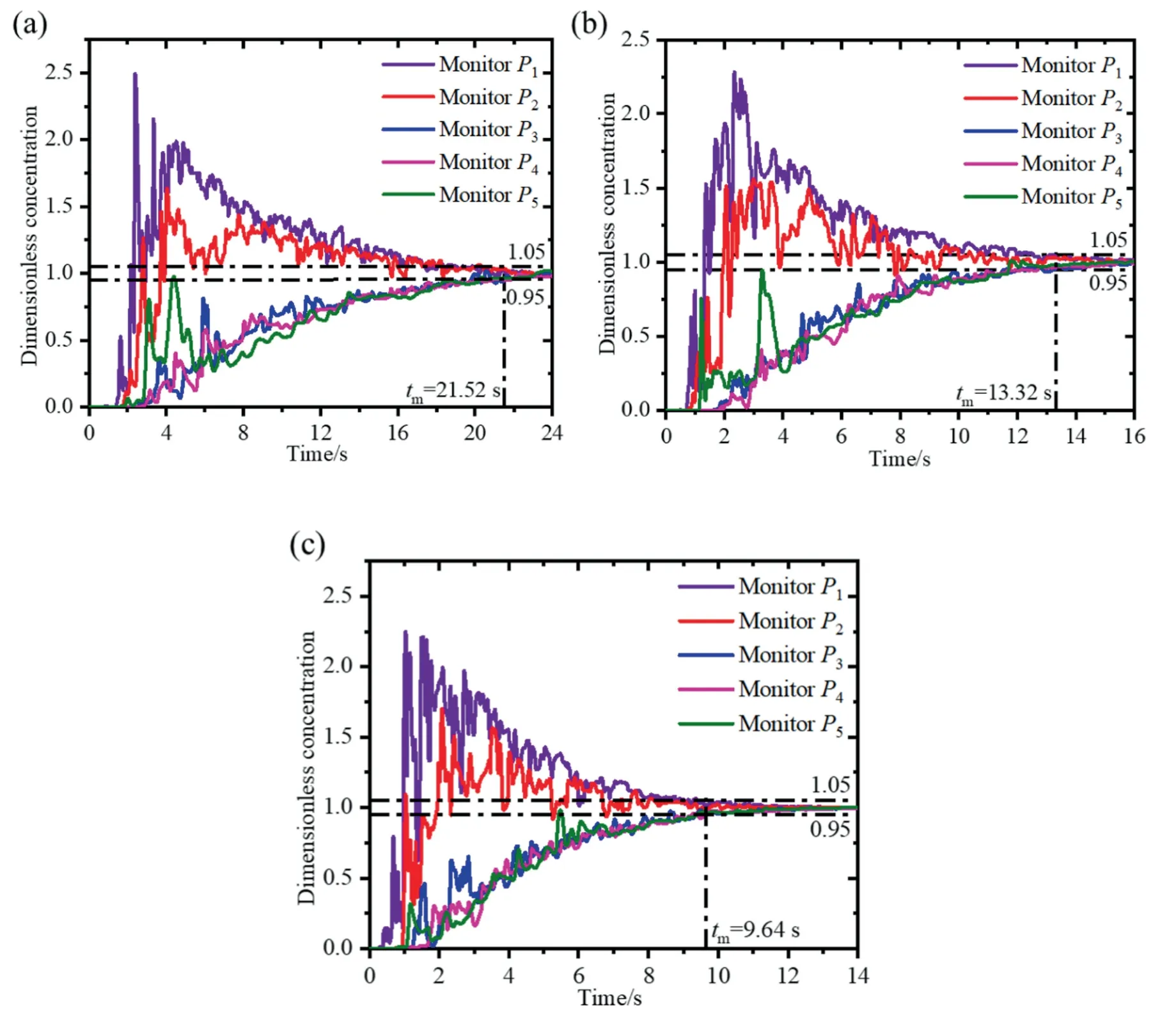
Fig.6. Concentration response curves for different detecting points at three rotational speeds: (a) 180 r∙min-1,(b) 300 r∙min-1,(c) 420 r∙min-1.

2.Experimental Set-up,Materials and Strategy

2.1.Experimental set-up

As illustrated in Fig.1(a),the experimental setup of CMC mixing was employed in present study.Main devices include data collection and control system,stirred equipment and electromotor.The rotational speed and direction of two shafts were independent of each other.Fig.1(b)shows the schematic diagram of the dual shaft eccentric mixer used in this study.The mixer was a cylindrical flatbottomed stirred tank with a diameter of 240 mm (T) and liquid filling up to 360 mm (H).Therefore,the height to diameter ratio of the stirred tank isH/T=1.5.The carboxyl methyl cellulose(CMC)was used as the working liquid.Four equally spaced baffles(width:w=T/10)were fitted in the tank,the clearance from baffle to the tank wall was 2 mm.Each shaft(diameter isd=14 mm)was equipped with a rotary torque sensor(HX-90D,Huaxin Electromechanical,China) to continuously measure the torque.As depicted in Fig.1(c),the three impellers used in experiment were Rushton turbine (RT),pitched blade down-pumping Rushton turbine(PBRTD) and pitched blade up-pumping Rushton turbine (PBRTU)(diameter isD=Dd=Du=T/3).

Three impeller combinations were formed from the above three impellers as shown in Table 1.

The inclined angles(θ)of PBRTDand PBRTUblade are all 45°.All blade thicknesses were 2 mm.In addition,the dimensions of various parts are list in Table 2.

2.2.Materials and measurement strategy

2.2.1.Materials

The concentration,densities,consistency index and power law index of the CMC solution applied in this study are listed in Table 3[28].

The CMC solutions are typical non-Newtonian fluid with shear thinning performance,and their rheological properties could be described by the power-law model [29,30].

The Reynolds number for non-Newtonian can be also defined in terms of the apparent viscosity,and the value of Reynolds number can be obtained as follows [13,31]:

whereDis the impeller diameter,m;ksis the Metzner–Otto constant.The Metzner–Otto constantkswas determined through the experimental calibration for RT,PBRTDand PBRTUto obtain the values of 11.5,11.2,and 11.2,respectively.

2.2.2.Power consumption

As mentioned above,the mixing power was calculated by the torque method.To eliminate the influence of friction and other factors,the torque was measured under load (Ml,l,Mr,l) and without load(Ml,0,Mr,0),respectively.All torque tests were repeated at least 3 times to eliminate accidental errors.Power consumption of the stirred tank can be calculated by the following formulas.

Fig.8. Distributions of Pv and average strain rate under different operating modes: (a) relationship between tm and Pv,(b) average strain rate.

wherePlandPris the power consumption of left impeller and right impeller,respectively,MlandMris the torque of left impeller and right impeller,respectively.

3.Numerical Methodology

3.1.Theoretical basis and boundary conditions

In this simulation work,the flow medium was a single-phase,isothermal,and incompressible flow of CMC inside the stirred tank.The rotational speed was set between 60 to 480 r∙min-1in computational fluid dynamics (CFD) calculation with the corresponding Reynolds numbers (Re*) range from 27.75 to 287.07.Therefore,the laminar flow model was employed to simulate the mixing of CMC solution [4,13].The continuity and momentum equations were solved separately and simultaneously.

The mass conservation equation [32] of the flow model is

where ρ is the fluid density;tis the flow time;u,v,andware velocity components inx,yandzdirections.

Momentum conservation equations [32] are as follows:

whereFx,FyandFzare the stresses received by the fluid element in thex,y,andzdirections,respectively.When the stress is gravity only,Fx=0,Fy=0,andFz=-ρg.

The fluid domain was discretized with tetrahedral mesh by the software Fluent 2020 customized.The entire flow region was divided into three regions: the upper impeller region,the lower impeller region and the other region.In addition,the region near the impeller and wall were refined owing to the large velocity gradient.For the same system,Fig.2 shows that the variation trend of velocity distribution at axial direction with different mesh number.The original 3D mesh had about 348526 cells.To verify the grid independence [24,27,29],the number of cells was increased to 669632 and 1188557 cells,respectively.These increased changed the velocity in rotor region by more than 3%.In order to balance the accuracy of simulation results and calculation resources,2 mm and 3 mm were the grid sizes of rotational domain and static domain,respectively.Finally,the number of cells was further increased to 2479973,the power number and velocity changed by less than 3%.Therefore,2,479,973 cells were employed in CFD simulations.Specific meshing is shown in Fig.3.

The multiple reference frame (MRF) method was adopted to simulate the rotation zone [33,34].This method has been found to make flow field predictions comparable to those obtained using the sliding mesh (SM) approach,and meantime,the MRF method can reduce computational requirements [35,36].The secondorder upwind discretization scheme was adopted to calculate the momentum equations.Pressure–velocity coupling was performed by SIMPLE algorithm [27,30].Fig.S1 (in Supplementary Material)shows that the specific boundary conditions of the stirred tank.The upper surface was assumed as ‘‘symmetry” boundary.The coincident surfaces between the rotor zone and stator zone were set as ‘‘interface”.Faces of the tank wall,shaft and impellers were all no-slip wall boundaries.The 3D coordinate origin was chosen at the center of bottom surface of the tank.Convergence residuals for each equation were set to 1 × 10-5.

3.2.Validation of computational model

The power consumption experiment of CMC with mass fraction of 0.5%was carried out to verify the simulated results.Experimental values of power consumption at different speeds were compared to CFD calculated values as shown in Fig.4.The calculated values showed very good agreement with experimental data at different stirred speeds in a maximum deviation no more than 9.5%.These data verified the correctness of the laminar flow model established in this work.

3.3.Mixing time

Mixing rate is an important indicator in industrial production which reflects the time required to complete the mixing process.Numerical simulations of the mixing procession are conducted through detecting the tracer concentration [37,38].The location diagram of feed point (P0) of the tracer and five monitoring points(P1–P5) is shown in Fig.5.The mixing time (tm) can be obtained based on the 95% principle [38],which is same as our previous work [27].The tracer concentration response curves at five monitoring points are shown in Fig.6.As can be seen,the concentrationcurves ofP1andP2increased rapidly and then gradually decreased,while the other three monitor points increased slowly.Finally,five curves tend to be a straight line,which means the completion of the mixing process.Thetmof 180,300 and 420 r∙min-1was 21.52,13.32 and 9.64 s,respectively.

Fig.9. Velocity distributions of different height difference at 300 r∙min-1: (a)velocity contours,(b)velocity vectors and streamlines,(c)velocity contours of cross section.

4.Results and Discussion

4.1.Effect of rotation modes

Fig.7 shows flow field with stirred speeds under two rotation modes.We can see that both impellers exhibit typical flow field of radial impeller.Fluid circulation in the stirred tank is significantly enhanced as speed increases from 180 to 420 r∙min-1.The overall flow field structure of the two rotation modes is similar.The main difference lies in the middle of the dual shaft and the right area of the stirred tank.It can be found that the vector distribution is more dispersed under the counter-rotation mode,and it is easy to form a larger vortex under the co-rotation mode.However,the powerful local circulation made the fluid stay too long at some places and blocked the overall mixing of fluid in a tank[24].Subsequent work will quantitatively analyze the mixing time and power consumption to determine the optimal rotation mode.The mixing efficiency is usually characterized by mixing energy per unit volume(Wv)and power consumption per unit volume(Pv)[39,40].TheWvandPvare defined as follows:

whereVis the total volume of fluid in a stirred tank,tmis the mixing time.

Fig.8(a) shows the relationship betweentmandPv.As we can see from Fig.8,tmdecreases continuously as the increase ofPv,and is very close for two rotation modes whenPvis higher than 0.4 kW∙m-3.At the samePv,thetmof counter-rotation mode is shorter than co-rotation mode.Therefore,counter-rotation mode can be recommended for the future in industrial production involving mixing of non-Newtonian fluids because of its shortest mixing time for the given power consumption.

Fig.8(b) shows the average shear rate separately on 24 cross sections perpendicular to the shaft.The shear rate near the RT impeller is the most intensive region under two rotation modes,and the shear rate in lower impeller region is slightly larger than that in upper impeller region.Moreover,the average shear rate in each plane increases with the increasing of impeller speed under both rotational modes.Compared with co-rotation,counterrotation enhances shear performance in the whole tank,especially in the area where the impeller is located,which means that the shear performance of the counter-rotation mode is better than that of co-rotation mode.The reason may be that the two blades could successfully achieve wave-riding and wave-passing to increase hydrodynamic interaction between the blades,as proposed by Yuanet al.[41].

4.2.Effect of impeller spacing

The velocity contours and 3D streamlines of different height between two impellers is showed in Fig.9 and Fig.S2,respectively.As shown in Fig.9(a)and(c),they are equivalent to a large impeller when both impellers are located at the bottom of the tank (C2/H=0.2,0.3),where only the fluid at the bottom region is mixed thoroughly.The 3D streamlines in the middle area of two shafts forms a vortex,which prevented circulation of fluid in the upper part of tank (as shown in Fig.S2).The dual shaft mixer strengthened the hydrodynamic interaction between impellers through increasing left impeller off-bottom heightC2(as shown in Fig.S2(C2/H=0.3–0.7)),enhanced the instability of flow field anddestroyed local circulation structure in the upper part of tank,resulting in more unstable flow field and higher mixing efficiency.The small impeller spacing enhanced the interaction between the two blades,but shortened the energy transfer process,so that the energy was directly dissipated in the form of small-scale vortices near the blades.As the spacing became larger,large-scale vortex discharged by the blade gradually evolved into a mesoscale vortex,and finally dissipated energy in the form of a small-scale vortex.The results were consistent with the transfer of energy and momentum.Therefore,it is recommended to place the two impellers on the upper and lower parts separately.

Fig.10. Distributions of velocity statistics,average shear rate,mixing time and mixing energy under different C2/H:(a) velocity,(b) average shear rate,(c) mixing time and mixing energy.

Fig.10 shows the distribution of velocity statistics,average shear rate,mixing time and mixing energy under differentC2/H.As shown in Fig.10(a),there is no significant difference in the overall velocity distribution between the six height differences at the velocity range above 0.3 m∙s-1,which is related to the fact that the high velocity zone is mainly distributed near the impeller region.At the low velocity range (0–0.2 m∙s-1),especially the speed value around 0.1 m∙s-1,we can find the largest ratio when the left impeller is located at 0.7H.At the same stirred speed,the velocity distribution in the vicinity of impellers is similar,and the difference is mainly reflected in the low-velocity flow region between the impellers.This is related to different coupling effects between the two blades at different spatial positions.To well analyze the flow field created by the dual shaft eccentric mixers,the average shear rate of each cross section under differentC2/Hare shown in Fig.10(b).It can be noticed that the average shear rate generated by the mixer with differentC2are reasonably different.The larger deformation (the peak value of average shear rate) is attained in the area close to the two impellers.It is started from an almost zero value in the lower part of the tank with an increase to the tip of lower impeller,and then it is reversed in middle area of impellers.As the axial height increases,the average shear rate also increases to the tip of upper impeller.Finally,it is reversed in the upper part of the upper impeller.The average shear rate of the whole tank is maximum when the bottom height of the left impeller is 0.7H,which indicated that the overall fluid in the tank has the best shear mixing performance.

For further analysis,the mixing time and mixing energy for different off-bottom heights of the left impeller was investigated.As can be seen in Fig.10(c),the mixing time and mixing energy decrease significantly with increasing theC2,and have the minimum values when the height is 0.7H.However,both values have a greater increase with the further increase of height from 0.7Hto 0.8H,which suggested that the synergistic effect between the two impellers is reduced.Therefore,the left impeller at a height ofC2=0.7Hshould be selected for the subsequent study.

4.3.Effect of impeller eccentricity

Fig.S3 shows the 3D streamlines of different impeller eccentricities.As depicted in Fig.S3,with increasing the eccentricity frome/T=0.18 toe/T=0.24,the 3D streamline distribution in the whole tank is denser and wider,especially in the middle region of theblades.The reason is that the increase of eccentricity enhances hydrodynamic interaction between impeller and wall,in turn strengthens the overall transport of fluid in the mixer,especially the region between impellers.

Fig.11. Distributions of velocity statistics,average shear rate,mixing time and mixing energy under different impeller eccentricities:(a)velocity,(b)average shear rate,(c)mixing time and mixing energy.

Distribution of velocity,average shear rate,mixing time and mixing energy under different impeller eccentricities were quantified and analyzed,as shown in Fig.11.As can be seen in Fig.11(a),the overall velocity distribution is similar for all three,but the velocity percentage increases with increasing eccentricity in the velocity interval 0.08–0.2 m∙s-1.This also verified that increasing the eccentricity is helpful to strengthen the coupling between the impellers and enhance the local velocity distribution.The average shear rate of each cross section under different impeller eccentricities is demonstrated in Fig.11(b).At the same height,the average shear rate ofe/T=0.24 is larger compared withe/T=0.18 ande/T=0.21.It is more obvious at the height where the impeller is located.At the larger eccentric position (e/T=0.24),more energy is dissipated at the region between two paddles due to the larger shear rate,which improved the overall shear mixing performance.

It is necessary to compare thetmandWvfor the tank at different impeller eccentricities.Fig.11(c) shows the comparison oftmandWvfor the three eccentricities under the same stirred condition.We find thetmandWvunsurprisingly decreased linearly as an increase in the eccentricity.At the sameRe*,thetmofe/T=0.24 is nearly 13.2% and 7.1% shorter than that ofe/T=0.18 ande/T=0.21,respectively.TheWvofe/T=0.24 is nearly 8.6% and 6.9% lower than that ofe/T=0.18 ande/T=0.21,respectively.It indicates that greater eccentricity can shorten mixing time on the basis of same stirred condition,where the advantages ofe/T=0.24 in terms of mixing rate are illustrated.

4.4.Effect of impeller types

The influence of impeller types on the shear rate and mixing performance in the stirred tank are further investigated under the optimal impellers position and counter-rotation mode.The RT,PBRT impeller were selected as the representatives of the radial flow impeller and mixed flow impeller,respectively,among which PBRT has two forms of down-pumping mode (PBRTD) and uppumping mode (PBRTU).The 3D streamlines of different impeller combinations are illustrated in Fig.S4.Compared with PBRTD+RT combination impellers,the PBRTU+RT and RT+RT agitated tanks have lower fluid velocity and flow range in the middle of the impellers.

Fig.12 shows the velocity statistics,average shear rate,mixing time and mixing energy distribution under different impeller combinations.As shown in Fig.12(a),the velocity statistics of different impeller combinations is mainly different in low-velocity range(0.08–0.2 m∙s-1).It can be found that the overall velocity distribution of PBRTD+RT combined impeller is optimal in the range.This is related to down-pump flow pattern generated by PBRTDimpeller,which strengthens the flow in the low-velocity zone,especially the region between two impellers.Fig.12(b) shows the average shearing rate distribution under different impeller combinations.Among three impeller combinations,the overall average shear rate distribution of the tank is similar.Compared with the PBRTDimpeller,PBRTUand RT have greater average shear rate in the upper region,and smaller in the lower region.Compared with PBRTUand RT impeller,the down-pumping mode of the PBRTDimpeller can produce a strong axial flow along with a radial flow in the middle region.Therefore,PBRTDas upper impeller is recommended for dual shaft eccentric mixers in agitation of non-Newtonian fluid.Comparison will be quantified later in this work with regard to the mixing time and mixing efficiency.

Fig.12. Distributions of velocity statistics and average shear rate under different impeller combinations: (a) velocity,(b) average shear rate,(c) mixing time and mixing energy.

Fig.13. The maximum deviations of the influence of different variables on mixing performance: (a) mixing time,(b) mixing energy per unit volume.

As can be seen from Fig.12(c),RT+RT(II)combination impeller has the lowest values oftm,but its larger torque value leads to the largestWvin the three impeller combination.TheWvof PBRTD+RT(I) were nearly 9.5% and 5.0% shorter than that of RT+RT (II) andPBRTU+RT (III),respectively.These results confirm our previous finding that the PBRTD+RT (I) was more effective than the other combination impellers in the mixing of non-Newtonian fluids.

Fig.14. Schematic diagrams of fluid transport mechanism: (a) e/T=0.18, C1=0.2H, C2=0.3H,(b) e/T=0.24, C1=0.2H, C2=0.7H.

4.5.Fluid transport mechanism

Combining with the former analysis and study,Fig.13 shows the maximum deviation of the influence of different variables on mixing performance.As shown in Fig.13,impeller spatial position,that is,the impeller spacing and impeller eccentricity,has the greatest influence on the mixing time and mixing energy per unit volume under the same stirred condition.Compared with the impeller spatial position,the rotation mode and impeller type have less effect on the mixing performance of non-Newtonian fluid.

Fig.14 shows the fluid transport mechanism of two impellers at different spatial positions.As shown in Fig.14(a),when the spatial distance between the blades was small,the convection rate between the blades was strong,which increased the fluid velocity and enhanced the local chaotic mixing.However,multiple isolated mixing regions were formed in the upper part of tank and near the blade ends,which was insufficient and unfavorable in momentum and mass exchange.Unsurprisingly,when the spatial distance was large (Fig.14(b)),the overall flow field has a more uniform axial velocity distribution.The vortex at the upper part of tank has disappeared significantly,and near the blade ends vortex begins to dissipate toward the wall.Vortex dissipation means the release of momentum inside the vortex,which is conductive to the overall flow field mass and momentum transfer effects.The reason for the disappearance of the isolated mixing region was that the process of transporting fluid between the blades became longer and the wave transfer range became wider,which in turn enhanced the nonlinear behavior of the fluid transfer process and formed the wave-vortex coupling phenomenon.In general,an optimized blade arrangement is essential to improve the mixing performance and energy efficiency of the mixing system.

5.Conclusions

The hydrodynamic performance of dual shaft eccentric mixer was assessed with regards to fluid field,shear strain rate and mixing time through numerical simulation.Moreover,the effects of the rotational modes,height difference between impellers,impeller eccentricity and impeller types on the hydrodynamic performance in a stirred tank were also made an exploration deeply.The conclusions are obtained as follows:

(1)The mixing time decreases continuously as the increasingPv.At the samePv,the mixing time of counter-rotation mode is shorter than co-rotation mode.The shear performance of counter-rotation mode is better than that of co-rotation under the same rotational speed.

(2) Under the counter-rotation mode,the height difference between two impellers has an important influence on the enhancement of hydrodynamic interaction.The stirred tank had better mixing performance when the heights of two impellers were 0.7Hand 0.2H,respectively.The greater eccentricity can shorten mixing time on the basis of same condition.

(3) TheWvof PBRTD+RT (I) were nearly 9.5% and 5.0% shorter than that of RT+RT(II)and PBRTU+RT(III),respectively.PBRTD+-RT (I) was more effective than the other combination impellers in the mixing of non-Newtonian fluids.

(4)Compared with rotation modes and impeller types,the spatial position of two impellers has a greater effect on mixing performance of non-Newtonian fluids.

The studied results could help further understand the mixing mechanism of the dual shaft eccentric mixing system,and provide a reference for the scale-up study of dual shaft eccentric stirred tank.

Data Availability

Data will be made available on request.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

This work was supported by the National Natural Science Foundation of China (22078030,52021004),National Natural Science Foundation of Chongqing (2022NSCQ-LZX0271),Fundamental Research Funds for the Central Universities (2022CDJQY-005),National Key Research and Development Project(2019YFC1905802,2022YFC3901204),Key Project of Independent Research Project of State Key Laboratory of coal mine disaster dynamics and control (2011DA105287-zd201902).

Supplementary Material

Supplementary data to this article can be found online at https://doi.org/10.1016/j.cjche.2023.03.004.

Nomenclature

bimpeller blade width,m

C1off-bottom clearance of right impeller,m

C2off-bottom clearance of left impeller,m

Drushton turbine impeller diameter,m

Ddpitched blade down-pumping rushton turbine impeller diameter,m

Dupitched blade up-pumping rushton turbine impeller diameter,m

dshaft diameter,m

eeccentric distance,m

Hfluid height,m

Kconsistency index,Pa∙sn

ksconstant of Metzner–Otto

Mtorque,N∙m

Nimpeller speed,r∙s-1

nrheological index

Ppower consumption,W

Tvessel diameter,m

tmmixing time,s

Vfluid volume,m3

wbaffle width,m

wtmass fraction

η non-Newtonian viscosity,Pa∙s

ρ density,kg∙m-3

μaapparent viscosity,Pa∙s.

τ shear stress,Pa

Subscripts

lleft

r right