Nonlinear optical rectification of GaAs/Ga1-xAlxAs quantum dots with Hulth´en plus Hellmann confining potential

Yi-Ming Duan(段一名) and Xue-Chao Li(李学超)

School of Mechanics and Optoelectronics Physics,Anhui University of Science and Technology,Huainan 232001,China

Keywords: nonlinear optical rectification,quantum dots,Hulth´en plus Hellmann potential,Nikiforov–Uvarov method

1. Introduction

Generation of laser can provide powerful conditions for uncovering the veil of nonlinear optics. High intensity and strong coherence are the characteristics of laser beams.[1–4]Moreover, generation of nonlinear optical effects requires beams with these characteristics to react with materials.When the laser was born,the theory of nonlinear optics also entered a rapid writing stage.[5,6]After that,scientists interact different laser beams with different media,and constantly find new nonlinear optical phenomena,such as optical sum frequency,optical rectification,third harmonic,and frequency doubling.[7–9]In the past ten years,new nonlinear optical effects were found,and furthermore scientists have paid more attention to development of nonlinear optical devices. For example, optical parametric oscillators are made by using three wave mixing in nonlinear optical effect. Light modulators can modulate phase and intensity of emitted light.[10,11]These optical devices mainly use the second-order and third-order nonlinear optical effects. In addition,nonlinear optical effects also have important applications in the field of information communication.

The appearance of semiconductor nano materials plays an important role in generation of new nonlinear optical phenomena. Due to the size effect of electrons in semiconductor nano materials, the changes of energy level and energy state will also be greatly affected in the process of interaction with light field.[12–14]Quantum dots (QDs) are particles composed of several atoms with a diameter of about 1–10 nm.QDs are generally presented in core-shell structure, and their cores can be composed of metal complexes such as CdSe and ZnO. The “shell” can be composed of metal complexes such as ZnS,which shields the core and forms an electrostatic potential with the core.[15–17]The physical properties of quantum dots are similar to those of basic atoms, but they are more “flexible” than atoms. It can enclose single or multiple electrons in a small space, so that the electrons are limited in the three-dimensional direction, which will make the quantum confinement effect and size effect more significant than other semiconductor materials such as quantum wires or quantum wells.[18–20]Recently, numerous research have focused on nonlinear optical properties of QDs. For instance,Prasadet al.[21]reported optical properties of disk shaped QDs with magnetic fields. Rezaeiet al.[22]examined the nonlinear optical properties on an ellipsoidal finite potential QDs. Liuet al.[23]studied nonlinear optical properties of disk-shaped QDs under a static magnetic field. Houet al.[24]investigated third-order nonlinear optical properties on black phosphorus QDs.Liuet al.[25]analyzed the nonlinear optical properties on the composite films with CdSe QDs. This paper mainly discusses the nonlinear optical rectification (NOR) of GaAs/Ga1-xAlxAs QDs under Hulth´en-plus-Hellmann(HPH)potential,which is influenced by external factors,for instance,Al-concentration and temperature. This research may provide new ideas and new methods for manufacture of new optical devices.

Our article is structured as follows: The bound state solutions of the Schr¨odinger equation are given with the Nikiforov–Uvarov (NU) method in Section 2. Then, the expression of the NOR coefficient is provided. In Section 2,we present the results of GaAs/Ga1-xAlxAs spherical quantum dots and discussions. Finally, in Section 4 we show a summary of the obtained results.

2. Model and analysis

The radial Schr¨odinger equation for a confined electron utilizes the effective mass approximation given by

whereV(r)means the limiting potential,m*(x,P,T)means the effective mass of electrons of GaAs/Ga1-xAlxAs materials affected byx,P,T.[26,27]In our paper,we takeV(r)as the HPH potential:[28–30]

whereαmeans the adjustable screening parameter,A=V2R0,B=V3R0,αis a parameter related to the dot radius.V1means the depth of Hulth´en potential,V2andV3represent the strengths of Coulomb and Yukawa confining potentials, respectively. To solve Eq. (1), we use the following Greene–Aldrich approximation:[31]

Combining Eqs.(1)–(3)yields

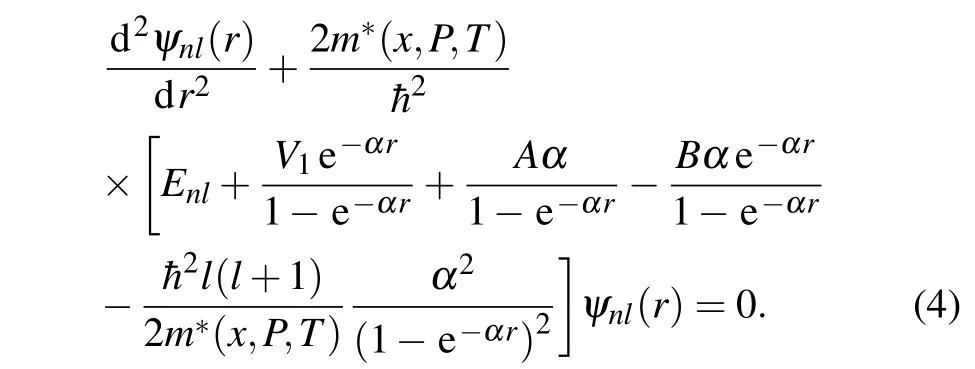
New coordinates will be introduced using the variabler →s:

Then

where

Finally, we obtain the expression of energy eigenvalues of HPH confining potential as follows:

Similarly,we obtain

where

In our work, the expression of the NOR coefficient is given as[32,33]

whereσvdenotes the electron density,Mij=|〈Ψj|rcosθ|Ψi〉|denotes the matrix element,δ01=|M11-M00|,ωij=(Ei-Ej)/¯hdenotes the transition frequency.

3. Results and discussions

In this section,the NOR coefficient in QDs with the HPH potential is explored. The parameters used in the calculations are taken as[32,33]σv=5×1024m-3,T1=0.2 ps,T2=0.14 ps,Γ1=1/T1,Γ2=1/T2,ε0=8.85×10-12F·m-1.

Fig.1. NOR coefficient susceptibility with photon energies for x=0.2,P=12 kbar,T =300 K,R0=5 nm,V2=100 meV,V3=100 meV as various values of V1.

Figure 1 shows the behavior of the NOR coefficient for different situations of the depthV1, take the fixed values ofx=0.2,P=12 kbar,T=300 K,R0=5 nm,V2=100 meV,andV3=100 meV. We can find that as the value ofV1increases i.e., the limit increases, the energy differenceE10increases, corresponding to the NOR coefficient peak moving towards the larger value of the photon energy direction, and the peak appears blue shift. Moreover,the increase ofV1will lead to the decrease ofδ01when the depth is changed from 80 meV to 120 meV.As seen in curves,when the depth value is 80 meV, the maximum of the NOR coefficient can reach 56.27×10-5m/V.When the depth increases to 100 meV,the maximum decreases distinctly to 53.16×10-5m/V.However,when the depth increases to 120 meV,the maximum decreases slightly to 49.85×10-5m/V.

As shown in Fig. 2, the variation of the NOR coefficient withR0is displayed by takingV0=100 meV,x=0.2,P=12 kbar,T=300 K,V2=100 meV,andV3=100 meV.We can obtain that the NOR coefficient shifts toward high energy direction with the strengthening ofR0. The physical reason is that,when theR0is within the limit,E10increases with increment. Specifically, the resonant peak of NOR coefficient increases asR0increases, which is owing to an increment in the matrix elementδ01.

Fig.2. NOR coefficient susceptibility with photon energies for V1 =100 meV, x = 0.2, P = 12 kbar, T = 300 K, V2 = 100 meV, and V3=100 meV with different values of R0.

Figure 3 presents a functional diagram for different Alconcentrationxon the NOR coefficient with other parameters being fixed. As seen in curves, it is clear that in the increasing process of Al-concentration from 0.1 to 0.3,the peak value slightly decreases with an increment of Alconcentration. We find that, whenxis 0.1, the value of theδ01is 6.49×10-27m3;whenxincreases to 0.2,theδ01becomes 6.37×10-27m3;whenxchanges to 0.3,the value of the density matrix increases to 6.28×10-27m3. Specifically,with increasingx,the location of NOR coefficient shifts blue,which results from the increasingE10.

Figure 4 shows the effects of (a) hydrostatic pressurePand (b) temperatureTon the NOR coefficients forx=0.2,V1=100 meV,V2=100 meV,V3=100 meV,andR0=5 nm.From Fig.4(a),as thePincreases,the NOR coefficient moves towards the high energy region,i.e.,blue shift. Moreover,the increase ofPreduces the size of the matrix element,which is owing to thePthat has the effect of reducing constraints. In Fig. 4(b), there is a red shift with increasingTand the NOR coefficient peak increases with increasingT,because the separation between energies decreases.

Fig.3. NOR coefficient susceptibility with photon energies with R0 =5 nm, P=12 kbar, T =300 K, V1 =100 meV, V2 =100 meV, and V3=100 meV for different x.

Fig.4. NOR coefficient susceptibility with photon energies with x=0.2,V1 =100 meV,V2 =100 meV,V3 =100 meV,and R0 =5 nm for different P(a)and T (b).

Figure 5 shows the coefficients of NOR for the potential strengthsV2(a)andV3(b)against ¯hω. We can conclude that the NOR coefficient peak blue shifts whenV2is reduced to 80 meV from 120 meV.Also,increasingV2results in a decrement in the peak amplitude. However, the increment ofV3in Fig.5(b)on the coefficient leads to an apparent redshift of the NOR coefficient peak and leads to an increase in the NOR coefficient.

Fig.5. NOR coefficient susceptibility with photon energies with R0 =5 nm,x=0.2,V1=100 meV,P=12 kbar,and T =300 K for different V2 (a)and V3 (b).

4. Conclusion

In summary, we have derived the expressions of wave function and energy eigenvalues by solving the Schr¨odinger equation in the framework of the NU method. We also calculate the NOR coefficient of GaAs/Ga1-xAlxAs QDs under HPH confining potential. The calculation results indicate that the peak of NOR coefficient increases with the increasingTandV3, but decreases with the increase ofV1,R0,P, andV2. In a specific Al-concentration range, the maximum value of NOR coefficient will decreases, meaning that we can obtain the required nonlinear optical properties by tuning Alconcentration. Furthermore,with the increasingTandV3,the resonance peak of NOR coefficient appears in a red shift,but with the increasingV1,R0,PandV2,the NOR coefficient appears in a blue shift. Also,the position of the peak has a blue shift as Al-concentration changes from 0.1 to 0.3. We think that the present results can play a guiding role in improving the nonlinear optical properties and optical modulation characteristics of low-dimensional semiconductor materials.

Acknowledgement

Project supported by the National Natural Science Foundation of China(Grant Nos.51702003,61775087,11674312,52174161,and 12174161).