Optimization of flow field in electrochemical trepanning of integral cascades (Ti6Al4V)

Gaopan LEI, Dong ZHU, Jiabao LI

College of Mechanical & Electrical Engineering, Nanjing University of Aeronautics and Astronautics, Nanjing 210016, China

KEYWORDS Edges electrolyte supply;Electrochemical trepanning;Flow field;Structure optimization;Surface quality

Abstract Ti6Al4V is widely applied in the integral cascades of aero engines. As an effective machining method,electrochemical trepanning(ECTr)has unique advantages in processing surface parts made of hard-to-cut materials. In ECTr, the state of the flow field has a significant effect on processing stability and machining quality.To improve the uniformity of the flow field when ECTr is applied to Ti6Al4V,two different flow modes are designed,namely full-profile electrolyte supply(FPES) and edges electrolyte supply (EES). Different from the traditional forward flow mode, the flow directions of the electrolyte in the proposed modes are controlled by inlet channels. Simulations show that the flow field under EES is more uniform than that under FPES.To further enhance the uniformity of the flow field,the structure of EES is optimized by modifying the insulating sleeve.In the optimized configuration,the longitudinal distance between the center of the inlet hole and the center of the blade is 6.0 mm,the lateral distance between the centers of the inlet holes on both sides is 16.5 mm,the length to which the electrolyte enters the machining area is 1.5 mm,and the height of the insulating sleeve is 13.5 mm.A series of ECTr experiments are performed under the two flow modes. Compared with EES, the blade machined by FPES is less accurate and has poorer surface quality,with a surface roughness(Ra)of 3.346 μm.Under the optimized EES,the machining quality is effectively enhanced, with the surface quality improved from Ra=2.621 μm to Ra=1.815 μm,thus confirming the efficacy of the proposed methods.

1. Introduction

Integral cascade structures effectively improve the performance of aero engines, as they reduce the number and weight of the parts.1Because of the harsh working environment,integral cascades are generally fabricated using dedicated materials with a high specific strength, high temperature resistance, and good corrosion resistance, such as titanium- and nickel-based alloys.2The titanium-based alloy Ti6Al4V has been widely applied in core components in aero industries.3Although Ti6Al4V has good comprehensive mechanical properties, its processing using traditional milling results in serious tool wear and high residual stress because of the low elastic modulus of titanium alloys. Moreover, it is difficult to achieve high machining efficiency because of the high cutting temperatures.4Electrochemical machining (ECM) is a noncontact method based on anode electrochemical dissolution. This has the advantages of no tool wear, no residual stress, and high processing efficiency, and is independent of the mechanical properties of the material.5Thus, ECM is suitable for manufacturing the core components in aero engines.6

As a typical application of ECM, electrochemical trepanning (ECTr) forms the blade through the relative movement between the hollow cathode sheet and the workpiece.7Because of the characteristics of ECTr,it has unique advantages in processing ruled surface parts.8Hence, ECTr has been widely applied in the manufacture of integral cascades. In ECTr, the state of the flow field has a significant influence on the stability and consistency of the machining process.9In the machining area, a lack of electrolyte may lead to instabilities in the processing state, and can even cause short-circuit phenomena.An uneven distribution of the flow field may also result in the electrolysis products being unevenly distributed, leading to unevenness in the electrical conductivity and material removal rate, thereby affecting the uniformity and consistency of the machining quality. This paper focuses on improving the flow field uniformity in the ECTr of integral cascades.

Many studies have attempted to improve the uniformity of the flow field in ECM. In ECTr, Lin et al. designed a liquidincreasing seam on the cathode tool to improve the uniformity and accessibility of the flow field.10For the cascade channels in blisk ECM, Xu et al. proposed a π-shaped flow pattern to improve the flow field stability.11In diffuser ECM, Gu et al.investigated the effect of the cathode structure on the flow field,12and Zhu et al. proposed a new flow mode in which the electrolyte can be supplied to the entire blade profile.13In terms of electrochemical cutting, Yang et al. optimized the number and spray angle of the outlets to enhance the consistency of the flow field in the machining gap.14For the application of electrochemical micromachining, Kunar and Bhattacharyya adopted a reusable masked tool to obtain a better flow state in the manufacturing of micro-square patterns.15Through flow field simulations,Lee et al.evaluated the fixtures and machining parameters used in the microscale flow channels of ECM.16In ECTr of diffuser with a special structure,Xu et al. effectively improved the uniformity of the flow field by adding a back pressure and optimizing the outletcornered gap, thereby enhancing the machining efficiency and surface quality.17Although many optimization studies of the flow field have been performed in ECM, there has been little research on the application of ECTr to integral cascades.

In this paper,to improve the uniformity and stability of the flow field in the ECTr of integral cascades, two different flow field modes for actively controlling the flow of electrolyte are designed, namely full-profile electrolyte supply (FPES) and edges electrolyte supply(EES).Through flow field simulations,the two modes are examined and analyzed. Compared with FPES, a better flow field state can be achieved under EES.To further enhance the flow uniformity under EES, the flow field structure is optimized by modifying the parameters of the insulating sleeve. A series of simulations are then conducted to obtain the optimal insulation sleeve structure.Finally, ECTr experiments using the two flow modes are carried out to verify the effectiveness of the proposed methods.

2.Optimization of flow field mode in ECTr for integral cascades

In ECM, there are generally three main flow modes: forward flow, lateral flow, and reverse flow. Compared with the other two flow modes, forward flow is easier to achieve. Hence, forward flow has been widely used in ECTr. Based on this forward flow configuration, the uniformity of the flow field is improved by designing two different flow modes for ECTr in integral cascades.

2.1. Design of flow field modes

Integral cascade components consist of a number of blades with identical cross-sections and a series of narrow channels.In the traditional forward flow for ECTr (Fig. 1), the electrolyte directly rushes to the tip of the machined blade, where it is passively diverted by the machined blades at the inlet,thereby being divided into several flow paths to the machining area. This may result in an uncontrollable amount of electrolyte being randomly supplied to various locations in the processing area. Because of the passive diversion of the electrolyte, the traditional forward flow structure of ECTr tends to produce an uneven distribution of the flow field.

Combined with the structure of the components and the characteristics of forward flow, two different flow modes that can actively control the flow direction of the electrolyte are proposed: FPES and EES.

In FPES,the electrolyte is pumped into the entire profile of the blade at the same time by controlling its flow path, as shown in Fig. 2. Under the constraints of the insulating block and the insulating sleeve, which have a similar shape to the blade, the electrolyte actively flows into the machining area through the fixed channel, thus supplying the entire contour of the blade.In this flow field mode,the narrow blade cascade channel greatly limits the width of the inlet channel, thereby affecting the supply of electrolyte to the entire profile of the blade. As the electrolyte has a certain randomness before entering the inlet channel, the electrolyte may still be unable to supply the entire profile of the blade uniformly over a certain length of the inlet channel. In addition, the thin shapes and obvious curvature changes at the leading and trailing edges restrict the accessibility of the flow field. The combined influence of these factors means that the distribution of the flow field in the processing area may still be uneven.

In EES, the electrolyte is supplied to the leading- and trailing-edge areas of the blade during the machining process,as shown in Fig.3.Under a specific insulating sleeve structure,the electrolyte is poured into the leading- and trailing-edge areas of the blade through the inlet channels of the insulating sleeve. The electrolyte then flows along the concave and convex parts of the blade.Finally,the machining area is filled with electrolyte, thereby achieving the supply of electrolyte to the entire profile of the blade in ECTr. Because the electrolyte is directly supplied to the leading-and trailing-edge areas,which are most prone to a lack of electrolyte, the accessibility of the flow field is effectively improved. Hence, EES may produce a better flow field state than the traditional forward flow and the proposed FPES.

Compared with the traditional forward flow of ECTr, the two proposed flow modes effectively prevent the electrolyte from directly rushing to the machined blade at the inlet. The electrolyte enters the machining area directly through preset channels. The overall profile of the blade being processed can be supplied under different flow paths, thereby improving the uniformity of the flow field in the machining area.

2.2. Simulation of different flow field modes

To examine the two flow modes in the integral cascade of ECTr, a series of flow field simulations were performed. First,the mathematical model of the flow field in ECTr was established.Based on the two proposed flow modes,geometric models of the simulations were then established.Finally,under the same simulation parameters, the flow field simulations were carried out.

2.2.1. Establishment of flow field simulations

In ECTr,when the processing is in a relatively stable state,the machining gap at the front face between the cathode sheet and the workpiece remains basically unchanged. This so-called equilibrium gap can be calculated by the following equation18:

where Δbis the equilibrium gap, ηω is the actual electrochemical volume equivalent,κ is the conductivity of the electrolyte,U is the applied voltage,δE is the polarization potential of the anode material, and v is the feed rate of the cathode.

According to the above equation, through relevant preset experimental parameters and material characteristic parameters, it can be concluded that Δbis approximately 0.25 mm.

The flow state of the electrolyte in ECTr can be determined by the Reynolds number. When the Reynolds number is greater than 2300,the flow field is in a turbulent state.Thus,to ensure a better flow state, the flow rate should satisfy the following equations19:

where Re is the Reynolds number,u is the flow rate of the electrolyte, ν is the dynamic viscosity coefficient of the electrolyte,and Dhis the hydraulic diameter.

To simplify the flow field models, the following assumptions are made. (1) The electrolyte is an incompressible fluid.(2) The gas bubbles and Joule heat generated during processing can be neglected. (3) There is no slippage on the wall.Based on these assumptions, the following Navier-Stokes equations are adopted to describe the movement of the electrolyte in ECTr:20

where xiand xjare the coordinate values in the i and j axis directions, respectively, P-is the time-averaged pressure, ui- is the time-averaged velocity component in the i direction, and τijis the component of the stress tensor in the plane.

Estimations of the electrolyte flow state indicate that the flow field is turbulent. The standard k-ε model is suitable for solving the turbulence problem,and is therefore adopted here.The standard k-ε model can be described by the following equations:21

where μ is the viscosity coefficient,ε is the turbulent dissipation rate, ρ is the density of the electrolyte, k is the turbulent flow energy of the fluid, and t is the time. The term Gkproduced by turbulent flow and the turbulent viscosity μtcan be calculated according to the following formulas:22

Table 1 Simulation parameters under different flow modes.

According to the two designed flow modes,geometric models of the flow field simulations were established (Fig. 4), in which the cloud cross-sections are located in the middle of the equilibrium gap.The boundary conditions listed in Table 1 are consistent under the two modes.

2.2.2. Results of flow field simulations Simulations were conducted to obtain the flow field distributions on the cloud cross-sections (see Figs. 5 and 6). To judge the state of the flow field, the average velocity (AV), variance of the velocity (VV), and number of low-velocity points(LVPN) were adopted. To analyze these indicators, 10,000 data points uniformly distributed in the flow velocity distribution cloud map were extracted.

For the determination of low flow velocity,the normal flow rate was considered when the flow state was turbulent.According to Eq.(2)and the geometric models of the flow field above,the flow velocity should be greater than about 5.1 m/s. Therefore, flow velocities of less than 5.1 m/s were considered to indicate low-velocity points. The evaluation index values obtained under the different flow modes are presented in Table 2.

Table 2 Evaluation index values under different flow modes.

Some important information can be obtained from the simulation results. (1) Compared with FPES, the pressure distribution is more even under EES. In Fig. 5(a), under FPES,obvious pressure stratification occurs in the convex and concave parts, and the pressure in the concave part is higher than that in the convex part.In Fig.6(a),the pressure stratification in the entire area is significantly improved. (2) AV is higher and VV is lower under EES than under FPES.This shows that EES renews the electrolyte faster and produces a more uniform flow field. (3) Comparing the velocity distribution cloud diagrams of the two structures, there are larger low-velocity regions and more obvious flow rate stratification under FPES.The LVPN value is smaller under EES,indicating a better flow field state.(4)The flow velocity vector maps reflect the trend of the electrolyte flow. In FPES, less electrolyte flows into the areas on both sides of the blade and the electrolyte preferentially flows to the concave part with lower pressure. In EES,although these issues have improved to some extent, there are still certain uneven areas.

According to the simulation results,the uniformity and stability of the flow field is effectively improved under EES compared with FPES.

3. Optimization of flow field structure under EES

The EES flow mode produces better flow field uniformity than FPES. However, under EES, the distribution of the flow field is still uneven.Therefore,to further enhance the consistency of the flow field distributions in the concave and convex parts,the EES structure was optimized.

3.1. Optimization strategy of flow field structure

In EES,the supply path of the electrolyte is determined by the structure of the insulating sleeve, as shown in Fig. 3.Thus, by optimizing the structure of the insulating sleeve, the EES flow field structure can be optimized.

In the insulating sleeve of EES, the inlet channels directly determine the position at which the electrolyte enters the machining area. The electrolyte flows along the contour of the machined blade, thus filling the entire machining area.Under different insulating sleeve structures, the position at which the electrolyte initially enters the machining area varies,resulting in a certain difference in the flow path of the electrolyte along the machining blade.To obtain the optimal position for the electrolyte to enter the machining area, that is, to obtain the optimal insulating sleeve structure, the structural parameters of the insulating sleeve in EES were optimized.

According to the structure of the insulating sleeve, the structural parameters that affect the position at which the electrolyte enters the machining area were selected for optimization. These parameters are the longitudinal distance (Y)between the center of the inlet hole and the center of the blade(O), the lateral distance (X) between the centers of the inlet holes on both sides, the length to which the electrolyte enters the machining area (L), and the sleeve height (H), as shown in Fig. 3.

In the insulating sleeve,the position at which the electrolyte enters the processing area and the length of the flow path by which the electrolyte enters the processing area are directly determined by Y and X. The unit area of electrolyte entering the machining area is determined by L.The length of the electrolyte reaching the entrance of the processing area and the volume of electrolyte that can be contained in the insulating sleeve are influenced by H. The influence of these parameters on the change of the flow field structure is illustrated in Fig.7.

3.2. Simulations using different insulating sleeves

According to the optimization strategy, flow field simulations were conducted with different values of the structural parameters of the insulating sleeve. The other simulation parameters for each different structure were as listed in Table 1.

3.2.1. Optimization of longitudinal distance

First, the longitudinal distance (Y) between the center of the inlet hole and the center of the blade was optimized.Changing Y in the insulating sleeve structure affects the point at which the electrolyte enters the processing area. Y was successively set to 2.5 mm, 3.5 mm, 4.5 mm, 5.5 mm, 6.5 mm, and 7.5 mm, and the distribution of flow velocity for each value was obtained, as shown in Fig. 8.

Using the flow velocity distribution cloud maps, 10,000 data points were extracted for analysis. VV and LVPN under the different Y values are shown in Fig. 9.

From Figs. 8 and 9, Y values of 4.5 mm, 5.5 mm, and 6.5 mm give VV values of 80.13 m2/s2, 75.5 m2/s2, and 78.0 m2/s2and LVPN values of 691,704,and 703,respectively.As Y varies from 4.5-6.5 mm,the uniformity of the entire flow field is relatively good, and the area of low flow velocity remains quite small.Therefore,the Y value was further refined in this interval. The flow field distributions for Y = 5.0 mm and 6.0 mm are shown in Fig. 10.

The VV and LVPN values are 72.8 m2/s2and 656(Y = 5.0 mm) and 72.5 m2/s2and 641 (Y = 6.0 mm). The smaller low-velocity area and most uniform distribution of the entire flow field occur when Y = 6.0 mm. The AV value is also higher (up to 21.7 m/s), and the flow rate stratification in the concave and convex parts is effectively improved.Thus,Y=6.0 mm was adopted as the longitudinal distance between the center of the inlet hole and the center of the blade.

3.2.2. Optimization of lateral distance

Based on the above optimization results, the lateral distance(X) between the centers of the inlet holes on both sides was optimized under a longitudinal distance of 6.0 mm.

According to the structure of the integral cascade,the value of X was successively set to 15.0 mm, 15.5 mm, 16.0 mm,16.5 mm,and 17.0 mm and the corresponding geometric models were established. Flow simulations were then performed.

The flow field distributions under the different X values are shown in Fig. 11. The case X = 16.5 mm gives the lowest VV and LVPN values(68.7 m2/s2and 615,respectively);this value of X also produces the optimal flow rate uniformity, with an AV of 21.4 m/s.Therefore,16.5 mm was used as the lateral distance between the centers of the inlet holes on both sides of the sleeve.

3.2.3. Optimization of length to which the electrolyte enters the machining area

Next, the length to which the electrolyte enters the machining area(L)was optimized. The value of L was successively set to 0.5 mm, 1.0 mm, 1.5 mm, 2.0 mm, 2.5 mm, 3.0 mm, and 3.5 mm, and the flow field simulation results are shown in Fig. 12.

When L=1.0 mm,VV reaches its minimum of 58.6 m2/s2.However, the corresponding LVPN is 686 and the AV was only 18.2 m/s. The minimum LVPN is 641 for L = 1.5 mm,which has corresponding VV and Av values of 60.3 m2/s2and 21.7 m/s, respectively.

LVPN characterizes the area of the low-velocity zone,which affects the stability of processing. Larger values of AV are associated with faster electrolyte renewal speeds, which make the processing more stable. Compared with the case L = 1.0 mm, using L = 1.5 mm gives a much lower LVPN and a higher AV,albeit with a small increase in VV.Therefore,under these considerations, the optimal value of L was determined to be 1.5 mm.

3.2.4. Optimization of height of the insulating sleeve

Finally, the height of the insulating sleeve (H) was optimized.According to the structure of the insulating sleeve,the value of H should be greater than the length of the blade (9 mm) to ensure normal processing. Thus, the value of H was successively set to 15 mm, 20 mm, 25 mm, 30 mm, and 35 mm,and the flow field distributions were simulated, as shown in Fig. 13.

Smaller values of H produce a more uniform distribution of the flow field.When H=15 mm,VV and LVPN are 73.5 m2/s2and 673, respectively. According to actual processing experience,in a forward flow structure,the height of the inner cavity is usually set to be at least 1.5 times the height of the blade,so that the machined products in the tip area can be discharged smoothly and the processing remains stable. This suggests a minimum H value of 13.5 mm. Thus, a flow field simulation was performed for H = 13.5 mm, giving VV = 72.5 m2/s2and LVPN = 641. Therefore, a value of H = 13.5 mm was adopted for the optimized sleeve structure.

Through this analysis of the structural parameters,the optimized insulating sleeve was obtained. The longitudinal distance between the center of the inlet hole and the center of the blade was set to 6.0 mm, the lateral distance between the centers of the inlet holes on both sides was set to 16.5 mm,the length to which the electrolyte enters the machining area was set to 1.5 mm, and the height of the insulating sleeve was set to 13.5 mm.This optimized structure improves the uniformity of the flow field.

4. Experiments and results

To verify the simulation results presented in the previous section, a series of ECTr experiments were performed under the two proposed flow modes. The machining accuracy and surface quality of the machined blades were measured and compared.

4.1. Establishment of experiments

According to the abovementioned characteristics of the flow modes, the corresponding insulation sleeves and combined cathodes were designed and fabricated. To carry out ECTr experiments, machining devices were built (Fig. 14). A pure electrolyte with a constant pressure was poured into different combined cathodes through the inlet pump to realize changes in the electrolyte flow structure. The combined cathode was mounted on the spindle of the machine tool and the workpiece was placed on the worktable. Through motion in the Y direction of the machining tool, relative movement between the cathode and the workpiece can be realized,thereby controlling the machining depth. The experimental parameters remained the same for both flow modes, as listed in Table 3.

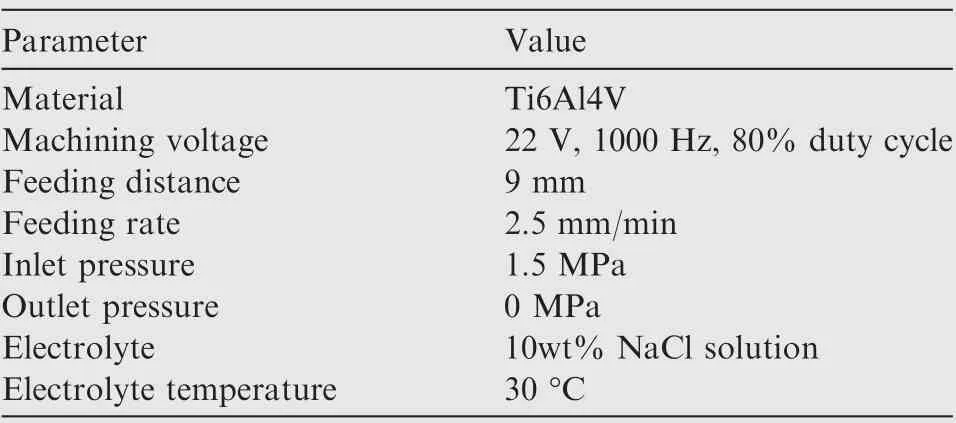
Table 3 Experimental parameters of ECTr.

4.2. Experimental results

Through ECTr experiments, machined blades were obtained under FPES, EES, and the optimized EES, as shown in Fig. 15. By performing Form Talysurf i-Series 5 surface measurements, the surface roughness values under the two flow modes were determined, as shown in Fig. 16. Area D in Fig. 15 is located about 1.5 mm from the root of the leaf,approximately the same position as the evaluation section used in the simulations described in Section 3.Area E is located on the leading and trailing edges of the top of the machined blade.Area T is the tip region,and area M denotes the local concave and convex parts.

From the experimental results,some important information can be obtained. (1) In Fig. 15(a), because of the poorer flow field state, the blade machined by FPES exhibits serious stray corrosion in area E, resulting in poor forming accuracy at the leading and trailing edges. In Fig. 15(b) and (c), the machined blade has a complete profile and does not present any obvious tapering in area E.Thus,under EES,the forming accuracy has effectively improved.(2)Severe surface pitting occurred on the blade surface machined by FPES,as shown in area T of Fig.15(a). Under EES, there is less surface pitting on the machined blades. (3) Under EES, there is some delamination on the machined surface and a relatively poor surface quality in area M,which may be caused by the uneven distribution of the flow field due to the presence of a low-velocity zone in the convex and concave parts.Under optimized EES,the machining quality of the concave and convex parts is consistent with that in other areas of the machined blade. (4) Compared with FPES,the surface roughness (Ra) under EES has improved from Ra= 5.346 μm to Ra= 2.621 μm in area D. Using the optimized insulating sleeve, the surface roughness can be further improved to Ra= 1.815 μm.

Combining the experimental and simulation results, it is clear that EES produces a better flow field in the machining area, the machined area suffers less stray corrosion, and the forming accuracy and surface quality are superior to those under FPES.In addition,using the optimized insulating sleeve,the uniformity of the flow field can be further improved, leading to greater consistency of the overall surface quality of the machined blade.These results verify the simulation results presented in Section 3.

5. Conclusion

For integral cascades formed from Ti6Al4V, the flow field in ECTr was optimized based on the forward flow mode.Through this optimization, the uniformity and stability of the flow field was effectively improved. Our conclusions can be summarized as follows:

(1) Two electrolyte flow modes, FPES and EES, were designed for the application of ECTr to integral cascades.The two flow modes effectively prevent the electrolyte rushing directly to the machined blade at the inlet. The electrolyte enters the machining area directly through preset channels,thereby improving the uniformity of the flow field in the machining area.

(2) Flow field simulations were performed using FPES and EES to examine the difference between the two modes. Compared with FPES,the flow field distribution was more uniform under EES. To further improve the uniformity under the EES mode, the flow field structure was optimized by modifying the insulating sleeve. Simulations were conducted to optimize the insulating sleeve, and it was found that the longitudinal distance between the center of the inlet hole and the center of the blade should be 6.0 mm, the lateral distance between the centers of the inlet holes on both sides should be 16.5 mm,the length to which the electrolyte enters the machining area should be 1.5 mm, and the height of the insulating sleeve should be 13.5 mm.

(3) ECTr experiments were performed under the two proposed flow modes. Compared with the blade machined by FPES, that produced by EES presented better forming accuracy, and the surface quality improved from Ra= 5.346 μm to Ra= 2.621 μm. Using the optimized EES, the surface roughness of the machined blade further improved to Ra= 1.815 μm and the consistency of the blade surface was further enhanced. The experimental and simulation results demonstrate the efficacy of the proposed methods for optimizing the flow state.

Declaration of Competing Interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgements

This work was supported by the National Nature Science Foundation of China (No.91860135) and the Fundamental Research Funds for the Central Universities (NE 2017003).