Classifying Electrolyte Solutions by Comparing Charge and Mass Transport

Bernhard Roling*,Janosch Kettner,and Vanessa Miß

The conventional classification of electrolyte solutions as “strong”or “weak”accounts for their charge transport properties,but neglects their mass transport properties,and is not readily applicable to highly concentrated solutions.Here,we use the Onsager transport formalism in combination with linear response theory to attain a more general classification taking into account both charge and mass transport properties.To this end,we define a molar mass transport coefficient Λmass,which is related to equilibrium center-of-mass fluctuations of the mobile ions and which is the masstransport analogue of the molar ionic conductivity Λcharge.Three classes of electrolyte solution are then distinguished:(i) “Strong electrolytes”with 4Λmass≈Λcharge;(ii)“weak charge transport electrolytes”with Λcharge≪4Λmass;and(iii)“weak mass transport electrolytes”with 4Λmass≪Λcharge.While classes(i)and(ii)encompass the classical“strong”and “weak”electrolytes,respectively,many highly concentrated electrolytes fall into class(iii)and thus exhibit transport properties clearly distinct from classical strong and weak electrolytes.

Keywords

charge and mass transport,superconcentrated electrolytes,energy storage,Ion correlations

Electrolyte solutions play a very important role in many scientific fields,such as electrochemistry,energy research,chemical synthesis,biochemistry,and pharmaceutical research.[1-10]The conventional classification of electrolyte solutions as“strong”or“weak” is based on the dissociation equilibriumHere,AX（solv）denotes solvated ion pairs,whiledenote solvated free ions contributing to the ionic conductivity of the solution.The degree of dissociation α =cA+／（cA+ +cAX）=cX-／（ cX- +cAX）is large(α≈1）in the case of strong electrolytes and small(α≪1）in the case of weak electrolytes.The molar ionic conductivity of an electrolyte solution,Λcharge= σion／csalt,with σionand csalt=cA++cAXdenoting the ionic conductivity and the overall salt concentration,respectively,increases with increasing degree of dissociation α.Thus,weak electrolytes,such as aqueous solutions of acetic acid,exhibit much lower molar ionic conductivities than strong electrolytes,such as aqueous KCl solutions.On the other hand,the mass transport properties of strong and weak electrolytes are similar,since free ions and solvated ion pairs exhibit typically similar self-diffusion coefficients and thus contribute both to the mass transport.For instance,the chemical diffusion coefficient of an acetic acid solution depends very weakly on the acetic acid concentration and thus on the degree of dissociation.When the acetic acid concentration is increased from 1.8 mMto 97.1 mM,the diffusion coefficient decreases by＜4% .[11]

It is important to emphasize that for electrochemical applications of electrolyte solutions,both charge and mass transport properties are highly relevant.As an example,in alkali-ion batteries,the transport of alkali ions between the electrodes takes place via a combination of electric field-induced migration and salt concentration gradient-induced diffusion.[12,13]Consequently,an electrolyte classification taking into account both charge and mass transport properties is highly desirable.

Currently,there is huge interest in using highly concentrated electrolytes for electrochemical energy storage in batteries and supercapacitors.[14-17]In highly concentrated electrolytes,a large part or even a major part of the solvent molecules is involved in the solvation of the ions.This results in a low chemical potential of the solvent molecules,leading to a low vapor pressure and to a broad electrochemical stability window of the electrolyte solution.An interesting example are water-in-salt electrolytes,[18]which exhibit a much broader electrochemical stability window than diluted aqueous solutions and thus pave the way for the development of aqueous Li-ion batteries.[19]Although several important models for the transport properties of concentrated electrolytes were published over the last decades,[20-24]there are still controversial viewpoints in the literature as to whether highly concentrated electrolytes exhibit a low degree of dissociation(“low ionicity”)and can thus be classified as“weak”or“diluted” electrolytes.[25-30]

In this Outlook,we show that a more general classification of binary electrolytes(one salt,one solvent),encompassing also highly concentrated solutions,can be achieved by taking into account both charge and mass transport properties.We use the Onsager transport formalism in combination with linear response theory to define a molar mass transport coefficient,Λmass,which is the mass transport analog of the molar ionic conductivity,Λcharge.We show that there is a modelindependent relation between Λmassand equilibrium center-of-mass fluctuations caused by the ion movements,while it is well known that Λionis related to equilibrium dipole fluctuations caused by the ion movements.By analyzing the relations between Λmassand Λcharge,we show that three classes of electrolyte solutions can be distinguished.

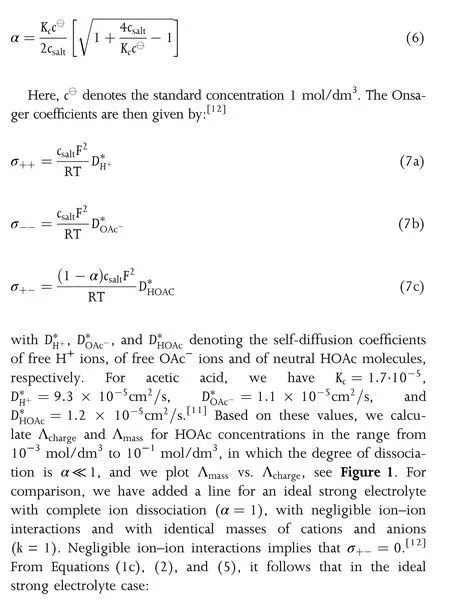
Figure 1.Plot of the molar mass transport coefficient Λmassvs.the molar ionic conductivity Λchargefor a number of 1-1 electrolyte solutions.The green line refers to the transport properties of an ideal strong electrolyte(no ion-ion interactions)with identical masses of cations and anions.The numbers added to some data points refer to the salt concentration in the respective solutions.

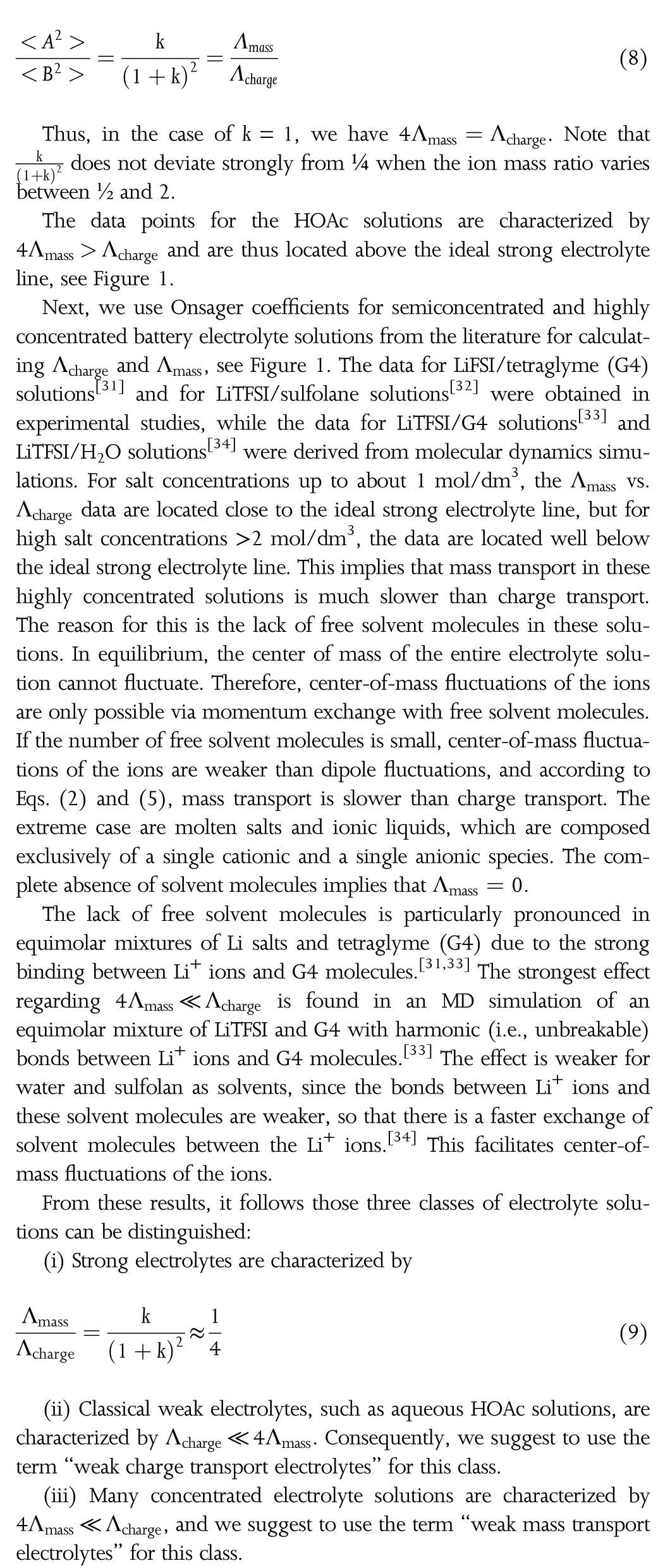
In order to support this classification of electrolyte solutions,the typical Walden plot of Λchargevs.reciprocal viscosity η-1can be complemented by a plot of 4Λmassvs.η-1.In Figure 2,such Walden plots are shown exemplarily for two solutions,for which viscosity data are available,namely for HOAc/H2O solutions[35]and for LiTFSI/H2O solutions.[34]The data show clearly that these solutions belong to different classes.The Λchargevs.η-1data of the HOAc/H2O solutions are located well below the ideal KCl line,while the 4Λmassvs.η-1data are located close to the ideal line(even slightly above the ideal line).The data of the LiTFSI/H2O solutions show the opposite trend,i.e.,the Λchargevs.η-1data are located close to the ideal KCl line,while the 4Λmassvs.η-1data at high concentrations are located well below the ideal line.

Figure 2.Walden plot of the molar mass transport coefficient Λmassand of the molar ionic conductivity Λcharge,respectively,versus the reciprocal viscosity of the electrolyte solution.The numbers added to some data points refer to the salt concentration in the respective solutions.

We note that Walden plots have also been used for analyzing the properties of“superionic”electrolytes with Λchargevalues far above the ideal KCl line,implying that charge transport is much faster than expected from the viscosity of the liquid,see for instance the work by Angell and coworkers[36]and by Sokolov and coworkers.[37]The classification proposed here can be extended to such electrolytes by generalizing the equations to polycations and/or to polyanions ions with higher charge number and masses.An example is a supercooled alkali silicate melt,in which monovalent alkali ions are transported in a polymerized silicate anion matrix.In this case,momentum conservation leads to much slower transport of the heavy polyanions,which then govern the viscosity,while the faster transport of the light cations governs the charge transport.This generalization will be the subject of future work.

In conclusion,we have shown that the conventional classification of electrolyte solutions in terms of“strong”and “weak”does not account for their mass transport properties and is therefore not readily applicable to highly concentrated electrolyte solutions.The theoretical background and the data analysis presented in this paper demonstrates that three classes of electrolyte solutions should be distinguished,namely 1)“strong electrolytes”with similar values of Λchargeand 4Λmass,2)“weak charge transport electrolytes”with Λcharge≪4Λmass,and 3)“weak mass transport electrolytes” with 4Λmass≪ Λcharge.The distinction between these classes is essential for a comprehensive understanding of the transport properties of electrolyte solutions in electrochemical devices.

We note that weak mass transport electrolytes with very low values for Λmassare disadvantageous for electrochemical cells,in which neutral salt diffusion plays an important role.Examples are:1)During cycling of alkali-ion batteries,salt concentration gradients in the electrolyte are formed,leading to a blocking of the anions.Slow neutral salt transport slows down Li+ion transport during cycling,2)In dual-ion batteries[38]with two ionic species being transported into opposite direction,salt concentration gradients are formed in order to speed up the transport of the less mobile species and to slow down the transport of the more mobile species.In this case,slow neutral salt transport slows down the transport of both species,and 3)Weak mass transport electrolytes in salt bridges lead to slow ion exchange between the half cells.

Acknowledgement

We would like to thank the Federal State of Hessen(Germany)for financial support of this work.

Conflict of Interest

The authors declare no conflict of interest.

Supporting Information

Supporting Information is available from the Wiley Online Library or from the author.