Residual stress and radial thermal deformation during hard turning bearing ring

Lan-ying XU，Qiang WU，Bao-lan HE，Peng ZHAO

（Guangdong Polytechnic Normal University，Guangzhou 510635，China）

Abstract：Thermal deformation is affected by the shape，material and surface residual stress of the machined parts.Based on the principle of elasticmechanics and thermodynamics，this paper establishes the thermal deformation model of the bearing ring based on the residual stress and quantitatively calculates the radial deformation produced by the residual stress.The thermal deformation error is less than 3.8%；the results and the experimental data are in good agreementwith themathematicalmodel.This study sets important guidance for precisionmachining of the joint bearings for robots and the bearings for wind power equipment.

Key words：Bearing，Residual stress，Thermal deformation

1 Introduction

Bearing is the key part ofmachinery，but also the weak link ofmachinery；its failure is one of the main faults of machine.Study on the surface quality of bearing has become a research focus for experts and scholars in the field of cutting processing［1-2］.The surface quality of the bearing ring exerts a significant influence on normal running of the whole bearing under complicated conditions［3-4］.Roughness，hardness and residual stress affect the normal service life and durability of bearings［5-7］.

Surface residual stress has a great influence on the performance such as reliability and stability of machine parts.Theoretical research and practical application results indicate that it can improve the fatigue strength and corrosion resistance of parts by controlling or adjusting the finished surface stress state［8］.Many scholars have been studying the bearing processing quality.Recently，Auezhan et al.adapt special heat treatment and a super polishing process to improve the rolling contact fatigue（RCF）strength of bearings［9］.Umbrello establishes a hybrid finite element method-artificial neural network approach for predicting residual stresses［10］.Atsushi et al.adopt light press of sheetmetal edge for reducing residual stress generated by laser cutting considering mechanical properties and intensity of residual stress［11］.Arshpreet et al.investigate on surface residual stress distribution in deformation machining process for aluminum alloy Arshpreet［12］.Saurabh et al.analyze modeling of residual stresses in orthogonalmachining of AISI4340 Steel［13］.Xu et al.study the surface quality of bearing rings after heat cutting［14］.Those scholars all analyzed the residual stress and its effect on surface quality.Based on those researches，this paper studies the effect of surface residual stress on thermal deformation，and establishesmathematicmodel of thermal deformation，to deduce a more accurate calculationmethod of the thermal deformation.

2 Residual stress and rad ial therm al deform ation m odel

In order to calculate thermal deformation caused by residual stress，it should be to determine the residual stress distribution first.At present，calculating residual stress accurately ismore difficult in theory，because the factors influencing the distribution of residual stress is toomuch，In this paper，supposing both ends of the bearing is free，temperature field is on theZaxis of symmetry and distributes along the radial direction，the value of temperature is only related to the radial size of ring and has nothing to dowith the position of theZaxis.To establish cylindrical coordinates of bearing（shown in Fig.1），we assume that axial displacement of any point（signedA）within bearing is zerofor the sake of calculation，and analyze the relationship between radial displacement and temperature of pointA；when the temperature changes，the radial displacement of pointAis determined，then the thermal deformation information of the bearing ring can be obtained.

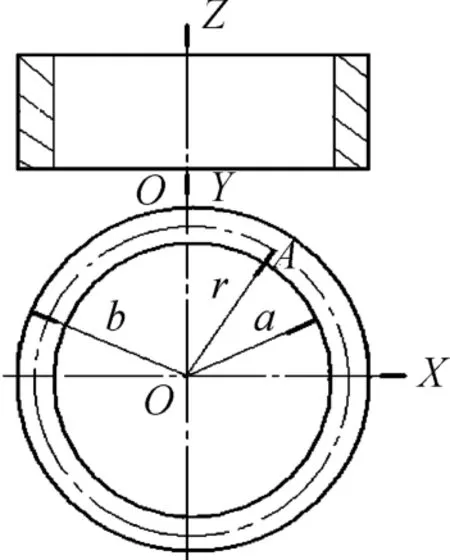
Fig.1 Cy Iind rica Icoo rd inates of bearing ring

Owing to temperature is on theZaxis of symmetry，the shear stress and shear strain are all zero.According to the related literature［15］，we can get the solution of the plane strain for the cylinder，which is shown as follows：

WhereEis the elasticmodulus of bearingmaterials；λ is the linear expansion coefficient of bearing materials；μis poisson’s ratio of bearingmaterials；tis the changing rule of the temperature，t=t（r）；σris radial stress of the bearing ring；σθis tangential stress of the bearing ring；σzis axial stress of the bearing ring；u0is the radial displacementof the bearing ring；CandDare the integral constants；they are determined by the boundary conditions.

For cylindrical parts，surface residual stress theoretically should be divided in three directions；they can be expressed as the tangential stressσθ，axial stress σzand radial stressσr［16］.In general，when the cylinder wall is thin，the radial residual stressσris very small，and in this case the tangential residual stressσθis themain part.As a result，when adopting the boundary condition to determine the integral constantCandDin this paper，we could only consider the effect of tangential residual stressσθin order to simplify the calculation.

Assuming that tangential stresses of the bearing inner ring and bearing outer ring areσθaandσθbrespectively，take them in the equation（1）above，and get the integral constant values ofCandD，then substitute them in the equation（2）above to get the value ofμ0.

In order to meet the conditions of the free end，based on the above solution，we apply a pair of uniform axial forceσzon both ends of cylinder surface to make whole axial force of the end be zero；at this time，due to the influence of axial stressσz，radial displacement need to add a termin right end of equation（3），that is

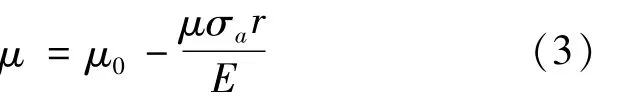
The rule of material’s elastic modulus changing with temperature can be expressed as follows：

WhereE0ismaterial’s elasticmoduluswhen temperature ist0，taket0=0；λEis the temperature coefficient ofmaterial’s elasticmodulus，it is far less than 1，and it often is negative value.

Assuming that temperature of bearing ring ist（a），while temperature of bearing outer ring ist（b），the di-ameter of inner ring isa，while the diameter of outside ring isb；then the temperature distribution inside the bearing is expressedln（r/a），which is taken into the equation（4）to acquire the value ofE.

The residual stress of surface layer is the local stress.It only effects local deformation of the surface layer，therefore，when calculating the deformation of the inner surface，it does not need to consider the influence of the outer surface residual stressσθb.Similarly，when calculating the deformation of the outer surface，it does not need to consider the influence of the inner surface residual stressσθa.Take equation（3）and（4）into equation（2）to acquire calculation mathematical model of thermal deformation of axial parts taking into account residual stress，they are as follows：

Equation（5）and equation（6）show that the bearing radial deformation due to temperature change consists of two parts，the first part is produced by the surface residual stress，namely it is the first term on the right side of equation above，and it is relativewith the size and direction of residual stress and bearing diameter；the second part is generated by the thermal expansion of the material，it includes the second item and the third item on the right side of equation above.From the analysis above，because of the influence of the surface residual stress，we can understand that the actual thermal deformation of bearing ring is not equal to deformation only generated by the thermal expansion.

3 Experim ental verification

3.1 Measuring residual stress

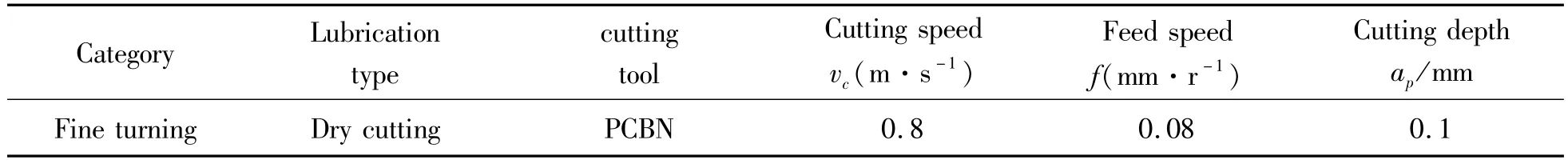
The parameters of bearing ring are as follows：r=85 mm，R=117.4 mm，B=41 mm，the material of bearing ring is Gr15 steel.Its elastic modulus is 210 GPa，poisson’s ratio is 0.3，the density is 7 800 kg/m3.The chemical component and proportion of Gr15 steel are shown in Table 1.This experiment adopts the numerical control lathe and PCBN tool，after tool is adjusted to the appropriate location，themachine feed automatically.Cutting parameters are shown in Table 2.

Tab Ie 1 Chem ica Icom ponent and p roportion of Gr15 stee I/%

Tab Ie 2 Cutting param eters

Wemeasure residual stress on the bearing ring surface throughX-ray diffractometer type of ADVANCE（shown in Fig.2）.From each 90 degree along the circumference of the bearing surfacewe select four different points to measure the residual stress and take their average value as the calculated value for later use.Among these measurement results（shown in Table 3），the positive value represents residual tensile stress，and the negative value represents residual compressive stress.It can be seen from themeasured data that residual stress values are basically negativewhich express compressive stresses，and its value is about 200 MPa.

Fig.2 Residua Istress test

Tab Ie 3 Average residua Istress ofm achined surface

3.2 Measuring radial thermal deformation caused by residual stress

Infrared thermometer can be used to collect the temperature data in real-time during and after the turning bearing rings，themeasurement of thermal deformation is also carried out at the same time as the temperature measurement.The thermal deformation produced by residual stress during processing bearing ring can be measured by Dial indicator（shown in Fig.3）.In the measuring process，the bearing ring rotates slowly，the dial indicatormeasuring headmoves linearly along the axis direction，and passes through thewhole outer ring and inner ring surface.Then，write down maximum and minimum reading of the dial indicator pointer and take the difference between the two readings ofmeasured elements as radial total run-out tolerances.The measured values and the calculated values of thermal deformation calculated by the above formula（6）are shown in Table 4.

Fig.3 Measurem ents of radiaI therm a Ideformation

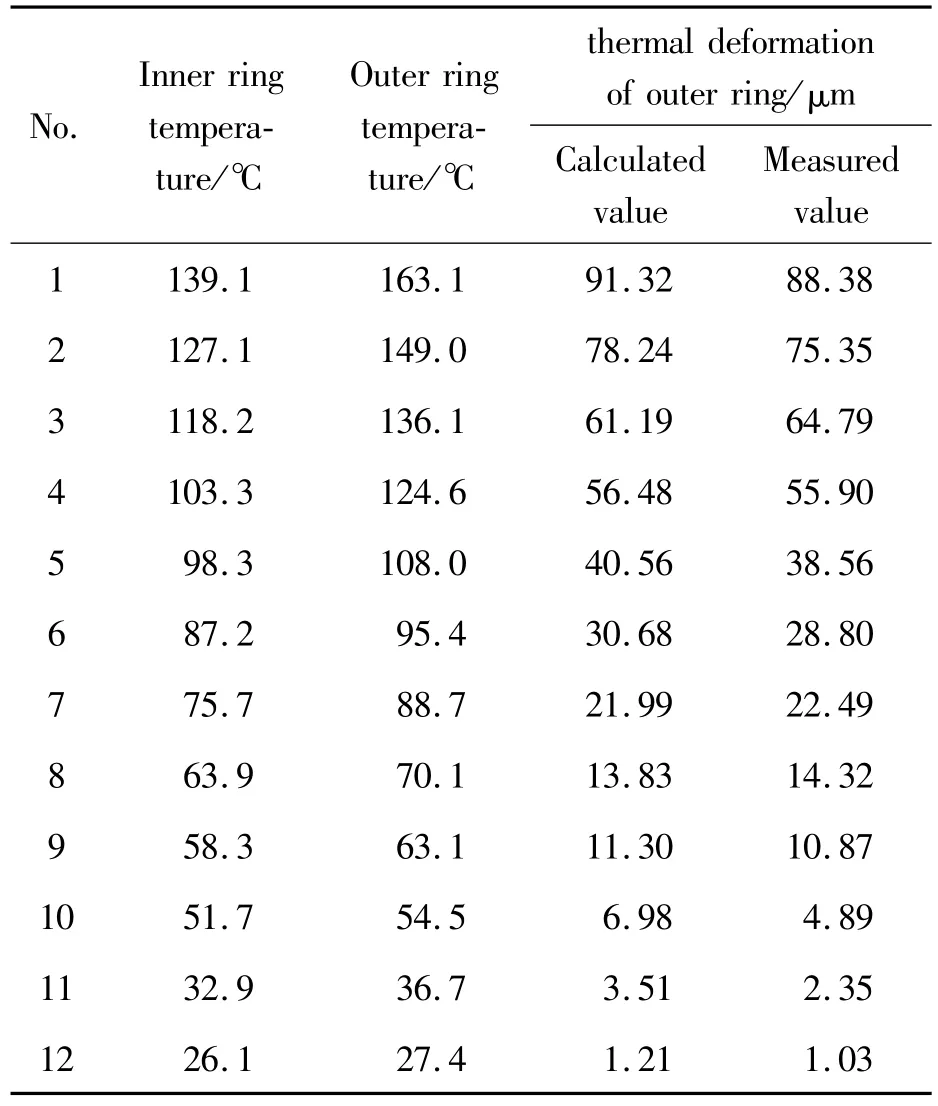
Tab Ie 4 The therm aI deformation and tem perature of bearing ring

By comparing thermal deformationmeasurement value of the bearing ring and the numerical calculation value derived from the above formula（6），it can be seen that both results approximately equal，which shows that themathematicalmodel of radial deformation caused by residual stress is correct.

4 Summary and p rospects

Through the analysis above，precision measurement result of thermal deformation for themechanical parts not only depends on partsmaterial thermal expansion but also depends on its internal residual stress.This paper deduces the relationship among radial thermal deformation and material thermal expansion and surface residual stress of bearing ring parts，then obtains the approximate equation calculating the radial thermal deformation.Themaximum error of this formula is 3.8%.The calculation formula is of great significance for precisemeasurement of the radial thermal deformation.Further study should use amechanicalmethod or other thermalmethods on adjusting machined surface residual stress to control the radial deformation quantitatively and accurately.The research results will be widely used in precisionmachining and quality control of robot bearings and wind power bearings.