Determination and correlation solubility of m-phenylenediamine in(methanol,ethanol,acetonitrile and water)and their binary solvents from 278.15 K to 313.15 K☆

Pengbao Lian,Huipeng Zhao,Jianlong Wang,Lizhen Chen,*,Yong Xiang,Qinghua Ren

1 School of Chemical Engineering and Technology,North University of China,Taiyuan 030051,China

2 Sichuan North Hongguang Special Chemical Co.,Ltd.,Yibin 644100,China

Keywords:m-Phenylenediamine Solubility Solid-liquid equilibrium Jouyban-Acree model Thermodynamic property

ABSTRACT In this study,the solubility of m-phenylenediamine in four pure solvents(methanol,ethanol,acetonitrile and water)and three binary solvent(methanol+water),(ethanol+water)and(acetonitrile+water)systems were determined in the temperature ranging from 278.15 K to 313.15 K by using the gravimetric method under atmospheric pressure.In the temperature range of 278.15 K to 313.15 K,the mole fraction solubility values of m-phenylenediamine in water, methanol, ethanol, and acetonitrile are 0.0093-0.1533, 0.1668-0.5589,0.1072-0.5356, and 0.1717-0.6438, respectively. At constant temperature and solvent composition, the mole fraction solubility of o-phenylenediamine in four pure solvents was increased as the following order:water ＜ethanol ＜methanol ＜acetonitrile;and in the three binary solvent mixtures could be ranked as follows:(ethanol+water)＜(methanol+water)＜(acetonitrile+water).The relationship between the experimental temperature and the solubility of m-phenylenediamine was revealed as follows: the solubility of mphenylenediamine in pure and binary solvents could be increased with the increase of temperature.The experimental values were correlated with the Jouyban-Acree model, van't Hoff-Jouyban-Acree model,modified Apelblat-Jouyban-Acree model,Sun model and Ma model.The standard dissolution enthalpy,standard dissolution entropy and the Gibbs energy were calculated based on the experimental solubility data.In the binary solvent mixtures,the dissolution of m-phenylenediamine could be an endothermic process.The solubility data,correlation equations and thermodynamic property obtained from this study would be invoked as basic data and models regarding the purification and crystallization process of m-phenylenediamine.

1.Introduction

m-Phenylenediamine(CAS NO.108-45-2;structure shown in Fig.1)is an important raw material for chemicals and intermediates. It is widely used for the manufacture of fine chemicals such as dyestuff industry[1-3],polymers[4-6],aramid fibers[7]and comparative medical research[8].Various synthetic methods of m-phenylenediamine have been reported in previous literatures[9-14].The industrial process for producing m-phenylenediamine was by using catalytic hydrogenation of m-dinitrobenzene over a Ni/SiO2catalyst in solution.However,when the dehydration reaction is carried out in a solvent,the targeted m-phenylenediamine dissolved in the reaction system can reach equilibrium with m-dinitrobenzene, which could lead to the decrease of the purity of m-phenylenediamine. In the production process, 100%complete reaction is almost impossible and it is not easy to separate m-phenylenediamine from this mixture.

Solvent crystallization is the primary method of separation and purification in the production process of m-phenylenediamine.Solubility can affect the ability of the crystallization process,as well as its capacity to reject unwanted compounds and minimize loss in the mother liquor.In the previous literatures,the solubility of m-phenylenediamine was measured only in water and benzene [15]. In order to further study the crystallization process,it is necessary to determine the solubility of m-phenylenediamine in the solvent mixtures,which is the essential data for operations and optimization of crystallization procedures[16].In the production of m-phenylenediamine,the impurities are mainly a small amount of unreacted raw materials and the formation of o-phenylenediamine and p-phenylenediamine.Solvents are selected based on the properties of m-phenylenediamine and the solubility of phenylenediamine in previous literature [15,17]. Therefore, in this study, the solubility of m-phenylenediamine in three binary solvent mixtures of (methanol + water), (ethanol + water), and(acetonitrile+ water) with different solvent compositions has been determined at temperatures ranging from(278.15 to 313.15)K with a gravimetric method.In order to expend the application of the solubility,data of the experimental solubility of m-phenylenediamine were correlated by the Jouyban-Acree model[18,19],a combination of the Jouyban-Acree model with the van't Hoff equation[20,21],a combination of the Jouyban-Acree model with the modified Apelblat equation[20,21],the Ma model[22,23],and the Sun model[22,23].The standard dissolution enthalpy,standard dissolution entropy,and Gibbs energy were calculated for the dissolution process of m-phenylenediamine in the binary solvent mixtures.

2.Experimental

2.1.Materials

m-Phenylenediamine obtained from Sichuan North Hongguang Special Chemical Co.,Ltd.,China,its mass fraction purity was 0.992,which was determined by HPLC.It was dried in vacuum until the mass became constant,and then stored in a desiccator.The materials methanol,ethanol and acetonitrile are analytical grade reagents from Tianjin Chemical Reagent Co.,Ltd.,China.Distilled water was made in our laboratory.The materials in this experiment used are listed in Table 1.

2.2.Apparatus and methods

The solubility of m-phenylenediamine was measured by the gravimetric method in (methanol, ethanol, acetonitrile and water) and their binary solvents.The similar measurement method was described in the literature [24]. Experimental apparatus consisted of a water bath controlled by a thermostat (type SYP,China)with a fluctuation within 0.05 K, a 100 ml jacketed glass vessel, an analytical balance(Mettler Toledo AL104,Switzerland)with an accuracy of 0.0001 g and a magnetic stirrer(Fig.2).The jacketed glass vessel was connected to the water bath by the rubber hose,and the circulating water ensures that the solution dissolves at a constant temperature.The magnetic stirrer is used to ensure that the solute is better dissolved in the solvent.

Fig.2.Experimental setup of the solubility measurement.1—air condenser;2—sampling hole;3—thermometer;4—crystallization device;5—magnetic stirrer;6—constant temperature water bath.

In the experiment, the mixed solvents were configured with an analytical balance,and the mass fractions of methanol,ethanol or acetonitrile in the binary mixtures ranged from 0 to 1.Approximately 60 ml solvent mixtures and excess of m-phenylenediamine were placed in the jacketed glass vessel. The contents of the vessel were stirred for 12 h to reach the solid-liquid equilibrium and stood for 12 h at a constant temperature. Then 15 ml of clear upper saturated solution was pipetted into three small glass bottles averagely by a pipette gun.The solution was put into the vacuum drying oven until the mass no longer changes under T = 303.15 K and P = 0.1 MPa. The mass of the empty bottle,the saturated solution into the bottle and the bottle after drying was weighed by an analytical balance.In order to ensure the accuracy of the experimental data,we need to note the following:(1) During stirring process, we can always see the solid particles in the glass bottle and the m-phenylenediamine is determined as excess.On the contrary,m-phenylenediamine should be added to the bottle continuously.(2)After pipetting the liquid should be weighed immediately to prevent the liquid from staying in the air too long to evaporate.(3) The solubility data of m-phenylenediamine are calculated as the average of the three weighings.

The saturated solution mole fraction solubility of m-phenylenediamine(xφ,T)in the binary solvent mixtures can be obtained according to Eq.(1),and the initial composition of binary mixed solvents is defined by using Eqs.(2)and(3).

Table 1 The details of the materials used

Here, xφ,Tis the mole fraction solubility of m-phenylenediamine in binary solvent mixtures at absolute temperature T.The mmand mwrepresent the mass of m-phenylenediamine and water,respectively;and ms,the mass of methanol,ethanol or acetonitrile.The Mmand Mwsignify the molar mass of m-phenylenediamine and water,respectively;and Ms,the molar mass of methanol,ethanol or acetonitrile;φ and φsstand for the initial mass fraction of methanol,ethanol or acetonitrile in the binary solvent mixtures of(methanol+water),(ethanol+water)and(acetonitrile+water);and φw,the initial mass fraction of water.

2.3.Solubility models

2.3.1.Jouyban-Acree model

The Jouyban-Acree model described the dependence of both temperature and composition of mixed solvent on solute solubility[18,19].The general form of the Jouyban-Acree model can be defined as Eq.(4).

Here xms,Tis the mole fraction solubility of m-phenylenediamine in methanol,ethanol or acetonitrile,and xmw,Tthe mole fraction solubility of m-phenylenediamine in water.Jiterms(J0,J1,J2)are the Jouyban-Acree model parameters.

2.3.2.van't Hoff-Jouyban-Acree model

The van't Hoff equation reveals the dependence of the natural logarithm of mole fraction solubility on the reciprocal of absolute temperature[25].The equation can be expressed as Eq.(5).

Here x is the mole fraction solubility of m-phenylenediamine in pure solvent;T is the absolute temperature;and A and B are the equation constants.

The van't Hoff-Jouyban-Acree model can be obtained by substituting Eq.(5)to Eq.(4).

Here A1,B1,A2,B2and Jiterms are the model parameters.The van't Hoff-Jouyban-Acree model can be used to depict the solute solubility in binary solvent mixtures at various temperatures and solvent compositions[20,21].

2.3.3.Apelblat-Jouyban-Acree model

The expression of the modified Apelblat equation is described as Eq.(7).It shows a nonlinear relationship between the natural logarithm of mole fraction solubility and the reciprocal of absolute temperature[26].

Here,A,B,and C are equation parameters.

Substituting Eq.(7)to Eq.(4),the combination of the Jouyban-Acree model with the modified Apelblat equation can be obtained[20,21].The Apelblat-Jouyban-Acree model can be expressed as Eq.(8).

Here A1,B1,C1,A2,B2,C2and Jiterms are the equation parameters of the Apelblat-Jouyban-Acree model.

2.3.4.Sun model

Sun and co-workers[22,23]got Eq.(9)by modifying the Jouyban-Acree model.It is used to correlate the solubility of a solute in binary mixed solvents at different temperatures.

Here D1,D2,D3,D4,D5,D6and D7are equation parameters.

2.3.5.Ma model

Ma and co-workers [22,23] propose another modified equation about the Apelblat-Jouyban-Acree model to correlate the solubility of a solute in binary solvent mixtures at different temperatures,which is described as

Here E1to E9are constants.

3.Results and Discussion

3.1.Solubility data and discussion

Tables 2-4 list the experimental mole fraction solubility data of m-phenylenediamine in binary solvent mixtures of(methanol+water), (ethanol + water) and (acetonitrile + water) with various mass fractions within the temperature range (278.15 to 313.15) K under 101.1 kPa. Due to comparison with the experimental points,the dependence of mole fraction solubility upon temperature and solvent composition is shown in Figs. 3-5. The solubility of m-phenylenediamine increases with increasing temperature and mass fraction of methanol, ethanol or acetonitrile for the systems of (methanol + water), (ethanol + water) and (acetonitrile +water), and the maximum solubility values of m-phenylenediamine are observed in pure organic solvent. Tables 2-4 also show that at the same temperature and mass fraction of solvent, the mole fraction solubility of m-phenylenediamine is in descending order of (acetonitrile+ water), (methanol+ water),(ethanol+ water)binary solvent mixtures. When comparing the solubility of mphenylenediamine with o-phenylenediamine (the previous literature by Yao[17])in water,methanol,ethanol,acetonitrile and binary solvent mixtures of (water + methanol), (water + ethanol) and(water + acetonitrile), it can be found that the solubility of mphenylenediamine is 2 to 12 times that of o-phenylenediamine.This conclusion is consistent with the literature[15].The influencing factors are comparatively complex, including the polarity of the studied solvents, intermolecular interactions between the solute and the solvent,and hydrogen bonding interaction.

Ta ble 2 Ex perimental mo le fraction so lu bility(xeφ,T)an d its stan dard un certainty u(x)to geth er with calculated so lu bility valu es(xcφ,T)by th e Jo uy ban-Acree mo del of m-ph en ylen ed iamine in binary so lv en t mixtures of(m ethano l(φ)+w ater(1-φ))with differen t m ass fractio ns w ithin the tem peratu re ran ge fro m(27 8.15 to 3 13.1 5)K u nd er 1 01.1 k Pa①u(x)xφ, Tc 0.05 35 0.06 29 0.07 39 0.08 71 0.10 28 0.11 93 0.13 94 0.16 11 0.18 99 0.22 14 0.25 67 0.29 58 0.34 10 0.39 43 0.45 15 0.00 21 0.00 25 0.00 23 0.00 19 0.00 29 0.00 35 0.00 16 0.00 30 0.00 11 0.00 24 0.00 28 0.00 31 0.00 09 0.00 06 0.00 18 0.49 98φ=xφ, Te 0.05 40 0.06 37 0.07 47 0.08 90 0.10 33 0.12 21 0.14 02 0.16 29 0.18 94 0.22 11 0.25 25 0.29 12 0.33 41 0.38 73 0.44 36 u(x)xφ, Tc 0.04 45 0.05 28 0.06 27 0.07 46 0.08 89 0.10 40 0.12 25 0.14 27 0.16 98 0.19 95 0.23 32 0.27 06 0.31 45 0.36 56 0.42 17 0.00 14 0.00 27 0.00 19 0.00 15 0.00 21 0.00 10 0.00 16 0.00 06 0.00 19 0.00 28 0.00 24 0.00 11 0.00 13 0.00 19 0.00 08 0.40 01φ=xφ, Te 0.04 52 0.05 40 0.06 36 0.07 53 0.08 94 0.10 37 0.12 26 0.14 36 0.16 89 0.19 66 0.22 84 0.26 49 0.30 65 0.35 35 0.40 82 u(x)xφ, Tc 0.03 56 0.04 28 0.05 12 0.06 15 0.07 41 0.08 74 0.10 39 0.12 19 0.14 63 0.17 33 0.20 44 0.23 89 0.27 99 0.32 73 0.38 05 0.00 23 0.00 15 0.00 28 0.00 21 0.00 26 0.00 06 0.00 09 0.00 18 0.00 16 0.00 20 0.00 19 0.00 33 0.00 23 0.00 20 0.00 14 0.30 03φ=xφ, Te 0.03 62 0.04 38 0.05 20 0.06 30 0.07 45 0.08 86 0.10 49 0.12 28 0.14 51 0.16 98 0.19 96 0.23 18 0.26 84 0.31 21 0.36 28 u(x)xφ, Tc 0.02 63 0.03 18 0.03 88 0.04 70 0.05 73 0.06 82 0.08 19 0.09 68 0.11 73 0.14 02 0.16 69 0.19 66 0.23 24 0.27 35 0.32 05 0.00 07 0.00 12 0.00 11 0.00 28 0.00 21 0.00 25 0.00 18 0.00 13 0.00 30 0.00 25 0.00 09 0.00 14 0.00 26 0.00 20 0.00 17 0.19 99φ=xφ, Te 0.02 79 0.03 30 0.04 01 0.04 82 0.05 70 0.06 92 0.08 25 0.09 84 0.11 69 0.13 78 0.16 31 0.19 27 0.22 59 0.26 48 0.30 83 u(x)xφ, Tc 0.01 72 0.02 10 0.02 59 0.03 18 0.03 92 0.04 75 0.05 73 0.06 82 0.08 36 0.10 08 0.12 13 0.14 41 0.17 21 0.20 40 0.24 12 0.00 11 0.00 15 0.00 21 0.00 19 0.00 23 0.00 20 0.00 28 0.00 29 0.00 18 0.00 14 0.00 08 0.00 19 0.00 35 0.00 33 0.00 28 0.2 k Pa.0.10 02φ=xφ, Te 0.01 79 0.02 21 0.02 70 0.03 27 0.03 93 0.04 86 0.05 85 0.06 93 0.08 30 0.09 81 0.11 74 0.14 23 0.16 92 0.20 14 0.23 25 0.05 K,u(p)=u(x)xφ, Tc 0.00 93 0.01 15 0.01 44 0.01 79 0.02 23 0.02 72 0.03 34 0.04 02 0.04 99 0.06 08 0.07 41 0.08 89 0.10 73 0.12 83 0.15 33 0.00 03 0.00 18 0.00 12 0.00 15 0.00 31 0.00 26 0.00 29 0.00 12 0.00 17 0.00 05 0.00 08 0.00 24 0.00 20 0.00 24 0.00 11 0φ=xφ, Te 0.00 93 0.01 15 0.01 44 0.01 79 0.02 23 0.02 72 0.03 34 0.04 02 0.04 99 0.06 08 0.07 41 0.08 89 0.10 73 0.12 83 0.15 33 T/K 27 8.15 28 0.65 28 3.15 28 5.65 28 8.15 29 0.65 29 3.15 29 5.65 29 8.15 30 0.65 30 3.15 30 5.65 30 8.15 31 0.65 31 3.15① Valu es o f the stand ard u ncertain ty u are u(T)=

?

Ta ble 3(con tinu ed)u(x)xφ, Tc 0.10 72 0.12 16 0.13 62 0.15 59 0.17 49 0.19 62 0.22 08 0.24 81 0.27 67 0.30 92 0.34 38 0.38 12 0.42 62 0.47 94 0.53 56 0.00 25 0.00 26 0.00 21 0.00 32 0.00 14 0.00 27 0.00 09 0.00 34 0.00 20 0.00 11 0.00 27 0.00 19 0.00 08 0.00 26 0.00 27 1φ=xφ, Te 0.10 72 0.12 16 0.13 62 0.15 59 0.17 49 0.19 62 0.22 08 0.24 81 0.27 67 0.30 92 0.34 38 0.38 12 0.42 62 0.47 94 0.53 56 u(x)xφ, Tc 0.09 62 0.12 44 0.14 34 0.10 99 0.16 24 0.18 35 0.20 80 0.23 51 0.26 47 0.29 81 0.33 42 0.37 31 0.41 99 0.47 48 0.53 35 0.00 06 0.00 10 0.00 20 0.00 17 0.00 31 0.00 17 0.00 19 0.00 28 0.00 26 0.00 17 0.00 10 0.00 15 0.00 29 0.00 13 0.00 19 0.90 02φ=xφ, Te 0.09 61 5 0.11 05 0.12 56 0.14 34 0.16 28 0.18 49 0.20 87 0.23 51 0.26 56 0.29 82 0.33 43 0.37 41 0.41 88 0.46 71 0.52 03 u(x)xφ, Tc 0.08 41 0.09 69 0.11 07 0.12 86 0.14 71 0.16 74 0.19 13 0.21 75 0.24 73 0.28 07 0.31 73 0.35 67 0.40 43 0.45 95 0.51 94 0.00 19 0.00 38 0.00 15 0.00 29 0.00 22 0.00 25 0.00 11 0.00 12 0.00 19 0.00 23 0.00 26 0.00 17 0.00 19 0.00 31 0.00 09 0.80 03φ=xφ, Te 0.08 43 0.09 72 0.11 14 0.12 83 0.14 72 0.16 89 0.19 13 0.21 84 0.24 71 0.28 01 0.31 77 0.35 87 0.40 36 0.45 32 0.50 84 u(x)xφ, Tc 0.07 25 0.08 41 0.09 72 0.11 37 0.13 14 0.15 06 0.17 35 0.19 85 0.22 79 0.26 08 0.29 72 0.33 66 0.38 41 0.43 90 0.49 92 0.00 22 0.00 16 0.00 17 0.00 29 0.00 18 0.00 26 0.00 17 0.00 10 0.00 35 0.00 31 0.00 19 0.00 13 0.00 26 0.00 13 0.00 23 0.70 05φ=xφ, Te 0.07 25 0.08 45 0.09 82 0.11 36 0.13 13 0.15 17 0.17 45 0.19 91 0.22 73 0.26 07 0.29 71 0.33 73 0.38 43 0.43 37 0.49 00 0.0 0 3 1 xφ, Tc 0.06 18 0.07 23 0.08 44 0.09 94 0.11 61 0.13 41 0.15 57 0.17 93 0.20 79 0.23 99 0.27 57 0.31 45 0.36 14 0.41 53 0.47 52 0.00 29 0.00 19 0.00 35 0.00 16 0.00 10 0.00 11 0.00 24 0.00 22 0.00 31 0.00 21 0.00 23 0.00 28 0.00 11 0.00 17 0.00 28 0.60 01φ=xφ, Te 0.06 19 0.07 27 0.08 51 0.09 95 0.11 56 0.13 47 0.15 63 0.18 02 0.20 97 0.23 98 0.27 58 0.31 53 0.36 09 0.41 08 0.46 76 T/K 27 8.15 28 0.65 28 3.15 28 5.65 28 8.15 29 0.65 29 3.15 29 5.65 29 8.15 30 0.65 30 3.15 30 5.65 30 8.15 31 0.65 31 3.15

water(1-φ))Ta ble 4 Ex perimental m ole fractio n solub ility(xeφ, T)and its stand ard u ncertain ty u(x)tog ether w ith calcu lated solub ility v alues(xcφ, T)b y the Jo uy ban-Acree m od el o f m-p heny lenediam in e in binary solvent m ix tu res o f(aceton itrile(φ)+with d ifferent m ass fractio ns w ithin the tem peratu re ran ge fro m(27 8.15 to 3 13.1 5)K u nd er 1 01.1 k Pa①u(x)xφ, Tc 0.06 90 0.07 95 0.09 25 0.10 77 0.12 59 0.14 49 0.16 78 0.19 37 0.22 84 0.26 51 0.30 47 0.34 72 0.39 64 0.44 98 0.51 13 0.00 15 0.00 21 0.00 12 0.00 23 0.00 18 0.00 32 0.00 09 0.00 22 0.00 13 0.00 25 0.00 23 0.00 33 0.00 09 0.00 14 0.00 28 0.49 98φ=xφ, Te 0.06 97 0.08 11 0.09 29 0.10 72 0.12 33 0.14 53 0.16 99 0.19 89 0.22 95 0.26 39 0.30 12 0.33 87 0.38 39 0.43 62 0.49 64 u(x)xφ, Tc 0.05 64 0.06 59 0.07 76 0.09 14 0.10 81 0.12 57 0.14 70 0.17 09 0.20 32 0.23 78 0.27 61 0.31 74 0.36 59 0.41 90 0.48 03 0.00 22 0.00 19 0.00 17 0.00 06 0.00 18 0.00 21 0.00 11 0.00 16 0.00 14 0.00 21 0.00 33 0.00 23 0.00 10 0.00 17 0.00 14 0.40 06φ=xφ, Te 0.05 78 0.06 82 0.07 99 0.09 34 0.10 92 0.12 75 0.14 81 0.17 28 0.20 06 0.23 49 0.27 13 0.31 29 0.35 49 0.40 54 0.45 85 u(x)xφ, Tc 0.04 39 0.05 19 0.06 21 0.07 40 0.08 85 0.10 39 0.12 28 0.14 39 0.17 26 0.20 38 0.23 91 0.27 74 0.32 30 0.37 33 0.43 18 0.00 25 0.00 12 0.00 19 0.00 24 0.00 29 0.00 21 0.00 18 0.00 23 0.00 16 0.00 18 0.00 13 0.00 35 0.00 28 0.00 17 0.00 26 0.30 01φ=xφ, Te 0.04 71 0.05 45 0.06 33 0.07 56 0.08 97 0.10 64 0.12 54 0.14 63 0.17 19 0.20 13 0.23 56 0.27 22 0.31 23 0.35 67 0.40 38 u(x)xφ, Tc 0.03 11 0.03 73 0.04 53 0.05 46 0.06 62 0.07 86 0.09 39 0.11 09 0.13 43 0.16 01 0.18 99 0.22 25 0.26 19 0.30 58 0.35 71 0.00 30 0.00 16 0.00 18 0.00 25 0.00 28 0.00 17 0.00 08 0.00 14 0.00 26 0.00 16 0.00 33 0.00 15 0.00 17 0.00 11 0.00 09 0.20 03φ=xφ, Te 0.03 20 0.03 94 0.04 62 0.05 65 0.06 76 0.08 08 0.09 54 0.10 73 0.12 68 0.14 93 0.17 85 0.21 12 0.24 82 0.29 16 0.34 37 u(x)xφ, Tc 0.01 90 0.02 31 0.02 84 0.03 48 0.04 28 0.05 14 0.06 22 0.07 41 0.09 07 0.10 93 0.13 13 0.15 55 0.18 53 0.21 87 0.25 82 0.00 12 0.00 25 0.00 28 0.00 05 0.00 09 0.00 14 0.00 28 0.00 29 0.00 17 0.00 13 0.00 13 0.00 25 0.00 28 0.00 21 0.00 33 0.2 k Pa.0.09 99φ=xφ, Te 0.02 02 0.02 47 0.03 02 0.03 61 0.04 42 0.05 26 0.06 37 0.07 57 0.09 08 0.10 71 0.12 76 0.15 13 0.18 19 0.21 54 0.25 15 0.05 K,u(p)=u(x)xφ, Tc 0.00 93 0.01 15 0.01 44 0.01 79 0.02 23 0.02 72 0.03 34 0.04 02 0.04 99 0.06 08 0.07 41 0.08 89 0.10 73 0.12 83 0.15 33 0.00 03 0.00 18 0.00 12 0.00 15 0.00 31 0.00 26 0.00 29 0.00 12 0.00 17 0.00 05 0.00 08 0.00 24 0.00 20 0.00 24 0.00 11 0φ=xφ, Te 0.00 93 0.01 15 0.01 44 0.01 79 0.02 23 0.02 72 0.03 34 0.04 02 0.04 99 0.06 08 0.07 41 0.08 89 0.10 73 0.12 83 0.15 33 T/K 27 8.15 28 0.65 28 3.15 28 5.65 28 8.15 29 0.65 29 3.15 29 5.65 29 8.15 30 0.65 30 3.15 30 5.65 30 8.15 31 0.65 31 3.15① Valu es o f the stand ard u ncertain ty u are u(T)=

Ta ble 4(con tinu ed)u(x)xφ, Tc 0.17 17 0.18 61 0.20 27 0.22 34 0.24 64 0.27 07 0.29 83 0.33 26 0.37 62 0.41 87 0.45 83 0.49 99 0.54 42 0.59 07 0.64 38 0.00 22 0.00 04 0.00 31 0.00 27 0.00 33 0.00 09 0.00 23 0.00 16 0.00 28 0.00 25 0.00 18 0.00 14 0.00 07 0.00 13 0.00 25 1φ=xφ, Te 0.17 17 0.18 61 0.20 27 0.22 34 0.24 64 0.27 07 0.29 83 0.33 26 0.37 62 0.41 87 0.45 83 0.49 99 0.54 42 0.59 07 0.64 38 u(x)xφ, Tc 0.14 53 0.15 94 0.17 59 0.19 60 0.21 86 0.24 24 0.26 97 0.30 28 0.34 53 0.38 75 0.42 83 0.47 12 0.51 78 0.56 70 0.62 32 0.00 09 0.00 21 0.00 23 0.00 27 0.00 15 0.00 27 0.00 23 0.00 25 0.00 29 0.00 16 0.00 13 0.00 36 0.00 08 0.00 07 0.00 13 0.90 02φ=xφ, Te 0.14 64 0.15 68 0.17 36 0.19 19 0.21 38 0.24 11 0.27 36 0.30 74 0.34 64 0.38 18 0.42 38 0.46 51 0.50 86 0.56 19 0.61 57 u(x)xφ, Tc 0.12 03 0.13 36 0.14 94 0.16 83 0.19 00 0.21 27 0.23 90 0.27 02 0.31 07 0.35 16 0.39 25 0.43 56 0.48 33 0.53 40 0.59 18 0.00 28 0.00 18 0.00 17 0.00 25 0.00 11 0.00 26 0.00 19 0.00 22 0.00 27 0.00 15 0.00 18 0.00 09 0.00 10 0.00 18 0.00 19 0.80 06φ=xφ, Te 0.11 81 0.13 15 0.14 83 0.16 66 0.18 85 0.21 58 0.24 83 0.28 21 0.31 41 0.35 47 0.39 45 0.43 48 0.47 93 0.52 96 0.58 17 u(x)xφ, Tc 0.09 95 0.11 19 0.12 68 0.14 44 0.16 50 0.18 64 0.21 16 0.24 09 0.27 93 0.31 87 0.35 93 0.40 23 0.45 07 0.50 24 0.56 15 0.00 13 0.00 11 0.00 28 0.00 24 0.00 19 0.00 16 0.00 07 0.00 12 0.00 17 0.00 35 0.00 28 0.00 22 0.00 10 0.00 27 0.00 31 0.70 01φ=xφ, Te 0.09 85 0.11 31 0.12 89 0.14 82 0.16 81 0.19 24 0.21 69 0.24 57 0.27 87 0.31 43 0.35 41 0.39 54 0.44 29 0.49 62 0.55 53 u(x)xφ, Tc 0.08 28 0.09 43 0.10 83 0.12 47 0.14 41 0.16 43 0.18 84 0.21 60 0.25 25 0.29 06 0.33 08 0.37 36 0.42 26 0.47 52 0.53 57 0.00 32 0.00 26 0.00 17 0.00 23 0.00 28 0.00 19 0.00 04 0.00 35 0.00 26 0.00 11 0.00 24 0.00 27 0.00 31 0.00 22 0.00 14 0.60 02φ=xφ, Te 0.08 29 0.09 61 0.11 01 0.12 74 0.14 63 0.16 86 0.19 31 0.22 29 0.25 49 0.28 95 0.32 43 0.36 56 0.41 21 0.46 64 0.52 15 T/K 27 8.15 28 0.65 28 3.15 28 5.65 28 8.15 29 0.65 29 3.15 29 5.65 29 8.15 30 0.65 30 3.15 30 5.65 30 8.15 31 0.65 31 3.15

Fig.3.Mole fraction solubility(x)of m-phenylenediamine in{methanol(φ)+water(1-φ)}mixed solutions with various mass fractions at different temperatures:●,φ=0;■,φ=0.1002; ▲, φ = 0.1999; ▼, φ = 0.3003; ★φ = 0.4001; □, φ = 0.4498; ○, φ = 0.6006;△,φ=0.7010;▽,φ=0.8005;☆,φ=0.9008;◑,φ=1;solid line,calculated curves by the Jouyban-Acree model.

Fig.6 shows some discrepancy,which exists between our solubility values of m-phenylenediamine in water with those of reference[15].We consider that the main reason for the discrepancy is the difference of the methods used. In addition, the solubility values have been checked many times when we study the recrystallization process.Therefore,we are absolutely sure that our values are accurate.

3.2.Correlation of the solubility data

Five solubility models are used to correlate the solubility results of m-phenylenediamine in mixed solvents of (methanol+ water),(ethanol+water)and(acetonitrile+water),which are the Jouyban-Acree model (Eq. (4)), van't Hoff-Jouyban-Acree model (Eq. (6)),Alphabet-Jouyban-Acree model(Eq.(8)),Sun model(Eq.(9))and Ma model(Eq.(10)).

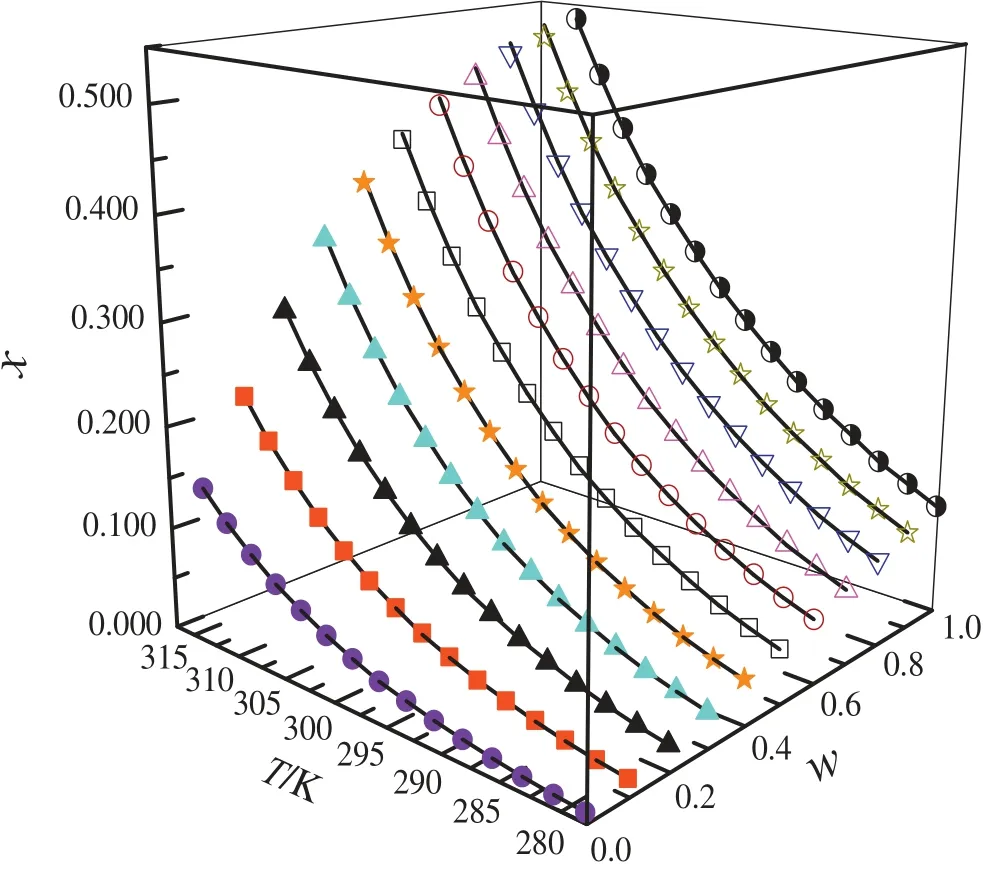
Fig.4.Mole fraction solubility(x)of m-phenylenediamine in{ethanol(φ)+water(1-φ)}mixed solutions with various mass fractions at different temperatures:●,φ=0;■,φ=0.1008;▲,φ=0.2001;▼,φ=0.3009;★,φ=0.3998;□,φ=0.5002;○,φ=0.6001;△,φ=0.7005;▽,φ=0.8003;☆,φ=0.9002;◑,φ=1;solid line,calculated curves by the Jouyban-Acree model.

Fig.5.Mole fraction solubility (x) of m-phenylenediamine in {acetonitrile (φ) + water(1 - φ)} mixed solutions with various mass fractions at different temperatures:●,φ=0;■, φ = 0.0999; ▲, φ = 0.2003; ▼, φ = 0.3001; ★φ = 0.4006; □, φ = 0.4998; ○, φ =0.6002; △, φ = 0.7001; ▽, φ = 0.8006;☆, φ = 0.9002; ◑,φ = 1; solid line, calculated curves by the Jouyban-Acree model.

The relative average deviation(RAD)and root-mean-square deviation(RMSD)are employed to estimate the error calculated with different models,which are described as Eqs.(11)and(12),respectively.

Here N is the number of experimental points;xcφ,Tand xeφ,Trepresent the calculated and experimental values of solubility,respectively.

Fig. 6. The comparison between experimental values and literature values of mphenylenediamine:■,experimental data;●,literature data.

The calculated values for five model parameters together with the RAD and the RMSD values are shown in Table 5. Table 5 shows that the values of RAD and RMSD are no greater than 1.91×10-2and 5.88×10-3,respectively, indicating that the calculated solubility of the five models agrees well with the experimental values.However,the lowest values for RAD and RMSD are obtained in the van't Hoff-Jouyban-Acree model of the five models.So the van't Hoff-Jouyban-Acree model is the best correlation equation to correlate the experimental values.In addition,the calculated solubility with the Jouyban-Acree model is shown graphically in Figs.7-9.

3.3.Prediction of thermodynamic properties

When the solubility of m-phenylenediamine in different solvents is confirmed,some thermodynamic properties such as the dissolution enthalpy,dissolution entropy and the Gibbs energy can be calculated.The function between the mole fraction solubility and the absolute temperature can be expressed as[27]:

where R denotes the gas constant (8.3145 J·K-1·mol-1), ΔdisH and ΔdisS are the dissolution enthalpy and dissolution entropy,which can be calculated by the slope and intercept of the lines in Figs.6-8,respectively.Figs.6-8 represent the plots of lnxφ,T～(1/T)for m-phenylenediamine dissolved in binary mixed solutions of(methanol+water),(ethanol+water)and(acetonitrile+water).The mean value of Gibbs energy of the dissolution in different solvents can be calculated as:

Fig.7.The relationship between lnx and 1/T of m-phenylenediamine in{methanol(φ)+water(1-φ)}mixed solutions with various mass fractions:●,φ=0;■,φ=0.1002;▲,φ=0.1999;▼,φ=0.3003;★,φ=0.4001;□,φ=0.4498;○,φ=0.6006;△,φ=0.7010;▽,φ=0.8005;☆,φ=0.9008;◑,φ=1.

where Tmeanrepresents the mean temperature of the experiment,which can be calculated by Eq.(15):

Table 5 Values of parameters obtained using solubility models

Fig.8.The relationship between lnx and 1/T of m-phenylenediamine in{ethanol(φ)+water(1-φ)}mixed solutions with various mass fractions:●,φ=0;■,φ=0.1008;▲,φ=0.2001;▼,φ=0.3009;★,φ=0.3998;□,φ=0.5002;○,φ=0.6001;△,φ=0.7005;▽,φ=0.8003;☆,φ=0.9002;◑,φ=1.

The contribution of enthalpy and entropy to the Gibbs energy in the dissolution process is compared by the following Eqs.(16)and(17):

The calculated dissolution enthalpy, entropy, and Gibbs energy change together with ξH,ξScalculated by the experimental values is shown in Table 6.It can be seen that the ξHis greater than ξSin every solvent,illustrating that the main contributor to the standard molar Gibbs energy of dissolution is the enthalpy rather than entropy.The values of ΔdisH are positive in all selected solvents,indicating that the dissolution of m-phenylenediamine is an endothermic process.

Fig.9.The relationship between lnx and 1/T of m-phenylenediamine in{acetonitrile(φ)+water(1-φ)}mixed solutions with various mass fractions: ●, φ = 0; ■, φ = 0.0999;▲, φ = 0.2003; ▼,φ=0.3001;★,φ=0.4006;□,φ=0.4998;○,φ=0.6002;△,φ=0.7001;▽,φ=0.8006;☆,φ=0.9002;◑,φ=1.

Table 6 The calculated values of dissolution enthalpy, entropy, and Gibbs energy in mixed solvents of(methanol+water),(ethanol+water)and(acetonitrile+water)at mean temperature(295.65 K)

4.Conclusions

The solubility of m-phenylenediamine in four pure solvents(methanol,ethanol,acetonitrile and water)and three binary solvents(methanol + water), (ethanol + water) and (acetonitrile + water)systems were determined from 278.15 K to 313.15 K by using the gravimetric method under 101.1 kPa in this work.

(1) For the four pure solvents and binary solvent mixtures at constant solvent composition, the solubility of m-phenylenediamine increases with the increase of experimental temperature.

(2) At constant temperature and solvent composition,the mole fraction solubility of m-phenylenediamine decreases in the following order:(acetonitrile+water),(methanol+water),(ethanol+water)mixed solvents.

(3) Five models were used to correlate the experimental data,and it was shown that calculated solubility values show good agreement with experimental values.The relative average deviations(RAD)and root-mean-square deviations(RMSD)are no greater than 1.91×10-2and 5.88×10-3,respectively.The lowest values for RAD and RMSD are obtained in the van't Hoff-Jouyban-Acree model of the five models.Although the calculated solubility of the five models agrees well with the experimental values, the van't Hoff-Jouyban-Acree model is the best correlation equation to correlate the experimental values.

(4) The standard dissolution enthalpy, standard dissolution entropy and the Gibbs energy are calculated on the basis of the experimental solubility data.The values of ΔdisH are positive,indicating the dissolution of m-phenylenediamine is an endothermic process.

The solubility data, correlation equations and thermodynamic property obtained from this study can be used for the purification and crystallization of m-phenylenediamine.