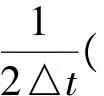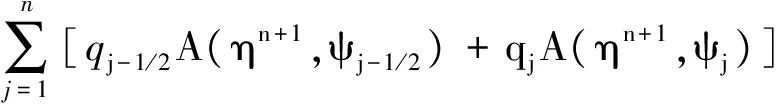一类地下水污染物运移问题的有限体积元方法

骆小芳, 朱泉涌, 林银河*

(1. 南京师范大学 数学科学学院, 江苏 南京 210023; 2. 丽水学院 数学系, 浙江 丽水 323000)

地下水作为水资源的重要组成部分,就水体污染而言,地下水的污染与地表水的污染相比更具有隐蔽性和难以逆转性.地下水一旦受污染,便很难治理及恢复.由于地下水污染流体动力系统的复杂性,传统的实验研究方法花费大、周期长、灵活性差且难以重复实验.因此,研究建立在数学物理方法基础上的地下水污染数值方法具有重要的理论和应用价值[1-8].本文研究地下水污染模型问题[9-13]

(1)

的有限体积元解,其中,R为阻滞因子,s表示地下水污染物的浓度,k表示地下水实际流动速度,d表示扩散系数,λ表示衰减系数,s0(x)为已知的光滑函数,D=[0,L].方程(1)在地下水污染物运移问题中具有深刻的物理背景,并且有着广泛的应用[14-17].

为方便讨论，R取为1，假设问题(1)的解存在唯一且有必要的光滑性,并满足下列条件:

|k(x,q1)-k(x,q2)|≤K1|q1-q2|;

(IV) ∀x∈D,有0

下文中用K和ε分别表示一般的正常数和充分小的正常数,它们在不同的地方可以表示不同的值.此外,记

1 有限体积元离散方法

选取试探函数空间Ch为相应于剖分Th的拉格朗日型二次有限元空间,则整节点xi与半整节点xi-1/2的基函数分别为

而任意sh∈Ch可唯一表示为

φi(x)+si-1/2φi-1/2(x)],

其中si=sh(xi,t),si-1/2=sh(xi-1/2,t).

则任一wh∈Wh可表示为

其中,wj=wh(xj),wj-1/2=wh(xj-1/2).

∀s∈Ch.

对∀sh∈Ch,引入下面的离散范数

|sh|1,h=

引理1[9]对任意的sh∈Ch,|sh|0,h、|sh|1,h分别与|sh|0、|sh|1等价,即存在与h无关的正常数c1、c2、c3、c4,使得

c1|sh|0,h≤|sh|0≤c2|sh|0,h,

c3|sh|1,h≤|sh|1≤c4|sh|1,h.

引理2[9]下列结论成立:

引理3[9]存在正常数h0、α和M,使得当0

c5‖sh‖1≤|||sh|||1≤c6‖sh‖1,

∀sh∈Ch.

引入记号

sh=si-1(2ξ-1)(ξ-1)+

4si-1/2ξ(1-ξ)+siξ(2ξ-1),

(3)

(4)

即

2 误差估计

记ξ=sh-Πhs,η=s-Πhs,则sh-s=ξ-η.由(2)和(4)式得误差方程

(6)

由引理3得

下面对(6)式右端各项分别进行估计,运用ε不等式,容易得到

但

故

由

而

故

则

由

M(△t)2,

则

由

故

以上各式联立，可得

(7)

综上所述可得如下定理.

定理1假设s是问题(1)的解,sh为全离散有限体积二次元格式(4)的解,当h与△t充分小时,有以下的误差估计成立

[1] TRAVERSO L, PHILLIPS T N, YANG Y. Mixed finite element methods for groundwater flow in heterogeneous aquifers[J]. Comput Fluids,2013,88(88):60-80.

[2] ZHU Q, WANG Q, FU J, et al. New second-order finite difference scheme for the problem of contaminant in groundwater flow[J]. J Appl Math,2012,2012:1-16.

[3] 朱泉涌,王全祥,付菊. 一类抛物型偏微分方程的特征中心差分方法[J]. 纯粹数学与应用数学,2012(4):507-515.

[4] ZHANG G, LU D, YE M, et al. An adaptive sparse grid high order stochastic collocation method for Bayesian inference in groundwater reactive transport modeling[J]. Water Resources Research,2013,49(10):6871-6892.

[5] ANDERSON M P, WOESSNER W W, HUNT R J. Applied Groundwater Modeling:Simulation of Flow and Advective Transport[M]. New York:Academic Press,2015.

[6] HE X, JIANG L, MOULTON J D. A stochastic dimension reduction multiscale finite element method for groundwater flow problems in heterogeneous random porous media[J]. J Hydrology,2013,478(3):77-88.

[7] VANDENBOER K, VAN BEEK V, BEZUIJEN A. 3D finite element method (FEM) simulation of groundwater flow during backward erosion piping[J]. Frontiers of Structural and Civil Engineering,2014,8(2):160-166.

[8] XIE Y, WU J, XUE Y, et al. Modified multiscale finite-element method for solving groundwater flow problem in heterogeneous porous media[J]. J Hydrologic Engineering,2014,19(8):04014004.

[9] LI R, CHEN Z, WU W. Generalized Difference Methods for Differential Equations:Numerical Analysis of Finite Volume Methods[M]. Boca Raton:CRC Press,2000.

[10] WANG Q, ZHANG Z, ZHANG X, et al. Energy-preserving finite volume element method for the improved Boussinesq equation[J]. J Comput Phys,2014,270(8):58-69.

[11] RUIZ-BAIER R, TORRES H. Numerical solution of a multidimensional sedimentation problem using finite volume-element methods[J]. Appl Numer Math,2015,95(C):280-291.

[12] BUELER E. Stable finite volume element schemes for the shallow-ice approximation[J]. J Glaciology,2016,62(232):230-242.

[13] KUMAR S, RUIZ-BAIER R. Equal order discontinuous finite volume element methods for the Stokes problem[J]. J Scientific Computing,2015,65(3):956-978.

[14] DILLON P J. An analytical model of contaminant transport from diffuse sources in saturated porous media[J]. Water Resources Research,1989,25(25):1208-1218.

[15] ZHANG Z. Error estimates of finite volume element method for the pollution in groundwater flow[J]. Numerical Methods for Partial Differential Equations,2009,25(2):259-274.

[16] GRATHWOHL P. Diffusion in Natural Porous Media:Contaminant Transport, Sorption/Desorption and Dissolution Kinetics[M]. New York:Springer-Verlag,2012.

[17] BEAR J, CORAPCIOGLU M Y. Advances in Transport Phenomena in Porous Media[M]. New York:Springer-Verlag,1987.