Transport properties of dilute ammonia-noble gas mixtures from new intermolecular potential energy functions

Farshid Zargari*,Delara Mohammad-Aghaie ,Maryam Lot fi,Masoume Ghorbanipour ,Mohammad Mehdi Alavianmehr ,Omolbanin Shahraki**

1 Department of Chemistry,Shiraz University of Technology,Shiraz,Iran

2 Medicinal and Natural Products Chemistry Research Center,Shiraz University of Medical Sciences,Shiraz,Iran

3 Department of Physical Chemistry,University of Tabriz,Tabriz,Iran

4 Zahedan University of Medical Sciences,Zahedan,Iran

1.Introduction

Transport properties of matters in different thermo-physical states are important features,required in different engineering design problems,such as simulations of viscous flows through channels and combustion chambers of various technical devices,such as flows in rocket engines,chemical reactors and shock tubes.There is a growing demand for more accurate transport properties,in response to the worldwide need for higher efficiency,process integration and energy awareness in the industry[1].

Three principal transport properties of industrial interest are the viscosity,η,the diffusivity,D,and the thermal conductivity,λ,which characterize the dynamics of fluids,involving the momentum transport related to the pressure difference,the mass transport related to the concentration difference,and the heat transport related to the temperature difference,respectively[2].

Prediction and determination of equilibrium and non-equilibrium properties of a matter,and interpretation of most phenomena involving atoms and molecules,depend on knowledge of intermolecular forces.Once the interaction potential is known,evaluation of thermophysical properties of fluids will be straightforward.This reduces the need for experimentation considerably.Various methods of determining intermolecular potentials have been used,either theoretical,based on experimental information,or combining both approaches[3,4].

Among these methods,inversion of dilute gas properties has been of particular importance.The semi-empirical method of inversion,developed by Maitl and and co-workers,determines unique potential energy function from thermo physical properties,without explicit assumption of a mathematical model[5-8].

The Chapman-Enskog solution of the Boltzmann equation of gas kinetic theory,provides expressions to relate the transport coefficients of gases to interaction potential energy,through collision integrals,Ω(l,s)(T*)[9,10].For noble gases,with spherically symmetric potentials,inversion of experimental second virialco efficients,B2(T),is directly related to the pair potential U(r).Combining this result with other experimental sources such as viscosity and diffusion coefficients,and quantum mechanical calculations,provides an accurate knowledge of U(r)for these simple substances[11-14].

However,the Chapman-Enskog theory for transport properties of polyatomic gases with non-spherical interactions is fundamentally different from that of monatomic molecules,due to the possibility of inelastic collisions,which makes change in the rotational and vibrational energies[15].

Another approximation has been proposed by Mason and Monchick[16]who assumed that the molecules have a fixed relative orientation,during a collision.The physical basis for this assumption is that most of the interaction in a collision occurs in the vicinity of the distance of the closest approach,during which the relative orientation does not change much,so that one relative orientation dominates in each collision.Therefore the Chapman-Enskog theory of polyatomic molecules retains its original form,but the collision integrals must be averaged over all possible orientations,occurring in collisions.

In the import antcase of dilute polargases,Mason and Monchick[17]applied this approximation,considering the facts that these molecules have an angle-dependent potential and the possible rotational-energy transfers on every collision must be taken into account.In order to calculate the collision integrals of some polar gases,they employed the Stock mayer potential as interaction potential and related it to the collision integrals Ω(l,s),using the following equations:

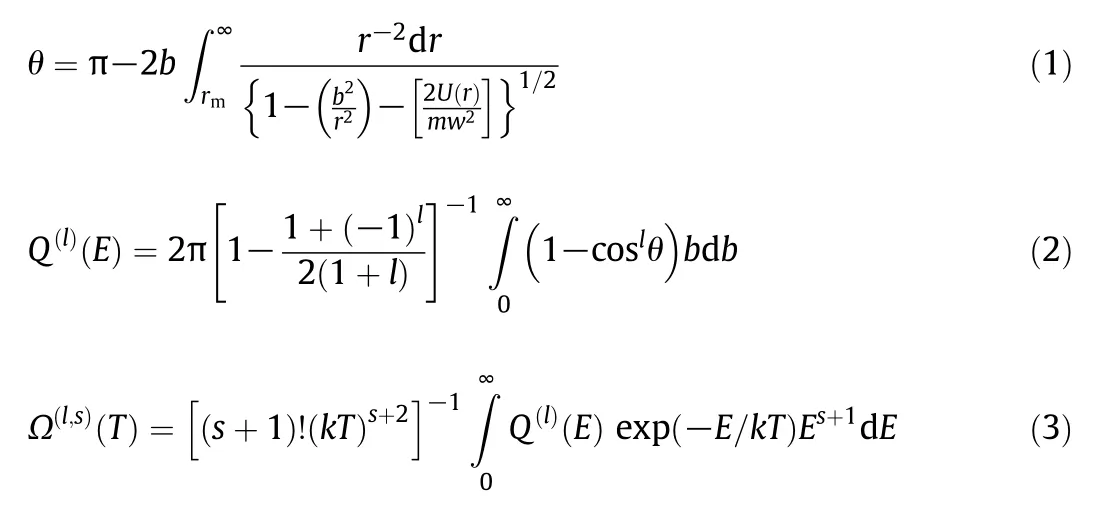
where θ is the classical deflection angle for U(r)interaction potential,b the impact parameter,rmthe distance of the closest approach in a collision,w the relative velocity of colliding partners and Q(l)(E)the collision cross section,corresponding to the l moment.They used the experimental viscosities and dipole moments of a number of polar gases to determine the potential parameters necessary to calculate other properties of these gases.Over-all agreement between the experiment and their model,makes the Stock mayer potential for polar gases,comparable to the Lennard-Jones(12-6)model for non-polar gases.It has been shown that effective total interaction energy between a polar molecule and non-polar molecule,has the same form as that of two non-polar molecules which are spherically symmetric[15],so in mixtures of polar and non-polar gases,the polar-nonpolar interactions have essentially a non-polar nature.

Ammonia has a widespread use in many industrial sectors and it is one of the chemicals with the largest production volume in the world.Its main uses are in fertilizers,industrial refrigeration systems,explosives,as well as in plastic and pharmaceutical product manufacturing[18].Furthermore ammonia is often the subject of experimental and theoretical studies,because of its ability to form hydrogen bonds and its simple symmetric molecular structure.

Reliable data for mixtures with ammonia are of special interest in the field of refrigeration.Furthermore,the correct description of the intermolecular interactions in such systems is a challenging task from a theoretical point of view due to the intra molecular inversion behavior of the ammonia molecule.

Recently,Papari et al.have applied the inversion method based on the law of corresponding states,to infer the intermolecular potential energies of non-polar polyatomic molecules[19,20]and their mixtures with noble gases[21-28],and predict their collision integrals,required to calculate transport properties.Their method was also applied to pure refrigerants[23,29]and refrigerant mixtures[30,31]as slightly polar molecules to obtain the effective and isotropic pair potential energies of this class of fluids.

The novelty of the present work is that the Papari et al.'s scheme is extended to a highly polar molecule ammonia and its mixtures with noble gases at low density.The numerical values of the inverted potentialenergies of ammonia-noble gas binary mixtures are then fitted to an analytical MSV model potential.The experimental viscosity,diffusion coefficient and thermal conductivity data,together with an iterative least squares method are used to obtain the best force parameters,ε12and σ12for each binary system.

Generated intermolecular potential energies are evaluated by comparison of our model potential with those obtained from other sources,both experimental and theoretical.In addition,the dilute gas transport coefficients(except thermal conductivity)of the studied mixtures,encompassing a wide range of temperatures,are predicted using the Chapman-Enskog version of the kinetic theory[9,10]and compared with the literature data.Computation of thermal conductivities for the studied mixtures through Schreiber et al.method[32]and comparing the results with literature data,provide a further test for the inverted potential energy functions.

2.Transport Properties of Gaseous Mixtures

Expressions for the viscosity η,thermal conductivity λ,and diffusion coefficient D of pure substances are given in Ref.[19].The mixture transport properties of dilute gases are obtained by the following relationships:

2.1.Viscosity

The viscosity of an arbitrary multicomponent mixture of dilute gases can be evaluated from the following equations:

where

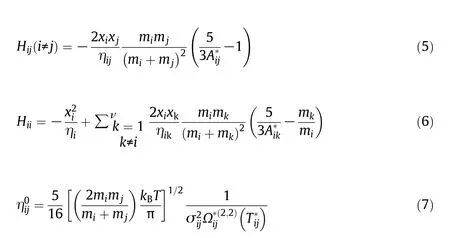
whereis the ratio of the collision integrals,defined by Eq.(11)in Ref.[19],xithe mole fraction of component i,and mithe molecular mass of the component i.kBT is the molecular thermal energy,σ the distance at which the potential energy is zero,andthe interaction viscosity.Subscripts i and j denote the heavier and lighter components of the i-j pair,respectively.

2.2.Diffusion

Diffusion in multicomponent mixtures is entirely described in terms of binary diffusion coefficients,Dij[15]:

where P is the pressure and Δijis a higher order correction term of the binary diffusion coefficient,which can be defined as:

where

2.3.Thermal diffusion factor

A tendency of a convection-free mixture to separate under a thermal(or temperature)gradient is known as the thermal diffusion process or Soret effect.In this process,the transport of matter takes place(i.e.a concentration gradient of the mixture constituents develops)with the temperature gradient.The thermal diffusion coefficient is the measure of this effect,and its sign determines the direction of the thermal diffusion[33].

The Chapman-Enskog expression for the thermal-diffusion factor,αT,of a binary mixture is:

where kTis a higher order correction term for the thermal-diffusion factor.This term is usually negligible compared with experimental uncertainties in αT.The corresponding formulas of quantities S1,ϱ1,and ϱ12in Eq.(13)can be found in Ref.[31],Eqs.(23)-(25).

The expressions for S2andϱ2are obtained from those ofS1andϱ1by interchanging subscripts 1 and 2.The sign convention for αTrequires that subscript 1 denotes the heavier component.

2.4.Thermal conductivity

The dependence of thermal conductivity of polyatomic molecular gases,on the internal degrees of freedom and the inherent details of inelastic collisions,prevents the application of Chapman Enskog theory for the calculation of this property.In this respect,the thermal conductivity of a multicomponent polyatomic gas mixture at zero density can be expressed in the form analogous to that for a mixture,consisting of monatomic species[32]:

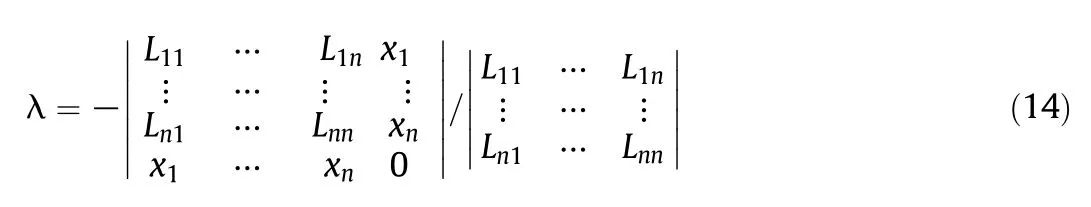
where,xiis the mole fraction of species i and the symbolλ,indicates the fullformal first-orderkinetic theory result,obtained by means of expansion in Thijsse basis vectors[34].Studies have shown that accurate and relatively simple expressions for pure polyatomic gases[35],atom-diatom mixtures[36],and atom-molecule mixtures[37]can be obtained by means of the Thijsse approximation,which identifies the total energy,as the dominant factor in determining thermal conductivity.

Employing the Thijsse et al.[34]and spherical approximations to the full results,gives rise to the following determinant elements,Lij[32]:

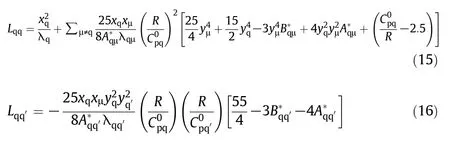
where λqis the thermal conductivity of pure molecular species q,λqq′,is the interaction thermal conductivity,is the ideal-gas isobaric heat capacity of q,R is the gas constant,and the quantities A*and B*are ratios of effective cross-sections,given by Eqs.(11)and(14)in Ref.[19].In addition yqis the mass ratio of species q,given by:

Here,Mqis the relative molecular mass of species q.The interaction thermal conductivity can be related to the more readily available interaction viscosity,ηqq′,through the equation below:

Inspection of above equations reveals that in order to obtain the thermal conductivity of a multi-component polyatomic gas mixture,knowledge of the thermal conductivity and the isobaric heat capacity of each of the pure species is required.This information is readily available for a large number of fluids as a function of temperature,either in terms of correlations or directly from experimental information.Three binary interaction parameters,namely,ηqq′,A*and B*,as functions of temperature,are the remaining required quantities which in the present work correspond to the interaction viscosity and collision integral ratios,obtained from the inversion method.

3.Results and Discussio n

Detailed iterative inversion technique was employed in this study to define the intermolecular pair interaction potential energies of five NH3-noble gas mixtures,from corresponding states correlations of viscosity.Implementation of the full inversion procedure,requires the extension of experimental data over as wide temperature range as possible.To achieve this,we considered the corresponding states correlation for functional viscosity collision integrals,Ω*(2,2)of noble gases proposed in Ref.[38],together with the one given for ammonia in Ref.[17],to obtain the mixed interaction functional〈Ω*(2,2)〉ijfor NH3-noble gas mixtures by employing the following simple combining rule:

Resulting 〈Ω*(2,2)〉ijvalues were employed to perform the inversion procedure in order to generate effective interaction potentials for considered systems.The inverted potential energy values were then fitted to an analytical multi-parameter MSV potential model.This model potential includes a Morse function and the van der Waals dispersion expansion that is respectively used for short range and long range interactions,along with a Spline function responsible for joining them smoothly.

The reduced form of MSV potential function reads as:

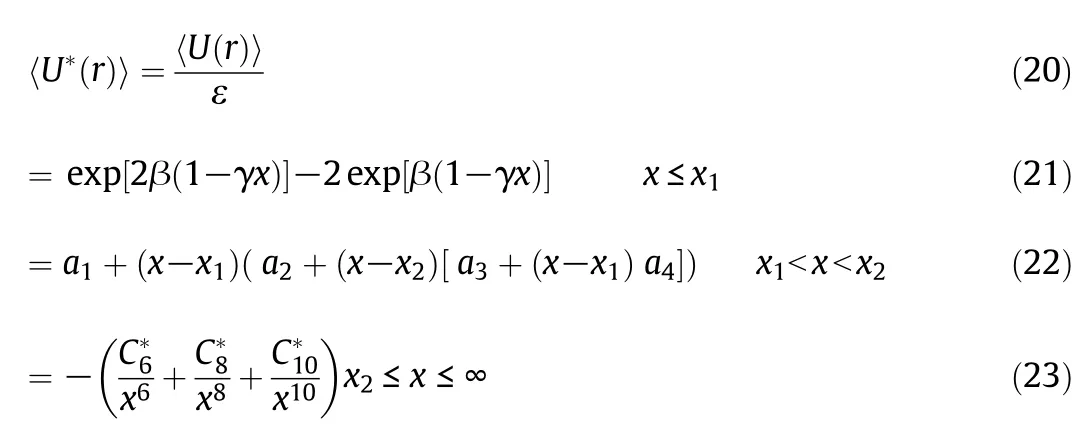
where=r/σ,x1=1.071444,and x2=1.405981.

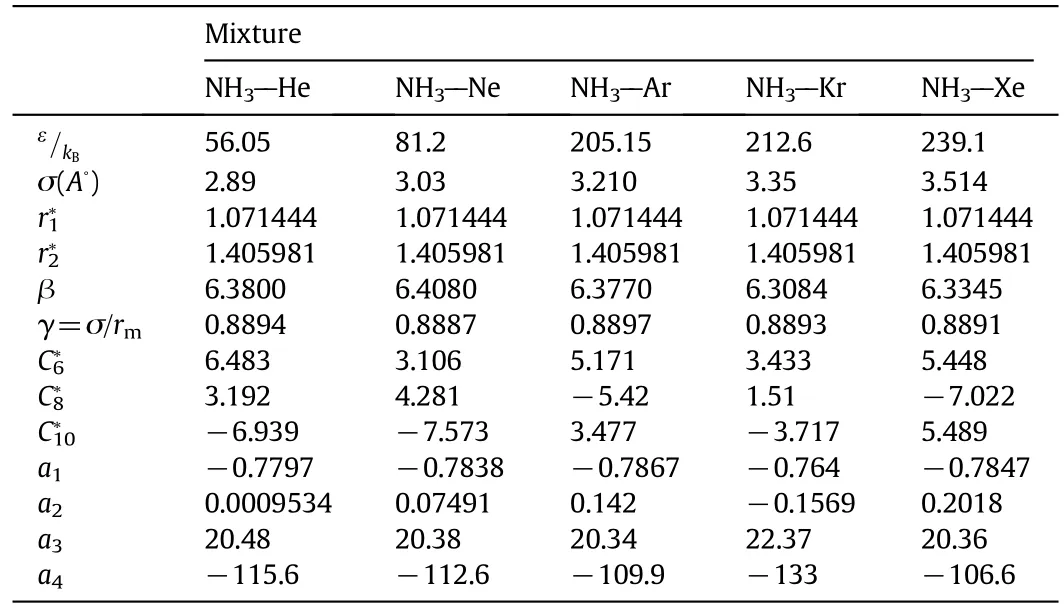
Table 1 Calculated parameters of MSV potential function(Eqs.(24)-(27))for NH3-noble gas mixtures

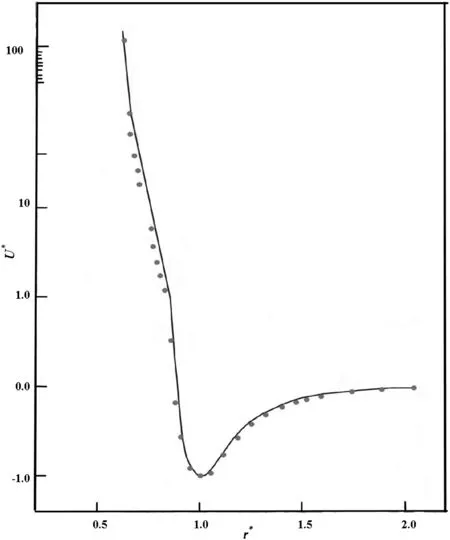
Fig.1.Reduced MSV potential model of NH3--Ne mixture(●)obtained by inversion of reduced viscosity collision integrals compared with improved Lennard-Jones(ILJ)potential model of Pirani et al.[39](―).

A nonlinear least squares method was performed for all studied binary mixtures,where the outcome of the fittings has been reported in Table 1.Our model potentials for mixtures of NH3with Ne,Ar,He and Krhave been compared with those obtained from molecular beam scattering measurements and infrared spectroscopic observations[39]in Figs.1 and 2 and S1 and S2 of supplementary data,respectively.The acceptable accordance,confirms the validity of our inverted potential energy functions for aforementioned mixtures.

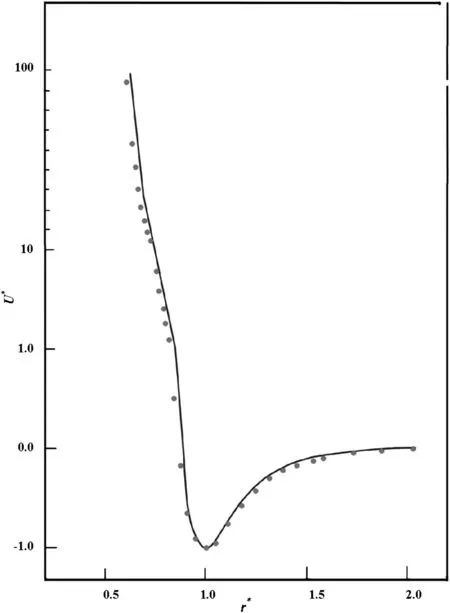
Fig.2.Reduced MSV potential model of NH3--Ar mixture(●)obtained by inversion of reduced viscosity collision integrals compared with improved Lennard-Jones(ILJ)potential model of Pirani et al.[39](―).

As a further test to assess the reliability of our proposed potential models for the present systems,mixture transport properties in low density region were computed.To achieve this,the effective potential energies obtained from the inversion method were used to perform the integration over the whole range and,in turn,to evaluate the improved kinetic theory collision integrals over the given range,using Eqs.(1)-(3).The results for the most commonly needed collision integrals and theirratios,obtained from Eqs.(11)-(15)in Ref.[19],for binary mixtures of NH3--Ne,NH3--Ar,NH3--He,NH3--Kr and NH3--Xe have been tabulated in Tables 2 and 3 and S1,S2 and S3 of supplementary material,respectively.

To calculate mixture transport properties,we needed to know the binary potential parameters,σijandεijof studied mixtures.Unlike interaction parameters characterizing binary interactions can be obtained from like parameters,σii,εiiand σjj,εjjby means of the combining rules.In this study,in order to obtainε12andσ12for NH3-noble gas mixtures,we employed experimental viscosity,diffusion coefficient and thermal conductivity data as reference properties.Our determination method of effective pair potential parameters,involved minimizingthe relative root mean square deviations(RMSD's)between reference transport properties and those predicted through Chapman-Enskog expressions for calculating viscosity,diffusion and thermal conductivity of binary mixtures.(Eqs.(24)-(26)).In fact,the leasts quares fitting method was employed to determine most consistent potential parameters,able to reproduce all mentioned transport properties within experimental accuracies.

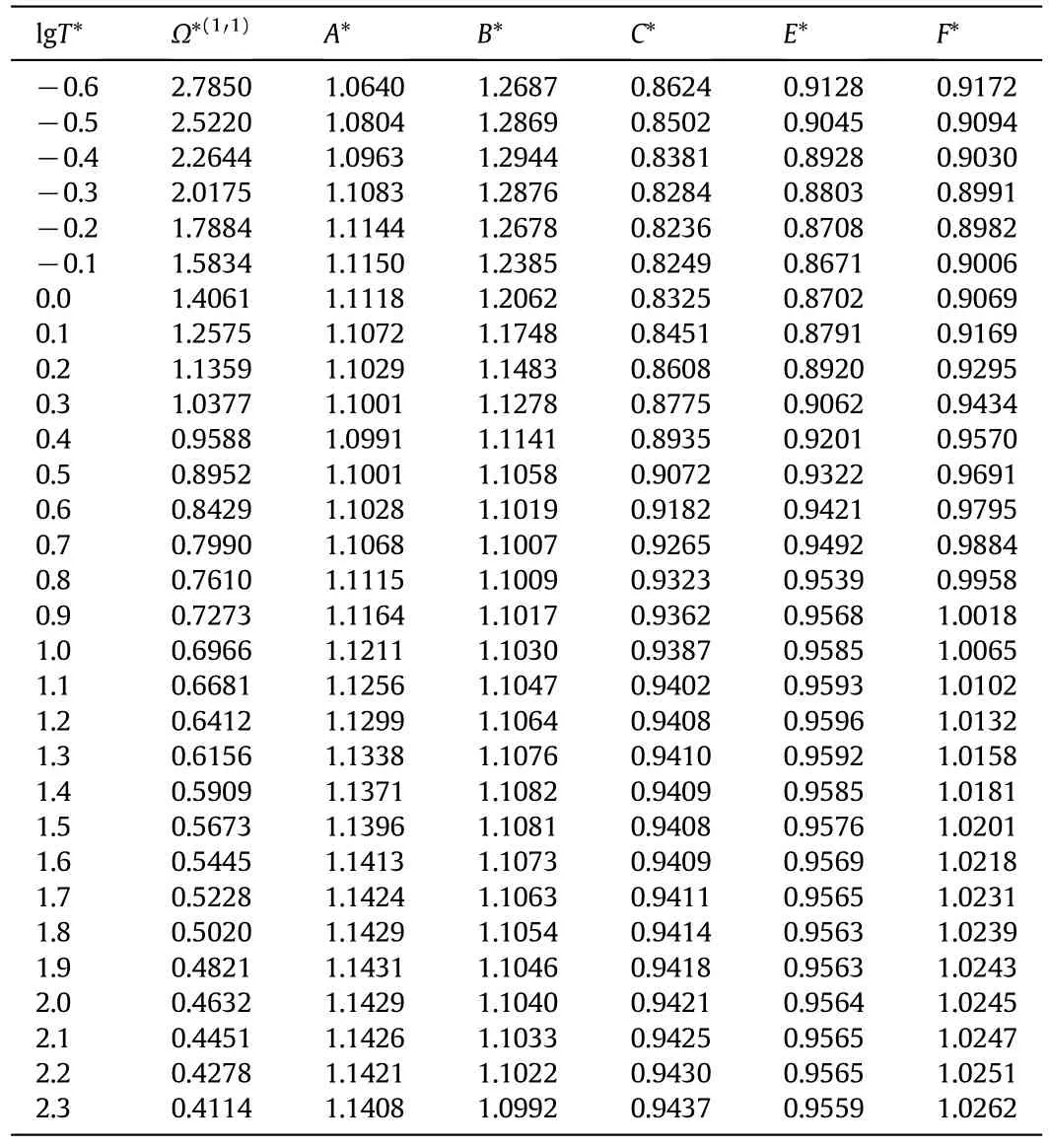
Table 2 The reduced collision integrals and their ratios for NH3--Ne mixture

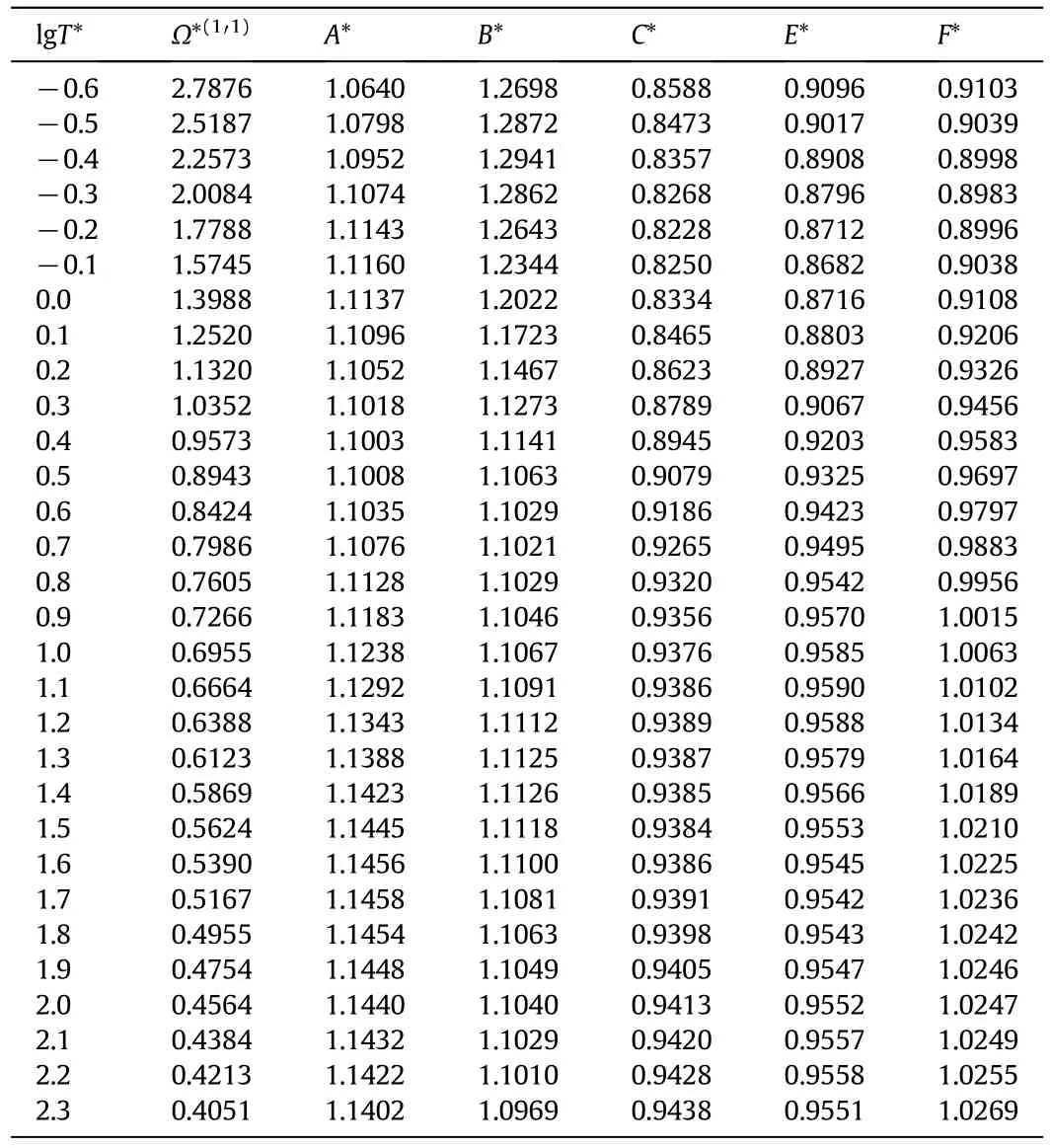
Table 3 The reduced collision integrals and their ratios for NH3--Ar mixture

Our predicted potential parameters,obtained from the above procedure have been reported in Table 4.For comparison we have added the same parameters from two references[40,41],calculated merely based on the viscosity and diffusion coefficients,respectively.

Table 4 Potential parameters(ε12 and σ12)of studied mixtures

As previously mentioned,the validity of any potential energy function is confirmed by its ability to reproduce thermo physical properties,within acceptable accuracies.Having the binary potential parameters,we employed the inverted pair potential energies of all five systems to the Chapman-Enskog[9,10]method to compute viscosity coefficients,binary diffusion coefficients,and thermal diffusion factors of equimolar NH3-noble gas mixtures at low density.

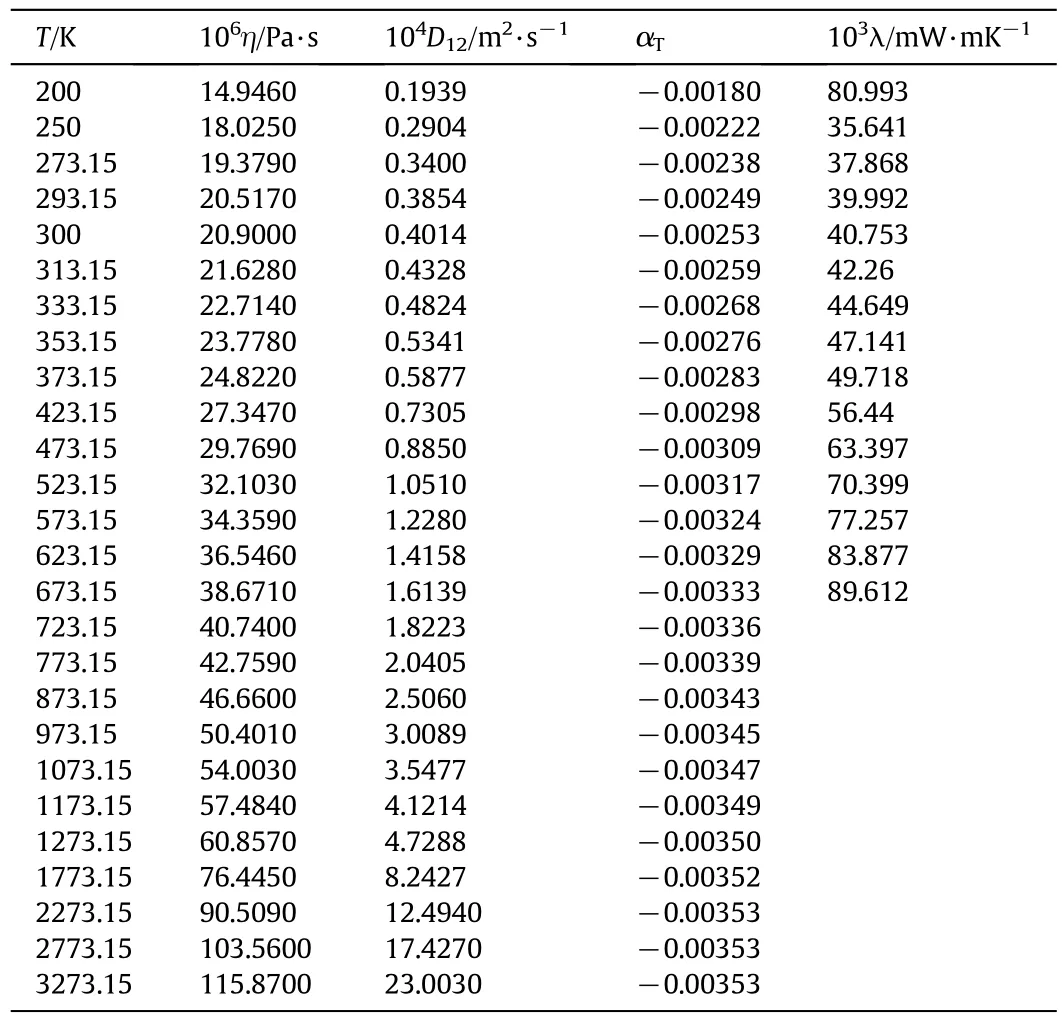
Table 5Predicted low density transport properties of equimolar NH3--Ne mixture

Moreover,the thermal conductivities of aforementioned binary gas mixtures were obtained using the Schreiber et al.method[33].The outcome of these computations for NH3--Ne,NH3--Ar,NH3--He,NH3--Kr and NH3--Xe mixtures,in a wide temperature range 250≤T≤3273.15 K,wastabulated respectively in Tables 5 and 6 and S4,S5 and S6 ofsupplementary material.

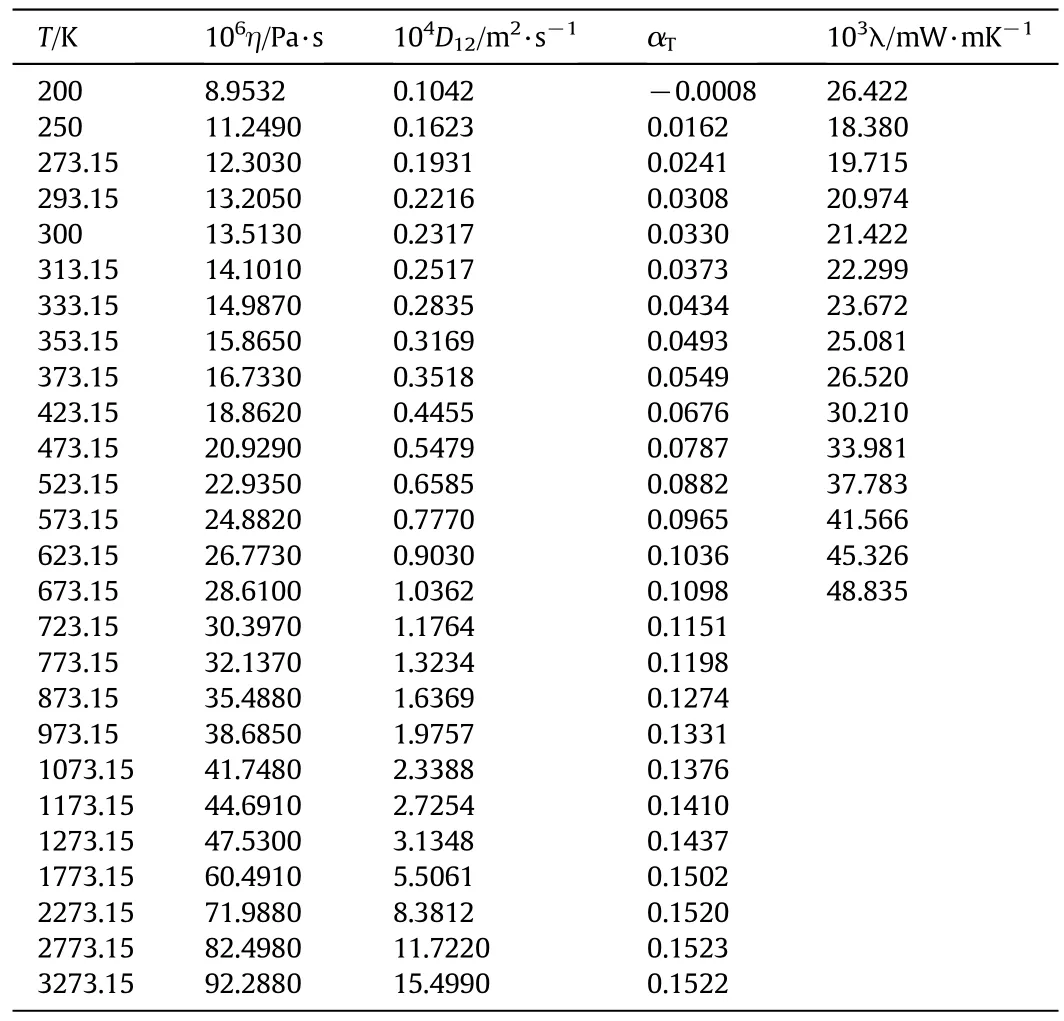
Table 6 Predicted low density transport properties of equimolar NH3--Ar mixture

Table 7 The non-linear least squares parameters,standard errors(SE)and correlation coefficients(R)of Eq.(27)

The interaction viscosity of dilute gas mixtures,η12,which is the hypothetical viscosity of a gas of mass μ=m1m2/(m1+m2)in which only unlike interactions take place,was obtained through using Eq.(7)and our model potential energies.The quantity was correlated for all five studied mixtures with the following equation:

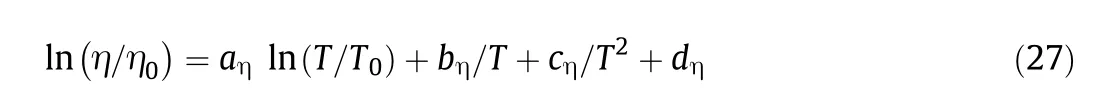
where,η0=1 μPa·s,and T0=1 K.

Further,the binary diffusion coefficients,thermal diffusion factors and also thermal conductivities of the five equimolar NH3-noble gas mixtures were correlated with Eqs.(27)-(30),respectively.

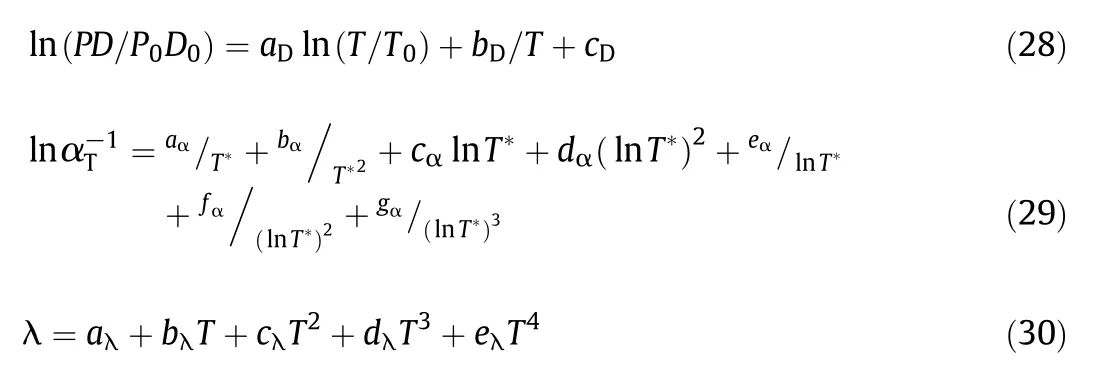
where P0=101325 Pa and D0=1 cm2·s-1.Parameters of the Eqs.(27)-(30)were allowed to vary for all five systems,using nonlinear least-squares method and listed in Tables 7-9 and S7 of supplementary data.

In order to assess the reliability of predicted transport properties and also our inverted potential energy functions,resulting transport properties were checked against the literature data.Calculated viscosities for the NH3--Ne,--Ar,--He,--Kr and--Xe mixtures were compared with experimental data[42,44-46]and the results depicted as deviation plots,in Figs.3 and 4 and S3-S5 of supplementary material,respectively.The average absolute deviations(AADs)of the calculated viscosities for aforementioned mixtures,from the experimental ones[42,44-46]were found to be 3.73%,3.57%,2.72%,4.79%and 2.68%,respectively.

Fig.3.Deviation plot for the dilute viscosity coefficients of NH3--Ne gaseous mixture in terms of temperature at different mole fractions of Ne:()x Ne=0.1;()x Ne=0.15;()x Ne=0.25;()x Ne=0.35;()x Ne=0.45;()x Ne=0.55;()x Ne=0.65;()x Ne=0.75;()x Ne=0.85;()x Ne=0.95 compared with experiment[42,44].

Table 9 The non-linear least squares parameter,standard errors(SE)and correlation coefficients(R)of Eq.(30)

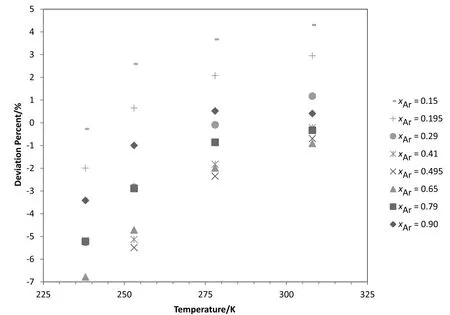
Fig.4.Deviation plot for the dilute viscosity coefficients of NH3--Ar gaseous mixture in terms of temperature at different mole fractions of Ar:()x Ar=0.15;()x Ar=0.195;()x Ar=0.29;()x Ar=0.41;()x Ar=0.495;()x Ar=0.65;()x Ar=0.79;()x Ar=0.90,compared with experiment[42,44-46].

It should be mentioned that these viscosity measurements have been performed,using an oscillating disk viscometer at atmospheric pressure and in the nominal temperature range,from-35 °C to 35 °C.The accuracy of the measurements has been claimed to be of 1%for NH3--He,--Ne,--Kr and--Xe mixtures.In the case of NH3--Ar mixture,the precision of measured viscosities by Rakshit et al.[46]is 1%at-35 °C to 35 °C temperature range,and it varies from 0.2%for argonrich to 1.5%for ammonia-rich mixture,in Iwasaki et al.reported data[45].

Predicted diffusion coefficients of ammonia-noble gas mixtures at several temperatures have been compared with experimental ones reported in the literature[43].Relative deviations of the diffusion coefficients,for NH3--Ar and NH3--Kr mixtures from experimental data[43]are plotted in Fig.5.The AADs for these mixtures were found to be 7.39%and 8%,respectively.Diffusion coefficients for mixtures of ammonia with argon and krypton have been measured using two-bulb technique in the temperature range of-20 to 60°C where the accuracy has been claimed to be within±1%.

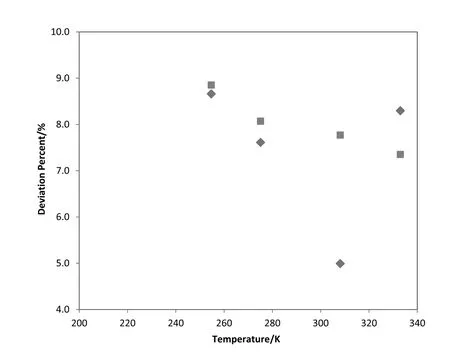
Fig.5.Deviation plot for the diffusion coefficients of NH3--Ar and NH3--Kr gaseous mixtures at different temperatures,compared with literature data[43]:()NH3--Ar,()NH3--Kr.

The thermal diffusion factor describes,physically,the actual extent of the spatial separation of an initially uniform gaseous mixture of different molecular partners,which takes place whenever that mixture is subject to a temperature gradient.Unfortunately we have not found any literature values of this property for the studied mixtures.Therefore,we could not evaluate our calculated thermal diffusion factors using the MSV model potential.

Thermal conductivities and heat capacities of pure species required by the Schreiber et al.'s method[32],were taken from NIST data[47].In order to assess the validity of predicted thermal conductivities of NH3-noble gas binary mixtures,Figs.6 and 7 and S6 of supplementary data depict the relative deviations of the calculated thermal conductivities of,NH3--Ne,NH3--Arand NH3--He mixtures from the experimental values[48],respectively.It should be cited that the hot-wire method has been used in this reference[48]to measure the thermal conductivities of aforementioned mixtures with the experimental uncertainty within 1%-1.5%at temperatures,39-199.6°C.The AAD values of our calculated thermal conductivities for NH3--He,NH3--Ne and NH3--Ar mixtures from the experimental values(each for 20 data points)were found to be 7.57%,7.83%and 10.63%,respectively.

Fig.6.Deviation plot for the thermal conductivities of NH3--Ne gaseous mixture at different temperatures,compared with experiment[48]:()x NH3=0.194;()x NH3=0.396;()x NH3=0.614;()x NH3=0.715.

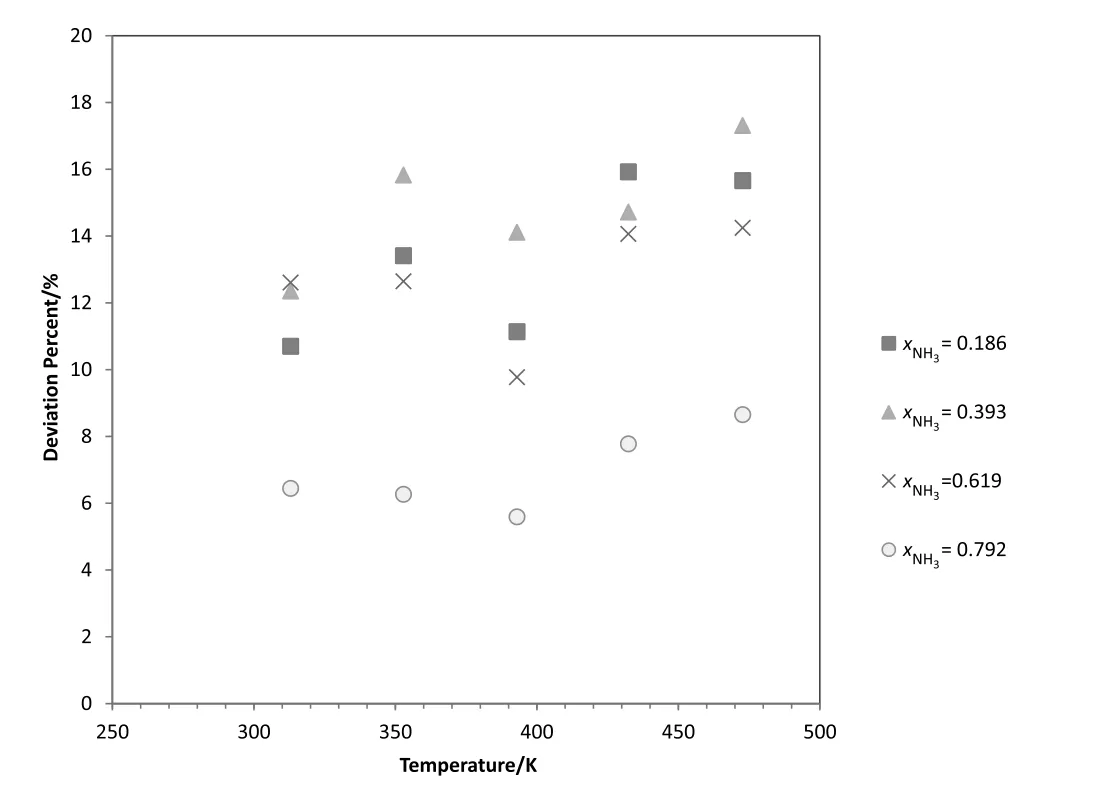
Fig.7.Deviation plot for the thermal conductivities of NH3--Ar gaseous mixture at different temperatures,compared with experiment[48]:()x NH3=0.186;()x NH3=0.393;()x NH3=0.619;()x NH3=0.792.

4.Conclusions

In this study the pair interaction energies of NH3-noble gas mixtures were obtained using the inversion method,based on the corresponding states correlations of viscosity,and fitted to the MSV model potentials.Our inverted potential functions showed reasonable agreement with previously obtained potential energies,based on the molecular beam scattering measurements and infrared spectroscopic observations.

Viscosities,diffusion coefficients,thermal diffusion factors and thermal conductivities of studied mixtures were calculated at low density and compared with literature data,where available.The acceptable agreement between calculated and experimental transport properties,provided further evidence to support the reliability of our inverted potentials for NH3-noble gas mixtures.The most important features of the inversion method are its independence on the explicit assumption about the functional form of potential model,the establishment of unique potentials,and leading to collision integrals which are prerequisite for determination of thermo physical properties.

Supplementary Material

Supplementary data to this articlecan be found online at http://dx.doi.org/10.1016/j.cjche.2017.07.007.

[1]H.Feng,X.Liu,W.Gao,X.Chen,J.Wang,L.Chen,H.-D.Lüdemann,Evolution of self diffusion and local structure in some amines over a wide temperature range at high pressures:a molecular dynamics simulation study,Phys.Chem.Chem.Phys.12(45)(2010)15007-15017.

[2]M.Mukhopadhyay,Natural Extracts Using Supercritical Carbon Dioxide,CRC Press,2000.

[3]G.Maitland,M.Rigby,E.Smith,W.Wakeham,D.Henderson,Intermolecular Forces:Their Origin and Determination,Physics Today 36(1983)57-58.

[4]I.A.McLure,J.E.Ramos,F.del Río,Accurate effective potentials and virial coefficients in real fluids.1.Pure noble gases and their mixtures,J.Phys.Chem.B 103(33)(1999)7019-7030.

[5]G.Maitland,E.Smith,The intermolecular pair potential of argon,Mol.Phys.22(5)(1971)861-868.

[6]D.Gough,G.Maitland,E.Smith,The direct determination of intermolecular potential energy functions from gas viscosity measurements,Mol.Phys.24(1)(1972)151-161.

[7]D.Gough,E.Smith,G.Maitland,The pair potential energy function for krypton,Mol.Phys.27(4)(1974)867-872.

[8]G.Maitland,W.Wakeham,Direct determination of intermolecular potentials from gaseous transport coefficients alone:part II.Application to unlike monatomic interactions,Mol.Phys.35(5)(1978)1443-1469.

[9]S.Chapman,T.Cowling,The Mathematical Theory of Non-uniform Gases,Camb.Univ.Press,1939.

[10]D.Enskog,Kinetische Theorie der Vorgänge in mässig verdünnten Gasen,1917.

[11]R.A.Aziz,V.Nain,J.Carley,W.Taylor,G.McConville,An accurate intermolecular potential for helium,J.Chem.Phys.70(9)(1979)4330-4342.

[12]M.L.Klein,R.A.Aziz,Inert Gases:Potentials,Dynamics,and Energy Transfer in Doped Crystals,vol.34,Springer-Verlag,1984.

[13]M.J.Slaman,R.A.Aziz,Transport properties and second virial coefficients for neon,Chem.Eng.Commun.104(1-3)(1991)139-152.

[14]R.Eggenberger,S.Gerber,H.Huber,D.Searles,Ab initio calculation of the second virial coefficient of neon and the potential energy curve of Ne2,Chem.Phys.156(3)(1991)395-401.

[15]J.O.Hirschfelder,C.F.Curtiss,R.B.Bird,M.G.Mayer,Molecular Theory of Gases and Liquids,vol.26,Wiley,New York,1954.

[16]L.Monchick,E.Mason,R.Munn,F.J.Smith,Transport properties of gaseous He3and He4,Phys.Rev.139(4A)(1965)A1076.

[17]L.Monchick,E.Mason,Transport properties of polar gases,J.Chem.Phys.35(5)(1961)1676-1697.

[18]A.Fenghour,W.A.Wakeham,V.Vesovic,J.Watson,J.Millat,E.Vogel,The viscosity of ammonia,J.Phys.Chem.Ref.Data 24(5)(1995)1649-1667.

[19]M.M.Papari,Transport properties of carbon dioxide from an isotropic and effective pair potential energy,Chem.Phys.288(2)(2003)249-259.

[20]J.Moghadasi,M.M.Papari,A.Nekoie,J.V.Sengers,Transport properties of some polyatomic gases from isotropic and effective pair potential energies(part II),Chem.Phys.306(1)(2004)229-240.

[21]M.M.Papari,D.Mohammad-aghaiee,B.Haghighi,A.Boushehri,Transportproperties of argon-hydrogen gaseous mixture from an effective unlike interaction,Fluid Phase Equilib.232(1)(2005)122-135.

[22]M.M.Papari,D.Mohammad-Aghaie,J.Moghadasi,A.Boushehri,Semi-empirically based assessment for predicting dilute gas transport properties of F2 and Ar-F2 fluids,Bull.Chem.Soc.Jpn.79(1)(2006)67-74.

[23]B.Haghighi,F.Heidari,B.Haghighi,M.M.Papari,B.Haghighi,Prediction of thermal conductivity of R32,R125,R134a,R143a and R152a at zero density via semiempiric ally-based assessment,Int.J.Air-Cond.Refrig.19(01)(2011)45-56.

[24]J.Moghadasi,M.M.Papari,F.Yousefi,B.Haghighi,Transport coefficients of natural gases,J.Chem.Eng.Jpn 40(9)(2007)698-710.

[25]J.Moghadasi,F.Youse fi,M.M.Papari,M.A.Faghihi,A.A.Mohsenipour,Transport properties in mixtures involving carbon dioxide at low and moderate density:test of several intermolecular potential energies and comparison with experiment,Heat Mass Transf.45(11)(2009)1453-1466.

[26]S.Nikmanesh,J.Moghadasi,M.M.Papari,Calculation of transport properties of CF4+noble gas mixtures,Chin.J.Chem.Eng.17(5)(2009)814-821.

[27]M.M.Papari,J.Moghadasi,S.Nikmanesh,E.Hosseini,A.Boushehri,Modeling thermo physical properties of noble gas involved mixtures,Int.J.Comput.Methods Sing.8(01)(2011)19-39.

[28]D.Mohammad-Aghaie,M.M.Papari,F.Zargari,Modeling transport properties of N2-noble gas mixtures at low and moderate densities,Bull.Chem.Soc.Jpn.85(5)(2012)563-575.

[29]J.Moghadasi,M.M.Papari,D.Mohammad-Aghaie,A.Campo,Gas transport coefficients of light hydrocarbons.Halogenated methane and ethane as candidates for new refrigerants,Bull.Chem.Soc.Jpn.81(2)(2008)220-234.

[30]J.Moghadasi,D.Mohammad-Aghaie,M.Papari,Predicting gas transport coefficients of alternative refrigerant mixtures,Ind.Eng.Chem.Res.45(26)(2006)9211-9223.

[31]J.Moghadasi,D.Mohammad-Aghaie,M.M.Papari,M.A.Faghihi,Predicting gas transport properties of light hydrocarbon mixtures as candidates for new refrigerants,High Temp.High Pressures 37(4)(2008)299-316.

[32]M.Schreiber,V.Vesovic,W.Wakeham,Thermal conductivity of multicomponent polyatomic dilute gas mixtures,Int.J.Thermophys.18(4)(1997)925-938.

[33]K.Shukla,A.Firoozabadi,Anew model of thermal diffusion coefficients in binary hydrocarbon mixtures,Ind.Eng.Chem.Res.37(8)(1998)3331-3342.

[34]B.Thijsse,G.t.Hooft,D.Coombe,H.Knaap,J.Beenakker,Some simplified expressions for the thermal conductivity in an external field,Phys.A 98(1-2)(1979)307-312.

[35]J.Millat,V.Vesovic,W.Wakeham,On the validity of the simplified expression for the thermal conductivity of Thijsse et al,Phys.A 148(1)(1988)153-164.

[36]V.Vesovic,W.Wakeham,Practical,accurate expressions for the thermal conductivity of atom-diatom gas mixtures,Phys.A 201(4)(1993)501-514.

[37]M.Schreiber,V.Vesovic,W.A.Wakeham,Thermal conductivity of atom-molecule dilute gas mixtures,High Temp.High Pressures 29(6)(1997)653-658.

[38]B.Naja fi,E.Mason,J.Kestin,Improved corresponding states principle for the noble gases,Phys.A 119(3)(1983)387-440.

[39]F.Pirani,L.F.Roncaratti,L.Belpassi,F.Tarantelli,D.Cappelletti,Molecular-beam study of the ammonia-noble gas systems:characterization of the isotropic interaction and insights into the nature of the intermolecular potential,J.Chem.Phys.135(19)(2011)194301.

[40]J.E.Ramos,F.del Río,I.A.McLure,Nonc on formal potentials and second virial coefficients in molecular fluids.II.Applications to nonspherical molecules,J.Phys.Chem.B 102(51)(1998)10576-10585.

[41]F.del Río,J.E.Ramos,A.Gil-Villegas,I.A.McLure,Collision diameters,interaction potentials,and virial coefficients of small quasi-spherical molecules,J.Phys.Chem.100(21)(1996)9104-9115.

[42]A.Rakshit,C.Roy,Viscosity and polar-nonpolar interactions in mixtures of inert gases with ammonia,Physica 78(1)(1974)153-164.

[43]B.Srivastava,I.Srivastava,Studies on mutual diffusion of polar-nonpolar gas mixtures,J.Chem.Phys.36(10)(1962)2616-2620.

[44]K.Stephan,T.Heckenberger,Thermal Conductivity and Viscosity Data of Fluid Mixtures:Tables,Diagrams,Correlations,and Literature Survey,vol.10,Scholium International,1988.

[45]H.Iwasaki,J.Kestin,A.Nagashima,Viscosity of argon—ammonia mixtures,J.Chem.Phys.40(10)(1964)2988-2995.

[46]A.Rakshit,C.Roy,A.Barua,Viscosity of the binary gas mixtures argon-methane and argon-ammonia,J.Chem.Phys.59(7)(1973)3633-3638.

[47]E.Lemmon,M.McLinden,D.Friend,P.Linstrom,W.Mallard,NIST Chemistry WebBook,Nist Standard Reference Database Number 69,National Institute of Standards and Technology,Gaithersburg,2011.

[48]B.Srivastava,A.D.Gupta,Thermal conductivity of binary mixtures of ammonia and inert gases,Br.J.Appl.Phys.18(7)(1967)945.