A Study on Connector Loads of Very Large Floating Structures near Islands and Reefs

LI Zhi-wei,ZHANG Zheng-wei,TIAN Chao,DING Jun

(China Ship Scientific Research Center,Wuxi 214082,China)

A Study on Connector Loads of Very Large Floating Structures near Islands and Reefs

LI Zhi-wei,ZHANG Zheng-wei,TIAN Chao,DING Jun

(China Ship Scientific Research Center,Wuxi 214082,China)

The variable seabed effect becomes significant for the floating structures deployed near island and reefs.In this paper,the Rigid Module Flexible Connector(RMFC)model is adopted with the seabed treated as a fixed body to study the influence of uneven seabed on the motion response and connector loads of Very Large Floating Structure(VLFS)near island and reefs.The numerical results indicate that the uneven seabed has a significant influence on the diffraction force and the connector loads are enlarged in low wave frequencies.

variable seabed bathymetry;VLFS;RMFC model;connector loads

0 Introduction

The effect of variable seabed bathymetry becomes significant in shallow water regions. Therefore,the influence of uneven seabed should be taken into account for the analysis of motion response and connector loads of VLFS deployed near island and reefs.A new kind of floating platform with horizontal pontoon[1]was put forward based on the fact that wave propagates perpendicular to the shoreline near islands.The motion response and connector loads of VLFS consisting of three such modules under both even and uneven seabed conditions are studied with RMFC model and complex seabed treated as a fixed body.The results of motion response are compared with experimental data to verify the theoretical model.The influence of uneven seabed on the diffraction and radiation of floating structures is investigated through numerical simulation.

1 Basic theory

In this paper,the module stiffness is assumed to be much larger than the connector stiffness.Therefore the RMFC model,namely Rigid Module Flexible Connector model is applied to study the connector loads of very large floating structures.This model has been studied andused by researchers home and abroad[2-4]which is proved to be reliable and robust.The variable seabed is treated as a fixed body to take its influence on the diffraction and radiation of floating structures into account[5].

1.1 RMFC model

In this model,each module is assumed to be rigid with six degrees of freedom,namely surge,sway,heave,roll,pitch and yaw,which can be expressed by the following vector：

The relative translational motions of adjacent modules are restricted by two connectors between them while the relative rotational motions are free.The i-th link point of the connector is on the k-th module and its motions have the following expression：

where i and j represent the link points of the i-th connector on module k and k+1 respectively.

The translational forces on the modules caused by the connectors also lead to bending moments respect to the module gravity center.Therefore,the connector caused loads on the k-th and k+1-th modules are：

where Kcis the three translational stiffness of the connector.Therefore,the stiffness matrix between adjacent modules in global coordinate system can be obtained from Eqs.(4-5)：

Thus,the structural stiffness matrix can be obtained by the assembly of stiffness matrixes between adjacent modules.

1.2 Treatment of uneven seabed

The uneven seabed is treated as a fixed body to account for its influence on the diffraction and radiation of floating structures.With the ideal fluid assumption adopted,the diffraction potential can be expressed as：

where φDis the time independent part of diffraction potential ΦDand Re is the real part of the variable in the bracket.The time independent part of diffraction potential φDsatisfies the following governing equation and boundary conditions.

The governing equation(Laplace equation)：

Linear free surface condition：

Body surface condition：

Uneven seabed condition：

Far field radiation condition：

The governing equation and boundary conditions of radiation potential are quite similar to those of diffraction potential with the body surface condition and uneven seabed condition substituted by the followings.

Body surface condition：

where njis the unit normal vector of the j-th mode.

Uneven seabes condition：

The Green function method is applied to solve the time independent part of diffraction and radiation potentials.

2 Model description

2.1 VLFS model

The VLFS in present study consists of three identical modules represented by M1 to M3 which are connected by four identical connectors represented by C1 to C4,as shown in Fig.1.

Fig.1 Sketch of VLFS

Each module is symmetric about XiOiZiplane and YiOiZiplane.The gap between two adjacent modules is 20 m at the upper deck where two connectors are located symmetrically about the X axis.Each connector is simplified as a spring with three translational stiffness Kx=Kz=2E9（ N/m） and Ky=1E9（ N/m） which is positioned 29 m from the module’s longitudinal center line and 24 m from the baseline of the module.

The hydrodynamic model of each module consists of 3 580 panel elements with the grid side length of about 3.5 m,as shown in Fig.2.

The main parameters of a single module are listed in Tab.1.

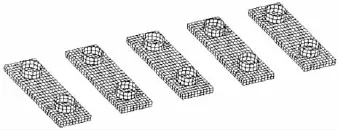
Fig.2 Hydrodynamic model of a single module

Tab.1 Main parameters of a single module

2.2 Seabed model

The seabed bathymetry near a typical island is adopted with an average water depth of 30 m.The layout of VLFS and hydrodynamic model of uneven seabed is shown in Fig.3.There is a shallow water region at the back side of VLFS with the water depth of only several meters.The water depth of the rest area varies from 20 m to 40 m.

The convergence analysis of uneven seabed size and grid side length is carried out similarly to Ref.[6].The seabed size is shown in Fig.3 with L representing the length of a single module,namely 300 m.The grid side length is 6 m and the uneven seabed is enclosed by sloped edges on the side and end[7-8].

3 Numerical results and discussion

3.1 Motion response

The motion responses of module one(M1)under even and uneven seabed conditions are plotted in Fig.4 together with experimental results to study the influence of variable seabedbathymetry on motion responses of VLFS.The water depth of even seabed condition is 30 m which is the average water depth of uneven seabed.

The motion responses under uneven and even seabed conditions are presented by solid lines with block and diamond symbols respectively in Fig.4 while the experimental results are given in circles[9].It is shown in Fig.4 that the heave and roll motions are little influenced by the uneven seabed and the numerical results are in good accordance with experimental results.With the existence of complex seabed,the fluid field becomes non-uniform and large yaw motion appears especially in low wave frequencies.The pitch motion is also obviously influenced by the uneven seabed and becomes larger than that under even seabed condition.

The hydrodynamic coefficients of pitch and yaw motion are calculated to further study the influence of uneven seabed.

The added mass and hydrodynamic damping of pitch and yaw motions under both even and uneven seabed conditions are plotted and compared in Fig.5.There is not much difference between results under different seabed conditions which means that the uneven seabed has only a little influence on the radiation force.

It is shown in Fig.6 that the diffraction force of pitch and yaw motions under uneven seabed condition is much larger than that under uniform water depth condition which means that the existence of complex seabed has an obvious influence on the diffraction force especially in low and mid wave frequencies.Therefore the motion response is enlarged by the diffraction force under uneven seabed condition compared with uniform water depth condition.

Fig.5 Added mass and hydrodynamic damping

Fig.6 Diffraction force

3.2 Connector loads

The connector loads can be calculated through the relative motion of adjacent modules and connector stiffness as mentioned in basic theory.

The previous study under uniform water depth condition shows[10]that the connector loads reach the peak around the wave angle of 85°which is much larger than that under the wave angle of 90°.The incident wave angle may be slight away from 90°due to some environmental disturbance.Therefore,the connector loads around the wave angle of 90°should be taken into consideration for structural safety of VLFS deployed near island and reefs.The numerical results under both seabed conditions are plotted and compared in Fig.7.

Fig.7 Transfer function of connector loads

It is shown in Fig.7 that the connector loads under both seabed conditions share some similar characters as follows：

(1)The connector load in X direction with that in Z direction next to it is much larger than the load in Y direction;

(2)The connector loads around the wave angle of 85°,namely 84°and 86°are much larger than those under the wave angle of 90°.

There is also some difference between the connector loads under different seabed conditions which is caused by the uneven seabed effects：

(1)The connector loads in low wave frequencies under uneven seabed condition are larg-er than those under even seabed condition,especially in Y direction.This is caused by the large yaw motion in low wave frequencies influenced by the variable seabed as shown in Fig.4;

(2)The connector loads under the wave angle of 90°are obviously influenced by the variable seabed bathymetry in low and mid wave frequencies.

4 Conclusions

The motion response and transfer function of connector loads of VLFS near island and reefs are numerically investigated in this paper with RMFC model and uneven seabed treated as a fixed body.The numerical results are compared with those under uniform water depth condition to investigate the influence of uneven seabed on motion responses and connector loads. The following conclusions can be drawn：

(1)Large yaw and pitch motions appear because of the non-uniform fluid field caused by the complex seabed,especially in low and mid wave frequencies;

(2)The diffraction force is significantly influenced by the uneven seabed while the added mass and hydrodynamic damping are quite similar with those under even seabed condition;

(3)The connector loads in low wave frequencies are enlarged by the existence of the complex seabed compared with that under uniform water depth condition.

The theory and results presented in the paper provide a technical foundation for the load determination and structure design of VLFS near island and reefs.Further improvement and development of basic theory and numerical method with the application of water wave functions would be carried out in the future study.

Acknowledgement

The study,partly reported in this paper,is supported by the National Basic Research Program of China(No.2013CB036100).

[1]Wu Xiaofeng.The preliminary design of a single module of very large floating structures[R].CSSRC,2013.

[2]Ertekin R C,Riggs H R,Che X L,Du S X.Efficient methods for hydroelastic analysis of very large floating structures[J]. Journal of Ship Research,1993,37(1)：58-76.

[3]Yu Lan,Li Runpei,Shu Zhi.Dynamic responses of mobile offshore base connectors[J].China Ocean Engineering,2003, 17(4)：469-479.

[4]Liu Chao,Qi Enrong,Lu Ye.Dynamic response of connectors of Very Large Floating Structures under shallow draft[J]. Journal of Ship Mechanics,2014,18(5)：581-590.

[5]Ni Xinyun,Tian Chao,Cheng Xiaoming,et al.The hydroelastic response of platform in complex seabed condition[J]. Journal of Ship Mechanics,accepted.

[6]Li Zhiwei,Ding Jun,Tian Chao,et al.Numerical study on hydroelastic responses of very large floating structures in variable seabed bathymetry[C]//In Proceeding of the Second Conference of Global Chinese Scholars on Hydrodynamics. Wuxi,2016,Accepted.

[7]Buchner B.The motions of a ship on a sloped seabed[C]//Proceeding of OMAE2006.Hamburg,Germany,2006：1-9.

[8]Ferreira M D,Newman J N.Diffraction effects and ship motions on an artificial seabed[C]//24th IWWWFB.Zelenogorsk, Russia,2009.

[9]Ma Xiaozhou,Ma Yuxiang.Model test report on hydrodynamic performance of very large floating structures near island and reefs[R].Dalian：Dalian University of Technology,2015.

[10]Li Zhiwei,Zhang Zhengwei,Lu Ye.A study on connector loads of VLFS based on RMFC model[R].Wuxi：CSSRC,2015.

近岛礁超大型浮体连接器载荷研究

李志伟，张正伟，田超，丁军
（中国船舶科学研究中心，江苏无锡214082）

对于布置在岛礁附近的浮体，复杂海底地形的作用不可忽略。文章基于RMFC模型，同时将地形作为固定物体，研究了复杂海底地形对布置于岛礁附近的超大型浮体的运功响应和连接器载荷的影响。数值计算结果表明，地形的存在对浮体绕射力存在显著的影响，同时增加了低频区域的连接器载荷。

变化地形；超大型浮体；RMFC模型；连接器载荷

TV131.2

：A

李志伟（1987-），男，中国船舶科学研究中心博士研究生；

1007-7294（2017）03-0329-10

TV131.2

：A

10.3969/j.issn.1007-7294.2017.03.008

张正伟（1985-），男，博士，中国船舶科学研究中心工程师；

田超（1978-），男，博士，中国船舶科学研究中心研究员；

丁军（1986-），男，中国船舶科学研究中心博士研究生。

Received date：2017-02-07

Foundation item：Supported by the National Basic Research Program of China(No.2013CB036100)

Biography：LI Zhi-wei(1987-),male,Ph.D.student of CSSRC,E-mail：lizhiwei@cssrc.com.cn; ZHANG Zheng-wei(1985-),male,Ph.D.engineer of CSSRC.