具有Markovian调制的随机资本系统数值解的收敛性

郑来运

(宁夏大学 机械工程学院， 银川 750021)



具有Markovian调制的随机资本系统数值解的收敛性

郑来运

(宁夏大学 机械工程学院， 银川 750021)

根据Euler数值方法，给出了一类具有Markovian调制的役龄相关随机资本系统的数值解，并应用It公式、Burkholder-Davis-Gundy不等式和Gronwall引理证明了数值解的收敛性，给出了数值解收敛于解析解的充分条件。

随机资本系统；Markovian调制；It公式

考虑如下具有Markovian调制的役龄相关随机资本系统：

(1)

带Markovian调制的系统具有很好的优点，它能描述(模型化)动力系统中结构的突然变化，如系统组成部分的失败或修复、突然的环境变化、子系统间互联的改变以及对不同的非线性部分的操作等[1]，因此受到了广泛关注[2-17]。由于一般很难或无法获得该系统的解析解，近年来，很多学者更加关注随机微分方程数值解的研究。例如：Yuan等[2]讨论了具有Markovian调制的随机微分方程 数值解的收敛性；Wang等[5]研究了带 Poisson 跳和 Markovian调制的随机时滞微分方程数值解的收敛性；Rathinasamy等[6]给出了带多时滞和Markovian调制的线性随机微分方程半隐式Euler法的均方稳定性，最近又讨论了具有Markovian调制的年龄相关随机种群系统分裂步数值方法[7]；Zhou等[8]证明了在局部 Lipschitz 条件下，带 Markovian调制的中立型时滞随机微分方程数值解的收敛性；Li等[9]讨论了带Markovian调制的随机时滞微分方程数值解的收敛性和稳定性，并研究了带Markovian调制[10]以及带跳和Markovian调制[11]的年龄相关随机种群方程数值解的收敛性；Jiang等[12]讨论了带Markovian调制的随机时滞积分微分方程分裂步向后Euler数值解的稳定性；张启敏等[13-14]研究了带Markovian调制的年龄相关随机种群系统数值解的渐渐稳定性以及半驯服Euler法的指数稳定性。对于投资模型问题，Markovian调制模型在金融经济学的多个重要领域均有应用[15-16]，但对于带Markovian调制的役龄相关随机资本系统，相关研究较少[17]。本文讨论给定条件下带Markovian调制的役龄相关随机资本系统数值逼近解的收敛性。

1　预备知识和Euler逼近

设(Ω,F,P)是一个完备概率空间，{Ft}t≥0是其上的一个滤子且满足一般性条件(即单调增右连续，且F0包含所有的P零测集)。设r(t),t>0为定义在(Ω,F,P)上取值于有限状态S={1,2,…,N}的右连续Markovian链，其生成元Γ=(γij)N×N定义如下：

其中，Δ>0，γij≥0(i≠j)表示从状态i到状态j的转移概率，且

(2)

其中，Kt=K(a,t)。

对于系统(1)，取Δt=h为离散时间步长(时间增量)，则其Euler逼近解迭代式为

(3)

初始值

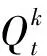
(4)

假定系统(1)满足如下条件：

1)μ(a,t)在Q上非负可测，γ(t)和A(t)在[0;T]上非负连续，满足

2)f(i,0)=0,g(i,0)=0,i∈S。

3) (Lipschitz条件)存在正常数Ki，对任意x,y∈H,i∈S，有

若上述条件成立，则方程(1)在(a,t)∈Q上存在唯一解K(a,t)，证明方法与文献[18]中方法类似，这里不再赘述。

2　相关引理

引理2若条件(A)-(D)成立，则存在常数k≥2和C1>0，使得

证明过程类似于文献[17]，且可得

证明由式(4)可得

对|Qt|2应用It公式，有

于是得

对∀t∈[0,T]，有

利用条件3)得

基于QoS综合匹配的语义Web服务选择方法过程中，两个QoS属性参数之间相关性的临界距离L，本文将其设定为1。QoS语义匹配成功后，对相应的QoS数值进行匹配。

(5)

应用Burkholder-Davis-Gundy不等式，对某些正常数M1>0，有

(6)

于是由式(5)和(6)可得

应用 Gronwall 引理，得

证明完毕。

引理4对任意的t∈[0,T]，存在正常数C3和C4，使得

引理5若条件1)～3)成立，则存在常数C5使

证明对任意的t∈[0,T]，存在正整数m，使得t∈[mh,(m+1)h)，有

于是，

应用Cauchy-Schwarz不等式和假设条件，有

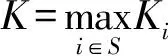
应用Doob不等式和引理3，有

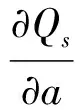
证明完毕。

3　数值解的收敛性

由引理1～5可证明在给定条件1)～4)下，具有Markovian调制的役龄相关随机资本系统的数值解收敛到其解析解。

定理1若条件1)～4)成立，则

证明由式(2)和(4)，有

于是，对∀t∈[0,T]，有

应用Burkholder-Davis-Gundy不等式，得

其中，k1和k2均为正常数。于是有

再利用Gronwall不等式，可得

即有

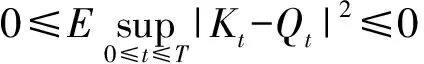
定理2若条件1)～4)成立，则数值逼近解(4)收敛于系统(1)的解析解，即满足

4　结束语

本文讨论了一种具有Markovian调制的役龄相关随机资本系统数值解的收敛性。结果表明：在相应条件下，系统的数值逼近解(4)收敛于其解析解。

[1]MARITON M.Jump Linear Systems in Automatic Control[M].New York:Marcel Dekker，1990.

[2]YUAN C G,MAO X R.Convergence of the Euler-Maruyama method for stochastic differential equations with Markovian switching[J].Mathematics and Computers in Simulation，2004，64:223-235.

[3]WANG Z，LIU Y，YU L，et al.Exponential stability of delayed recurrent neural networks with Markovian jumping parameters[J].Physics Letters A，2006，356:346-352.

[4]戴伟星，胡适耕.带Markov调制的随机微分延迟方程的稳定性(英文)[J].数学研究与评论，2008，28:511-520.

[5]WANG L S，XUE H.Convergence of numerical solutions to stochastic differential delay equations with Markovian switching and Poisson jump[J].Applied Mathematics and Computation 2007，188:1161-1172.

[6]RATHINASAMY A，BALACHANDRAN K.Mean square stability of semi-implicit Euler method for linear stochastic differential equations with multiple delays and Markovian switching[J].Applied Mathematics and Computation，2008，206:968-979.

[7]RATHINASAMY A.Split-step-methods for stochastic age-dependent population equations with Markovian switching[J].Nonlinear Analysis:Real World Applications，2012，13:1334-1345.

[8]Zhou S B，Wu F K.Convergence of numerical solutions to neutral stochastic delay differential equations with Markovian switching[J].Computational and Applied Mathematics，2009，229:85-96.

[9]LI R H，HOU Y M.Convergence and stability of numerical solutions to SDDE with Markovian Switching[J].Applied Mathematics and Computation，2006，175:1080-1091.

[10]LI R H，LEUNG P K，PANG W K.Convergence of numerical solutions to stochastic age-dependent population equations with Markovian switching[J].Journal of Computational and Applied Mathematics，2009，233:1046-1055.

[11]LI R H，PANG W K，LEUNG P K.Convergence of numerical solutions to stochastic age-structured population equations with diffusions and Markovian switching[J].Applied Mathematics and Computation，2010，216:744-752.

[12]JIANG F，SHEN Y，HU J H.Stability of the split-step backward Euler scheme for stochastic delay integro-differential equations with Markovian switching[J].Communications in Nonlinear Science and Numerical Simulation，2011，16:814-821.

[13]MA W J，ZHANG Q M.，WANG Z P.Asymptotic stability of stochastic age-dependent population equations with Markovian switching[J].Applied Mathematics and Computation，2014，227:309-319.

[14]杨洪福，张启敏.具有Markov调制的随机年龄结构种群系统半驯服Euler法的指数稳定性[J].数学年刊A辑(中文版)，2016，37:7-88.

[15]GUO X.Information and Option Pricing[J].Quantitative Finance，2001(1):38-44.

[16]ELLIOTT R J，HINZ J.Portfolio analysis，hidden Markov models and chart analysis by PF-diagrams[J].International Journal of Theoretical and Applied Finance，2002，5:385-399.

[17]ZHANG Q M，LIU Y T，LI X N.Strong convergence of split-step backward Euler method for stochastic age-dependent capital system with Markovian switching[J].Applied Mathematics and Computation，2014，235:439-453.

[18]ZHANG Q M，LIU W A，NIE Z K.Existence，uniqueness and exponential stability for stochastic age-dependent population[J].Applied Mathematics and Computation，2004，154:183-201.

[19]ANDERSON W J.Continuous-time Markov Chains[M].Berlin:Springer，1991.

(责任编辑陈艳)

Convergence of Solution for Stochastic Capital System with Markovian Switching

ZHENG Lai-yun

(School of Mechanical Engineering, Ningxia University, Yinchuan 750021, China)

The numerical solution of a class of stochastic age-dependent capital system with Markovian switching was given according to the Euler method in time discretization. Utilizing It’s formula, Gronwall lemma and Barkholder-Davis-Gundy inequality, some criteria were obtained for the convergence of the numerical solution.

stochastic capital system; Markovian switching; It’s formula

2016-05-08

宁夏自然科学基金资助项目(NZ14048)

郑来运(1979—),女，宁夏人，讲师，主要从事运筹学与控制理论的研究，E-mail: zhenglaiyun@126.com。

format：ZHENG Lai-yun.Convergence of Solution for Stochastic Capital System with Markovian Switching[J].Journal of Chongqing University of Technology(Natural Science)，2016(10):156-162.

10.3969/j.issn.1674-8425(z).2016.10.025

O231

A

1674-8425(2016)10-0156-07

引用格式：郑来运.具有Markovian调制的随机资本系统数值解的收敛性[J].重庆理工大学学报(自然科学)，2016(10):156-162.