一类带有治疗的乙肝病毒动力学模型的稳定性和Hopf分支

袁晓霞[1]，薛亚奎



一类带有治疗的乙肝病毒动力学模型的稳定性和Hopf分支

袁晓霞[1]，薛亚奎

（中北大学 理学院，山西 太原 030051）

建立了一类具有Logistic增长和治疗的乙肝病毒动力学模型，分析确定了疾病是否流行的阈值．当时，证明了无病平衡点是全局渐近稳定的，疾病消亡；当时，运用稳定性和分支理论，证明了系统可能出现Hopf分支．数值模拟验证了理论结果．

乙肝病毒动力学模型；药物治疗；稳定性；Hopf分支

乙肝病毒的动力学研究是针对乙肝病毒的致病机理及流行规律进行理论性分析的一种重要方法．通过建立模型，对疾病的发展和传播进行预测，为乙肝传染病的防治提供重要依据．1996年，Nowak等最早提出了乙肝病毒动力学模型[1]．Hews等假设健康肝细胞以Logistic 增长而非常数增长[2]，Min等采用标准发生代替双线性发生率[3]，这些改进使得模型更接近乙肝疾病的实际传播，较为合理．近年来，抗病毒治疗成为治疗乙肝的重要手段，临床上的抗病毒药物主要包括干扰素及核苷类药物２大类，如干扰素和拉米夫定等，他们能有效地抑制乙肝病毒的增殖[4]．本文建立了一类具有Logistic增长和治疗的乙肝病毒动力学模型，通过分析确定了疾病是否流行的阈值．

1模型的建立

3系统的Hopf分支

4数值模拟

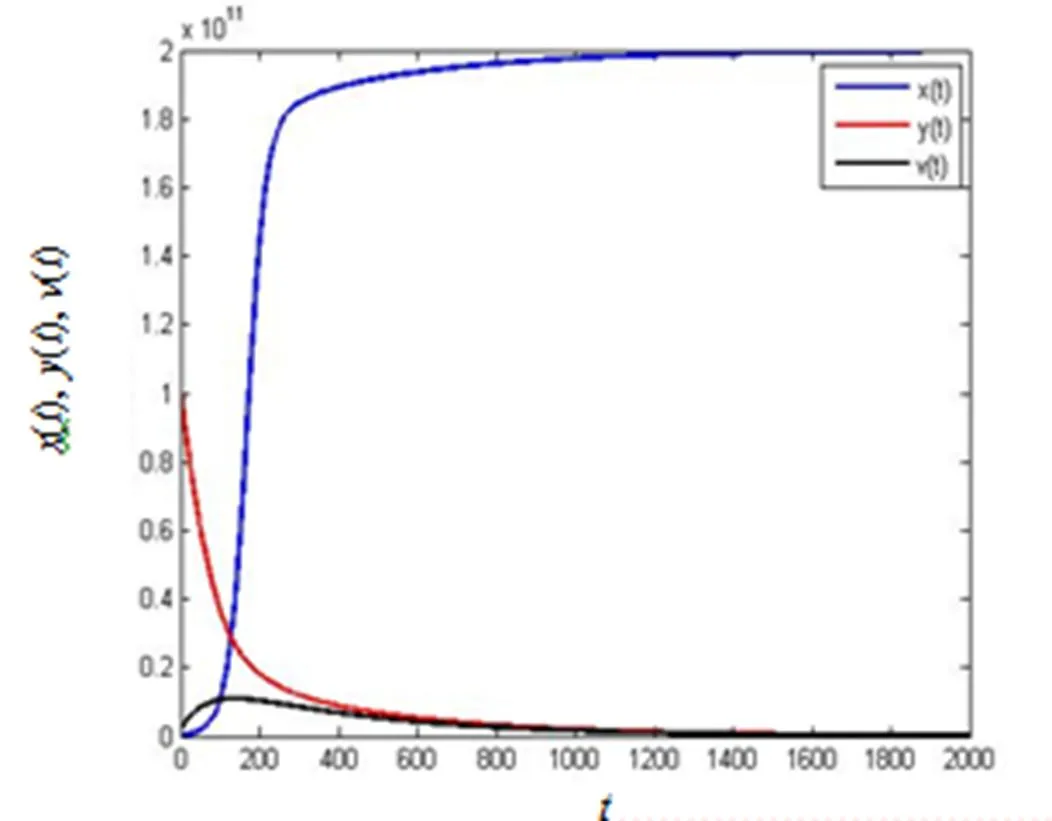
图1　时，变化曲线

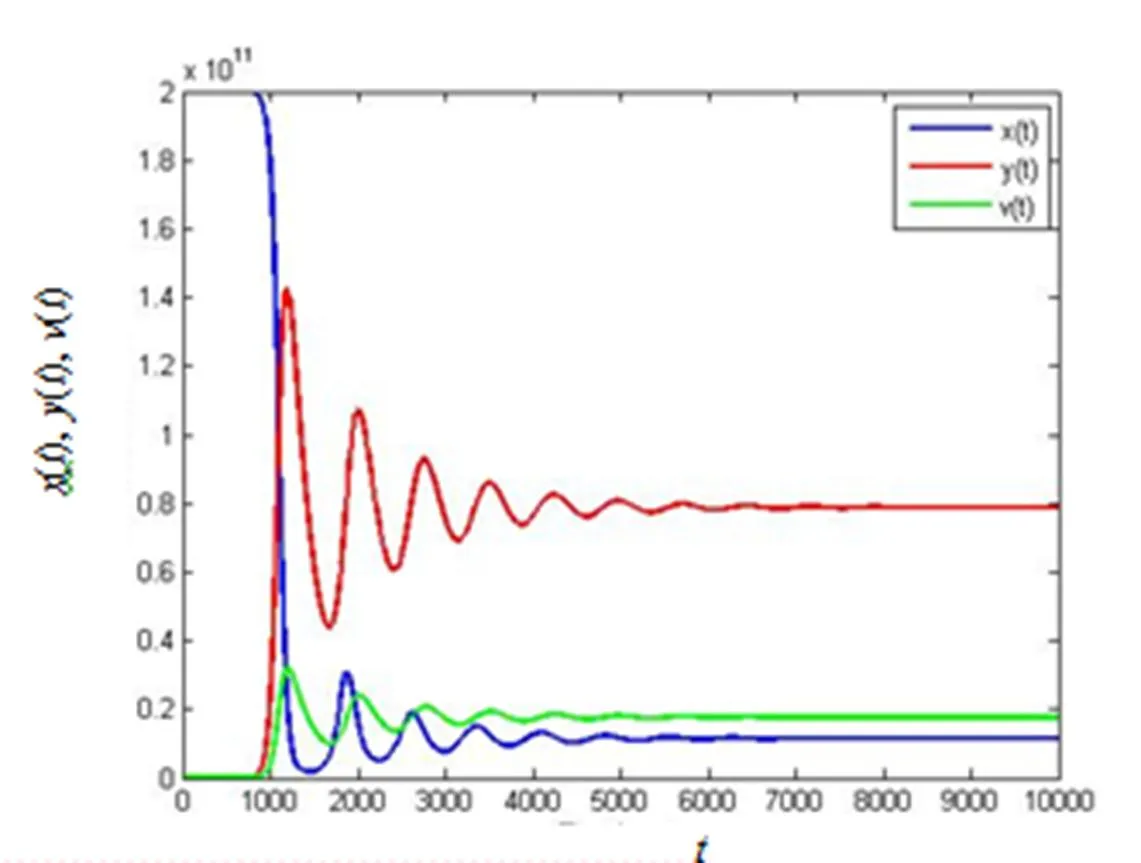
图2　时，变化曲线

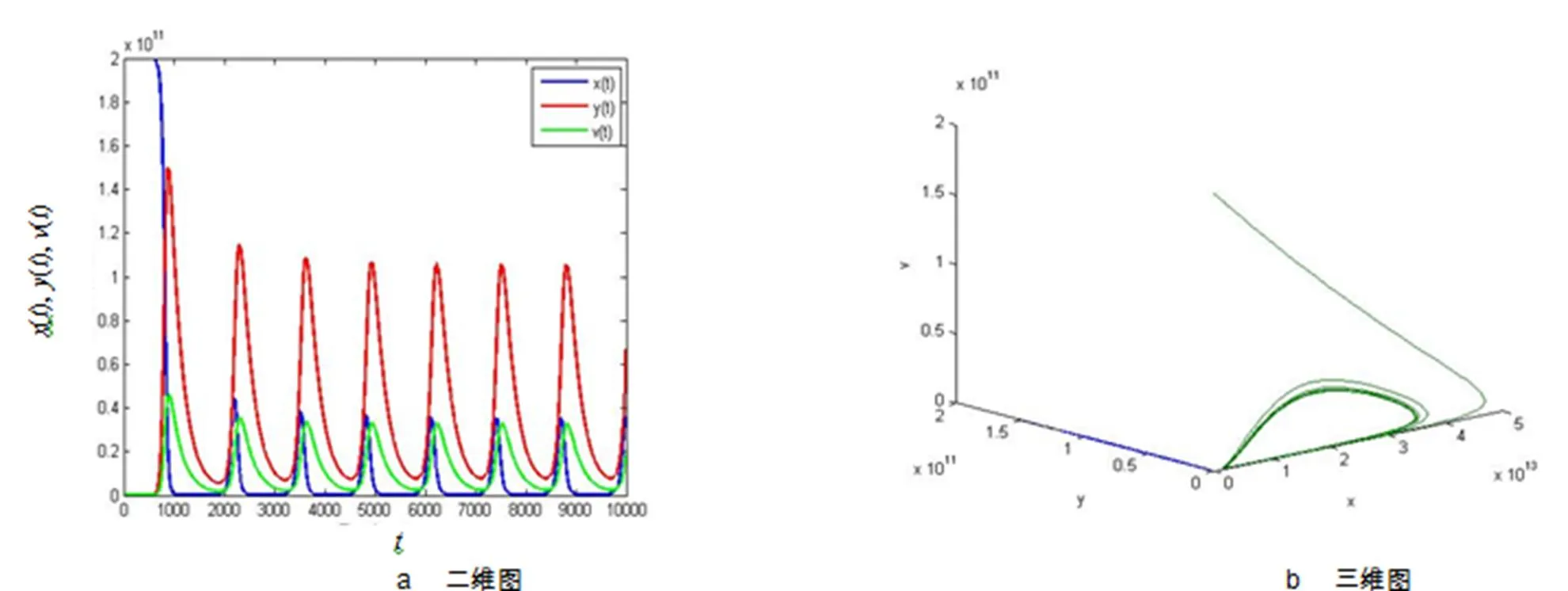
图3　时，变化曲线

本文研究了一类具有Logistic增长和治疗的乙肝病毒动力学模型．分析了平衡点的稳定性，给出了系统发生Hopf分支的临界条件．通过数值模验证了理论结果，对预防和控制乙肝传播具有实际的指导意义．

[1] Nowak M A，Bonhoeffer S，Hill A M，et al．Viral dynamics in hepatitis B virus infection[J]．Proceedings of the National Academy of Sciences，1996，93（9）： 4398-4402

[2] Bonhoeffer S，Coffin J M，Nowak M A．Human immunodeficiency virus drug therapy and virus load[J]．Journal of Virology， 1997，71（4）：3275-3278

[3] Min L，Su Y，Kuang Y．Mathematical analysis of a basic virus infection model with application to HBV infection[J]．Journal of mathematics，2008，38（5）：585-594

[4] Hews S，Eikenberry S，Nagy J D，et al．Rich dynamics of a hepatitis B viral infection model with logistic hepatocyte growth[J]．Journal of mathematical biology，2010，60（4）：573-590

[5] Hattaf K，Yousfi N．Hepatitis B virus infection model with logistic hepatocyte growth and cure rate[J]．Application Mathematical Science，2011，5（47）：2327-2335

[6] Van den Driessche P，Watmough J．Reproduction numbers and sub-threshold endemic equilibria for compartmental models of disease transmission[J]．Mathematical biosciences，2002，180（1）：29-48

Stability and Hopf bifurcation for a hepatitis B virusmodel with drug therapy

YUAN Xiao-xia，XUE Ya-kui

（School of Science，North University of China，Taiyuan 030051，China）

Formulated and analyzed a hepatitis B virusdynamics model with Logistic hepatitis growth and drug therapy．Obtained the basic reproduction number，which determines the disease is epidemic or not．Proved that the disease-free equilibrium is globally asymptotically stable and the infection becomes extinct if．When，using theories of stability and bifurcation，it was proved that a Hopf bifurcation may occur．Finally，numerical simulations were presented to verify the theoretical analysis．

hepatitis B virusdynamics model；drug therapy；stability；Hopf bifurcation

1007-9831（2016）02-0001-04

O29∶Q332.4

A

10.3969/j.issn.1007-9831.2016.02.001

2015-12-10

国家自然科学基金资助项目（11301491）

袁晓霞（1990-），女，山西太原人，在读硕士研究生，从事生物数学研究．E-mail：1244784275@qq.com

薛亚奎（1973-），男，山西太原人，教授，博士，从事生物数学研究． E-mail：xyk5152@163.com