强阻尼随机Kirchhoff方程的随机吸引子

秦闯亮，杜金姬

（信阳学院 数学与信息学院，河南 信阳 464000）

强阻尼随机Kirchhoff方程的随机吸引子

秦闯亮，杜金姬

（信阳学院 数学与信息学院，河南 信阳 464000）

讨论具有可加噪音的强阻尼随机Kirchhoff方程的随机动力系统，利用O-U过程对随机项进行处理，采用同构映射的方法，得到强阻尼随机Kirchhoff方程解的存在唯一性和随机吸引子的存在性.

O-U过程；随机Kirchhoff方程；随机吸引子

1 引言及预备知识

本文主要研究具有可加噪音的强阻尼随机Kirchhoff方程

其中：u=u(x,t)是D×[0,+∞)上的实值函数，D是Rn(n∈N)上具有光滑边界的有界开集；α>0；β>0；f(⋅),q(⋅)∈H01(D)IH2(D)；g(⋅)∈C1(R,R)；ρ>−1，且ρ为整数；qdW描述了一个可加白噪声；W(t)是概率空间(Ω,F,P)上的一维双边Wiener过程，Ω={ω∈C(R,R):ω(0)=0}，F为Ω上由紧开拓扑生成的Borelσ-代数，P为概率测度[1].

设(Ω,F,P)是概率空间，{θt:Ω→Ω,t∈R}是一族保测度变换，映射(t,Ω)θtω是可测的，θ0=id ，且θt+s=θtθs(s,t∈R)，则(Ω,F,P,(θt)t∈R)是一遍历度量动力系统，且θtω(⋅)=ω(⋅+t)−ω(t).

对于任意u,v∈L2(D)，令内积，范数；对于任意，令内积，范数；对于任意u,，令内积范数.

2 解的存在唯一性及随机动力系统

由文献[2-4]可知，式（4）中的算子L是Hilbert空间E上的C0-半群eLt上的无限小生成算子，对每个ω∈Ω，函数E→E关于ϕ是整体Lipschitz连续的.由Hilbert空间E上的随机微分方程解的存在唯一性[5-6]可知，方程（4）对初值和每个ω∈Ω具有唯一解ϕ，即且

对任意t≥0，a.e.ω∈Ω.设从E→E的解映射，则定义了方程（4）在E上的一个随机动力系统.

因此，对随机动力系统S1(t,ω)，只需考虑与其等价的随机动力系统S(t,ω).

3 随机吸引子的存在性

记空间E中的所有缓增子集合为D(E).

引理1[7]设，对任意，若，其中，有

引理2 设φ是问题（3）的一个解，则存在一个有界的随机紧集，使得对任意缓增随机集B(ω)∈D(E)，都存在一个随机变量TB(ω)>0，使得

引理3 对任意B(ω)∈D(E)，设φ(t)是方程（3）在初值φ0=(u0,u1+μu0)T∈B的解，它可分解为φ=φ1+φ2，其中：φ1,φ2分别满足

则

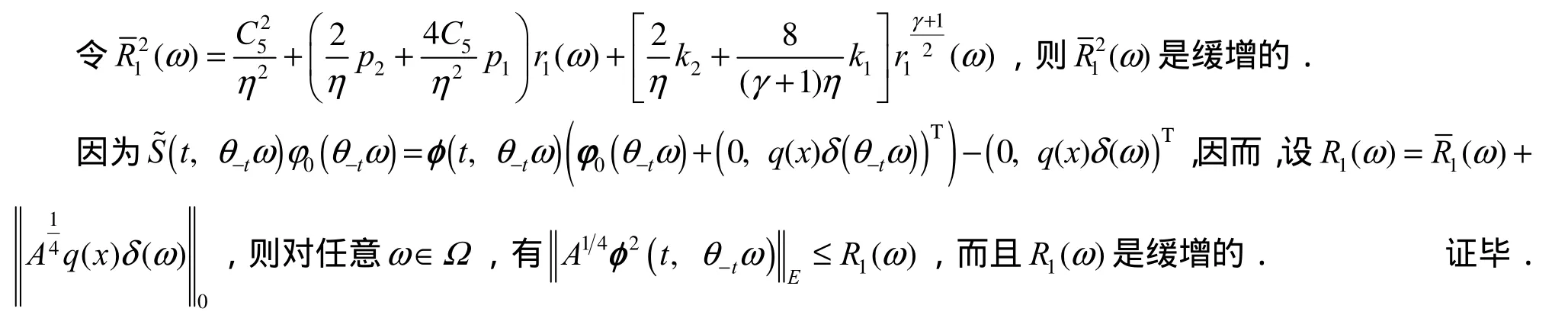
根据引理1～4，得到定理.

[1]ARNOLD L.Random dynamical systems[M].New York：Springger-Verlag，1998

[2]PAZY A.Semigroups of linear operators and applications to partial differential equations[M].New York：Springger-Verlag，1983

[3]Massatt P.Limiting behaviour for a strong damped nonlinear wave equation[J].J Diff Equ，1988（48）：334-349

[4]FAN X.Random attractor for a damped sine-Gordon equation with white noise[J].Pacific J Math，2004（216）：63-76

[5]CRAUEL H，NUALART D.Random nonlinear wave equations：Smoothness of the solutions[J].Probab Theory Related Fields，1988（79）：469-508

[6]Prato G D，Zabczyk J.Stochastic equations in infinite dimensions[M].London：Cambridge University Press，1992：115-236

[7]Zhou S F.Global attractor for strongly damped nonlinear wane equations[J].Functional differential equations，1999，6（3-4）：451-470

[8]Zhao Caidi，Zhou Shengfan.Sufficient conditions for the existence of global random attractors for stochatic lattice dunamical systems and applications[J].J Math Appl，2009（354）：78-95

Random attractor for strongly damped stochastic Kirchhoff equation

QIN Chuang-liang，DU Jin-ji
（School of Mathematics and Information，Xinyang College，Xinyang 464000，China）

It is discussed that the stochastic dynamical system of the strongly damped stochastic Kirchhoff equation with additive white noise.By using O-U process to deal with random itemsanda dopting the method of isomorphic mapping，proves the existence and uniqueness of solution and the existence of random attractor for such an equation.

O-U process；stochastic Kirchhoff equation；random attractor

O211.63

A

10.3969/j.issn.1007-9831.2016.12.002

2016-09-20

信阳学院资助项目（2015yb42）

秦闯亮（1981-），男，河南许昌人，助教，硕士，从事随机动力系统研究.E-mail：qincl168@163.com

1007-9831（2016）12-0006-06