一类非线性方程组正解的存在性

胡 玲

（黄山学院数学与统计学院，安徽黄山245041）

一类非线性方程组正解的存在性

胡 玲

（黄山学院数学与统计学院，安徽黄山245041）

研究了一类非线性二阶常微分方程组m-点边值问题的正解存在性，在合适的条件下，运用抽象的不动点定理，得出了正解及多重解的存在性。

m-点边值问题；格林函数；锥

1 引言

近年来，常微分方程的边值问题在数学及工程科学中受到很大的关注，据我们所知，绝大多数结果都是针对单个方程及简单的边值条件的，例如：[1,2]

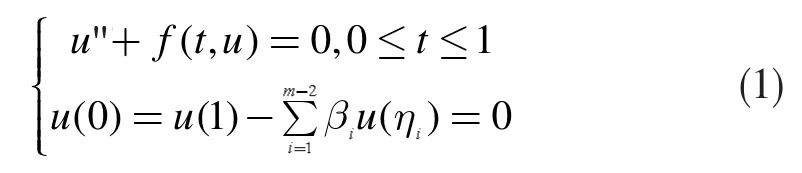
运用锥的不动点定理，可以获得（1）的正解存在性。再看下面的方程组：

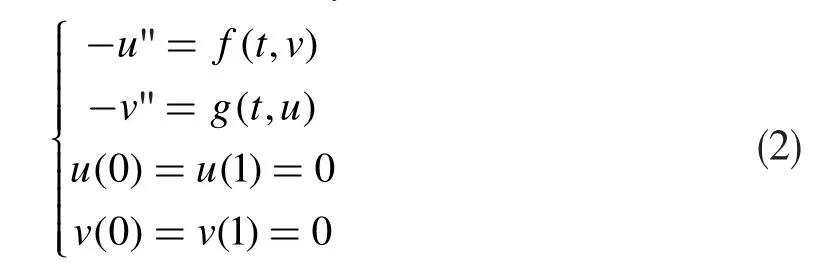
运用度理论[3]，（2）的正解也可以获得，类似还有很多结果[4-6]。可以看到，（1）中只有单个方程，而（2）中的边值条件是最简单的。

结合（1）与（2），本文研究的是如下方程组：

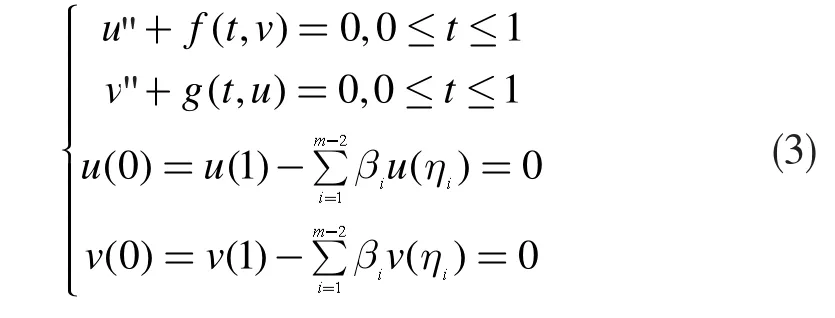
为了得到（3）的解的存在性，我们要用到格林函数，并定义一些锥，最后通过不动点定理得出正解的存在性及多重性。

2 引理

显然，方程组（3）可以转化为如下积分方程组：

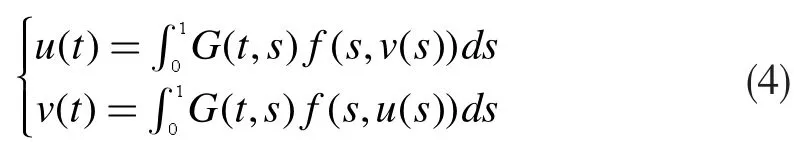
其中G（t,s）为格林函数，积分方程组（4）又可以化为非线性积分方程：

引理1：格林函数G（t,s）为如下形式：

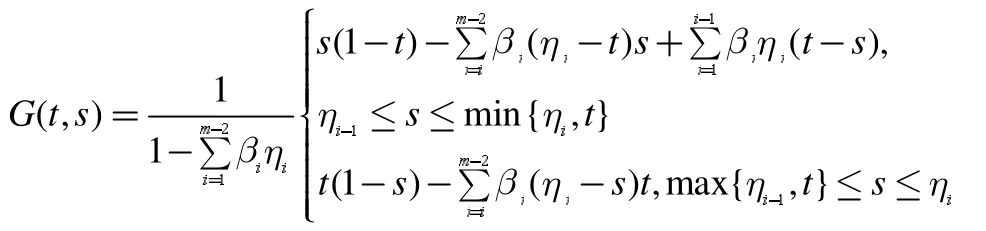
其中i=1，2，…m-1，显然，G（t,s）＞0，G（0,s）=G（1,s）=0。

设m=min∫G( t, s) ds, M=max∫G( t, s) ds，显然，M＞m＞0。

设E=C[0,1],∀u∈E,定义u=maxu,那么{E，||·||}是一个巴拿赫空间，定义

K={u∈E u( t)≥0,minu( t)≥γu,η≤t ≤1}，

显然K是E中的一个正锥。

由α(u)=minu( t )，我们定义函数α:K→R。定义算子

Tu( t)=∫G( t, s) f( s,∫G( s,σ)g(σ,u(σ))dσ)ds, u∈K,(6)由以上讨论可知，（3）的正解的存在性可以转化为算子T的正不动点的存在性问题。

3 定理

定理1：设a，b，c＞0，满足a＜b≤min{γ,m }c,f( t,0)≠0,g( t,0)≠0且M

那么边值问题（3）至少有3个正解u1，u2，u3，满足

证明：T如上定义，∀Ω∈K ，开集且非空，我们容易知道T:→K是全连续的。设

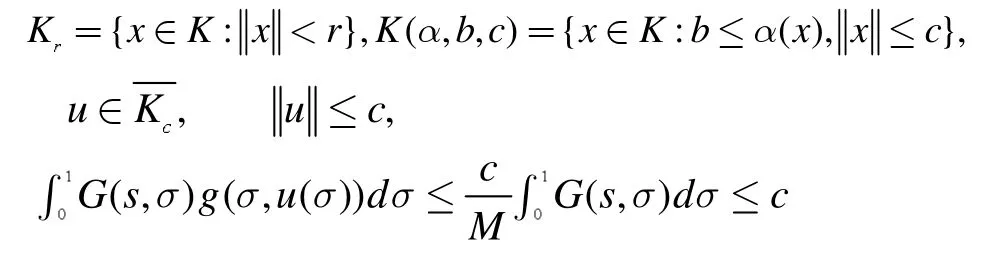
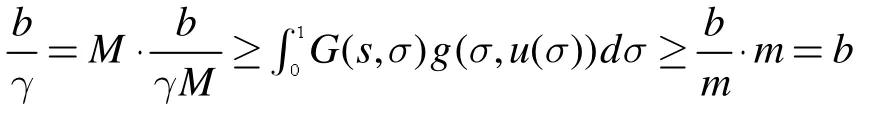
所以，我们有

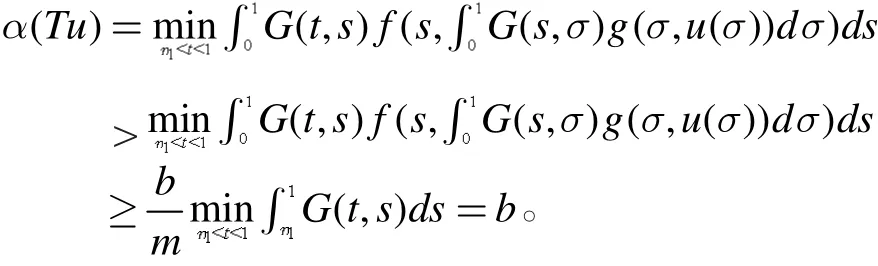
综上所述，锥压缩锥拉伸不动点定理的条件得到满足，我们得到至少有3个不动点u1，u2，u3,满足，即是边值问题（3）的3个正解。

[1]Guo Y,Ge W,Three positive solutions for second order m-point boundary value problems[J].Appl Math.Camp，2004,156(3)：733-742.

[2]Guo Y,Ge W.Positive solutions for second order mpoint boundary value problem[J].Comp.Appl.Math,2003,151:415-424.

[3]Hu Ling and L.L.Wang,Multiple Positive Solutionsof Boundary Value Problems for Systems of Nonlinear Second-Order Differential Equations[J].Journal of Mathematical Analysis and Applications,2007,335：1052-1060.

[4]K.Deimling,Nonlinear Functional Analysis[M].Springer-Verlag,Berlin 1985.

[5]Leggett R W,Williams L R,Multiple positive fixed solutions of nonlinear operators on ordered Banach spaces[J].Indiana Univ.Math，1979,673-688.

[6]杨志林，孙经先，非线性二阶常微分方程组边值问题的正解[J].数学学报,2004,47(1)：111-118.

责任编辑：胡德明

Abstract:The paper is concerned with positive solutions to m-point boundary value problems of a class of nonlinear second order differential equations.Under suitable conditions,the existence of positive solutions and multiplicity of solutions are proved by using abstract fixed-point theorems.

Key words:m-point boundary value problem;Green's function;cone

Positive Solutions of m-Point BVPs for Nonlinear Differential Equations

Hu Ling
(School of Mathematics and Statistics,Huangshan University,Huangshan245041,China)

O175.8

A

1672-447X（2012）03-0004-002

收搞日期：2012-03-21

安徽省教育厅教学研究项目（20100996）；黄山学院质量工程项目（2011JPKC06）

胡玲（1981-），安徽黄山人，黄山学院数学与统计学院讲师，硕士，研究方向为微分方程。