Ordering in liquid and its heredity impact on phase transformation of Mg-Al-Ca alloys

Jing You ,Cheng Wng,b,∗ ,Shun-Li Shng ,Yipeng Go,b ,Hong Ju ,Hong Ning ,Yi Wng,Hui-Yun Wng,b,Zi-Kui Liu

a State Key Laboratory of Automotive Simulation and Control & School of Materials Science and Engineering,Jilin University,Nanling Campus No. 5988 Renmin Street,Changchun 130025,China

b International Center of Future Science,Jilin University,Changchun 130012,China

c Department of Materials Science and Engineering,The Pennsylvania State University,University Park,PA 16802,United States of America

Abstract It is a long-sought goal to achieve desired mechanical properties through tailoring phase formation in alloys,especially for complicated multi-phase alloys.In fact,unveiling nucleation of competitive crystalline phases during solidification hinges on the nature of liquid.Here we employ ab initio molecular dynamics simulations (AIMD) to reveal liquid configuration of the Mg-Al-Ca alloys and explore its effect on the transformation of Ca-containing Laves phase from Al2Ca to Mg2Ca with increasing Ca/Al ratio (rCa/Al).There is structural similarity between liquid and crystalline phase in terms of the local arrangement environment,and the connection schemes of polyhedras.The forming signature of Mg2Ca,as hinted by the topological and chemical short-range order originating from liquid,ascends monotonically with increasing rCa/Al.However,Al2Ca crystal-like order increase at first and then decrease at the crossover of rCa/Al=0.74,corresponding to experimental composition of phase transition from Al2Ca to Mg2Ca.The origin of phase transformation across different compositions lies in the dense packing of atomic configurations and preferential bonding of chemical species in both liquid and solid.The present finding provides a feasible scenario for manipulating phase formation to achieve high performance alloys by tailoring the crystal-like order in liquid.

Keywords: Liquid alloy;Solidification;Crystal embryo;Short-range order;Ab initio molecular dynamics.

1.Introduction

Magnesium (Mg) alloys are the lightest structural materials developed so far,with higher specific strength than most aluminum alloys and steels [1–4].Especially,the recently developed Mg-Al-Ca alloys have come into notice owing to their low cost,excellent formability,and improved creep resistance[5–7].It was reported that the Ca-containing Laves phases,with high thermal stability,play a critical role in determining the mechanical properties of Mg-Al-Ca,whose formations are sensitive to alloy composition.With increasing Ca/Al mass ratio (rCa/Al),the discontinuous Mg17Al12will be suppressed,and the Ca-containing Laves phases evolve sequentially from Al2Ca (C15) to (Mg,Al)2Ca (C36) and to Mg2Ca (C14) [8].It was found that the deformable Al2Ca could result in large work hardening and improve tensile elongation.However,exceeding Ca leads to the formation of C14-Mg2Ca,which is detrimental to ductility but enhances the strength of Mg alloys [9].Besides,the C36-(Mg,Al)2Ca phase was related to the improved creep resistance of Mg alloys due to its stability at high temperatures [10].

Considerable researches have been carried out to tailor the secondary phases with respect to their morphology,size,amount,and distribution,aiming at optimizing mechanical properties [5,8,11].However,most previous studies are frequently at a phenomenological level regarding the formation of Ca-containing Laves phases caused by therCa/Al.For example,typical studies of phase formation are usually performed by thermodynamic modeling,empirical computations,and/or experimental measurements of thermodynamic properties (Gibbs energy,phase boundary,etc.) for the phases of interest [12,13].As an example,Zhang et al.[14] investigated thermodynamic properties at finite temperatures of Laves phases in the Mg-Al-Ca system by first-principles calculations.They elucidate that C14-Mg2Ca has a weaker bonding in comparison with C15-Al2Ca,confirmed by its larger volume,lower bulk modulus,and lower enthalpy of formation[14].Nevertheless,the underlying physics regarding the phase transformation process during solidification is still mysterious,motivating the present study from an atomic perspective to understand phase transformation in the Mg-Al-Ca alloys.

As the parent state for most solid phases,the nature of liquid is important in determining the selection among competitive phase transformations.Despite the lack of long-range translational symmetry,liquid is considered to be in shortrange order.Short-range order in liquid is critical to tailor the forming ability and properties of amorphous alloys[15–17].This is because amorphous alloys are originated from the liquid forming at an extremely high cooling rate,and thus have a structural heredity character with their liquid counterparts [18].Meanwhile,the topological order in amorphous alloys has structural homology with respect to the competing metastable crystalline phases [19],which has been utilized to understand geometric frustration in glass transition[20].For nucleation during crystallization,two elements with the preferred nearest-neighbor pairing (strong element interaction) indicates the formation trends of the crystalline phase.For example,Ma et al.[21] found strong ordering trends in the liquid Ba-Bi alloys corresponding to the compositions of crystalline phases.Furthermore,the similarity of atomic interaction between liquid and solid is an indication of heredity in multi-component alloys.Nevertheless,the critical question is to provide direct evidence of structural heredity between liquid and stable crystalline phases.

The scope of the present work is to elucidate the effect of liquid structure on the formation of crystalline phases in the Mg-Al-Ca alloys.To this end,we provide direct evidence demonstrating structural similarity at atomic scale between liquid and crystalline phases in terms ofabinitiomolecular dynamics (AIMD) simulations.Furthermore,we illustrate that the phase transformation sequence with respect torCa/Alin Mg-Al-Ca,i.e.,from C15-Al2Ca to C36-(Mg,Al)2Ca and then to C14-Mg2Ca,can be correlated with the evolution of crystal-like order extracted from liquid.Our results shed light on the long-existing issue regarding the role of atomic order identified in the Mg-Al-Ca liquid,acting as fundamental building blocks of crystalline phases.

2.Simulation details

Based on the phase transformation sequence with increasingrCa/Alobserved in the as-cast Mg-Al-Ca alloys,we se lected fourrCa/Alvalues,i.e.,0.33,0.6,0.74,and 1 [22,23].Criteria for selecting these ratios were determined by including the formation of C15-Al2Ca,C36-(Mg,Al)2Ca,and C14-Mg2Ca with increasingrCa/Al.The Ca-containing Laves phases corresponding to the specificrCa/Alratios are shown in Table 1.

Table 1Designed rCa/Al values in Mg-Al-Ca together with the corresponding Cacontaining Laves phases,atomic concentrations,density,and liquidus temperature in the present AIMD simulations.

In the present work,the AIMD simulations were conducted by employing the Viennaabinitiosimulation package(VASP) [24,25] with the generalized gradient approximation by Perdew-Burke-Ernzerhof [26] for the exchange correlation functional.The electron-ion interaction was handled with the projector augmented wave pseudopotentials [27].Newton’s equation motion was solved via the Verlet’s algorithm with a time step of 3 fs.The AIMD simulations were performed in the NVT ensemble (i.e.,the fixed number N,volume V,and temperature T),wherein the temperature was controlled by the Nose-Hoover thermostat [28,29].The Brillouin zone was sampled at a singleΓpoint only and the plane wave cutoff energy was set as 300 eV for AIMD simulations.

All the AIMD simulations were performed in a cubic supercell containing 200 atoms with periodic boundary condition.The chemical compositions of each atomic configuration employed in the present AIMD simulations are shown in Table 1.The initial configurations were constructed with the atoms randomly arranged according to the composition,and these initial configurations had negligible effects on the AIMD results.The equilibrium volume of supercell was adjusted to ensure that the total external pressure was close to zero by a parabolic fitting of the AIMD predicted pressure(P) vs.volume (V) data points,i.e.,using the equation ofP=aV2+bV+c,witha,b,andcbeing the fitting parameters [30].It is worth mentioning that the total external pressures of all AIMD simulations are within ± 0.2 GPa,causing negligible influence on structural analyses [31].

In each AIMD simulation,the supercell was heated up to 1500 K for 6 ps to eliminate the effect of the initial configuration.It was then sequentially cooled down to 1000 K with a cooling rate of 3.33 × 1013K/s and then the configurations were equilibrated for another 24 ps at 1000 K.The analyses of structural and dynamic properties were performed at 1000 K,which corresponded to the equilibrium liquid state for all the compositions.By employing the phase diagram calculation driven by PANDAT 2018,the liquidus temperature of the four Mg-Al-Ca alloys in this study are 844 K,970 K,996 K,and 1039 K respectively,which are also listed in Table 1 and Fig.S1(Supplementary Information).The simulation temperature,i.e.,T=1000 K,is above the liquidus temperature of the first three compositions,but with a degree of undercooling for the Ca/Al ratio=1.It is well known that temperature is a critical factor on the liquid structure.To ensure the determination of structural and dynamic properties under equilibrium condition,especially for Ca/Al ratio=1,we calculated the relaxation time through self-intermediate scattering function (ISF).It was found that the equilibrium time was far more than the relaxation time (see more details in Fig.S2 and Fig.S3).Consequently,the studied temperature 1000 K is deemed as rational.The atomic configurations were rendered by the OVITO [32] and Device Studio [33] which provided a number of functions for performing visualization.

3.Results and discussion

3.1. Structural order of liquid Mg-Al-Ca alloys

3.1.1.Paircorrelationfunction

Pair correlation function (PCF) was used to reveal atomic distributions of the Mg-Al-Ca liquid at 1000 K.PCF is a statistical analysis of global structural signature,reflecting the ensemble average of structural information.The partial PCF of ani-jatomic pair is defined by the probability of findingjatom in the spherical shell takingias the center.It can be expressed by [18]:

whereLis the length of the cubic supercell,NiandNjare the number of atoms ofiandjspecies in the supercell,respectively,andnij(r,Δr) represents the number ofjspecies in the(r→r+Δr) shell.The angle brackets denote the time average of different configurations.The last 2000 configurations were used for statistical analysis of partial PCFs.It should be noted that the partial PCFs in this study had been smoothed out by using a Gaussian filter kernel with the standard deviation of 0.2.The comparison between raw data and smoothing data of partial PCFs is presented in Fig.S4.

Fig.1 shows the partial PCFs,gij(r),in liquid Mg-Al-Ca as a function ofrCa/Alat 1000 K.The position of the first sharp peak in partial PCF curves is designated as the bond length of specific species.Accordingly,the bond lengths of Mg-Mg,Al-Al,Ca-Ca,Mg-Al,Mg-Ca,and Al-Ca are 3.12,2.91,3.43,2.72,3.18,and 3.81 ˚A,respectively.These bond lengths are in a good agreement with the calculated results derived from Debela’s [34] and Sheng’s work [16],and the reported experimental result [35].It verifies the accuracy of our simulation results,and the smoothing procedure makes no sense on characterizing the partial PCFs.The position of the first deep valley denotes the cutoff distance of short range,forming atomic bonding within this distance.The cutoff distances of Mg-Mg,Al-Al,Ca-Ca,Mg-Al,Mg-Ca,and Al-Ca are 4.36,3.81,4.98,4.07,4.71,and 4.50 ˚A,respectively.The intensity of the first peak of partial PCF signifies the preferred nearest-neighbor pairs,providing critical information on chemical affinity between elements [36,37].Accordingly,the pronounced first peaks of gMg-Al(r) (Fig.1d),gMg-Ca(r)(Fig.1e),and gAl-Ca(r) (Fig.1f) reflect the significant chemical interaction in the short range between these atomic pairs.The first peak of gAl-Ca(r) is higher than that of gMg-Ca(r)and gMg-Al(r),indicating the Al-Ca interaction is more favorable.Nevertheless,their peak values show diverse variations with increasingrCa/Al,i.e.,essentially constant for gMg-Al(r),increasing for gMg-Ca(r),and decreasing for gAl-Ca(r).

Fig.1.Partial PCFs of the liquid Mg-Al-Ca alloys for the studied rCa/Al ratios at 1000 K,including (a) gMg-Mg(r),(b) gAl-Al(r),(c) gCa-Ca(r),(d) gMg-Al(r),(e) gMg-Ca(r),and (f) gAl-Ca(r),respectively.

It should be noted that our models contain limited solute atoms of Al and Ca in order to retain the solvent nature of Mg.These models would cause a fluctuation of results,especially for the Ca-Ca pair as shown in Fig.1c,similar to the other AIMD simulations [36].Albeit the deviation cannot be eliminated,it shows that the chemical affinity increases for the Mg-Ca pairs,while it decreases for Al-Ca asrCa/Alincreases.To further unveil liquid structure in Mg-Al-Ca,local topological structures are extensively characterized in the following sections.

3.1.2.Coordinationnumber

Coordination number (CN) signifies the number of atoms around the center of a given atom.Partial CN,Zij,is the number of thejspecies that are in the nearest-neighbor of the speciesi.The nearest distance was determined through the location of the first deep valley of the gij(r) curve.For accurate statistics,we directly count the atomic number ofjspecies,rather than by integrating gij(r).The total CN of speciesiis defined as the sum of all related partial CN’s.For example,ZMg=ZMgMg+ZMgAl+ZMgCa.

Fig.2 presents the histogram distribution of the partial CN’s for the studiedrCa/Alratios at 1000 K.It shows that the number of total CN for a given species is essentially constant with increasingrCa/Al,i.e.,the Mg-,Al-,Ca-centered CN’s are around 13,11,and 16,respectively.According to the partial CN’s,we conclude that the fractions of atoms constituting the coordination shells are Mg,Al,and Ca from high to low in sequence.The number of Al atoms in the coordination shells appears to be the least affected by chemical composition.The number of Mg atoms acting as shell atoms decreases asrCa/Alincreases,while it shows an obvious increase for Ca atoms in the coordination shell.Note that these variations are closely associated with the present models,i.e.,the number of Mg decreases,in principle Al stays constant,but Ca increases with increasingrCa/Al.

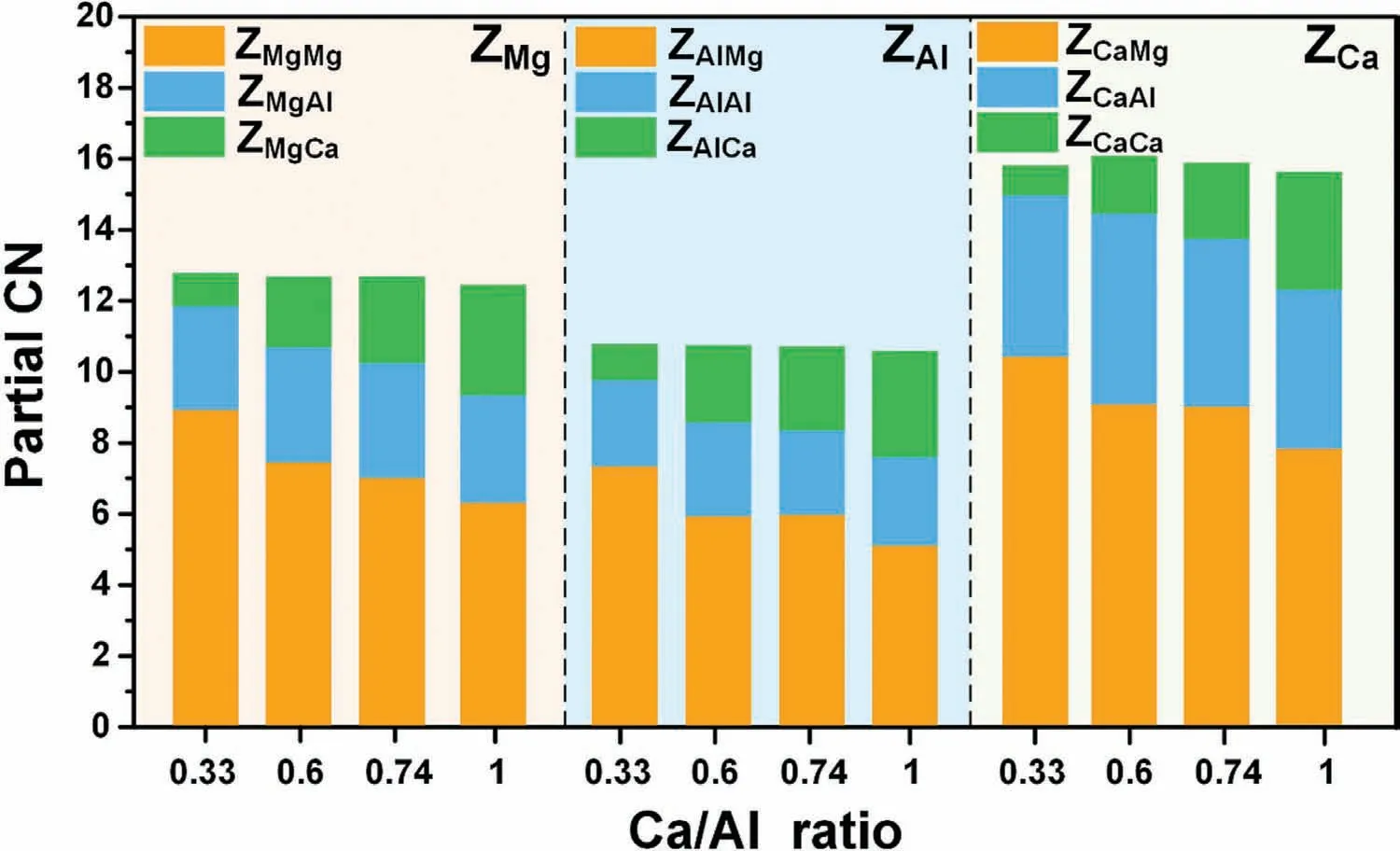
Fig.2.Distribution of the partial CN’s of the liquid Mg-Al-Ca for the studied rCa/Al at 1000 K.

Consequently,the chemical composition only affects the proportion of different atoms in the coordination shell,as the total CN of a specific species is essentially unchanged.The characters of the intra-cluster can be determined by the Voronoi tessellation and bond pair analysis as follows.

3.1.3.Voronoitessellation

Voronoi tessellation method can characterize topological features in a configuration.It is a scheme to divide the three-dimensional (3D) space into cells by considering the faces of the Voronoi polyhedron (VP) enclosing the particles [18].According to this method,we can differentiate the characteristic arrangement of the near neighbors surrounding a center atom.Different VPs can be labeled by the Voronoi index ,wherenidenotes the number of i-edged faces on the surface of the VPs andis the total CN.For example,the Voronoi index of <012,0>represents the ideal icosahedra (ICO);<0,2,8,1>,<0,2,8,2>,<0,3,6,3>,and <0,1,10,2>are the distorted ICO;<0,3,6,4>stands for the FCC-type polyhedra;<0,2,8,0>is the bi-capped square antiprism polyhedra;<0,3,6,0>signifies the tricapped trigonal prism polyhedra;and <0,0,12,4>represents the Z16 Frank-Kasper polyhedra.

In the present work,the VPs with amounts above 1% were presented to focus on the pivotal local order of the liquid structure.As shown in Fig.3,the amounts of the ICO-type polyhedra with Voronoi indices of <0,0,12,0>,<0,2,8,1>,<0,2,8,2>,<0,3,6,3>,and <0,1,10,2>exhibit a dominant population across all compositions,which is a common character of liquid.The local order in liquid depends strongly on compositions.For example,the amount of the ICO-type Voronoi index,<0,2,8,1>arises with increasingrCa/Al.In addition,the fractions of <0,0,12,0>and<0,1,10,2>display a peak whenrCa/Al=0.74,indicating the liquid Mg-Al-Ca possesses an structural transition at this composition.Meanwhile,the fraction of the FCC-type polyhedra with the index of <0,3,6,4>consistently decreases with increasingrCa/Al.

Fig.3.Distribution of VPs in the liquid Mg-Al-Ca with studied rCa/Al at 1000 K.

3.1.4.Honeycutt-Andersenbondpairanalysis

Common-neighbor analysis can be used to further investigate the local atomic configurations in liquid Mg-Al-Ca.According to the Honeycutt-Andersen’s formula[38],the indicesi,j,k,lcan be used to interpret the bonded atomic pair with their common neighbors,characterizing the local bonding environments.The first integerispecifies whether the bonds can be formed between two atoms,i.e.,i=1 for bonding andi=2 for non-bonding.The second indexjmeans the number of neighbors of the bonded pair.The third indexkdenotes the number of bonds between neighbors.The last parameterlis used to distinguish the bond geometries when the first three indices are the same.The typical bond pairs are related to specific local order [39,40].For instance,the 1551 bonded pair corresponds to a pentagonal bipyramid,which is the building block of an ideal icosahedron.1541 and 1431 are treated as defective icosahedron.The bond pairs with indices 1441 and 1661 are considered as the constituent parts of a BCC-type structure.1421 and 1422 reflect the characteristics of FCC-and HCP-type structures.The other indices of bond pairs are usually classified as random structures.

Fig.4a represents the percentage of typical Honeycutt-Anderson bond pairs,and Fig.4b shows the corresponding local ordering structures of the liquid Mg-Al-Ca with differentrCa/Alratios at 1000 K.It is illustrated that except for the 1551 bond pairs,the other bond pairs show abrupt changes(descend or ascend) at therCa/Al=0.74,indicating structural transition at this point.More importantly,the ICO-type bond pairs(red circles in Fig.4b)are more than 50%,indicating the predominance of ICO-type ordering in liquid Mg-Al-Ca.The fraction of the ICO-type and FCC/HCP-type bond pairs(black squares in Fig.4b) increase with increasingrCa/Al,while the BCC-type (blue triangles in Fig.4b) decreases clearly at therCa/Al=0.74.Meanwhile,the fraction of random-type order(green rhombus in Fig.4b) decreases monotonically,indicating the increased degree of ordering of the local structures.All these results are consistent with the analyses by Voronoi tessellation in Section 3.1.3.

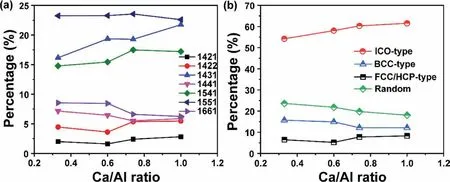
Fig.4.Fraction of (a) bond pairs,and (b) ICO-type (1551,1541,and 1431),BCC-type (1441 and 1661),FCC/HCP-type (1421 and 1422),and others (random types) in the liquid Mg-Al-Ca with the studied rCa/Al at 1000 K.

The above discussion indicates an exhaustive view of structural signature in liquid Mg-Al-Ca.We conclude that with increasingrCa/Al,the chemical affinity of Al-Ca decreases,while that of Mg-Ca increases roughly,with the total number of CN for a specific atomic species essentially constant.Moreover,the predominant orders are ICO-type in liquid,and an abrupt structure transition appears at therCa/Al=0.74.

3.2. Structural heredity characteristics

It is a challenge to establish a causal link of structural building blocks between liquid and their crystalline counterparts.In this section,we compare the structural signature between liquid and crystals in Mg-Al-Ca,from the viewpoint of atomic arrangements on the local features,as well as the connection schemes of polyhedras.Furthermore,we provide direct evidence at the atomic scale,unveiling the underlying mechanism of phase transformation in Mg-Al-Ca.

3.2.1.Similarityinlocalatomicarrangement

To illustrate the relationship between the three Laves phases and their liquid ordering characteristics,we use Voronoi tessellation to describe the possible clusters relative to a centered atom spatially and visually.Owing to the described sensitivity of Voronoi tessellation to the perturbations of atomic coordinates,its application to crystal is not pervasive as the analysis of liquid and glasses [41].Herein,small faces and short edges were eliminated with a threshold in construction of VPs for crystal,so that the related multiple vertices should still be counted as one [41].

Fig.5 illustrates the coordination polyhedra extracted from C15-Al2Ca,C36-(Mg,Al)2Ca,and C14-Mg2Ca.The criterion of Voronoi tessellation is to ensure the coordination polyhedra extracted from Laves phases with the highest atomic-packing efficiency.It is schematically depicted in Fig.5a that C15-Al2Ca is a Cu2Mg-type cubic crystal.The local atomic environment in the C15-Al2Ca phase is that Al (16d site of space group Fd-3mZ) is centered by six Al atoms and six Ca atoms,and each Ca (8a site) is surrounded by 12 Al atoms and 4 Ca atoms.Al-centered polyhedra are characterized by the Voronoi index of <0,0,12,0>,indicating a structural signature of ideal icosahedra,and consequently,the CN of Al is 12.The Voronoi index of Ca-centered polyhedra is<0,0,12,4>,denoting the Z16 Frank-Kasper polyhedra with a high CN of 16.As shown in Fig.5b,C14-Mg2Ca is a MgZn2-type hexagonal crystal with two non-equivalent Mg sites (2a and 12k of space group P63/mmc) and one Ca site(4f).The atomic coordination environments of Mg and Ca in C14-Mg2Ca are analogous to those of Al and Ca in Al2Ca,respectively.The C36-(Mg,Al)2Ca shown in Fig.5c is an orthorhombic crystal with one Mg site (4a of space group Pbcm),two Al sites (4c,4d),and four Ca sites (4d).The atomic coordination polyhedra of Mg and Al in (Mg,Al)2Ca show icosahedral signature,while the Ca centered coordination polyhedra with Voronoi index <0,0,12,3>represents the high coordination structure.

Fig.5.Illustration of coordination polyhedras centered by atoms at non-equivalent occupation sites in crystalline phases,including (a) C15-Al2Ca (Fd-3mZ),(b) C14-Mg2Ca (P63/mmc),and (c) C36-(Mg,Al)2Ca (Pbcm).The number and type of constituent atoms in each coordination polyhedra are labeled below.Wyckoff positions are shown with polyhedra.The light green,cyan,and blue spheres stand for Mg,Al,and Ca atoms,respectively.

Furthermore,we clarify whether the phase transformation caused by the variation of alloy compositions has already been reflected by the liquid order.The coordination polyhedra in the three Laves phases have been identified with Voronoi indices of Mg-centered <0,0,12,0>,Al-centered<0,0,12,0>and <0,2,8,2>,and Ca-centered <0,0,12,3>and <0,0,12,4>.Based on this characterization,we designate the liquid order with the identical Voronoi indices as the crystal-like order (highlighted in Fig.6a).These crystal-like orders can be used to signify structural heredity between liquid and its crystal counterpart.However,the atomic arrangements of polyhedra in liquid cannot be modeled by a uniquely prescribed stereochemical structure (as stated in Section 3.1.2).Herein,we only consider the geometry of local ordering and category of centered atoms,without differentiating the types of atoms in the nearestneighbor shell when extracting the crystal-like order in the liquid.

Fig.6.(a) Crystal-like order extracted from the Mg110Al60Ca30 liquid,(b) fraction of the crystal-like order in liquid Mg-Al-Ca for the studied rCa/Al ratios at 1000 K.The light green,cyan,and blue spheres stand for Mg,Al,and Ca atoms,respectively.

As shown in Fig.6b,the fraction of Mg-centered crystallike order in liquid increases gradually with increasingrCa/Al.Two types of Al-centered orders follow a similar trend: initially increase and then decrease,with the same crossover atrCa/Al=0.74.The population of Ca-centered crystal-like order is scarce and exhibits slight fluctuations with increasingrCa/Al.Thermodynamic studies on phase formation in the Mg-Al-Ca alloys have revealed that the amount of C15-Al2Ca increases with therCa/Alranging from 0.37 to 0.75,and then decreases when therCa/Alratio is above 0.75.Experimentally,Ninomiya et al.[8] found that with therCa/Alratio higher than 0.8,the C14-Mg2Ca phase forms in Mg-Al-Ca.The variation trend of crystal-like order in liquid coincides with crystallization sequence from C15-Al2Ca to C14-Mg2Ca and thus acts as an indicator of crystal transformation induced by chemical variation.

3.2.3.Similarityinpolyheraconnectivity

It is essential to explore how the crystal-like order extends up to longer distance in both liquid and crystalline phases of Mg-Al-Ca when filling the 3D space.The first question is the geometric inner-link of the three Laves phases.Owing to the connection schemes of adjacent polyhedra,it must be intercross-connected (share 7 atoms) [42],which cannot signify the characteristic feature of the polyhedra connection.Herein,we consider the connection schemes between two relatively isolated coordination polyhedra linked by‘glue’atoms[43].To explicitly differentiate the packing characteristics of C15-Al2Ca,C36-(Mg,Al)2Ca,and C14-Mg2Ca,we study the connection schemes of Al and Al-centered coordination polyhedra (namely <0,0,12,0>and <0,2,8,2>) in C15 and C36,as well as Mg and Mg-centered coordination polyhedra(namely <0,0,12,0>) in C14 and C36,as shown in Fig.7.The neighboring polyhedrons share one,two,and three atoms,which are denoted as Vertex-sharing,edge-sharing,and facesharing,respectively.

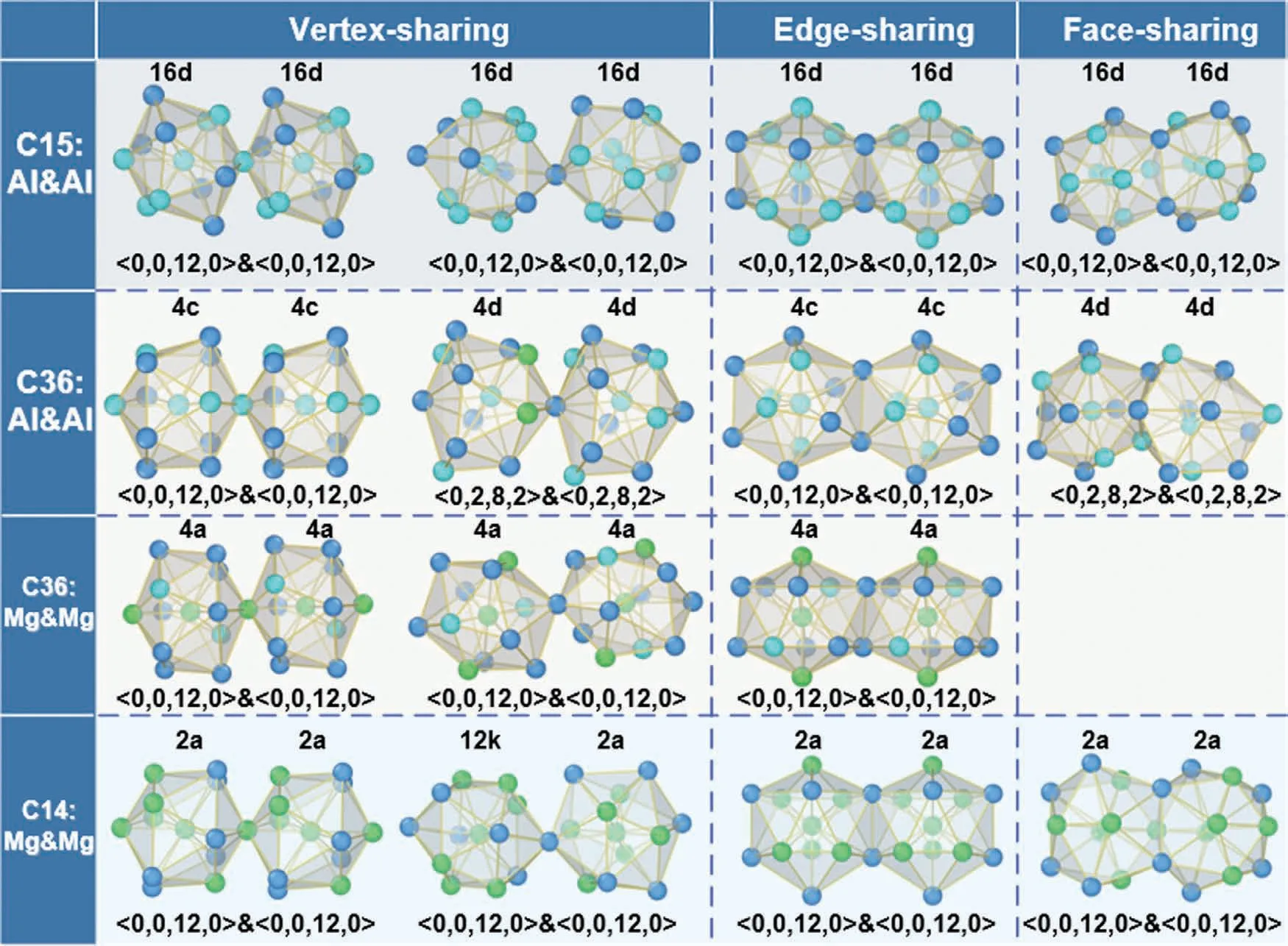
Fig.7.Connection schemes of Al and Al,Mg and Mg centered coordination polyhedra in three Laves phases,which includes C15-Al2Ca,C36-(Mg,Al)2Ca,and C14-Mg2Ca.The symols upper coordination polyhedra represents Wyckoff sites in the corresponding Laves phases,the symbols below coordination polyhedra are designated as Voronoi indices.The light green,cyan,and blue spheres stand for Mg,Al,and Ca atoms,respectively.

Fig.8 represents the evolution of cluster connection schemes in liquid Mg-Al-Ca.As the length scales beyond short range,the nature of connection is complex in the multicomponent liquid,herein the types of shell-atoms in coordination polyhedra differ from one polyhedra to another (white atoms in Fig.8c).Therefore,we did not distinguish the type of shell-atoms in coordination polyhedra and rather characterize the connection schemes by concentrating on the type of centered atom(red atoms in Fig.8c)and the type of coordination polyhedra;that is,the Al-centered crystal-like order (<0,0,12,0>and <0,2,8,2>) together with the Mg-centered crystal-like order (<0,0,12,0>) in liquid Mg-Al-Ca.In this study,polyhedra connectivity is quantified as the number of shared atoms divided by the number of these crystal-like polyhedra.

Fig.8.Connectivity of the (a) Mg-centerd,(b) Al-centerd crystal-like order in liquid Mg-Al-Ca at 1000 K,and (c) schematically showing their connection schemes in liquid.The red and white spheres represent the centered atoms and shell atoms,respectively.

Fig.8a shows that the number of the linked Mg-centered crystal-like order increases monotonously with increasingrCa/Al.However,as depicted in Fig.8b,the connectivity of Al-centered polyhedra increases with therCa/Alranging from 0.33 to 0.74,and then it decreases withrCa/Alincreasing to 1.The different variation tendencies of connectivity arise due to the efficient filling of space with the linked atoms.The variation tendency of polyhedra connectivity is also consistent with the phase transformation process in Mg-Al-Ca.Therefore,the connectivity of coordination polyhedra is also a heredity indicator from liquid to solid.It can be utilized to reflect the phase transformation process as a function ofrCa/Alin Mg-Al-Ca.

3.3. Origin of phase transformation

In the analysis above,we provide an atomic insight into phase transformation in Mg-Al-Ca,i.e.,the crystal-like order identified in liquid is projected to manipulate phase formation.Under the theoretical framework of classical nucleation theory,the presence of crystal-like order could stimulate the formation of corresponding crystal embryo by decreasing the free energy of crystal-liquid interface and surface tension.Once the particle attachment meets the required size of critical crystal embryo,the crystal growth occurs,thus forming the corresponding crystalline phase.The underlying mechanism of the effect of crystal-like order on crystal embryo formation in Mg-Al-Ca is further analyzed in this section.

3.3.1.Efficienttopologicalpacking

As indicated by the CN results for liquid Mg-Al-Ca with different compositions (Section 3.1.2),the values ofZMg,ZAl,andZCain liquid are almost definite around 13,11,and 16,respectively.Note that the CN values of each chemical species in crystalline phases are similar to that of liquid.Specifically,the CN’s for Mg and Al in three Laves phases are 12,the CN for Ca is 16 in C15-Al2Ca and C14-Mg2Ca,and it is 15 for C36-(Mg,Al)2Ca.The origin of similarity in CN values is the structural order tending to achieve dense packing in both liquid and crystalline phases.In this scenario,the local atomic packing in liquid depends strongly on atomic size and their chemistry [44].The solute-centered average CN in liquid is controlled by the effective atomic size ratio(R∗)between the solute and solvent atoms[44].With decreasing R∗,the preferred polyhedra change from the Frank-Kasper type (R∗>1.2) with high coordination to the icosahedron type (R∗<0.902),and then to the bicapped square antiprism type(R∗<0.835),and finally to the tri-capped trigonal prism packing type (R∗<0.732) [45].The atomic radii of Mg,Al,and Ca are 1.60 ˚A,1.43 ˚A,and 1.97 ˚A,respectively [46].The R∗value of Al/Mg is 0.89,corresponding to the icosahedron type.R∗=1.23 for Ca/Mg corresponds to the high coordination Z16 Frank-Kasper type.As for coordination environments of the three crystalline phases,the Al-centered polyhedra are <0,0,12,0>and <0,2,8,2>,indicating the ICO ordering.The Ca-centered Voronoi polyhedra are <0,0,12,3>and <0,0,12,4>,representing the high coordination Z16 Frank-Kasper polyhedra.These results validate that the atomic size dependence of local packing is applicable not only to amorphous structure but also to crystalline compounds,both of which are inclined to reach the densely topological cluster-packing.To be specific,the underlying mechanism of efficient polytetrahedral packing lies in the preferential formation of triangulated faces to diminish rotational symmetry [15].

Furthermore,we illustrate the structural relationship between the ICO and the stable crystalline phases in Mg-Al-Ca.It is suggested that the ICO ordering may suppress crystallization due to its non-crystallographic five-fold symmetry.However,we illustrate that the ICO-type local ordering not only acts as the prominent structural order in liquid Mg-Al-Ca but also is the building block for crystalline packing.This also verifies that the structural orders of liquid and crystalline phases are believed to be identical in some ways to achieve topological packing efficiency.Aside from the topological packing efficiently,it should be noted that therCa/Al(wt.%) in Al2Ca phase is exactly 0.74,which corresponds to the critical phase transition point in Mg-Al-Ca system.Therefore,the observed changes in the local structure of liquid can also be related to such stoichiometry concentration.Consequently,in terms of atomic configurations,the preferable structural order extracted from liquid are not exclusive to amorphous structure,but also act as the structural units for the formation of crystalline embryo.

3.3.2.Chemicalinteractiondependenceofinheritedstructure

In the theoretical framework of topological local ordering,metallic atoms select preferable neighbors to lower total energy [15].Chemical short-range order (CSRO),signifying how far the local chemical order deviates from the expectation of a random solution,is utilized to reflect this kind of chemical ordering in our work.The Warren–Cowley parameter is usually used to quantify the CSRO,defined as [18]:

whereCjis mole fraction ofjspecies atoms in the system,andZi,andZijare total and partial CN with respect toispecies,respectively.A negativeαijdenotes the number ofjspecies in the first-neighbor shell centered byispecies exceeding the average concentration ofjspecies,meaning that atomsiandjfavor the bonding tendency.While a positive value implies the repulsive tendency against thei-jpair.The larger the absolute value of this parameter,the stronger the interaction (attractive or repulsive) between two species of elements.According to the definition of Warren–Cowley CSRO,the ordering parameter is asymmetric,i.e.,αij/=αji.Herein,it is essential to considerαijandαjisimultaneously for judging the CSRO as a function of composition.

Fig.9 shows the evolution of the Warren-Cowley CSRO with increasingrCa/Alfor liquid Mg-Al-Ca at 1000 K.The self-interaction of Mg exhibits a random distribution(αMgMg=0),but Al shows repulsive interaction (αAlAl>0).Both remains essentialy unchanged with increasingrCa/Al.The interaction of Ca-Ca shows a distinct change from a strongly repulsive interaction to a weak extent.The interaction of the Mg-Al pair is repusilve and essentially unchanged with increasingrCa/Al.The attractive interaction of Al-Ca is stronger than that of Mg-Ca as demonstrated by the larger absolute value of Al-Ca.However,the attractive character of Al-Ca diminishes,while that of Mg-Ca intensifies with increasingrCa/Al,especially at the crossover ofrCa/Al=0.74.Consequently,it can be concluded that Ca atoms favor bonding with Al at lowrCa/Al,while the preferable bonding atom is Mg for a higherrCa/Al.

Fig.9.Warren-Cowley chemical short-range order (CSRO) as a function of rCa/Al in the liquid Mg-Al-Ca at 1000 K.

Based on the description of liquid structure in Mg-Al-Ca discussed above,it can be concluded that different solutecentered polyhedras show divergent variation tendency with increasingrCa/Al.The formation of favorable order with different compositions is a consequence of the change of chemical bonding.The non-directional nature of metallic bonding via delocalized electrons leads to even greater flexibility for favorable chemical bonding [15].It has been shown that the diverse variation tendency of CSRO induced by the fluctuation of composition is a chemical driving force for the formation of the inherited crystal-like order.

3.3.3.Effectofdynamicpropertiesonphaseformation

The transition from liquid to crystalline phase entails the atomic arrangement and diffusion.Thus,the effect of atomic diffusion on phase formation needs to be considered.The mean square displacement (MSD) characterizes the displacement of one atom under a certain time interval [47,48]:

Fig.10a-c represents the MSD of Mg,Al and Ca in liquid Mg-Al-Ca,respectively.It shows that the MSD is proportional to the square of time but independent of temperature within the time shorter than around 100 fs,which is expected as ballistic motion and vibration.While above 100 fs,the MSD is characterized by a thermally activated process,as expected for long-range diffusion.Consequently,the results with time over than 500 fs are used to estimate self-diffusion coefficient,as shown in Fig.10d.With increasingrCa/Al,the self-diffusion coefficient of Al decreases monotonously.However,the selfdiffusion coefficients of Mg and Ca decrease at first and then increase with an crossover atrCa/Al=0.74.The increasing diffusion ability provides more opportunities for atomic arragement during the process of phase formation,indicating the larger kinetic driving force for the formation of Mg2Ca embryo whenrCa/Alexceeds 0.74.

Fig.10.MSD of (a) Mg,(b) Al,and (c) Ca in liquid Mg-Al-Ca alloys at different Ca/Al ratios and (d) the corresponding calculated self-diffusion coefficients.

4.Conclusions

In terms of AIMD simulations,we reveal the internal structural order in the Mg-Al-Ca liquid with respect to therCa/Alratio and compare them with those of the three Laves phases,C15-Al2Ca,C36-(Mg,Al)2Ca,and C14-Mg2Ca.We demonstrate the structural similarity between liquid and solid in the viewpoint of global atom distribution,local arrangement environment,and connection schemes of polyhedra.It is observed that with therCa/Alratio increasing from 0.33 to 1,the Mg2Ca-like structural order in liquid increases monotonically.While the Al2Ca-like structural order increases at first and then decreases at the crossover ofrCa/Al=0.74,which also corresponds to the experimental composition transition from C15-Al2Ca to C14-Mg2Ca.We unveil that the underlying mechanism of this transition is the necessity of dense packing of atomic configurations in both liquid and crystal,and satisfies the evolution of chemical interaction with respect to compositions.Meanwhile,the increasing diffusion abilities of Mg and Ca whenrCa/Alexceeds 0.74 provide larger kinetic driving force for the formation of Mg2Ca embryo.Since the liquid ordering is independent of materials processing history,we suggest that the frequency of crystal-like orders in liquid is a potential parameter to predict phase formation in the alloy of interest.

Declaration of competing interest

None.

Acknowledgements

Financial supports from The National Natural Science Foundation of China (Nos.52074132,51625402,and U19A2084) are greatly acknowledged.Partial financial support came from The Science and Technology Development Program of Jilin Province (Nos.20200401025GX and 20200201002JC),The Central Universities,JLU,Program for JLU Science and Technology Innovative Research Team(JLUSTIRT,2017TD-09).The authors at Penn State acknowledge the finacial support from the U.S.Department of Energy via Award number DE-NE0008945.We gratefully acknowledge HZWTECH for providing computation facilities.

Supplementary materials

Supplementary material associated with this article can be found,in the online version,at doi:10.1016/j.jma.2021.11.024.