Validation and error analysis of wave-modified ocean surface currents in the northwestern Pacific Ocean*

Zhenli HUI , Ying LI , Jia SUN , Long YU , Xia JU , Xuejun XIONG ,**

1 First Institute of Oceanography, and Key Laboratory of Marine Science and Numerical Modeling, Ministry of Natural Resources,Qingdao 266061, China

2 Laboratory for Regional Oceanography and Numerical Modeling, Pilot National Laboratory for Marine Science and Technology(Qingdao), Qingdao 266237, China

3 Shandong Key Laboratory of Marine Science and Numerical Modeling, Qingdao 266061, China

Abstract By incorporating the wave-induced Coriolis-Stokes forcing into the classical Ekman layer, the wave-modified ocean surface currents in the northwestern Pacific Ocean were estimated. Thus, the ocean surface currents are the combination of classical Ekman current from the cross-calibrated multi-platform(CCMP) wind speed, geostrophic current from the mean absolute dynamic topography (MADT), and waveinduced current based on the European Centre for Medium-Range Weather Forecasts (ECMWF) Interim Re-Analysis (ERA-Interim) surface wave datasets. Weight functions are introduced in the Ekman current formulation as well. Comparisons with in-situ data from Lagrangian drifters in the study area and Kuroshio Extension Observatory (KEO) observations at 32.3°N, 144.6°E, and 15-m depth indicate that wave-modified ocean surface currents provide accurate time means of zonal and meridional currents in the northwestern Pacific Ocean. Result shows that the wave-modified currents are quite consistent with the Lagrangian drifter observations for the period 1993-2017 in the deep ocean. The correlation (root mean square error, RMSE) is 0.96 (1.45 cm/s) for the zonal component and 0.90 (1.07 cm/s) for the meridional component. However, wavemodified currents underestimate the Lagrangian drifter velocity in strong current and some off shore regions,especially in the regions along the Japan coast and the southeastern Mindanao. What’s more, the wavemodified currents overestimate the pure Eulerian KEO current which does not consider the impact of waves,and the zonal (meridional) correlation and RMSE are 0.95 (0.90) and 11.25 cm/s (12.05 cm/s) respectively.These comparisons demonstrate that our wave-modified ocean surface currents have high precision and can describe the real-world ocean in the northwestern Pacific Ocean accurately and intuitively, which can provide important routes to calculate ocean surface currents on large spatial scales.

Keyword: ocean surface current; Ekman current; geostrophic current; Stokes drift

1 INTRODUCTION

Ocean circulation is the basic framework of the entire ocean, and ocean surface current is the primary grip of the circulation system. It is the aperiodic flow of massive ocean water and is one of the main factors aff ecting ship’s navigation, as well as one of the main transporters of ocean heat, salt, and chlorophyll(Boccaletti et al., 2005; Sikhakolli et al., 2013a, b).Moreover, long-term variations of ocean surface currents are very important to the climate change and climate studies (Dohan and Maximenko, 2010; Lee et al., 2010; Dohan, 2017; Du et al., 2021), which have attracted extensive attention from scholars at home and abroad in recent years. Ocean surface currents are also important for tracing the trajectories of sea ice drift (Tang and Gui, 1996), fish eggs and larvae(Brickman and Frank, 2000; Reiss et al., 2000), the dispersal of off shore oil spills and other pollutants(Morinta et al., 1997), which can also provide important reference for the search and rescue of floating objects (Smith et al., 1998). For example,after the tsunami wave near Fukushima in 2011, ocean surface currents have been used to trace the debris(Matthews et al., 2017). And ocean surface current information in the southern Indian Ocean has also been used to track the trajectories of Malaysian Airlines Flight MH370 floating debris (Trinanes et al., 2016; Gao et al., 2018). Furthermore, ocean surface currents are fundamental for quantifying the role of horizontal advection on the surface heat budget in diff erent regions (Picaut et al., 2002; Sudre and Morrow, 2008). And the onset and evolution of El Niño events as well as the ocean response to the North Atlantic Oscillation can also be investigated by ocean surface currents (Esselborn and Eden, 2001; Lagerloef et al., 2003).

The above analyses indicate that the continuous,high precision ocean surface currents are essential for various applications. However, ocean surface currents from in-situ observations in the past have very limited observations and coarse spatial resolutions, which have been observed from ships, floating buoys, and a small number of current meter moorings (Reverdin et al., 1994; Frankignoul et al., 1996; Sikhakolli et al.,2013a, b). Satellite-tracked Lagrangian drifters provide large amount of near-surface ocean surface current observations since 1979, however, their spatial and temporal distributions are quite uneven,which are easily captured by the strong current areas either. Therefore, calculating ocean surface currents on large scales solely from in-situ observations are inadvisable. With the rapid development of satellite remote sensing technology since 1970s and 1980s,combining satellite data with dynamics method becomes an important route to calculate ocean surface currents on large spatial scales (Hui and Xu, 2016).Many scientific researchers have done related works.Lagerloef et al. (1999) have proposed a two parameter regression model in the tropical Pacific Ocean, in which ocean surface current is calculated based on the absolute dynamic topography and surface wind datasets. The results are compared well with in-situ observations from Lagrangian drifters and equatorial current moorings. Based on the QuikSCAT wind stress and AVISO sea surface height data, the global ocean surface current spanning from 2000 to 2008 has been retrieved (Sudre and Morrow, 2008; Sudre et al.,2013), and the spatial resolution is 0.25°×0.25°. The global comparisons between this satellite-derived currents and Lagrangian drifter observations as well as Acoustic Doppler Current Profiler current observations show a very good agreement in the subtropical and mid-latitude bands. For most of the world oceans, the correlation is about 0.7 for the zonal means, while in the regions of strong boundary currents, this correlation almost exceed 0.8 both for the zonal and meridional components. Besides, by combining sea surface temperature data of advanced very high resolution radiometer (AVHRR) and in-situ observations, TOPEX/Poseidon altimeter data and the special sensor microwave/imager (SSM/I)scatterometer surface wind dataset, Bonjean and Lagerloef (2002) have investigated a diagnostic model in the tropical Pacific Ocean based on the quasi-linear and steady physics. The results are well adapted to equatorial Tropical Ocean-Atmosphere(TAO) current meters as well as buoy drifter observations at 15-m depth. Surface current products formed using this method of the global ocean are known to be the Ocean Surface Current Analysis Real-Time (OSCAR), which have been widely used up to now. These studies indicate that satellite-derived ocean surface currents can describe the real world ocean flow objectively and accurately.

In spite of this, traditional ocean surface currents calculated from the geostrophic current and classical Ekman current have some drawbacks, as there are some discrepancies between the classical Ekman component and in-situ observations (Price and Sundermeyer, 1999; Lewis and Belcher, 2004; Polton et al., 2005; Wu and Liu, 2008; Song, 2009).Therefore the classical Ekman current has to be modified. Some studies have shown that surface waves, especially the Stokes drift can change the nature of the Ekman layer qualitatively and determine the wind-driven current profile. As a common phenomenon on the ocean surface, the Stokes drift is produced by the surface waves, and the direction of which is consistent with that of the wave propagation(Stokes, 1847; Liu et al., 2007). Generally, the Stokes drift velocity is the diff erence between the average Lagrangian flow velocity of a fluid parcel and the average Eulerian flow velocity of the fluid (van den Bremer and Breivik, 2018; van Sebille et al., 2020),and recent theoretical developments have been made for the solutions of Stokes drift velocities in the equatorial region (Henry, 2019). Furthermore, the main areas of application of the Stokes drift have also been discussed in the study of van den Bremer and Breivik (2018), which include the eff ects of surface Stokes drift on the Eulerian ocean models.Previous studies have indicated that the Coriolis-Stokes forcing generated through the interaction between the Stokes drift and planetary vorticity will have a great impact on the classical Ekman model(Hasselmann, 1970). These studies indicate that the classical Ekman current can be improved by considering the impact of waves (Stokes drift). By taking the wave-induced Coriolis-Stokes forcing into account, both the mean flow and depth of the mixed layer are improved, which proves to agree much better with observations than the classical Ekman model (Lewis and Belcher, 2004; Polton et al., 2005).On the basis of the above studies, Hui and Xu (2016)have incorporated the wave-induced Coriolis-Stokes forcing into the classical Ekman model and confirmed that the improved wave-modified satellite-derived ocean surface currents agree much better with Lagrangian drifter observations than that without waves. Especially in the Southern Ocean region between 40°S and 65°S, 90% and 91% of the results have been improved for the zonal and meridional component respectively. In this paper, we estimate the wave-modified ocean surface currents in the northwestern Pacific Ocean based on the research of Lagerloef et al. (1999) and Hui and Xu (2016).Besides, more improvements are made with weight functions introduced in the Ekman formulation according to the study of An et al. (2013), and the data sources are with much higher temporal resolutions. The results are validated by comparing with in-situ data from Lagrangian drifter currents and Kuroshio Extension Observatory (KEO) observations,all of which have not been studied before and will certainly have very important values in the field of surface circulation research.

The paper is organized as follows. Section 2 lists the material we use and the method to calculate each part of the ocean surface currents as well as the error analysis in detail. Section 3 gives our result. Section 4 presents the conclusions. And finally, Section 5 provides the data availability statement.

2 MATERIAL AND METHOD

2.1 Material

2.1.1 MADT data

The daily mean absolute dynamic topography(MADT) data provided by Copernicus Marine and Environment Monitoring Service (CMEMS) are used to calculate the surface geostrophic current. This L4 level product is processed by the sea level thematic center (SL-TAC) multi-mission altimeter data processing system, which merges multiple altimeter missions available at a given time (Jason-1/2/3,Sentinel-3A, HY-2A, and so on). The spatial resolution is 0.25° both on its latitudinal and longitudinal scales.We assume that this variable is consistent throughout the whole day and can be used to retrieve the 6-hourly geostrophic current in the analysis.

2.1.2 Cross-calibrated multi-platform (CCMP) ocean surface wind vector

The CCMP wind analysis provides global surface wind vectors with high spatial and temporal resolutions. In addition, the time period is from 1987 to the present. These wind products consist of a 6-hourly gridded ocean surface wind analysis, with the spatial resolution 0.25°×0.25°. The CCMP wind products have been updated using improved and additional input data, which is produced by a variational analysis method (VAM) and is a merged product ofin-situ wind observations (moored buoy wind data), satellite remote sensing data (QuikSCAT,ASCAT scatterometer wind vectors, and Version-7 RSS radiometer wind speed), and reanalysis European Centre for Medium-Range Weather Forecasts(ECMWF) Interim Re-Analysis (ERA-Interim)surface wind fields. In this paper, we use its datasets from 1 January 1993 to 31 December 2017 to calculate the classical Ekman current.

2.1.3 ERA-Interim surface wave data

The ERA-Interim surface wave datasets (mean wave direction, mean wave period, and significant height of combined wind waves and swell) provided by the European Center for Medium-Range Weather Forecasts (ECMWF) are used to retrieve the surface Stokes drift and wave-induced current in this paper.The data are available at 6 hour and at 0.25°×0.25°spatial resolution. This dataset covers the period from 1979 to the present and we use its time scales spanning from 1 January 1993 to 31 December 2017.

2.1.4 Lagrangian drifter observation

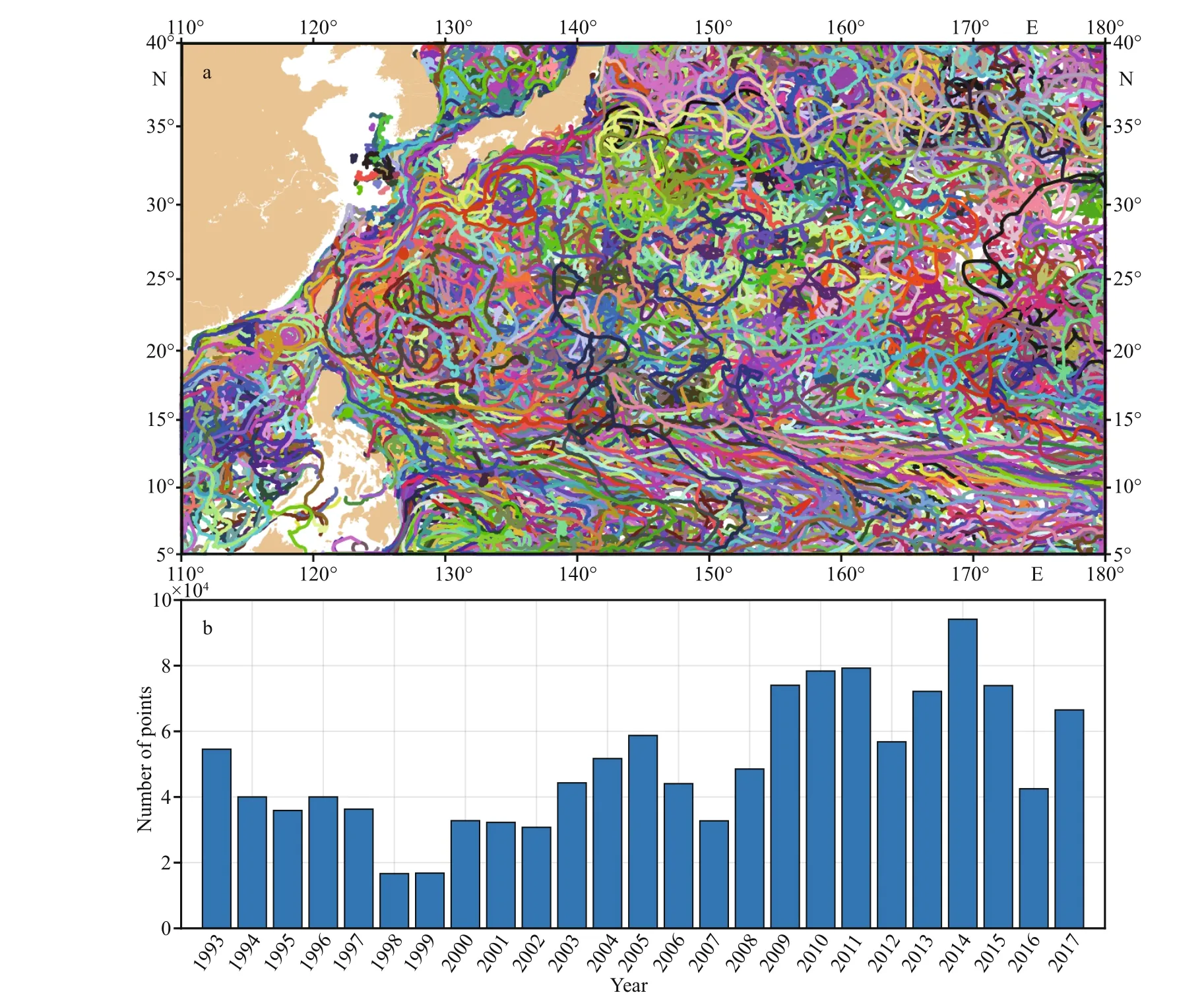
Fig.1 Lagrangian drifter observations from 1993 to 2017 in the northwestern Pacific Ocean

We use the quality controlled in-situ Lagrangian drifter observations from the Global Drifter Program(GDP) to validate the wave-modified ocean surface currents from 1 January 1993 to 31 December 2017.This dataset provides 6 hourly near-surface current observations from the satellite-tracked drifters at each point. To reduce the errors of the downwind slip to～0.1% of the wind speed for winds higher than 10 m/s,a drogue has been centered at 15-m depth for each drifter (Niiler and Paduan, 1995; Lumpkin and Pazos,2007; Hui and Xu, 2016). The loss of this drogue will increase the downwind slip ～1%-1.5% of the wind speed (Pazan and Niiler, 2001). In this study, after a manual re-evaluation of drogue presence, we only select the drogued drifters (Lumpkin and Johnson,2013; Lumpkin et al., 2013). From 1993 to 2017, we collected 2 759 floating buoys in the northwestern Pacific Ocean, which include 1 253 917 current profiles in total (Fig.1). The drifter trajectories are shown in Fig.1a. In addition, Fig.1b shows numbers of available current profiles in each year from 1993 to 2017, which displays that the number of current profiles within any one year is from 16 670 to 94 137.For a single drifter, the longest lasted for 1 276 days from 6 August 2009 to 15 February 2013. These drifter observations are quality controlled and have been interpolated via Kriging to regular 6-h intervals(Hansen and Poulain, 1996).

2.1.5 KEO observation

The Ocean Climate Stations (OCS) KEO surface mooring is located at 32.3°N, 144.6°E, south of the Kuroshio Extension Current, which was first deployed in June 2004. This dataset provides zonal and meridional current components at 5-, 15-, and 25-m depth from 30 May 2005 to 31 December 2017. In this paper, we use its data at 15-m depth to validate our wave-modified ocean surface currents in the northwestern Pacific Ocean, about 3 771 current profiles in total.

2.2 Method

In this paper, we refer to the method mentioned in Lagerloef et al. (1999) and Hui and Xu (2016) to calculate the wave-modified ocean surface currents in the northwestern Pacific Ocean, which consist of three terms, the geostrophic current (Ug) from the MADT, the classical Ekman current (Ue) from the CCMP wind speed, and the wave-induced current(Uw) from the ERA-Interim reanalysis surface wave dataset. Weight functions ofF10andF25are introduced in the Ekman current formulation as well based on the study of An et al. (2013). The total ocean surface currents can be calculated as the sum of the three vectors, which can be expressed as

2.2.1 Geostrophic current

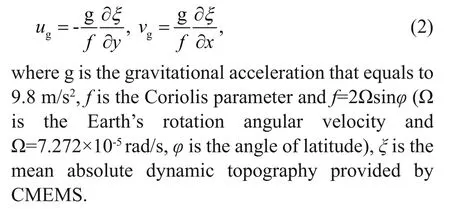
The zonal (ug) and meridional (vg) geostrophic velocities in the northwestern Pacific Ocean outside the equatorial regions can be calculated as

2.2.2 Classical Ekman current

In this part, we use the surface wind stress data to calculate the classical Ekman current at 15-m depth based on the two parameter regression model proposed by Lagerloef et al. (1999) as

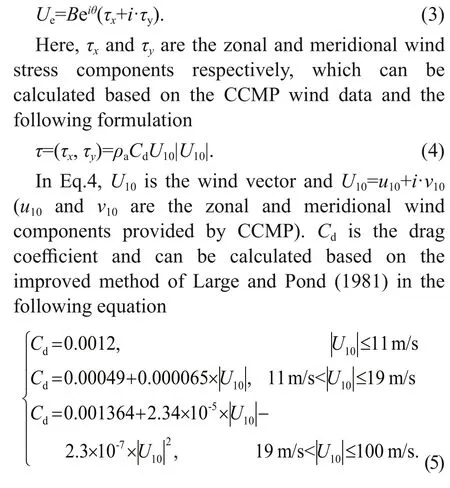
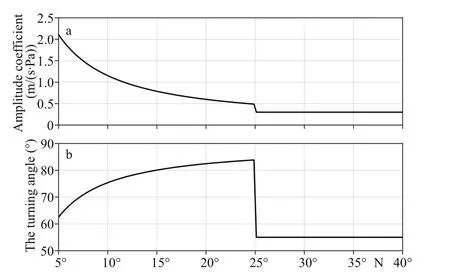
Fig.2 The amplitude coeffi cient B (a) and the turning angle of the classical Ekman current relative to the wind (b)

Furthermore, in Eq.3,θis the deflection of Ekman flow relative to the wind direction,Bis the amplitude coeffi cient. Assuming constant eddy viscosity,classical Ekman theory indicates that the wind drift spiral and decay with depth, andθis 45° on the ocean surface. Bressan and Constantin (2019) have proposed a perturbative approach, which provide a formula for the deviation of the deflection angle from the 45° reference value. Constantin et al.(2020) have investigated the deflection angle of wind drift relative to the surface wind stress based on the vertically varying eddy viscosity. In this paper, we did not use such a sophisticated analysis.Instead, to compare directly with ocean surface current at 15-m depth, we use the regression results in Lagerloef et al. (1999), which is calculated based on satellite data and in-situ observations. In the regions between 5°N and 25°N,Bandθcan be estimated as

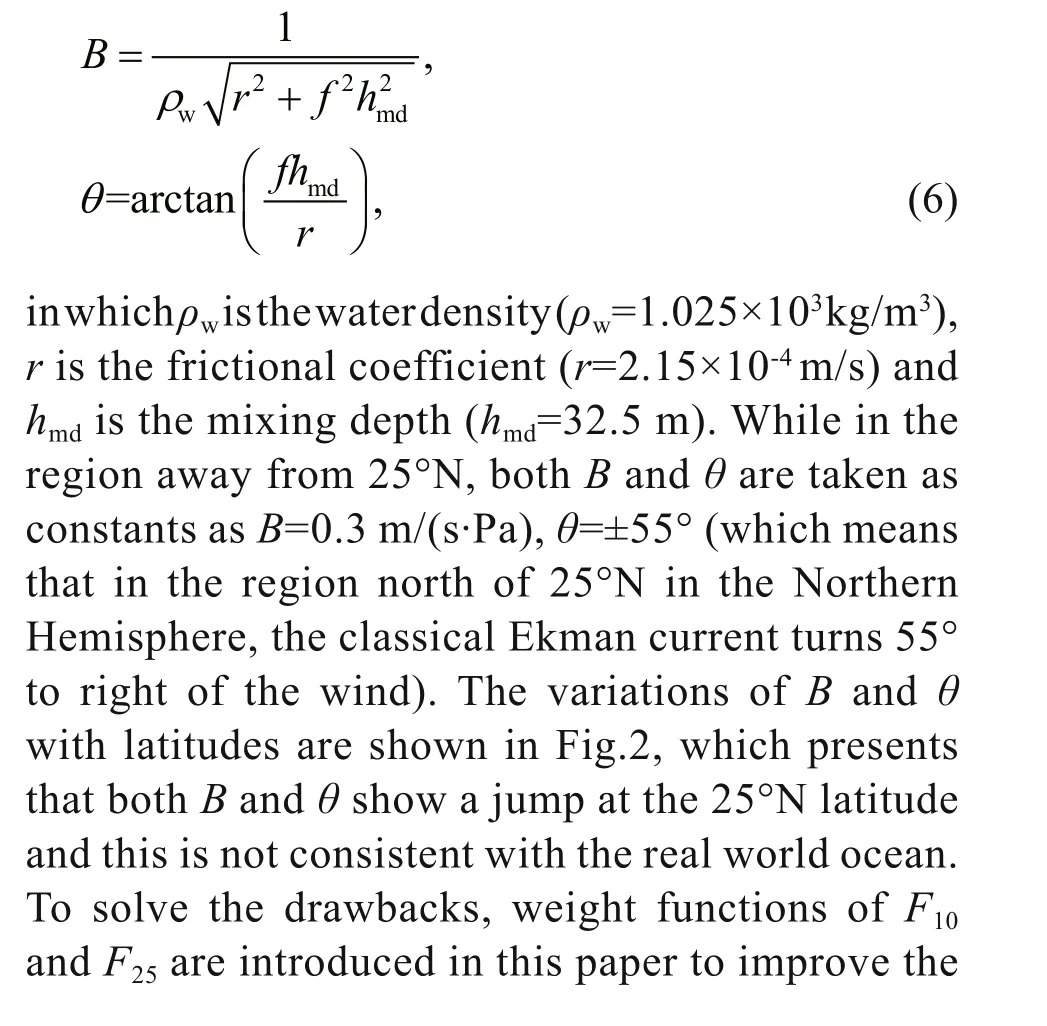
Fig.3 Weight coeffi cients of the Ekman formulation

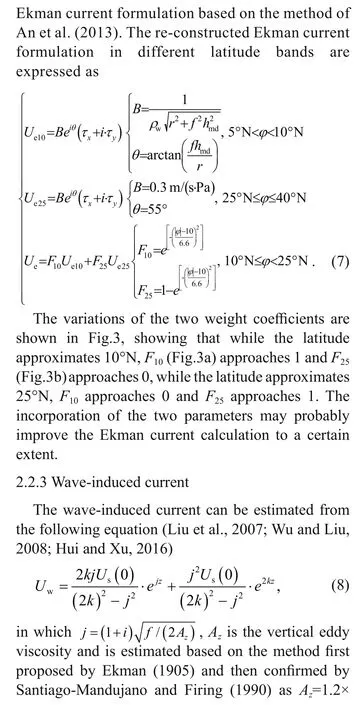
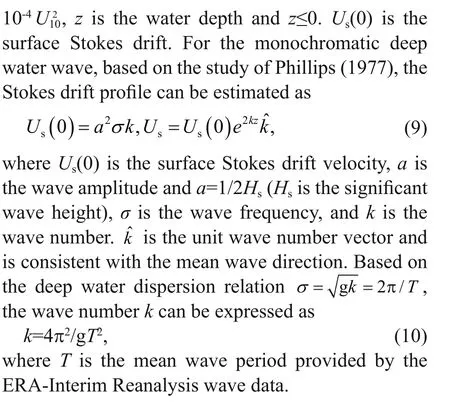
2.2.4 Error analysis

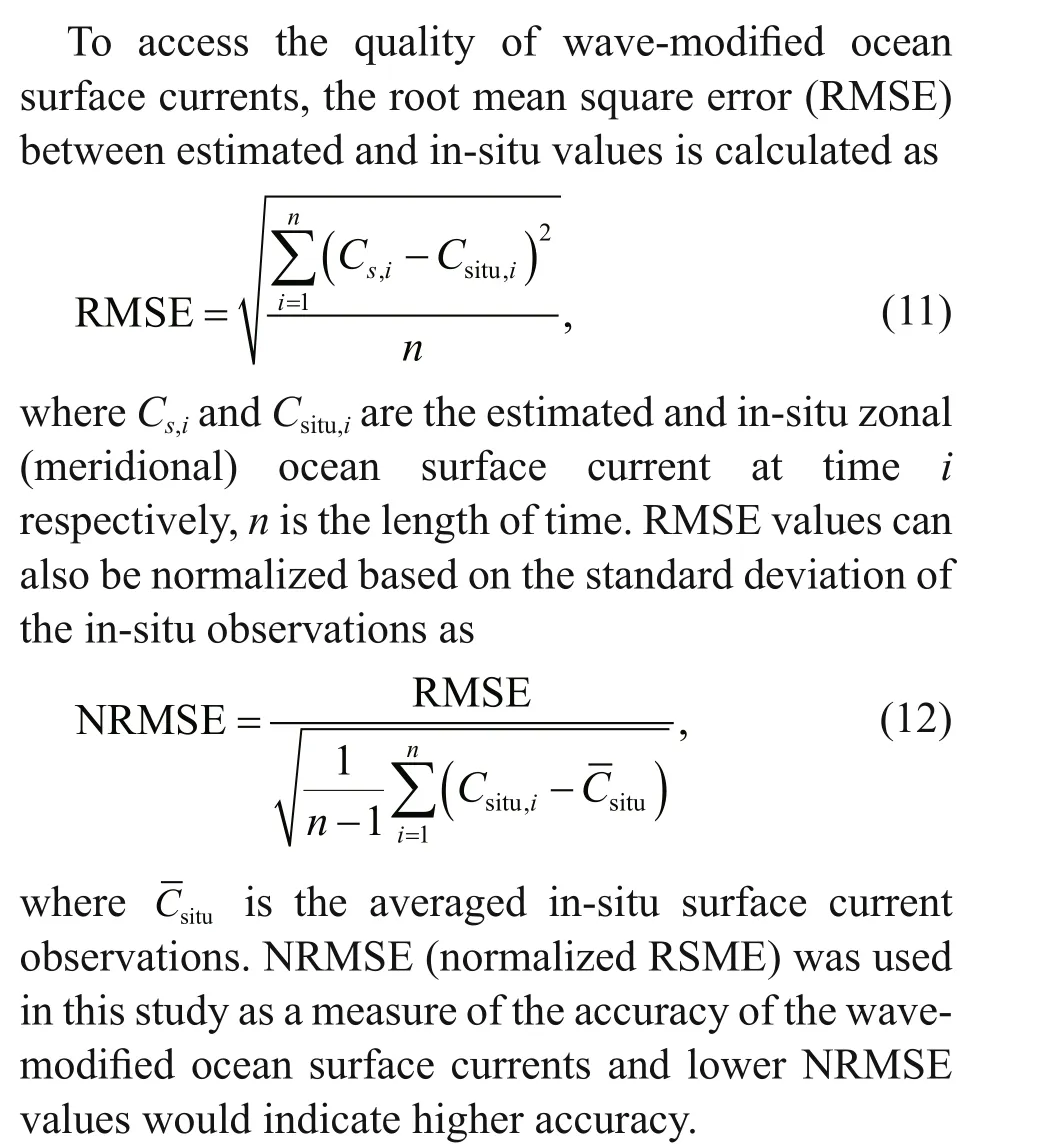
3 RESULT

The 6-hourly, 0.25°×0.25° gridded ocean surface currents with wave eff ects in the northwestern Pacific Ocean are estimated based on the methods in Section 2.2, which include geostrophic current from the MADT, classical Ekman current from the CCMP wind speed, and the wave-induced current estimated from the ERA-Interim surface wave datasets. This wave-modified ocean surface currents are validated by comparing with in-situ data from Lagrangian drifters in the study area and KEO observations(32.3°N, 144.6°E) moored at 15-m depth. Before comparison, the gridded wave-modified ocean surface currents are temporal and spatial interpolated to each drifter current vector and KEO observations both for zonal and meridional components. Detailed analysis are shown in Sections 3.1 and 3.2.

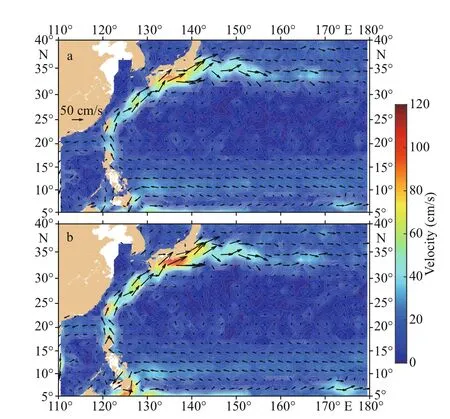
Fig.4 Ocean surface currents averaged from 1993 to 2017

Fig.5 Correlations between wave-modified ocean surface currents and Lagrangian drifter observations from 1993 to 2017

3.1 Comparison with Lagrangian drifter

In this section, validations are performed using Lagrangian drifter observations in the northwestern Pacific Ocean from 1 January 1993 to 31 December 2017. To present the spatial distributions of the comparison results, we have binned all the corresponding data into 1°×1° resolution boxes.Figure 4 is the averaged result, which presents that wave-modified ocean surface currents (Fig.4a) agree well with drifter observations (Fig.4b) in most of the northwestern Pacific Ocean, especially in deep water regions away from the coast, where ocean surface currents are barely aff ected by the terrain. Furthermore,the Kuroshio flow path at the depth of 15 m is well characterized, which is quite similar between wavemodified currents and drifter observations. However,there still exist some discrepancies. The wavemodified ocean surface currents underestimate in-situ drifter observations in amplitude, while current directions are almost consistent. Especially in the Luzon Strait, northeast of Taiwan, China, as well as regions along the south coast of the Honshu island and southeast of the Mindanao. There are two possible reasons for this phenomenon. One is that drifter velocity is a combination of a series of current vectors,which includes geostrophic current and ageostrophic flow. Except for the classical Ekman current and the wave-induced current, this ageostrophic flow also contains many other current terms, which cannot be retrieved using these limited datasets mentioned in this article alone. And another reason is that these regions are near the coast and terrain is an important factor aff ecting the accuracy of ocean surface currents,which needs to be taken into account in the future analysis.

To evaluate the quality of the wave-modified current products, we have estimated correlation and NRMSE in each grid point. To reduce the errors, only eff ective drifter observations exceeding 30 in each grid point are used. The zonal and meridional correlations between 6-hourly wave-modified ocean surface currents and Lagrangian drifter observations from 1993 to 2017 are shown in Fig.5a-b respectively.Result shows that in the northwestern Pacific Ocean,the zonal (meridional) correlation exceeding 0.5 occupies about 96.9% (92.3%) of the ocean, and zonal correlations are higher than the meridional component for most of the ocean, especially in the Kuroshio and Kuroshio Extension regions. This is probably caused by the strong geostrophic current,which is the main current component in this area and the method for calculating this velocity is particularly mature. While for the ageostrophic current, there are still some complex unknown elements, which need to be explored in the future studies.

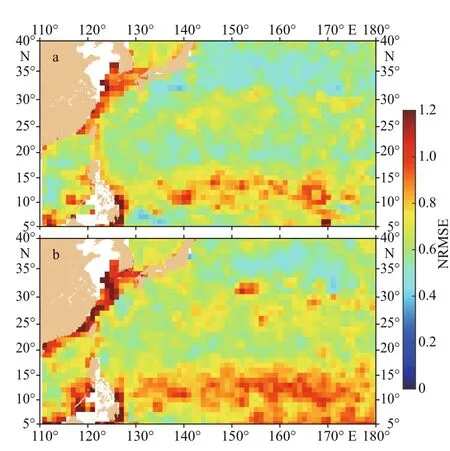
Fig.6 NRMSE between wave-modified ocean surface currents and Lagrangian drifter observations from 1993 to 2017

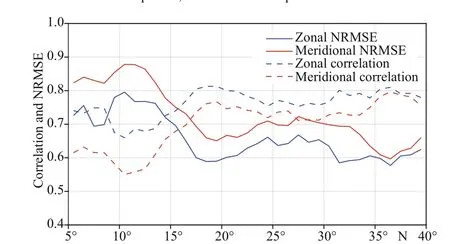
Fig.7 Zonal average of the correlation and NRMSE between wave-modified ocean surface currents and Lagrangian drifter observations

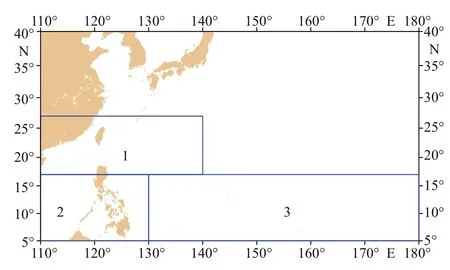
Fig.8 Three selected regions (1, 2, and 3) of the northwestern Pacific Ocean

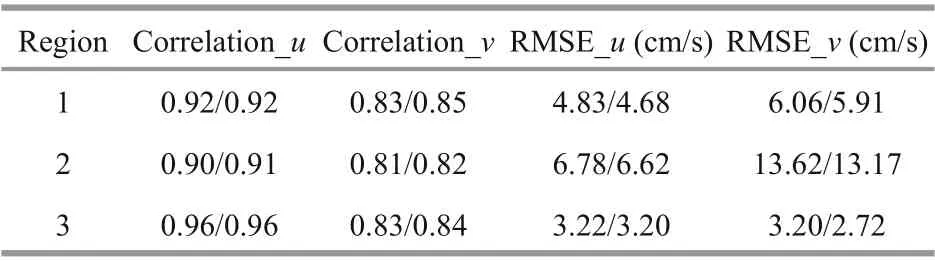
Table 1 Correlation and RMSE between satellite-derived ocean surface currents and Lagrangian drifter observations of the three representative regions

To assess and compare the errors between estimated ocean surface currents and in-situ observations in each grid point, we have estimated the NRMSE between wave-modified ocean surface currents and Lagrangian drifter observations for the same period(Fig.6). The lower NRMSE indicates that the estimated wave-modified ocean surface currents fluctuate within a much smaller range of drifter observed values. Result shows that both for the zonal and meridional components, the NRMSE is lower than 0.8, with much higher values in the low-latitude bands and some off shore regions. What’s more, the zonal NRMSE is considerably lower than the meridional component for most of the ocean, which means that the zonal error is smaller than the meridional component. Figure 6 also shows that the pattern of NRMSE is very similar with that of the correlation coeffi cient (Fig.5), but presents good negative correlation characteristics. This suggests that the larger the correlation, the smaller the error. To better express the corresponding relationship between correlation and NRMSE, we have shown zonal average of the two validation parameters (Fig.7),which shows that both for the zonal and meridional components, the correlation (NRMSE) is higher(lower) than 0.55 (0.88). In addition, the zonal correlation (NRMSE) is considerably higher (lower)than the meridional component in the study area.Furthermore, the variation of correlation is negatively correlated with that of the NRMSE, and the correlation coeffi cient is 0.97 (0.99) for the zonal (meridional)component. The two parameters indicate that our wave-modified ocean surface currents agree well with Lagrangian drifter observations. Moreover, the quality of wave-modified zonal component is relatively higher than that of the meridional component.

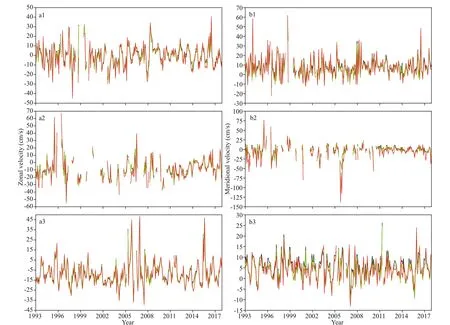
Fig.9 Time series of the monthly averaged ocean surface currents without waves (black solid line), wave-modified ocean surface currents (green solid line) and drifter observations (red solid line) of the three representative regions

According to the spatial distribution characteristics of the correlation and NRMSE, three representative regions in the study area are selected to do detailed analysis (Fig.8). To better understand the eff ects of surface waves, monthly averaged wave-modified ocean surface currents as well as ocean surface currents without waves and Lagrangian drifter observations within each region are calculated (Fig.9),in which left panels (Fig.9a1-a3) are the zonal velocity and right panels (Fig.9b1-b3) are the meridional velocity. Table 1 shows the comparison results of the correlation and RMSE in each region.Our estimates present that considering the impact of waves, the correlation (RMSE) in each region has been increased (decreased), which means that the wave-modified currents are better adapted to in-situ drifter observations than that without waves both for the zonal and meridional components. Nevertheless,the zonal correlation (RMSE) is considerably higher(lower) than that of the meridional component, which means that the coincidence for the zonal component is much better than that of the meridional component.Furthermore, Fig.9 also shows that the wave-modified ocean surface currents underestimate drifter observations, especially for the meridional component.We estimate that the 1993-2017 averaged velocity in regions 1-3 is 12.77, 17.52, and 14.00 cm/s,respectively, while for the drifter observations, the velocities are 15.02, 21.19, and 14.07 cm/s, respectively.This underestimation is caused by the complex components of drifter observations, which cannot be calculated by finite velocity components in this study.This needs to be revised in the future analysis.

Fig.10 The comparison between calculated ocean surface currents and the drifter (ID number 81961) with the maximum life span in the northwestern Pacific Ocean

Fig.11 Comparisons of the estimated ocean surface currents with KEO observations located at 32.3°N, 144.6°E, 15-m depth

Besides, the drifter (ID number 81961) with the maximum life span in the northwestern Pacific Ocean is selected to validate the wave-modified ocean surface currents estimated in this paper, which has 5 076 6-hourly current data points in total and the operating date is from 6 August 2009 to 15 February 2013. The drifter’s trajectory is shown in Fig.10a. In addition, the zonal and meridional scatterplots of ocean surface currents without waves as well as wavemodified ocean surface currents versus drifter observations are shown in Fig.10b-c & d-e,respectively. This comparison result shows that both the zonal and meridional components of wavemodified ocean surface currents (Fig.10d-e) coincide better with this Lagrangian drifter observations than that without wave eff ects. Considering the impact of waves, the correlation (RMSE) has increased(decreased) from 0.85 (17.33 cm/s) to 0.86 (16.52 cm/s)for the zonal component and from 0.83 (16.01 cm/s) to 0.84 (15.65 cm/s) for the meridional component. The much higher correlation and lower NRMSE for the zonal component is consistent with the above analysis.Furthermore, the higher RMSE and NRMSE for this comparison may have two important reasons. One is that some floating areas of this selected drifter are near the coast, and terrain becomes an important factor aff ecting the accuracy of wave-modified ocean surface currents. The other is that the ocean surface circulation in the Kuroshio and Kuroshio Extension regions are considerably stronger, thus drifter velocities have been underestimated much larger than low current regions.

The comparison between wave-modified ocean surface currents and Lagrangian drifter observations indicates that wave-modified ocean surface currents can generally describe the real world ocean circulation objectively and accurately in the northwestern Pacific Ocean, especially in deep water regions for the zonal component. While in the strong current and off shore regions, especially for the meridional component, the deviations are relatively larger, which need further improvements in our further research. Diff erent correction parameters can be set aiming at diff erent velocity intervals to reduce the errors between wavemodified ocean surface currents and in-situ drifter observations. What’s more, as the classical Ekman current representing 15-m depth in this study is derived under the hypothesis ofinfinite water depth,terrain eff ect in off shore regions should be taken into account in the future analysis as well.

3.2 Comparison with KEO observations

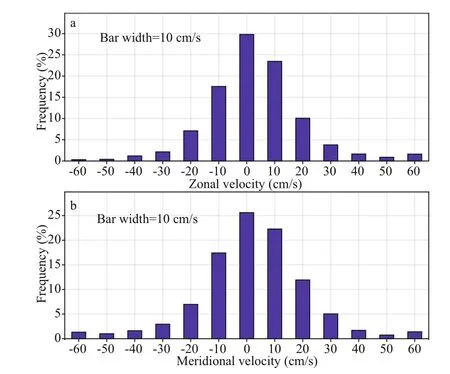
Fig.12 Histograms of the diff erence between wave-modified ocean surface currents and KEO observations

Table 2 Correlation and RMSE between the estimated ocean surface currents and KEO observations

In this part, the estimated ocean surface currents without waves as well as wave-modified ocean surface currents are compared with KEO observations at 15-m depth from 30 May 2005 to 31 December 2017, with 3 771 available current points in total.Time series of the monthly averaged ocean surface currents is shown in Fig.11. Result shows that both the zonal (Fig.11a) and meridional (Fig.11b)components of the estimated ocean surface currents are consistent with the corresponding KEO observations. However, the direct comparison of the time series both for the zonal and meridional components shown in Fig.11 cannot clearly illustrate the eff ects of surface waves. Therefore, we provide the correlation and RMSE between the ocean surface currents without waves as well as wave-modified ocean surface currents versus KEO observations,which are listed in Table 2 in detail. Result shows that the correlation (RMSE) is 0.95 (11.12 cm/s) for the zonal component and 0.90 (12.00 cm/s) for the meridional component between ocean surface currents without waves and KEO observations. While for the wave-modified ocean surface currents, the RMSE is 11.25 cm/s for the zonal comparison and 12.05 cm/s for the meridional comparison. This result manifests that considering the impact of waves, the error between the estimated ocean surface currents and KEO observations becomes larger. This is probably due to the fixed point observations of the KEO, which merely represents the Eulerian current and does not consider the impact of waves.

Histograms of diff erences between wave-modified ocean surface currents and KEO observations are presented in Fig.12. Result shows that percentages of the diff erence approaching zero are the highest both for zonal and meridional components, which is 29.81% and 25.62% respectively. In addition, the diff erence approaching ±10 cm/s takes the second place, which is 41.02% and 39.70%. The diff erence between ±40 and ±60 cm/s is 6.05% (7.80%) for the zonal (meridional) component. High rates within a smaller error range (lower than ±20 cm/s) for the zonal (88.01%) and meridional (84.22%) components means that our wave-modified ocean surface currents can better describe the zonal and meridional components of the KEO observations, especially for the zonal component.

4 CONCLUSION

By incorporating the wave-induced Coriolis-Stokes forcing into the classical Ekman model, wavemodified ocean surface currents in the northwestern Pacific Ocean were calculated based on the CMEMS MADT, CCMP wind speed, and ERA-Interim surface wave datasets from 1 January 1993 to 31 December 2017. Weight functions ofF10andF25were introduced in the Ekman current formulation as well. The current products were validated by comparing the in-situ data from Lagrangian drifters and KEO observations moored at 32.3°N, 144.6°E, 15-m depth.

Result shows that the modified ocean surface currents are in good agreement with in-situ observations, especially for the zonal component. The comparison with Lagrangian drifter observations indicates that by considering the impact of waves, the estimated ocean surface currents have been improved compared with that without waves. Moreover, the zonal (meridional) correlation exceeding 0.5 occupies about 96.9% (92.3%) of the ocean. Furthermore, the monthly comparison with KEO observations at 15-m depth demonstrates that the wave-modified currents are consistent with KEO observations and the zonal(meridional) correlation and RMSE are 0.95 (0.90)and 11.25 cm/s (12.05 cm/s) respectively. Even so,there still exist some discrepancies. Our wavemodified ocean surface currents underestimate the Lagrangian drifter velocities, especially in the strong current and some off shore regions, but overestimate the KEO observations in some individual months.The reasons are as follows. Drifter velocity represents the Lagrangian motion, which contains a complex set of current components. However, some of these components have not been included in our wavemodified products except for the classical Ekman current, geostrophic current, and the wave-induced current, all of which needs further research in the future studies. Furthermore, this discrepancy between wave-modified currents and drifter observations is mainly situated in off shore regions, where complex topography and shallow waters may have a great impact on the accuracy of ocean surface currents retrieval. The KEO observations are the Eulerian mean currents, which do not include the Stokes drift.Next step, we plan to work out an ocean surface current retrieval method based on the satellite remote sensing data, high precision bathymetric topographic data and in-situ observations, which may provide more reliable ocean surface current products in the future study.

5 DATA AVAILABILITY STATEMENT

The MADT data is provided by CMEMS (http://marine.copernicus.eu/services-portfolio/access-toproducts/?option=com_csw&view=details&product_id=SEALEVEL_GLO_PHY_L4_REP_OBSERVATIONS_008_047) and is downloaded from ftp://my.cmemsdu.eu/Core/SEALEVEL_GLO_PHY_L4_REP_OBSERVATIONS_008_047/dataset-duacs-rep-globalmerged-allsat-phy-l4. The CCMP Version-2.0 vector wind is produced by the Remote Sensing Systems.Data are available at: http://data.remss.com/ccmp/v02.0/. The ERA-Interim surface wave data is downloaded from http://apps.ecmwf.int/datasets/data/interim-full-daily. The in-situ Lagrangian drifter currents are downloaded from https://www.aoml.noaa.gov/phod/gdp/. The in-situ KEO observations are available at https://www.pmel.noaa.gov/ocs/data/disdel/.