Prescribed fast tracking control for flexible air-breathing hypersonic vehicles:An event-triggered case
Xingling SHAO ,Yi SHI ,Wendong ZHANG ,Jiang ZHAO
a The Key Laboratory of Instrumentation Science &Dynamic Measurement,Ministry of Education,North University of China,Taiyuan 030051,China
b School of Instrument and Electronics,North University of China,Taiyuan 030051,China
c School of Automation Science and Electrical Engineering,Beihang University,Beijing 100191,China
KEYWORDS Flexible air-breathing hypersonic vehicle;Prescribed performance control;Switching event-triggered;Tracking differentiator;Uncertainty and disturbance estimator
Abstract In this paper,a prescribed fast tracking control scheme is proposed for Flexible Airbreathing Hypersonic Vehicles (FAHV) subject to lumped disturbances and limited resources.To maintain tracking errors of velocity and altitude converge to a predefined region with a prescribed time and release the transient intense fluctuations encountered in classical Prescribed Performance Control (PPC) using a fast decaying rate,a tracking differentiator-based PPC is presented,where the reaching time and the maximum time differentiation of preselected envelopes can be regulated as a prior via fixing an acceleration factor,so that a guaranteed fast convergence speed can be realized with reduced oscillations.Besides,to avoid the excessive occupation of limited resources (energy and communication)and guarantee a remarkable tracking accuracy,switching event-triggered mechanisms are constructed for FAHV control realization,which provide a promising way to pursue a desired level of tracking performance with a low energy consumption.Subsequently,Uncertainty and Disturbance Estimators (UDE) and Sigmoid function-based Tracking Differentiators(STD)are employed to provide disturbance estimation and reference derivation with a low computational complexity.Finally,robust control laws are designed to compensate for the sampling error induced by event-triggered conditions,meanwhile Zeno phenomena can be effectively eliminated.The simulation results and comparisons validate the effectiveness of the proposed scheme.
1.Introduction
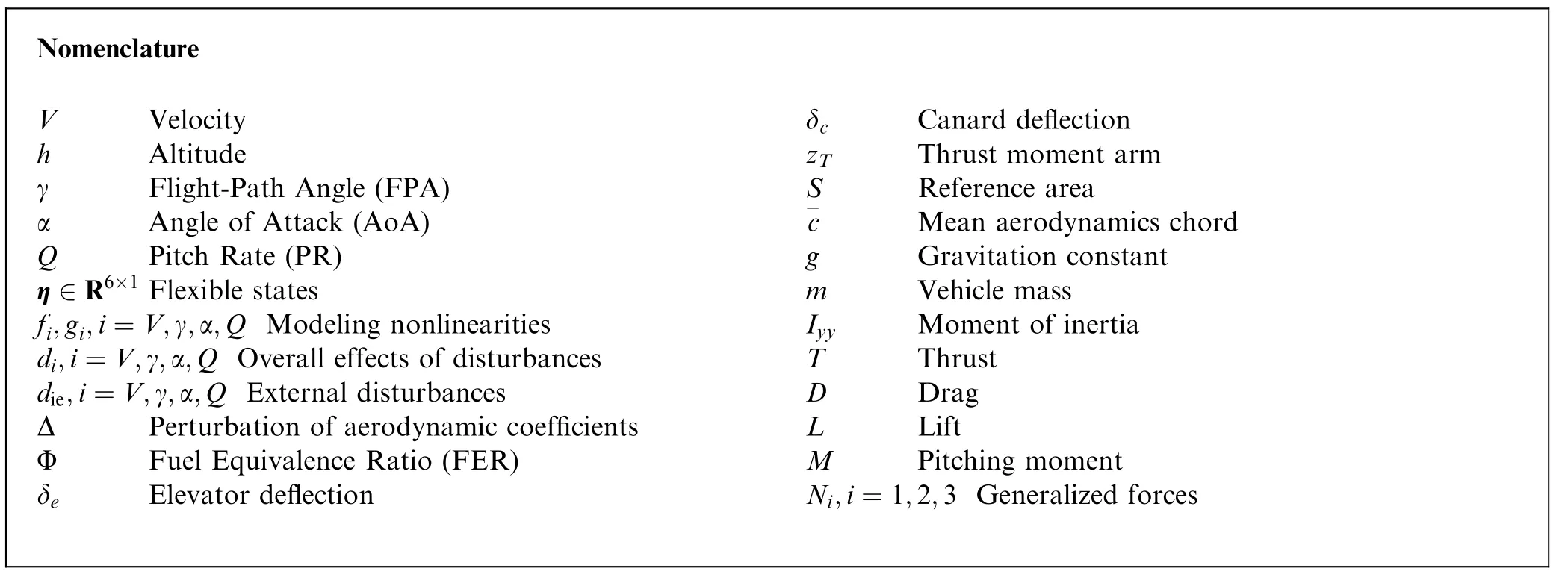
Recent years have witnessed rising interests in Flexible Airbreathing Hypersonic Vehicles (FAHV) in near-space transportation,owing to its cost effectiveness and high speed.1–3However,it is still a challenging task to realize a desirable fight control for FAHV,owing to its undesirable flexible effect to the rigid-body dynamics,intricate coupling between the propulsion system and flight dynamics,as well as parametric uncertainties caused by the lack of precise flight aerodynamics database.What’s more,the unknown atmosphere disturbance is another significant obstacle to achieve FAHV closed-loop stability.
In accordance with aforesaid analysis,it is imperative to achieve an anti-disturbance control performance for FAHV within a wide range of working points.Up to now,quantities of results have been reported to address FAHV robust tracking problem,which can be mainly segmented into feedback linearization control,Sliding Mode Control (SMC),4adaptive control,5–6neural control7–8and so on.In Ref.[4],an exponential SMC technique is devoted to FAHV considering mismatched disturbances,while a robust term is added at every design step to make the closed-loop control system reach a steady state along the constructed sliding mode surface.By incorporating an adaptive technique,in Ref.[5],a high-order SMC strategy is established for FAHV,so that the chattering phenomena in traditional SMC studies can be alleviated.In Ref.[6],an adaptive control is established for variable geometry inlet-configurated air-breathing hypersonic vehicles considering time-varying parameter uncertainties,where these parameter uncertainties can be handled with reduced number of parameter updating laws.A disturbance observer based neural adaptive control is devoted to FAHV in Ref.[7]considering wind effects.In Ref.[8],a prescribed anti-disturbance control performance is achieved for FAHV in the premise of a scarce communication bandwidth.
Although enhanced tracking performances of FAHV with consideration of uncertainties can be achieved within the aforementioned control designs,how to determine the frequency of control signals in the condition of digital implementation is ineluctably encountered in these investigations constructed within the time-triggered paradigm.Commonly,the sampling frequency is selected to be enough small for FAHV controller to pursue robust control performance under the consideration of a worst-case scenario.However,this configuration will lead to excessive occupation on communication bandwidth and energy dissipation,while resource reservation is imperative for FAHV subject to scarce bandwidth along communication channel and limited onboard fuel,particularly for a long endurance cruise mission.Note that if a larger sampling interval is utilized,the controller may not be able to realize a rapid maneuverability.For the sake of eliminating the drawbacks of current periodic sampling control paradigms,many efforts have been made to investigate the eventtriggered control design of nonlinear systems9–13for energy saving.In Ref.[9],a relative threshold triggering mechanism is devoted to ensuring the global convergence of nonlinear networked systems.By introducing a relative threshold triggering mechanism,in Ref.[10],an event-triggered adaptive compensation scheme is devised for uncertain nonlinear systems concerned with actuator dead-zone,while Zeno phenomena are proved to be eliminated.To compromise between tracking performance and computational burden,a switching threshold triggering mechanism is proposed for a class of uncertain nonlinear systems in Ref.[11],which preserves the merits of both fixed threshold triggering and relative threshold triggering methods.In Ref.[12],a switching threshold strategy is employed to construct an event-triggered adaptive backstepping controller for plug-and-play spacecraft with less communication burden and satisfactory attitude tracking.However,the existing tracking results with regard to event-triggered techniques cannot be specified within a preassigned transient and steady-state boundary.In addition,a recursive design structure without considering the explosion of differentiation is utilized for the reported controller realization,which will generate a deficiency in real-time capability.To our mind,it is still an open problem for FAHV to pursue a controller simultaneously considering constrained resources,guaranteed tracking performances,as well as low computational complexity.
Another motivation of this paper is to obtain a fast tracking capability for FAHV with a predefined convergence time,while a rapid convergence speed is beneficial for performing a strong maneuver climb and strengthening the mission efficiency.14To accelerate convergence process compared to traditional asymptotic convergence researches,a finite time control scheme incorporated with a non-homogeneous disturbance observer has been studied for FAHV in Ref.15,while the convergence time is involved with initial errors and design parameters.As an extension of finite time stability,a fixed time convergent backstepping control strategy is investigated in Ref.[16] via augmented sliding mode observers,so that the convergence time of FAHV tracking errors can be assigned via control augments independent of initial errors.However,although a fast convergence result can be obtained via regulating proper control parameters for fixed-time solutions,the overestimation of upper bound of convergence time always gives rise to the conservative design of controllers,and thus in this sense,the transient behavior cannot be easily prescribed to fulfill user defined configurations.To achieve a guaranteed tracking performance without tuning parameters tediously,Prescribed Performance Control (PPC),where a logarithmic function incorporated with preselected exponential boundaries is utilized to achieve error-bounded tracking within preselected residual sets,has been applied extensively.17–22In Ref.[17],by incorporating PPC into an adaptive critic design paradigm,an adaptive critic design-based controller guaranteeing predetermined tracking performances is contrived for hypersonic vehicles.In Ref.18,a neural learning control is investigated for nlink manipulators considering uncertain system dynamics and external disturbances,while exponential boundary functions are introduced to serve as a prior condition imposed on closed-loop system.By merits of a squeeze theorem,a novel PPC is exploited for FAHV to realize trajectory tracking with an arbitrarily small overshoot in Ref.[19].Unfortunately,there still exist two defects that hinder the practicability of the aforesaid PPC techniques.Firstly,the tracking error convergence is governed with an exponential convergence rate via an exponentially decaying function,that is,the system responses will reach to preset final values only when time tends to be infinite,while a fast reaching time is of utmost significance for FAHV to perform penetration missions.Besides,for the existing PPC,a prescribed fast tracking performance can be readily obtained by increasing the convergence ratelof an exponentially behavioral function ρ(t)=(ρ0-ρ∞)∙exp(-l∙t)+ρ∞.Nevertheless,due to the fact that time differentiation,i.e.,˙ρ(t)=-l∙(ρ0-ρ∞)∙exp(-l∙t) exhibits rapid fluctuations during initial process,the corresponding proportional feedback term ˙ρ(t)∙e(t)/ρ(t)contained in the controller will lead to actuator possessing high-frequency oscillations at transient phase,especially under a large convergence ratel,which unavoidably deteriorates the FAHV control safety.Consequently,new solutions are urgently required to overcome the weaknesses of traditional PPC in guaranteeing a fast tracking capability for FAHV.
To address the aforementioned problems,we propose a prescribed fast tracking control for FAHV within an eventtriggered paradigm,which has the following striking contributions:
(1) Contrasting to the current continuous-time controller designs for FAHV,1–3the problem on reducing the communication data between control module and actuator subject to some satisfactory tracking performances of FAHV is addressed with the aid of a switching eventtriggered mechanism.Compared with fixed or relative event-triggered techniques,the designed event-triggered condition can preserve the merits of both and better balance the conflict between communication constraint and tracking accuracy.Furthermore,to compensate for the sampling error,a novel robust control law is devised to theoretically guarantee the closed-loop system stability and eliminate the Zeno phenomena.
(2) Different from the existing PPC-based results,18–21where only asymptotic convergence to the origin can be assured and the harmful oscillations in control signals during transient stage are unavoidable when using a fast convergence rate,we propose a Tracking Differentiatorbased PPC (TDPPC) to overcome the above issues,so that the tracking errors of altitude and velocity can converge to a predefined region with a prescribed time without repeatedly tuning control parameters,and a prescribed fast tracking performance with decreased chattering control signals can be obtained.
(3) To circumvent the notorious difficulty of timeconsuming learning process in NN-based methods,6,8,23meanwhile guaranteeing an effective disturbance compensation,we make efforts from the following perspectives:Firstly,compared to the existing Extended State Observer (ESO) design24–26requiring the differential of lumped disturbances to be bounded,by using a relaxed hypothesis that the bandwidth of designed filter can sufficiently cover the spectrum of disturbances,Uncertainty and Disturbance Estimator(UDE)27–28is constructed to online estimate the lumped disturbances,where only a time constant in linear filter is needed to be tuned,which is of great practicability for FAHV owing to its lowcomplexity computation and convenient parameter tuning.Secondly,a Sigmoid function-based Tracking Differentiator (STD) is constructed at every step to simultaneously surmount the so-called ‘‘explosion of complexity”and release the unfeasible initial condition occurring widely in Dynamic Surface Control (DSC)framework.29
The remainder of this paper is organized as follows.In Section 2,some preliminaries for FAHV modeling and PPC are stated.Design process is developed in Section 3.In Section 4,stability analysis is given.Simulation studies are performed in Section 5 to validate the effectiveness of the designed controller.Conclusionsare drawn in Section 6.
2.Preliminaries and problem formulation
2.1.Problem formulation
The diagrammatic sketch for a FAHV is presented in Fig.1.By referring to Refs.[30–31],the mathematical longitudinal model of FAHV can be given with the following strictfeedback form:

Fig.1 Schematic of a FAHV.

wherep=0.5ρaV2denotes the dynamic pressure with ρa=ρa0exp(-(h-h0)/hs).
2.2.Traditional PPC
Traditional PPC19–21is utilized to transform the constrained error into an unconstrained one with predetermined transient behavior,the profile of which is shown in Fig.2.
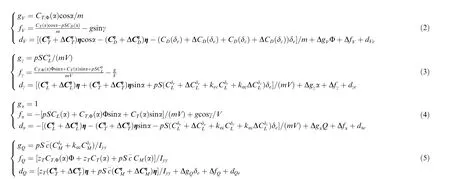
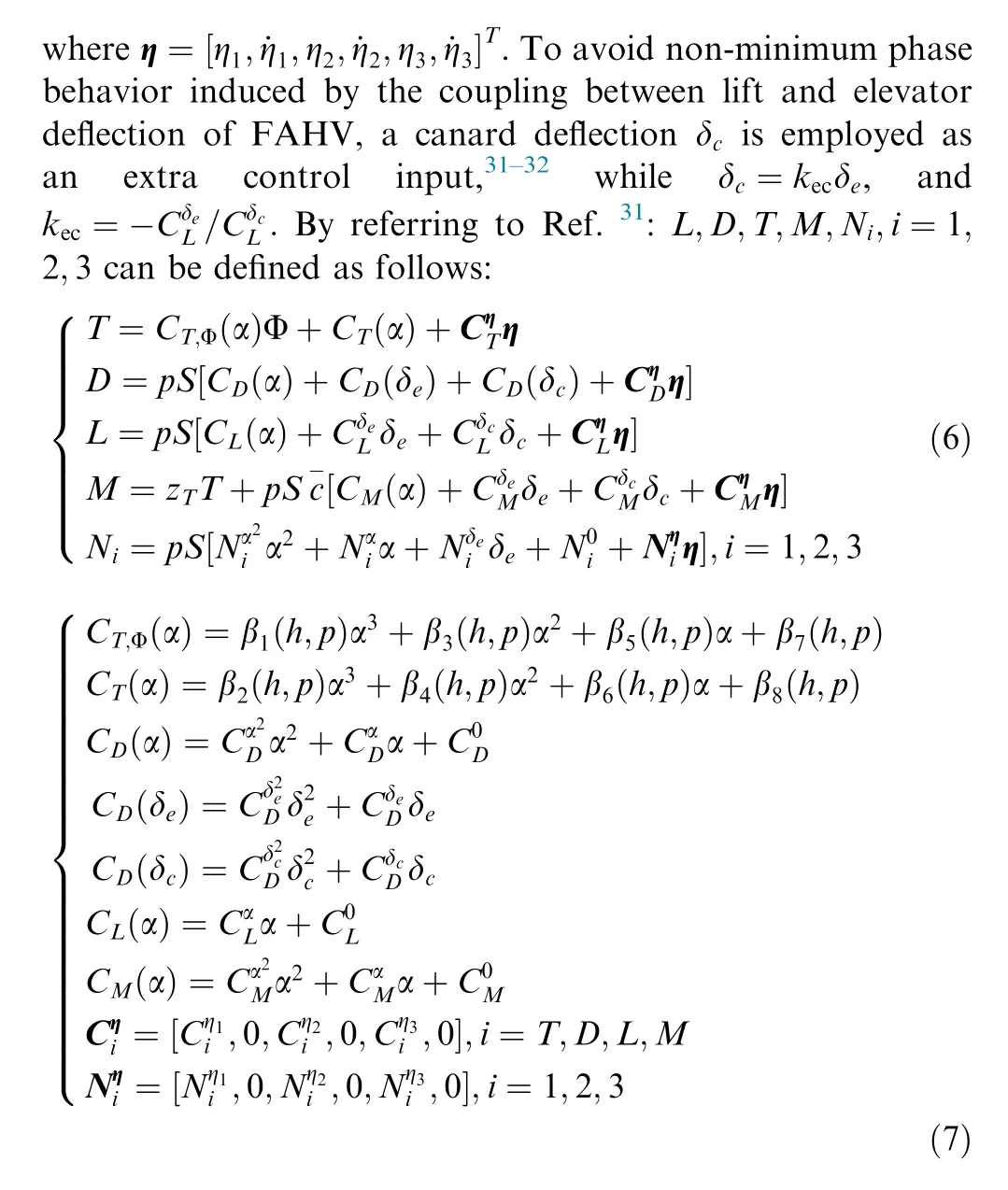

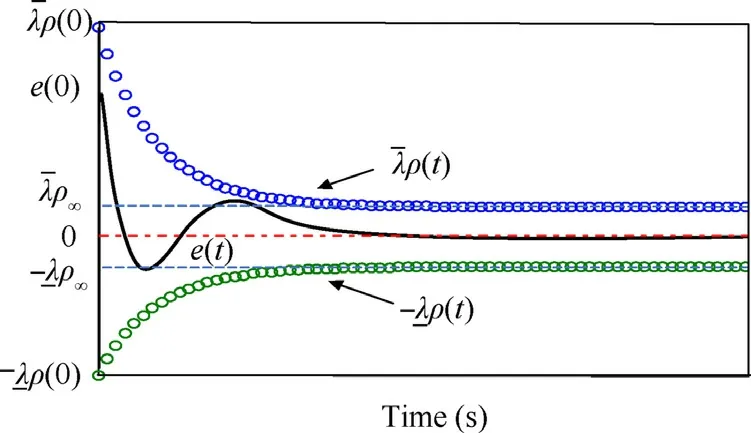
Fig.2 Graphical illustration of PPC.
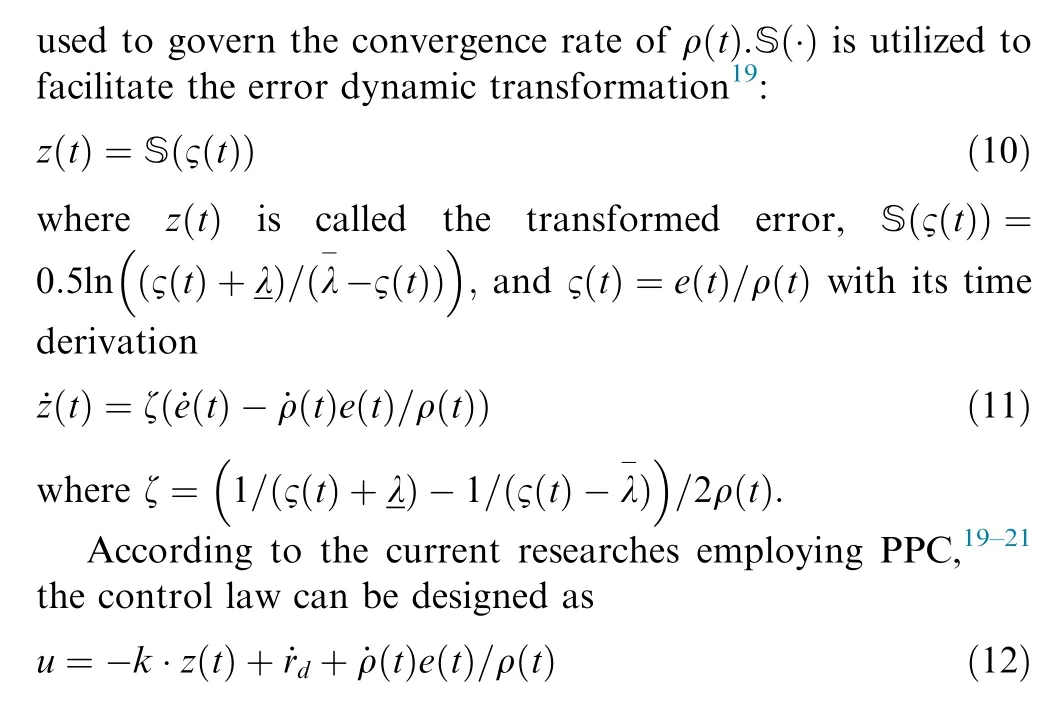
wherekdenotes the control gain,and ˙rddetermines the time differentiation of reference signalrd.
Remark 1.To achieve predetermined fast tracking performance,it is suggested to select a sufficiently largelin the defined prescribed performance function,while the drastic fluctuations occurring in control signal are inevitable.Note that such weakness may cause potential structural damages to FAHV actuators and deteriorate the control safety33.Additionally,the utilized behavioral function in traditional PPC involves an exponential convergence rate,which cannot ensure that the system response evolves within a prespecified region after a preassigned settling time to meet the demands of fast maneuvering.Consequently,it is imperative to construct an effective method for FAHV to achieve fast tracking performance equipped with smooth control signals and predetermined fast settling time.
3.Prescribed fast tracking controller design
In this section,a prescribed fast tracking control for FAHV is proposed as shown in Fig.3,where a TDPPC is constructed to achieve a predefined appointed time tracking and meanwhile guarantee a smooth control signal profile at transient stage.Besides,a switching threshold event-triggered mechanism is utilized in controller construction under the premise of a scarce communication bandwidth and a desired level of tracking accuracy.In addition,a UDE is devised at every step for disturbance reconstruction,where an STD is utilized to produce a smooth signal differentiation.

Fig.3 Structure diagram of presented controller.
3.1.TDPPC
Define the tracking error of FAHV velocity subsystem as follows:

whereVd(t) denotes the reference signal for velocity subsystem.To achieve a predetermined fast tracking performance for velocity subsystem,the following constraint should be satisfied:

Definition 1.T(t):R≥0→R+can be determined as a prespecified behavioral function equipped with an appoin ted reaching time only if the following two conditions are fulfilled:1)T(t)is a positive and monotonously decaying function over t∊[0,ta).2)T(t)≡T∞for ∀t≥ta,whiletacontributes to a preselected reaching time,andT∞is a non-negative constant.
Han’s tracking differentiator (Han’s TD),established in Ref.34,is widely recognized as an effective tool to supply smooth and bounded reference and its derivative to be tracked,in the premise of given initial and finial points,as well as the maximum capability of control efforts.Particularly,the state response of Han’s TD can approach to the desired steady values in a minimal and finite time,which is perfectly consistent with Definition 1,and thus here we extend the idea of Han’s TD to serve as an appointed time performance functionTV:

Remark 2.Contrasting to the current PPC-based investigations,19–21the TDPPC established in this paper has the following striking superiorities:(1) It can be intuitively observed from Fig.4 that the time derivative of boundary under TDPPC can be divided into an acceleration process and a deceleration process with the same time,whiletakes maximum valueat the instantaccording to Ref.34.Obviously in Fig.4,the maximum value ofunder TDPPC is much smaller than that of PPC envelope with nearly the same reaching time,which alleviates the fast change of compensation term in the control law,and thus,a control signal with a lower chattering degree can be realized.(2)For TDPPC,the preassigned time ofTV(t) can be determined byderived in Ref.34,and once the initial and final values of Han’s TD are determined,one can directly regulate the acceleration factor to allow for different appointed time tracking property,as shown in Fig.4.However,for PPC,the utilized exponential behavior function does not satisfy Definition 1,where the settling time cannot be prespecified accurately,which unavoidably leads to the design conservatism.(3) With the aid of TDPPC,both of the preselected bound and time derivation can be simultaneously obtained,which avoids the extra analytical computation for time differentiation in the PPC techniques.Note that although the construction of TDPPC is more complicated compared to traditional PPC,only a series of algebraic operations instead of differential calculations are needed during each sampling period,preserving the computational burden at a competitive level.Additionally,similar to the current PPC technique,only three design parametersTVi,TVs,rV,are required to be governed in TDPPC,so that the practicability of TDPPC can be guaranteed.Besides,Han’s TD (15) is contrived for FAHV controller in the feedforward channel,which will not impact later stability analysis of the whole closed-loop control system.
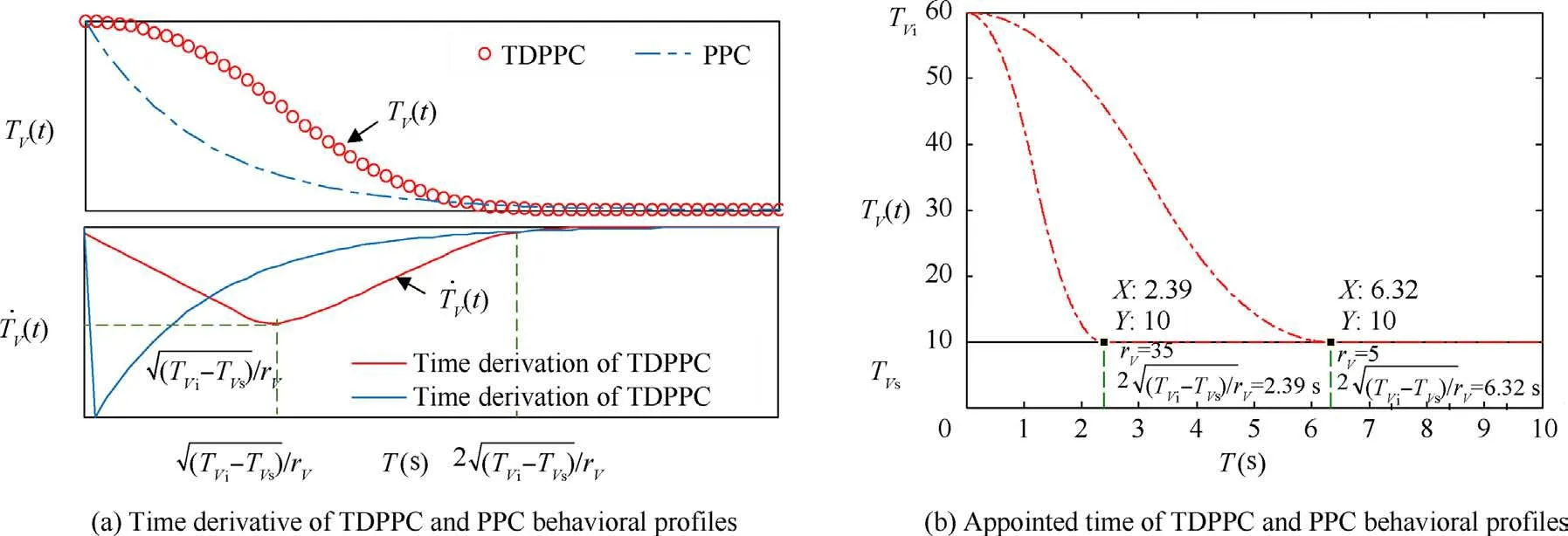
Fig.4 Graphical illustration comparison between TDPPC and PPC.
Remark 3.Although Finite time Tracking Differentiator(FTD),35–36STD37and robust exact differentiator38–39are also capable of generating a smooth behavioral envelope with finite time,the maximum acceleration constraints of system response are not taken into consideration in these differentiators,i.e.,the time derivative of the boundary cannot be governed in a time-optimal formulation,unavoidably causing fluctuations to control signals during transient phase,which is the reason why we choose Han’s TD to replace the exponential type function to seek for an optimized transient profile.
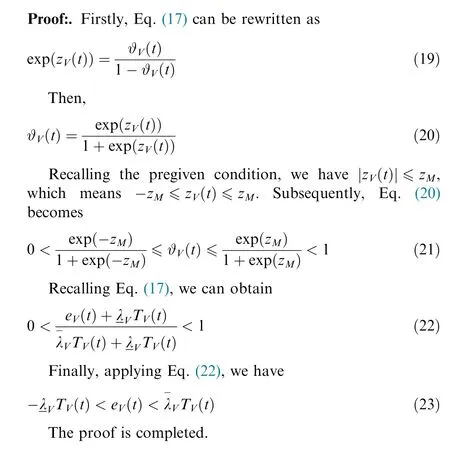
Utilizing the tracking erroreV(t),we devise the following transformed tracking error:
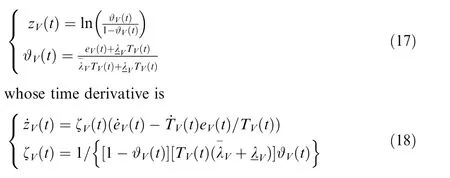

3.2.Velocity controller design

To online estimate lumped disturbancesdVwith measurable system information,there are many powerful techniques,such as ESO40and UDE.28However,for ESO,the time derivative of lumped disturbances is inherently required.This assumption is rigorous for FAHV in some flight surroundings suffering from abrupt external disturbances,where the time derivative of lumped disturbances tends to be infinite.Without this restriction,UDE provides a promising way to obtain a disturbance estimation,based on an assumption that the lumped disturbances can be estimated by a low pass filter equipped with a sufficiently large bandwidth.That is,via UDE technique,if the filter bandwidth is wide enough,the lumped disturbances can be extracted from measurable system responses.
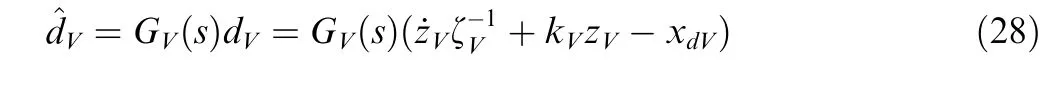
Then,with the same line of UDE philosophy,28the estimate ofdVcan be expressed as whereis the estimation ofdV.GV(s) determines a low-pass filter withsbeing a Laplace operator.Note that althoughGV(s)can be configured differently to meet specified demands,a first-order filter with a bandwidth covering the spectrum of lumped disturbances is enough in most of cases,which can be designed as

From Eq.(31),it is intuitive that unknownxdVcan be extracted from measurable system dynamicszVandFinally,the assistant control signal υVcan be given as

Considering the fact that FAHV generally suffers from the limitations on energy and communication resource,23,14a switching event-triggered technique11is employed in our controller design to realize a reference tracking with a reduced sampling cost.Subsequently,a robust control law vΦis established for FAHV velocity subsystem

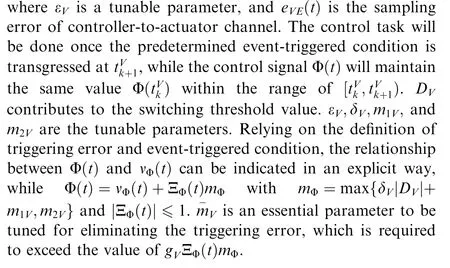
Remark 4.A switching event-triggered mechanism is designed for FAHV control design,contributing to a fact that the unnecessary resource dissipation occurring in the continuoustime framework can be effectively reduced,and meanwhile a competitive tracking accuracy can also be achieved.
Remark 5.A relative threshold triggering is effective in reducing resources consumption especially for stabilization issue owing to its changeable threshold.9,10However,when the magnitude of control signal becomes excessively large,the sampling error will also increase dramatically,leading to an unexpected chattering in control signal.For fixed threshold triggering,although the sampling error can be restrained to a predefined value independent of control input,unnecessary energy consumption may occur when system states vary slowly.Note that the switching threshold triggering preserves the advantages of both and offers a flexible solution to balance the triggering times and tracking performances.

3.3.Altitude controller design

To transform the constrained altitude dynamics into an unconstrained one,similar to the velocity subsystem,we devise the following error transformation:
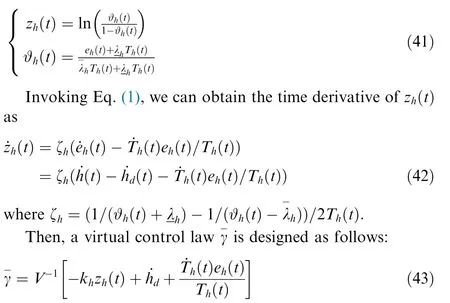
wherekhcontributes to a controller gain.The following STD is constructed to obtain the time derivation of γd:
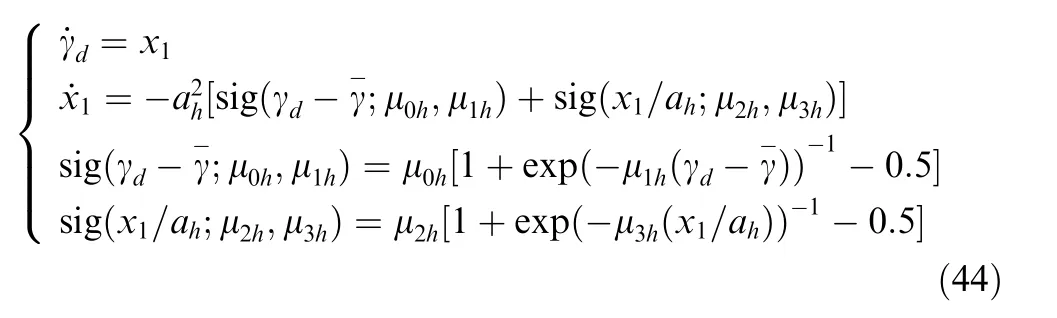
whereah>0,μih>0,i=0,1,2,3 are the tunable parameters to ensure the convergence of STD.
Lemma 2.37Consider the following system:
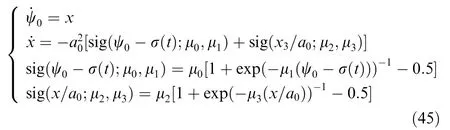
where ψ0is the filtered command of STD.xcontributes to the differentiation signal of ψ0generated by STD.a0>0,μi>0,i=0,1,2,3 are the tunable parameters.Only if the input signal is continuous and bounded,the following formulation will hold after a finite time ■.

From Eq.(46),it can be concluded that system state ψ0tracks the provided signal σ(t) after a finite time,whilexis capable of estimating the time derivative of ψ0.
Remark 6.The reasons why we employ STD to produce the time differentiation of virtual control law for FAHV are twofold:Firstly,for DSC,29,41a strict initial condition that the initial value of virtual control law should be equivalent to the initial value of filter is commonly demanded,while initial virtual control value is hard to be measured exactly from an engineering aspect.Differently,STD is capable of providing a time derivative of input signal without the requirement of any initial condition.(2) Contrasting to Hybrid Tracking Differentiator (HTD),42STD preserves a competitive signal differentiation capability,and meanwhile the undesirable chattering phenomena using HTD can be effectively circumvented.
Step 2.For FPA error stabilization,we firstly define the tracking error aseγ=γ-γd,and the derivative ofeγcan be further given based on FPA dynamics (1)
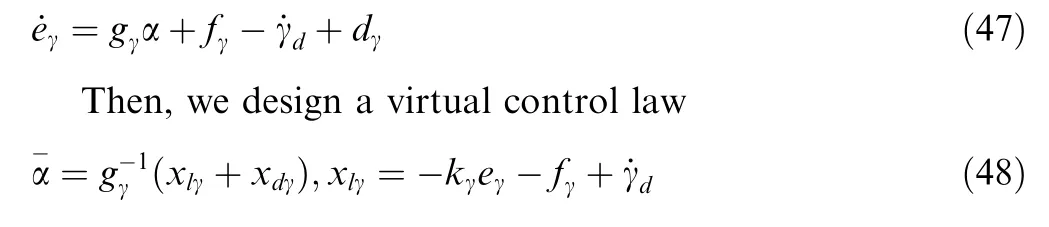
wherexlγ,xdγdenote the feedback linearization term and disturbance compensation term,respectively,whilexdγis unknown and cannot be used in controller realization.To facilitate the design process,similar to the velocity subsystem,we introduce UDE technique,such that

wherekγcontributes to control gain,and χγis a positive design parameter.The following STD is devised to obtain the time derivative of virtual control law

whereaα>0,μiα>0,i=0,1,2,3 are design parameters.
Step 3.Similarly,the tracking error for AoA subsystem is defined aseα=α-αd,while time derivative can be further computed via invoking AoA dynamics

Similar to the derivation elaborated in Step 2,to stabilize AoA tracking,a virtual control lawQ-based on UDE technique is established

wherekαdenotes the control gain for AoA,and χαis a positive design parameter.
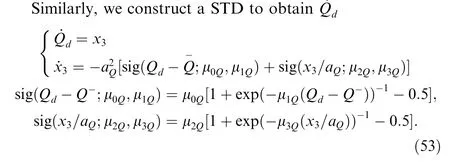
whereaQ>0,μiQ>0,i=0,1,2,3 are the tunable parameters utilized to guarantee the convergence of STD.
Step 4.For pitch rate subsystem,the tracking error is defined aseQ=Q-Qd,while its time derivative containing the event-triggered control law can be given by invoking system dynamics (1):


4.Stability analysis
Theorem 2.Consider the FAHV model(1),the virtual control laws(43),(48),(52),the control signals(33),(55),switching threshold triggering conditions(34),(56),STDs(44),(50),(53),and UDEs(31),(49),(52),(55).For any bounded initialconditions satisfyingi=V,h,thereexist constantsκi∊R/i=1,2,...,5defined by Eq.(60),so that all closed-loop signals are governed to be bounded and the tracking errors eV,eh are restrained within the prescribed residual sets.Besides,the notorious Zeno phenomena are excluded.


According to Lemma1,it can be obtained thatsi→0(i=1,2,3) with appropriately selected parameters.Besides,by referring to the pioneering investigations on UDE-based techniques,27–28the estimation errors of UDEi=V,γ,α,Qare restrained by non-negative upper boundsThen,we can derive


Remark 7.Tuning guidelines of main design parameters for the presented controller are summarized as follows:Firstly,for TDPPC,sufficiently largeTji,j=V,hare demanded to guarantee the satisfaction of initial conditionsNote that though a largerrjwill allow for a shorter convergence time for tracking profiles,a larger control authority will also be produced,which may exceed the physical accessible ranges of actuators.Other parameters in TDPPC are related to behavioral boundaries,which should be selected appropriately in accordance with mission requirements.For UDE,time constants χj,j=V,γ,α,Qshould be tuned as small as possible to capture a remarkable estimation performance.However,a high bandwidth of UDE will also result in system instability especially when the lumped disturbances vary with large magnitudes.Consequently,the design of time constants needs a tradeoff between disturbance rejection performance and stability performance.For switch triggering condition,smaller δj,m1j,m2j,Dj,j=V,Qwill induce a better tracking precision with an increase in triggering times,which indicates that there exists a tradeoff between tracking error and sampling cost.Larger control gainskV,kh,kα,kγ,kQwill give rise to larger control efforts and further reduce the tracking error in corresponding subsystem,while overlarge control gains will produce intense fluctuations during initial phase and deteriorate the system stability.
5.Simulation results
Simulation results are presented in this section to illustrate the validity of the proposed controller.All physical parameters and initial state values in simulation are borrowed from a representative research.14Moreover,we consider that the parameter uncertainties during cruise mission are+40%with regard to nominal values in Ref.[14].In addition,external disturbances are represented in the form of Eq.(67) and Eq.(68):

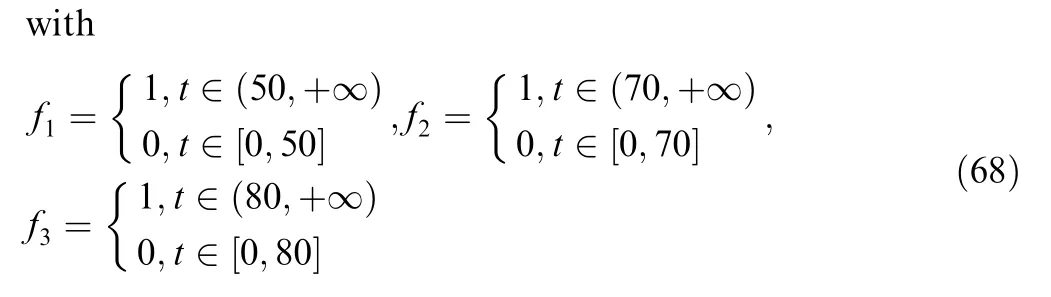
Based on the guideline elaborated in Remark 7,the parameters of the proposed control algorithm are listed in Table 1,while the parameter settings of contrastive techniques are provided in Tables 2-3.
Case 1.Comparison between TDPPC and existing PPC.17
For the purpose of validating the superiority of the presented TDPPC outperforming PPC in achieving a smooth control signal at transient,we make the following comparative simulations with different prescribed times being 29.73 s,3.22 s.The convergence time of tracking profile is defined as the moment whenzi,i=V,hfirstly fall into the convergence setsrespectively,while prescribed time is determinedaccording to the pioneering research.34,45–46Besides,according to Ref.47:we introduce the following indexLΔuto measure control signal chattering degree:

where ϖ is the number of sampling times during the whole simulation andu(∙) represents the control signal.The results are depicted in Figs.5–7 with relevant data analysis collected in Table 4.It can be intuitively observed from Fig.5 that nearly the same tracking performance for both FAHV velocity and altitude subsystems can be achieved under TDPPC and PPC.17However,when a shorter prescribe time is assigned for altitude subsystem response,as seen in Fig.6,a prescribed fast tracking is achieved at the expense of introducing intense fluctuations in the control signal under PPC,which is harmful to FAHV control safety.Differently,for TDPPC,since the time differentiations of preselected envelopes are governed reasonably in a time-optimal formulation,fast fluctuations in compensation terms can be effectively reduced,so that a smoother control profile at the initial stage can be achieved even with a shorter prescribed time.From Table 4,it can be seen that the chattering degree of control signal under TDPPC is decreased by 19.5% compared tothe one using PPC when the prescribed time is selected as 29.73 s.Note that when shortening the preassigned time to 3.22 s,the superiority of TDPPC in alleviating control signal chattering degree becomes more obvious with a reduction of 41.1% compared to PPC,which reflects the capability of TDPPC in realizing the prescribed fast tracking performance for FAHV.Fig.7 shows the tracking results for FPA,AoA,and pitch rate (row 1),the UDE estimation results (row 2),and the profiles of FAHV flexible states (row 3) under presented controller,and we can state that FPA,AoA,and PR of FAHV can accurately follow the reference signal by the presented method,while the lumped disturbances can be effectively compensated for by UDEs with a low computational burden.

Fig.5 Comparison of tracking performance between TDPPC and PPC (1 ft=30.48 cm).17
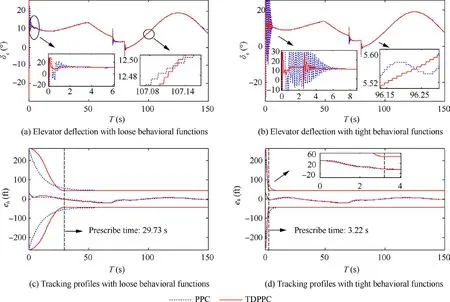
Fig.6 Comparison of control signal δe and tracking performance eh between TDPPC and PPC.17

Fig.7 Tracking performance of γ,α,Q,estimation results,and flexible states of FAHV under TDPPC.
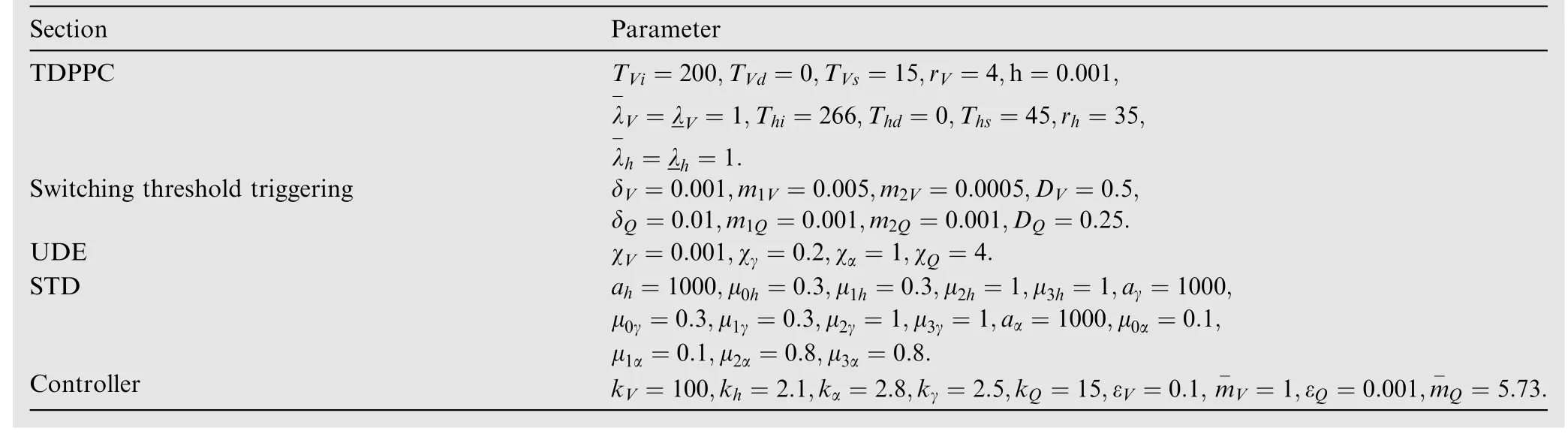
Table 1 Parameters for proposed algorithm.

Table 2 Parameters of contrastive algorithms in Case 2.

Table 3 Parameters of contrastive algorithms in Case 3.
Case 2.Performance comparison among proposed controller,adaptive controller6and backstepping controller30
In this case,comparison simulations for FAHV altitude subsystem are performed to validate the competences of the proposed controller surpassing adaptive control6and backstepping control30in terms of convergence time,tracking accuracy and triggering times.Note that the design parameters in adaptive control and backstepping control are selected via trial and error for pursuing a similar tracking accuracy in steadystate phase compared to the proposed controller,while detailed parameters are collected in Table 2.Fig.8 shows the involved results.Subsequently,we consider standard deviation as a criterion for altitude tracking error.48Based on the aforementioned index,the results of data analysis are provided in Table 5.Obviously in Fig.8,although adaptive control and backstepping control are also capable of realizing UUB for altitude tracking,the prespecified behavioral functions are violated,while for the proposed controller,the tracking profile satisfying predetermined performance envelopes can be achieved.Moreover,for the proposed controller,the triggering times can also be dramatically reduced by merits of eventtriggered technique contrasting to adaptive controller and backstepping controller,while the convergence time and tracking accuracy are maintained at a similar level.

Fig.8 Performance comparison among proposed controller,adaptive controller6 and backstepping controller.30

Table 4 Comparison of altitude tracking performance between PPC17 and TDPPC.
Case 3.Comparison among different triggering conditions
Simulations on FAHV velocity subsystem are presented to test the superior control performance of switching threshold based event-triggered control compared to fixed threshold43triggering,relative threshold triggering,44as well as continuous-time updating schemes.The design parameters employed in subsequent simulations are collected in Table 3.Simulation results under aforesaid four algorithms are presented in Fig.9 and Table 6.It is obviously seen that eventtriggered mechanisms can reduce the number of triggering times by 98% at least compared to the continuous-time control,and meanwhile the tracking accuracy maintains at a similar level.This reflects the competence of event-triggered control technique in pursuing favorable tracking profiles with a low occupation on communication resources.The performance comparison among three event-triggered methods in terms of control signals,triggering times,and error profiles is shown in Fig.9 and Table 6.Intuitively,as shown in Table 6,the triggering times under relative triggering technique are the least compared to fixed and switching threshold triggering in both FER and elevator deflection,owing to the fact that a time-varying triggering threshold related to the magnitude of control signal is incorporated.However,this superiority is achieved at the expense of deteriorating tracking accuracy.For fixed threshold triggering,the tracking accuracy can be improved at the cost of increasing triggering times along communication channels.Differently,the switching threshold triggering preserves the merits of both relative and fixed threshold triggering methodologies,which achieves a tradeoff between tracking accuracy and resource saving for FAHV cruise control.

Table 6 Comparison among different triggering conditions.

Fig.9 Performance comparison for velocity subsystem among relative threshold triggering,44 switching threshold triggering,and fixed threshold triggering techniques.43
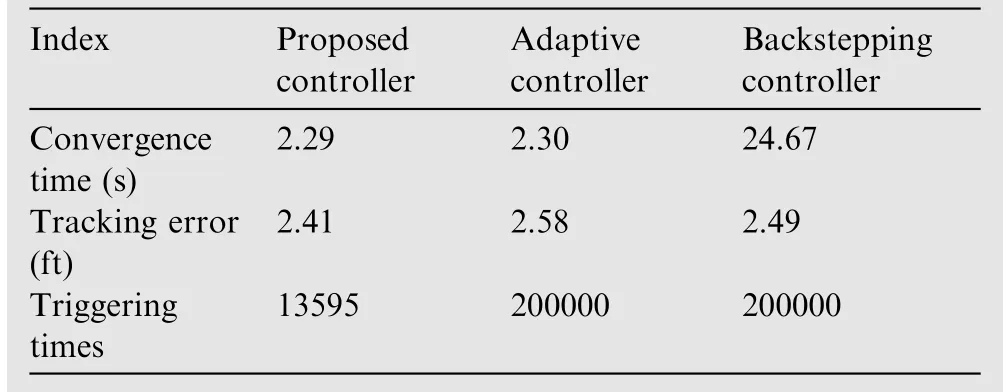
Table 5 Comparison of altitude tracking performance among proposed controller,adaptive controller6 and backstepping controller.30
6.Discussion
Different from the current NN-based FAHV control designs,where a complex online differential calculation is demanded for updating every neural cell output,which will inevitably occupy considerable computational resources and deteriorate real-time capability,herein,a UDE is designed for every FAHV subsystem in pursuit of disturbance reconstruction based on an assumption that unknown disturbances can beextracted from measurable system dynamics via properly selecting a filer,while the requirement of complex computation burden is no longer demanded.
Besides,by inserting switching threshold based samplers into controller-to-actuator channels,continuous control signals can be converted into intermittent control values under the supervision of preselected events,so that the unreasonable sampling costs in the existing continuous-time control methodologies can be dramatically reduced to adapt to the FAHV communication channel equipped with a scarce bandwidth.
Moreover,a TDPPC is constructed to specify tracking profiles with predesignated configurations without incurring intense fluctuations in control signals,which is inherently unavoidable especially with a fast convergence rate in PPC.Note that although the complexity of TDPPC surpasses classical PPC,leading to a more computational resource occupancy,the control efforts can be effectively diminished.
Notwithstanding with aforesaid distinguished merits,there are still unsolved challenges in model formulation to capture the FAHV characteristics,such as the non-affine dynamics and coefficients variations in different working points.We will focus on these issues in pursuit of a better feasibility of our controller design.
7.Conclusions
This paper presents a prescribed fast tracking controller with a reduced communication occupation and energy consumption for FAHV subject to lumped disturbances.A TDPPC is proposed to make FAHV tracking profiles reach to a predefined region with a preassigned settling time,and smooth the control signal transients by governing the magnitudes of compensation terms in control laws.Then,switching event-triggered mechanisms are constructed to dramatically reduce the unnecessary energy and communication resources consumption with a comparable tracking accuracy.Moreover,UDEs are employed to compensate for lumped disturbances with a low-complexity computation and a convenient parameter tuning,while STDs are employed to avoid the explosion of complexity and preserve the precise signal differentiation.Future work will focus on a reliable flight control design for FAHV considering actuator faults,AOA limitation and non-affine dynamics.
Declaration of competing interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.
Acknowledgements
This research was supported by National Natural Science Foundation of China (No.61803348),National Nature Science Foundation of China as National Major Scientific Instruments Development Project (No.61927807),State Key Laboratory of Deep Buried Target Damage,China (No.DXMBJJ2019-02),Scientific and Technological Innovation Programs of Higher Education Institutions in Shanxi,China(No.2020L0266),Shanxi Province Science Foundation for Youths,China (No.201701D221123),Youth Academic North University of China(No.QX201803),Program for the Innovative Talents of Higher Education Institutions of Shanxi,and Shanxi ‘‘1331 Project’’ Key Subjects Construction,China(1331KSC).
 CHINESE JOURNAL OF AERONAUTICS2021年11期
CHINESE JOURNAL OF AERONAUTICS2021年11期
- CHINESE JOURNAL OF AERONAUTICS的其它文章
- Parameter effects on high-speed UAV ground directional stability using bifurcation analysis
- Supersonic flutter control and optimization of metamaterial plate
- Review of in-space assembly technologies
- Utilisation of turboelectric distribution propulsion in commercial aviation:A review on NASA’s TeDP concept
- The influence of inlet swirl intensity and hot-streak on aerodynamics and thermal characteristics of a high pressure turbine vane
- Full blended blade and endwall design of a compressor cascade
