Effects of short-range attraction on Jamming transition∗
Zhenhuan Xu(徐震寰) Rui Wang(王瑞) Jiamei Cui(崔佳梅)Yanjun Liu(刘彦君) and Wen Zheng(郑文)
1Institute of Public-safety and Big Data,College of Data Science,Taiyuan University of Technology,Taiyuan 030060,China
2Center for Big Data Research in Health,Changzhi Medical College,Changzhi 046000,China
Keywords: short-range attraction,Jamming transition,short-range attraction susceptibility
1. Introduction
The earliest study of jamming transition of attractive particles can be traced back to an early experimental study,[14]which proved that the glass transition temperature or the nonvanishing yield stress may extend to low packing fractions because of the presence of attraction. Loiset al.[18]showed that jamming transition in particulate systems with strongly attraction belongs to a new universality class, distinct from repulsive jamming and percolation transition without force balance constraints. Zhenget al.[19]showed that considering the presence of weak attraction in particle system,the static structure of shear-driven solids was sensitive to the change of packing fraction and shear stress. By simulating the soft particles with an attractive shell, Koezeet al.[20]presented an evidence for two distinct jamming scenarios. For weak attraction systems,a spanning cluster appears suddenly at the jamming transition pointφcwhile for strongly attraction systems,the rigid cluster undergoes a continuous growing. And they proposed that the weakly attractive scenario is a finite size effect, means that sufficiently large systems will fall in the strongly attractive universality class no matter how weak the attraction is. But it remains unclear when the attraction tends to disappearance,what are the characteristics of the susceptibility of short-range attraction.
As we all know that the magnetic susceptibility can be defined by assuming a “ghost spin” in the limit of vanishing density in spin systems.[21]In the case of percolation or correlated percolation,susceptibility corresponds to the addition of low-probability“short routes to the infinite cluster”.[22,23]The pinning susceptibility can be calculated by considering the responses of “ghost pins” in the limit of vanishing pinning.[24]Similarly,if the attraction acts as a small perturbation,another way to compute a susceptibility is to calculate the response to a perturbation. We expect the “short-range attraction susceptibility”to show critical behavior in the thermodynamic limit on jamming systems by calculated the responses of“ghost attractions” when the attraction of particles is vanishing. We define the short-range attraction susceptibility in the limit of vanishing attraction which describes the degree of response of the probability of finding jammed statespjto short-range attraction strengthµ.

In this work, we concentrate our attention on the effects of dilute attraction on the jamming transition. We find that no matter how the system increases, we can always find such an estimated crossover attraction strengthµ∗which can be defined to separate the short and long range attractions based on the behavior of the jamming transition point. In the shortrange attraction regime, we define the short-range attraction susceptibilityχpas the response of jamming probability to the increasing of attraction strength, which should be constant in the limit of small attraction.[21–23]Our central result is that the short-range attraction susceptibility diverges in the thermodynamic limit asχp∝|φ −φ∞c|−γp, withγp=2.0 in two-dimensional (2D) system andγp=1.57 in threedimensional(3D)system,whereφ∞cis the jamming threshold in the absence of attraction. Furthermore, such susceptibility obeys scaling collapse with a scaling function in both two and three dimensions,illuminating that the jamming transition can be considered as a phase transition as proposed in previous work.[24]
We simulateNattractive soft spheres in a fixed square(two dimensions,d=2) or cubic (three dimensions,d=3)box with periodic boundary conditions. The diameter ratio of the large to small spheres is 1.4 and their numbers in the box are equal to avoid crystallization. The inter particle potential is[7,19,25]
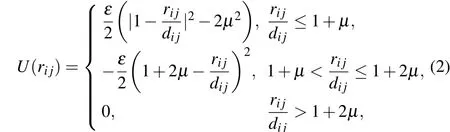
whererijis the separation between particlesiandj, anddijis the sum of their radii,andµis a tunable parameter used to control the range and strength of attraction. We generate static states at fixed packing fraction by applying the fast inertial relaxation engine (FIRE) method[26–28]to minimize the potential energyU=∑i jU(ri j)of random configurations,where the sum is over all pairs of particles. The contact force law for a pair of particles with an attractive shell used in our simulations is shown in the lower inset of Fig.1(b). We set the units to be the particle massm,the characteristic energy scale of the potentialε,and the small particle diameterds.
The system is characterized by its packing fractionφ,number of particlesN,and the attraction strengthµ.We calculate the probability of finding jammed statespj(φ,N,µ)when the temperature changes rapidly from infinite(T=∞)to zero(T=0). In our simulation, each particle’s initial location is generated randomly by the standard preparation protocol.[7]The probability of finding jammed statesp(φ) for different values of attraction strength are calculated by minimizing the potential energy of 10000 random states. When the energy of the system is minimized,it is considered that the jammed configuration is obtained when the absolute value of pressure is greater than 10−12. The pressure can be negative in a jammed solid with attractive interactions,but it has little effect on high density system.[29]Which means that the existence of negative pressure has little effect on our results.
2. Results
2.1. Critical attraction strength
As shown in Fig. 1(a), we vary the attraction strengthµfrom 5×10−5to 5×10−3to study the jamming probabilitypj(φ,N,µ)atT=0, and the zero attraction case is shown as well. The black square line shows that there is a rapid increase ofp(φ)at a packing fraction close toφ ≈0.84 where there is no attraction(µ=0). At fixedµ,we approximatepj(φ,N,µ)into a complementary error function[30]


Fig. 1. (a) Probability of finding jammed states pj(φ,N,µ) versus packing fraction φ for varying attraction strength µ from 5×10−5 to 5×10−3 in 2D system with size N=1024. The solid curves are the fits using Eq. (3). (b) The critical packing fraction φc as a function of attraction strengthµ for 2D systems with different system sizes N=256,512,1024,2048,4096,and 8192. Lower inset shows the contact force law for a pair of particles with an attractive shell used in our simulations.
whereφcis the critical packing fraction at whichpj(φ,N,µ)=0.5 andwis the width ofpj(φ,N,µ).It is clear that with the attraction strength increasing,the change ofp(φ)becomes more gradual andφc(N,µ)becomes smaller, and there is a distinct difference between the two fitting results ofµ= 2×10−3and 3×10−3. The error function is no longer applicable on the bottom-left when the attraction strength is greater than a certain value. To determine the transition attraction strengthµ∗quantitatively,we study the relationship between the critical packing fractionφcand attraction strengthµ. As shown in Fig. 1(b), for different system sizes, theirφccurves are almost identical at the lower attraction strength. However,they diverge suddenly from each other whenµ >2.5×10−3.This result is in excellent agreement with previous work.[20,31]Therefore we find an estimated crossover attraction strengthµ∗=2.5×10−3to distinguish the short and long range attractions.In the rest of this paper,we will focus on the short-range attraction regime(µ<µ∗)to investigate the susceptibilityχp.
2.2. Short-range attraction susceptibility
The probability curves ofpj(φ,N,µ)versus packing fractionφfor varying short-range attraction strengthµand different system sizes are redisplayed in Fig. 2(a). It is clear that with the increasing ofµ,pjat any given value ofφincreases.Meanwhile, as the system sizeNincreases, the change ofpjas a function ofφbecomes steeper. The relationship between the critical packing fractionφcand the attraction strengthµis shown in the inset of Fig.2(a). We find thatφcvaries linearly withµfor a given system size. It supports the idea that when the attraction strength is lower than the estimated crossover valueµ∗,this short-range attraction can be regarded as perturbation which is different to the repulsive system,validating the calculation of the attraction susceptibility.
另外,为了提高学生学习的积极性确保“趣味四部曲”能在学生中长期自主地坚持下去,笔者开始设置表扬卡:在“闪电快看”这一环节中最先圈画出题目中重要条件的,奖励一张表扬卡;“趣味怪读”读得有声有色、读出“感觉”、找对“重点”的奖励一张表扬卡;上台当小先生来“情境说题”的,不管说得对错、好坏,只要敢上台展示自己,一律发表扬卡一张;“动笔解题”完成后,点名回答正确的,也有表扬卡。笔者每周对学生所获得的表扬卡统计一次,评出“闪电看”周冠军、“趣味读”周冠军、“最佳小先生”“计算高手”,在全班进行表彰。

Fig.2.(a)Probability of finding jammed states pj(φ,N,µ)versus packing fraction φ for varying attraction strength in 2D system. Square,circle, and diamond symbols correspond to different attraction µ =0,5×10−6, and 1×10−5. The solid and dashed lines represent the different system sizes N =256 and N =1024. The fitting curves based on error function(3). Inset: φc(N,µ) vs. µ for N =256(square)and N =1024 (circle). (b) Data collapse of the fraction of jammed states for varying system sizes N=256,512,1024,2048 with µ =1×10−5 in 2D and 3D, respectively. The solid lines are the data collapse for varying system sizes without attractionµ =0.
In Fig. 2(b), the probabilitypjof different system sizes can be collapsed by plotting versus(φ −φc)Nν. We find that data collapse for positive values of the exponentν, whenpjapproaches a step function in the system size infinite. However,the values ofνproviding the best collapse for the plotted range ofNis different for 2D and 3D systems. We obtainedν=0.5 in 2D andν=0.42 in 3D systems,this is exactly the same as what is observed for systems without attraction.[7,32]
As the attraction strengthµis an independent control parameter for small values. By the finite-difference method,we can approximate the short-range attraction susceptibility as

where bothµandµ'are in the short-range attraction regime.
Usingµ'=5×10−6,µ=0 in the finite difference, we obtain smooth curves for 2D(Fig.3(a))and 3D(Fig.3(b))systems. Choosing other values ofµin the short-range attraction regime to computeχpdoes not change our results.

Fig. 3. (a) The attraction susceptibility curves in 2D system with different system sizes N =256, 512, 1024, and 2048 calculated by finite difference method. (b) The short-range attraction susceptibility curves in 3D system with different system sizes N =256, 512, 1024, and 2048. The solid lines are the derivative of the fitting lines in Fig.2(a)with respect toµ.
We find that the short-range attraction susceptibility exponentχpis clearly different between 2D and 3D systems.
2.3. Finite size scaling
Since we have two control parameters, the packing fraction and the attraction strength,a two-variable finite-size scaling function can be constructed for the jamming probability.There is significant evidence that the upper critical dimension of the jamming transition isd=2.[33,34]Ford ≥2,we could expect that finite scaling depends not on linear system size,but on particle numberN. So we propose that

where ∆φis the distance from the jamming transition for the infinite system without attraction ∆φ=φ −φ∞c.
Combined with Eq.(1),we obtain the scaling form of the short-range attraction susceptibility as
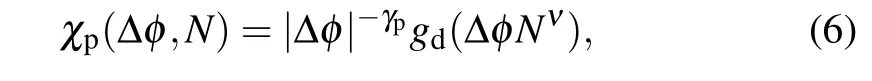
Figure 4 shows the finite-size scaling ofχp. The points and curves are perfectly matched and the peaks ofχpN−γpνare at(φ −φ∞c)Nν=−0.05 for both 2D and 3D systems. We find an excellent scaling collapse in agreement with the prediction of Eq. (6), wheregdis the derivative of the complementary error function. By comparing the goodness of the scaling collapse as the parameters are varied, we calculate the value of critical exponentsνandγp. The theoretical value ofνshould be 0.5 for the jammed solid of soft vibrational modes.[35,36]We obtain the value ofν=0.5 for 2D system andν=0.42 for 3D system,which are the same values used in Fig.2(b). It has been shown that the value ofνis independent of the dimension of the system,but the precise numerical value varies widely throughout the previous research.[37–39]So, establishing the precise value ofνand determining whether all these values of exponentνare the same is still a crucial issue. Our results are in good agreement with the results of theoretical analysis whend=2,while the value ofνis obviously smaller in the case ofd=3,but this result remains in excellent agreement with previous works.[32,40]

Fig. 4. Plot of scaled attractive susceptibility χpN−γpν vs. scaled critical volume fraction (φ −φ∞c )Nν for the data of Fig. 3. We find a good scaling collapse using values φc = 0.8412,γp = 2.0,ν = 0.5 in 2D and φc =0.6442,γp =1.57,ν =0.42 in 3D. Data point symbols correspond to those used in Fig.3.
Further more, our results show thatγp=2.0 for 2D system andγp= 1.57 for 3D system. The value ofφ∞cin 2D system is about 0.8412(Fig.1(a))and in 3D system the value ofφ∞cis 0.6442.
3. Discussion and conclusion
In conclusion, we find that the attraction strength can be divided into long and short range attractions in particle systems which exhibit distinct characteristics. Our study reveals that at the jamming point, the system is infinitely susceptible to the short-range attraction which makes the jamming transition occur at a lower value of packing fractionφ.By finite size scaling analysis we find that the short-range attraction susceptibility exhibits power law divergence. More work needs to be done to understand the interplay of the jamming transition and long range attraction. Foremost,we hope to validate our conclusions through experimental methods in the future. There has been a lot of research on how to systematically tune the attractive interaction in experiments.[41,42]Based on the colloidal particle system, the particle system with different attraction strength can be obtained by adjusting the temperature.Experimental verification of our predictions can be done in granular materials and non-Brownian colloids. For colloidal systems with short-range attraction,how the attractive interaction extends the jamming phase diagram to qualitatively different phenomena will be interesting to explore next.
- Chinese Physics B的其它文章
- Coarse-grained simulations on interactions between spectrins and phase-separated lipid bilayers∗
- Constraints on the kinetic energy of type-Ic supernova explosion from young PSR J1906+0746 in a double neutron star candidate∗
- Computational model investigating the effect of magnetic field on neural–astrocyte microcircuit∗
- Gas sensor using gold doped copper oxide nanostructured thin films as modified cladding fiber
- Exact explicit solitary wave and periodic wave solutions and their dynamical behaviors for the Schamel–Korteweg–de Vries equation∗
- Suppression of ferroresonance using passive memristor emulator

