Evolutionary game analysis of problem processing mechanism in new collaboration

ZHANG Ming, ZHU Jianjun,*, and WANG Hehua

1. College of Economics and Management, Nanjing University of Aeronautics and Astronautics, Nanjing 211106, China;2. School of Business, Jinling Institute of Technology, Nanjing 211169, China

Abstract: This paper analyzes a problem processing mechanism in a new collaboration system between the main manufacturer and the supplier in the “main manufacturer-supplier” mode,which has been widely applied in the collaborative development management of the complex product. This paper adopts the collaboration theory, the evolutionary game theory and numerical simulation to analyze the decision-making mechanism where one upstream supplier and one downstream manufacturer must process an unpredicted problem without any advance contract in common. Results show that both players ’ decision-makings are in some correlation with the initial state, income impact coefficients, and dealing cost. It is worth noting that only the initial state influences the final decision, while income impact coefficients and dealing cost just influence the decision process. This paper shows reasonable and practical suggestions for the manufacturer and supplier in a new collaboration system for the first time and is dedicated to the managerial implications on reducing risks of processing problems.

Keywords: collaborative development management, unpredicted problem, problem processing mechanism, evolutionary game theory.

1. Introduction

The concept of the main manufacturer-supplier (M-S)mode is driven by the collaboration between the main manufacturer and some suppliers, and has been applied widely in the collaborative research and development(R&D) program of complex products, like aircraft, aircraft engines, mobile communication systems, national smart grid systems and railway transportation systems [1]. The M-S mode is designed to solve problems related to multidisciplinary integration, multi-enterprise participation, customization, and other supply chain issues that impede the optimization [2,3]. In the M-S mode, the main manufacturer is responsible for the whole design and assemble of the end product, and procurement from the suppliers [4-6] and the suppliers are responsible for the detailed design and production of the parts, and providing the most suitable finished parts to the manufacturer instead of the parts ’ technology [7-9]. The M-S mode requires the manufacturer and the suppliers to share the benefits as well as the costs and risks [10], and the R&D processes of complex products are at the expense of high costs and high risks [11]. Therefore, it is easier for the participants in the M-S mode to establish a long-term trusted R&D partnership. For example, Boeing and Airbus, the two biggest manufacturers of large passenger aircraft worldwide, have employed the M-S mode for a very long time and have achieved substantial success with their old suppliers [12].

However, increasing new M-S collaboration R&D programs of the complex products are still being established.Two reasons can be taken into consideration for explaining this phenomenon. Firstly, with the advancement of material civilization, the progress and innovation of science and technology, the requirements for the complex products are becoming larger and more diversified. Some consumers of complex products nowadays, such as some emerging large logistics enterprises like UPS, have more customized requirements for aircraft. Some traditional airlines demand more aircraft to cope with the growing number of passengers. In the example of the 737MAX project, Boeing canceled its contract with GKN Automotive, the engine thrust device supplier. In order to increase production, it adopted the Leap-1B engine and established a new relationship with CFM International on November 11, 2015, according to the Wall Street Journal report. Secondly, the emergence of some new M-S collaborative relationships is to meet the needs of national strategic development. For example, China has set up its own large passenger aircraft manufacturing enterprise,the Commercial Aircraft Corporation of China Ltd(COMAC), in May 2008. The establishment of the new manufacturer has inevitably led to new relationships with many international suppliers of aircraft parts.

New relationships would lead to some new problems,such as the disputes on culture and strategy [13-15],technology [16-18], cost and price [19-21]. Some problems may have never come up or been considered before by some experienced manufacturers or some experienced suppliers in the old R&D projects. Thus, the participants would have never thought about contracting for the neglected problems before their collaboration. This kind of problems is defined as unpredicted problems in this paper.

As tiny problems may cause huge loss especially for complex products, the unpredicted problems are easily overlooked but crucial. In the C919 project, the relationship between its manufacturer, COMAC and the suppliers is newly established. During the collaboration between COMAC and CFM International, CFM International provides previously the invented CFM LEAP-1C engines due to the high cost of inventing a new engine. This creates technical problems because the engines are just close to rather than completely suited for the original C919 design. Some technical problems are unpredicted even for the experienced CFM International. Still, some unpredicted communication problems exist because COMAC is less experienced and there are cultural differences between them. This kind of problems may cause much more loss of delay than the contracted problems as the unpredicted problems have not been fully considered in the schedule of the R&D process. Therefore, it is essential to do some research on the unpredicted problems in a new M-S collaboration system, which has not been fully considered in early literature. Modeling and simulation can better facilitate the research on the unpredicted problem in a new M-S collaboration system for the R&D of the complex product. However, the characterization of the unpredicted problem in a quantitative model is a difficult work. Besides, the depiction of the behaviors and attitudes of the manufacturer and the suppliers towards processing an unpredicted problem, which is called the problem processing mechanism in this paper, is another intricate but practical work. Moreover, it is complicated to group the factors that influence the payoffs of the manufacturer and the supplier after every behavior.

The gaps are filled with the evolutionary game theory(EGT) applied in this paper. The EGT is different from the classic game theory in that the hypothesis of EGT’s objects is no longer rational but boundedly rational. It is dedicated to the research that players cannot fully grasp the whole information, and their decision-making would evolve based on the updated knowledge of information on long-term benefits [22]. In practice, when the manufacturer and the supplier face an unpredicted problem in a new collaboration, the information they can obtain is extremely incomplete and the collaboration is likely to be extremely asymmetric, especially for less-experienced participants like COMAC. They may come across a problem but in low abilities to process it. Besides, in the new M-S collaboration, the manufacturer and the supplier have to share the risk and profit with regard to the unpredicted problem. As a result, the manufacturer and the supplier cannot get to know more about the unpredicted problem especially before discovering the problem. Moreover, both sides are not familiar with each other especially in a new collaboration system, and they cannot even precisely predict the decision the other player will make when making their own decision. Thus the manufacturer and the suppliers are boundedly rational. Hence, the classic game theory is limited in this research because it requires complete information and that participants are completely rational. Furthermore, compared with the classic game theory, the EGT emphasizes a dynamic equilibrium. In this paper, the evolving decision-making of the manufacturer and the supplier is detected on their longterm benefits in this work. Therefore, the EGT is used here to address the following three research questions:(i) How many kinds of final decisions might the manufacturer and the supplier make, and what are the associated scenarios and their existing conditions? (ii) Will the initial states of the manufacturer and the supplier affect their final decisions, and if so, how? (iii) How do the manufacturer and the supplier make decisions based on the income and cost of processing the problem? This paper has been dedicated to the theoretical and practical implications from two aspects. Firstly, this study draws upon the EGT identifying the influence of the initial proportion of the strategic choice on processing an unpredicted problem within a new M-S collaboration. This identification can assist the manufacturer and the supplier in making better decisions and avoiding hesitation delay. Secondly,the application of the evolutionary game identifies the factors related to the income and cost of processing an unpredicted problem, such as the income with the problem processed and the problem processing investment.Examing these factors can help the manufacturer and the supplier make decisions. This also identifies which factors should be focused on to reduce risk.

2. Literature review

The work presented in this paper is based on two main themes found in the literature: the M-S mode and the problem processing mechanism in M-S collaboration. Both themes draw on contribution to an understanding of the collaboration income and cost.

2.1 M-S collaborative mode

The M-S collaborative mode has been increasingly popular in the applications of various kinds of supply chain areas, including the textile and clothing industry [23], environmental sustainability [24,25], and integration of raw materials [26], attracting increasing researches on it. Most of the literatures focus on the application of the R&D process of the complex product. Pun and Ghamat [27]considered two competing suppliers, who have to develop a key component for their products, and have to decide whether to develop a different component or form an R&D joint venture (RJV) with their competitors (manufacturers) to develop a common component. They studied how much optimization research should be carried out to improve the quality of this component and reduce R&D investment. Zhang et al. [28] believed that the performance and market uncertainty of the developed technology are uncertain. Original equipment manufacturers(OEMs) (manufacturer) can license the technology to a third party and share the revenue with contract manufacturer (Supplier). They describe equilibrium pricing and licensing strategies in two situations, that is, making licensing decisions before or after the uncertainty is resolved.

As this mode is about the collaboration between the upstream supplier and the downstream manufacturer,discussions on the collaboration income and the sharing cost are also the focuses of some M-S researchers. Chen et al. [17] believed that the OEMs are also centralized,and the interaction between key component suppliers and OEMs is strategic. It has an impact on how existing OEMs choose their product lines and interact with potential competitors. They prove that according to the cost structure and relative performance of the product line extension, if the low-end product line extension is produced by competitors rather than by themselves, even if the OEM cannot get any license revenue from it, the OEM may benefit more from it. Jin et al. [29] considered a duopoly model, in which two supply chains are composed of a manufacturer and a supplier respectively. They sell alternative products in the market. When each manufacturer orders components from its supplier and decides whether to integrate with the supplier, they focus on how to reduce the manufacturer’s cost. Nosoohi and Nookabadi [30] studied the supply contract design of leading manufacturers facing stochastic demand in the sales season, and studied the optimal profit distribution among contract partners under different contracts.

However, to the best of our knowledge, most of the literature are based on the consideration of a mature or longterm collaboration system. In a newly established M-S collaboration supply chain, more factors should be considered in terms of both income and cost, which should be much more complicated, especially when the risk to be shared would be much more than in a mature or longterm system.

2.2 Problem processing mechanism in M-S collaboration

To the best of our knowledge, the problem processing mechanism in most of the supply chain literature is usually discussed within the contracting issue. General supply chain problem without consideration of the collaboration would be dedicated to maximizing the interests of individual practitioners separately instead of the overall interests of the supply chain [31-34]. However, in the M-S collaborative supply chain, the manufacture and the supplier have to share the income and cost all the time. Processing a problem should consider not only the benefits of the manufacturer and the supplier separately but also the whole payoffs of both [7,35,36]. Prior researches have been done on the collaboration income and the sharing cost with regards to processing a problem in the M-S collaborative supply chain. Chiang and Wu [37] proposed an incentive-compatible mechanism on suggesting when to accelerate the R&D timeline and best engage the supplier.Wu and Kao [38] investigated the competitive and cooperative interactions between the independent remanufacturer (manufacturer) and the original equipment manufacturer (supplier) regarding technology licensing or RJV mechanisms to provide suggesions that will help achieve better outcomes beyond the firm boundary.

Regarding processing an unpredicted problem without contractual constraints, especially in a newly established immature system, it would be very easy to give rise to an argument between the manufacturer and the supplier over the sharing issues on income, cost, and even the risks.However, to the best of our knowledge, few literature have been focused on this kind of research. Compared with [37] which is about new project development contracting, this paper focuses more on the complex product R&D, uncontracted problem processing mechanism, and the long-term collaboration relationship.

2.3 Aims of this study

Most of the literature reports emphasize the importance of a collaborative relationship between a manufacturer and a supplier, in particular, which falls under the M-S collaborative mode. Some researchers solved problems arising from that mode by shifting to a study of contracting. However, many problems in the collaborative development process still cannot be predicted beforehand, especially for a new M-S collaborative system, and allowances must be made for them.

The work presented in this paper differs from previous researches in that it focuses on how the manufacturer and its supplier in a new system make decisions about dealing with unpredicted problems without any advance relative contract, and it models the problem processing mechanism as a decision that requires prior initial decisions by the two players and includes the predictable income and cost associated with dealing with the problem.

An additional factor is that when individuals make evolving decisions on their long-term benefits, they are limited in their rationality, including the problem decision tractability, the cognitive limitations, and the decisionmaking period, which constitute the elements of bounded rationality [39]. The EGT offers better assistance with problem-solving when players are boundedly rational(e.g., [40-42]). For practical and beneficial reasons, the subsequent discussion is based on bounded rationality.

3. Model

To address the research questions posed in Section 1, the work presented here includes consideration of a suppliermain manufacturer evolutionary game-playing model with the following features.

Supply chain structure: A new collaboration system with one upstream supplier and one downstream manufacturer who must process a problem in common is considered here. Both are assumed to be boundedly rational.If the problem is dealt with, the quality of the final product will be improved. The framework is applicable for many collaborations in the M-S mode, in which the manufacturer (e.g., COMAC) and the supplier (e.g., Honeywell) can share the increased income to be derived from processing the problem.Income structure: If neither of the firms chooses to take measures to solve the proposed problem through collaborative development, the manufacturer and the supplier are assumed to acquire only the conversion incomes πmand πs, respectively.

Fixed incomes are assumed for both if the problem is dealt with, regardless of who takes measures to solve the problem. These incomes are designated Imfor the manufacturer and Isfor the supplier. Considering that the ability and attitude of each firm differs concerning the collaborative development process, income impact coefficients for the manufacturer and the supplier are assumed as α and β, respectively. That is, the conversion incomesπmand πsfor both increase by either α or β if the manufacturer or the supplier takes measures to solve the problem.The stronger the ability and the more positive the attitude,the higher the income impact coefficient. D represents“deal with the problem”, and N represents “not deal with the problem”. Thus, the incomes for the supplier and the manufacturer respectively in the situation that both choose to deal with the problem (strategy condition DD)can be obtained:

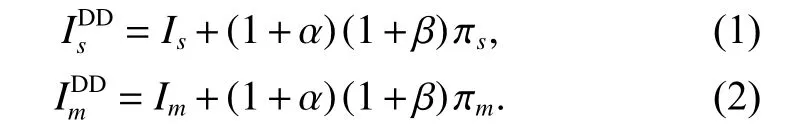
Accordingly, the incomes for the supplier and the manufacturer in other situations can be also obtained.

Cost structure: Regarding the cost of processing the problem, some degree of dealing costs is assumed here.The following assumptions about dealing costs for both are made for four cases: (i) If both choose to take measures to solve the problem, the dealing costs for the manufacturer and the supplier will berespectively. (ii) If the manufacturer takes measures to solve the problem alone (strategy condition ND), the dealing costs for the manufacturer will bewhile that for the supplier will obviously be zero. (iii) If the supplier takes measures to solve the problem alone (strategy condition DN),the dealing cost for the supplier will bewhile that for the manufacturer will clearly be zero. (iv) If neither of the firms takes measures to solve the problem (strategy condition NN), the dealing costs for both will, of course, be zero.

As well, opportunity costs exist for both in the problemproposing mechanism which is considered to possibly exist in the newly established “M-S” system [43]. They are assumed to be cmand csfor the manufacturer and the supplier respectively. In fact, if the manufacturer is more likely to propose the problem than the supplier, the manufacturer will be charged with a greater risk of loss while the supplier will be charged with a less one. Thus, the costs for the supplier and the manufacturer respectively in the situation that both choose to deal with the problem can be obtained:

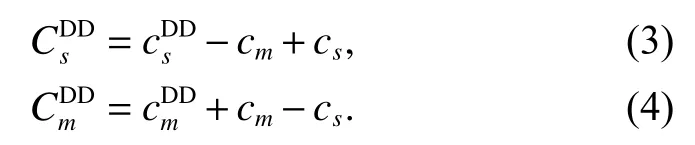
Accordingly, the costs for the supplier and the manufacturer in other situations can be also obtained.

Payoff matrix: The two firms are assumed to make their choice about whether to deal with the problem at the same time. According to (1) to (4), the payoffs for the supplier and the manufacturer respectively in the situation that both choose to deal with the problem can be obtained:

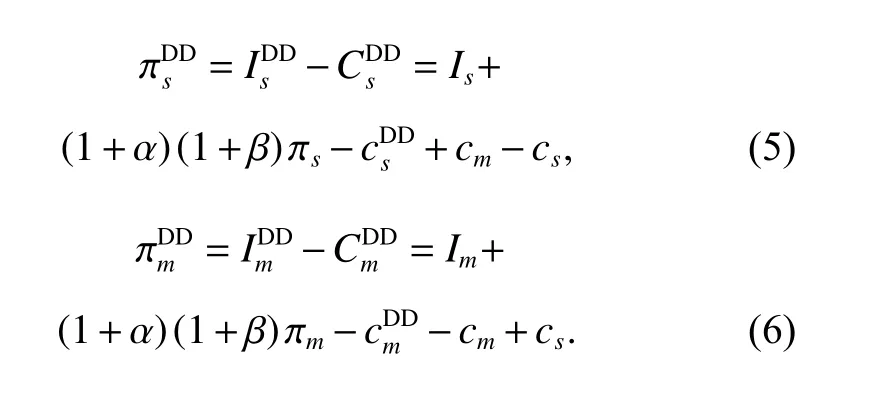
Accordingly, the payoffs for the supplier and the manufacturer in other situations can be also obtained. Then the payoff matrix for the manufacturer and the supplier are obtained as indicated in Table 1.

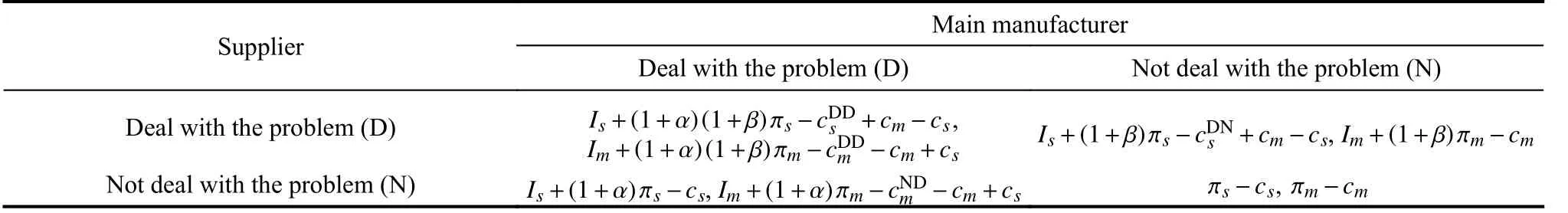
Table 1 Payoff matrix for the main manufacturer and the supplier

Therefore, there exist four strategy conditions for the manufacturer and the supplier, they are { DD,DN,ND,NN}.

4. Analysis

4.1 Equilibrium points

This paper intends to explore the evolutionary processes of the two players, the manufacturer and the supplier,making decisions on their long-term benefits. Thus, the probability of the supplier choosing D is supposed to be x, while that of choosing N is 1−x. Similarly, the probability of choosing D for the manufacturer is supposed to be y, while that of choosing N is 1 −y.

According to the Malthusian equation [44], the replicator dynamics equations for the supplier and the manufacturer can be easily established:

where UsD, UsNandrepresent the expected payoff of D and N, and the average expected payoff for the supplier,while UmD, UmNandrepresent those for the manufacturer, which can be obtained based on Table 1.

Therefore, the following two-dimensional dynamical system (I) for the evolutionary game-playing model can be derived:

For convenience in performing the following analysis,note that

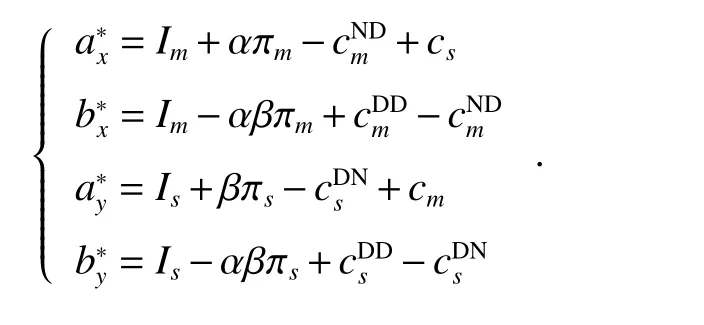
Proposition 1There must exist at least four Nash equilibrium points in the system (I): E1( 0,0), E2( 1,0), E3(0,1)and E4( 1,1). Giventhe pointis the potential mixed strategy Nash equilibrium point in the system.

4.2 Stability analysis of the equilibrium points

According to the research conducted by Weibull [44],Selten [45], and Christian et al. [46], and the method proposed by Friedman [47], not all of the Nash equilibrium points shown in Proposition l can be the evolutionarily stable points. To explore whether the Nash equilibrium point in Proposition 1 is the evolutionarily stable strategy(ESS) in the system, we will analyze the local stability of the Jacobian matrix of the two-dimensional dynamical system and thus determine the stability of each Nash equilibrium point. The Jacobian matrix of the dynamical system (I) is

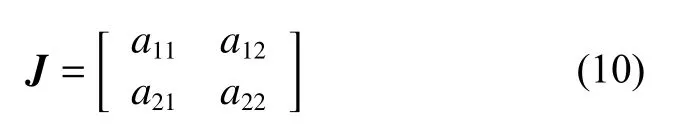
Then, the signs of the calculated relative determinant and trace of J can be used here to ascertain the local stability of the Nash equilibrium points. The result shown in Proposition 2 can be acquired.

Proposition 2The Nash equilibrium points E1( 0,0),E2( 1,0), E3( 0,1), and E4( 1,1) may be the ESSs of the system (I), while the potential mixed strategy Nash equilibrium pointcan be the only saddle point of the system. The following four scenarios shown in Table 2 are possible in the system (I).

Table 2 Possible scenarios and the relative ESSs

Based on Proposition 2, the existence of an ESS in the system according to the following two corollaries will be explicitly discussed.

Corollary 1Given0, the potential mixed strategy Nash equilibrium pointexists as the saddle point of the system (I) as shown in Table 3 and verified in Fig. 1.

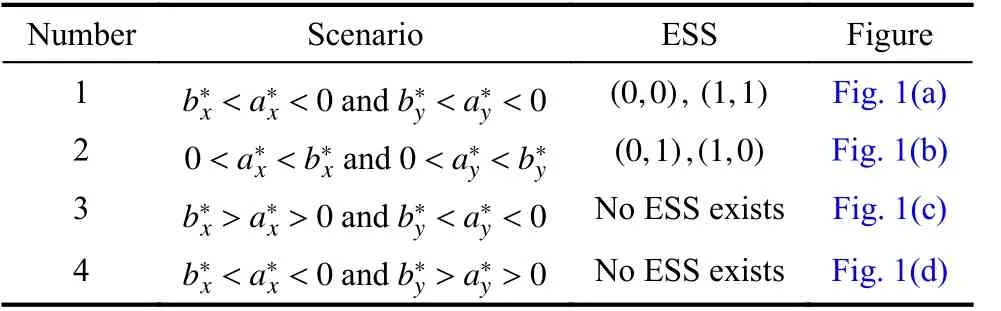
Table 3 Scenarios in which saddle point E5 exists

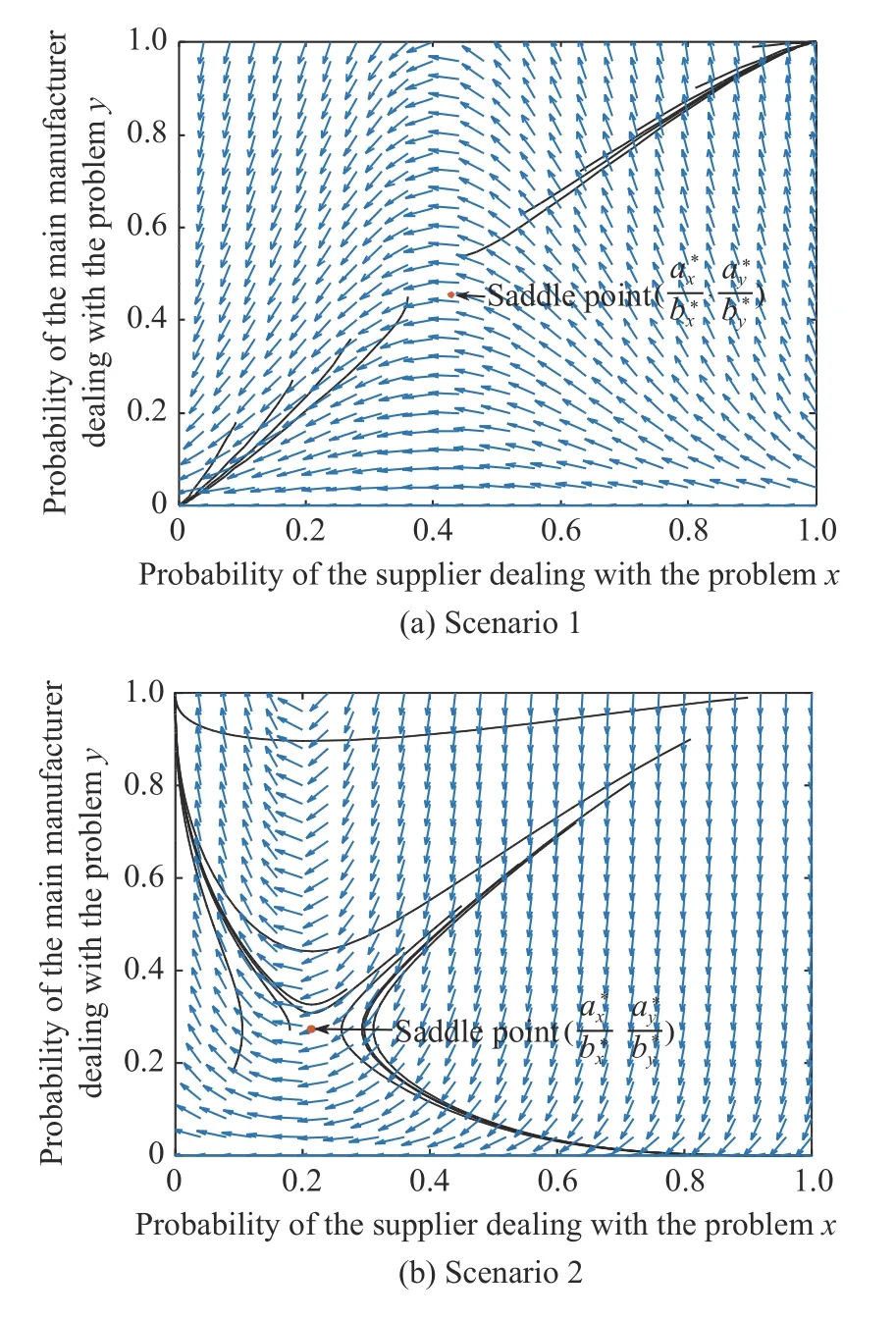
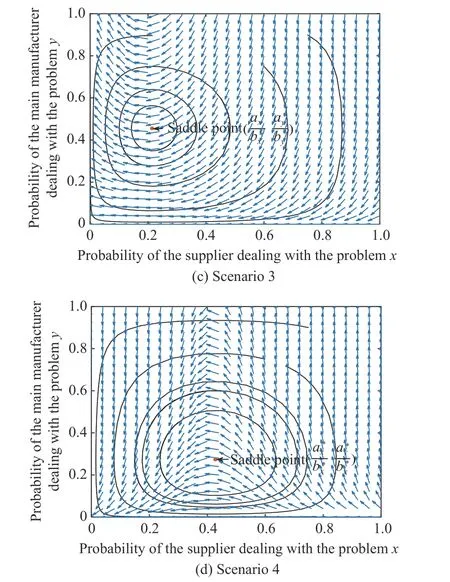
Fig. 1 Phase diagrams and vector fields for system (I) in the scenarios in which saddle point E5 exists

Corollary 2There are four detailed scenarios in which the potential mixed strategy Nash equilibrium pointdoes not exist in the system (I), as shown in Table 4 and verified in Fig. 2.

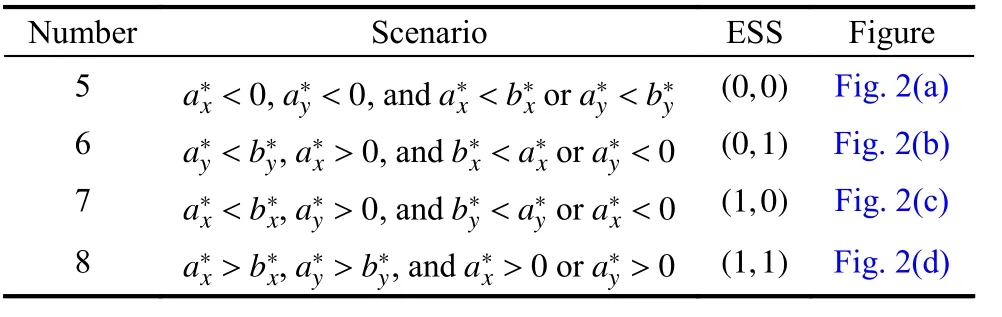
Table 4 Scenarios in which saddle point E5 does not exist

Based on the two corollaries, the effects of initial probability, income impact coefficient, and cost of dealing with the problem can be observed in detail, as set out in Subsections 4.3, 4.4, and 4.5. In practice, the manufacturer can benefit directly from the end product and the general manager of the R&D process. When there is a problem in the R&D process, it should guide the suppliers in processing the problem. Due to the leading role of the manufacturer in the collaborative R&D process, the manufacturer is assumed to deal with the problem. Therefore, only Scenarios 1, 2, 6, and 8 will be discussed in detail in the following sections.

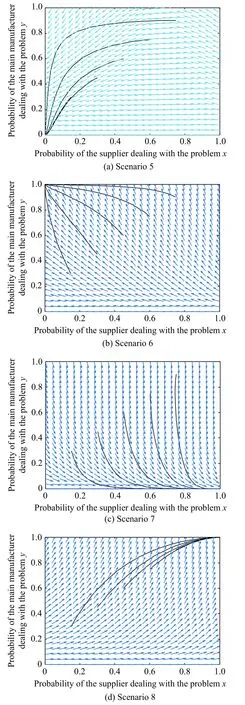
Fig. 2 Phase diagrams and vector fields for system (I) in the scenarios in which saddle point E5 does not exist

4.3 Impact of initial probability

The impact of the initial probability is first established here. As shown in Fig. 3 and Fig. 4, the evolution states with different initial probabilities are expressed in the form of curves identified by different colors. The initial probabilities, (0.25, 0.4), (0.25, 0.6), (0.75, 0.4), (0.75,0.6), (0.75, 0.8), and (0.75, 0.98), are shown in orange,rose red, red, green, light blue, and blue, respectively.The dotted lines represent the changing curves of x over time, and the solid lines signify those of y. Moreover, in order to show more clearly in simulation figures, the values ofare set in Table 5 based on the values of the parameters as Im, Is, πm, πs, α, β,close to the practical application.

Fig. 3 Evolution curves with different initial probabilities in the scenarios in which saddle point E5 exists

From Fig. 3(a), for the scenario where the ESSs are(0,0), (1,1), the following can be observed: (i) When initial probabilities for both the supplier and the manufacturer choosing D is small (≤ 0.5), neither player will choose D in the end. From this aspect, the small initial probabilities (≤ 0.5) are decisive for the manufacturer and the supplier in making decisions on N. When the initial probability for the supplier choosing D keeps smaller than 0.5, and that of the manufacturer grows bigger than 0.5, the probability for the supplier will first increase for a while, and then the probabilities for both will decrease to 0. The period when the decision probability for the supplier first increase and then decrease is regarded as the hesitation period for the supplier in the analysis. This verifies the decisive role of the manufacturer in the supplier’s making decision. Due to the leading role of the manufacturer considered, the manufacturer is assumed to choose to deal with the problem. Therefore, this kind of situation should be avoided in the next discussion.(ii) When the initial probability for the manufacturer is small (≤ 0.5), and that for the supplier is greater than 0.5,surprisingly, the probability for the supplier choosing D will first decrease for a while, and then the probabilities for both will increase to 1. That the supplier hesitates is considered due to the initial state of the manufacturer.This verifies the decisive position of the small initial probability in making decisions from another aspect.However, the supplier’s initial probability may have an effect on both choosing to deal with the problem in the end. This kind of interesting situation happens when the manufacturer is less experienced like COMAC and has no experience in processing an unfamiliar problem. Thus,the relatively experienced supplier plays a strong decision controller in this situation. (iii) When both initial probabilities are greater than 0.5, they will increase to 1.This reflects the decisive role of the initial states in the final decision-making from another aspect. Besides, if the initial probability for the supplier remains constant, the increase rate for the supplier will increase with the increasing of the initial probability for the manufacturer.The period between the initial state and the final decision state is considered as the reaction period for the participant. Thus, if the increase rate for the supplier increases, the reaction period for the supplier becomes shorter. Therefore, this phenomenon means when the manufacturer shows more willingness in dealing with the problem, the supplier would decide to deal with the problem in a much shorter period. This reflects the leading role of manufacturers in the whole R&D management.(iv) If the probabilities for both reach 1 as the ESS, the probability for the manufacturer fluctuates over a very small range around 1 rather than remaining stable. This fluctuation is considered as another form of hesitation for the manufacturer. This may be due to the manufacturer’s lack of experience in processing the unpredicted problem.

From Fig. 3(b), for the scenario where the ESSs are(1,0), ( 0,1), the following can be observed: (i) In the situations of (0.25, 0.4), (0.75, 0.4), (0.75, 0.6), and (0.75,0.98), the probability of the one with higher initial probability will become 1 while that of the other will become 0.This reflects that the initial states are decisive on decisionmaking. Due to the leading role of the manufacturer considered, the manufacturer is assumed to choose to deal with the problem. Therefore, the situations in Scenario 2 where the initial probability of the supplier is higher than that of the manufacturer should be avoided in the next discussion. (ii) Interestingly, the probability of the one whose final probability is 1 will first decrease for a while,while the probability of the one whose final probability is 0 will decrease immediately. Because one participant chooses not to deal with the problem, the other participant would hesitate. This reflects the mutual pull-in between the manufacturer and the supplier in the M-S collaboration R&D mode.

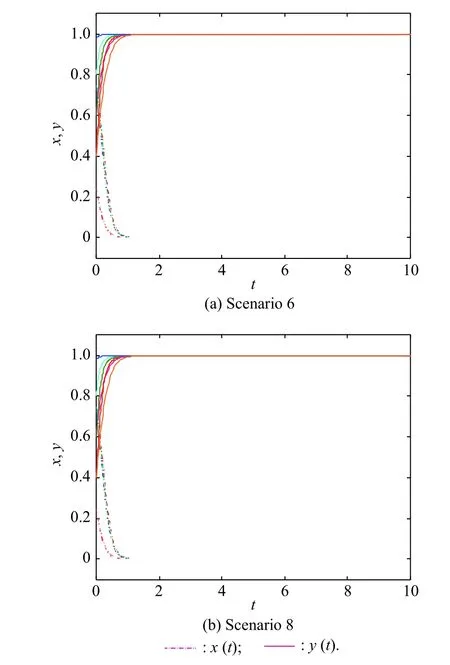
Fig. 4 Evolution curves with different initial probabilities in the scenarios in which saddle point E5 does not exist

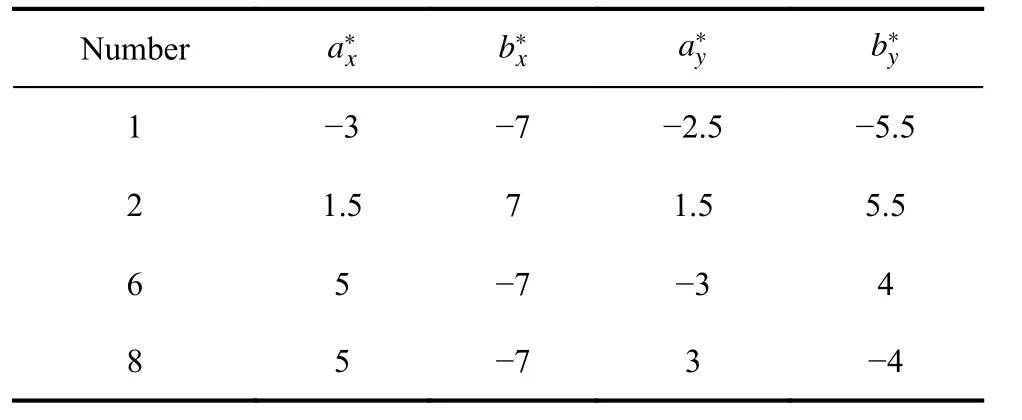
Table 5 Value list of the parameters in the analysis of the impact of initial probability

From Fig. 4(a), for the scenario where the unique ESS(0,1)exists, the following can be observed: (i) If the initial probability for the supplier remains unchanged, its descent rate changes slightly no matter how much initial probability for the manufacturer changes. Thus, the initial states are much more decisive than the manufacturer though as the leading role of the R&D management.(ii) If the initial probability for the manufacturer remains unchanged, the initial probability for the supplier becomes greater.

From Fig. 4(b), for the scenario where the unique ESS(1,1)exists, the following can be observed: (i) If the initial probability for the supplier remains unchanged and the initial probability for the manufacturer rises, the increase rate for the supplier stays almost the same. This reflects that the initial state is more decisive than the manufacturer who should be the leader in the R&D management in decision making from another aspect. (ii) If the initial probability for the manufacturer remains unchanged, the increase rate for the manufacturer becomes greater as the initial probability for the supplier increases. The manufacturer makes a much faster decision to deal with the problem when the supplier shows more willingness to deal with the problem in the initial state. (iii) The probability for the manufacturer fluctuates over a very small range around 1. The kind of hesitation may be due to the lack of experience for the manufacture in facing an unpredicted problem. This also happens when it is in Scenario 1 and the strategy station is DD.

Based on the observations about Fig. 3 and Fig. 4, the following conclusions can be drawn.

Firstly, the initial states play a decisive role in final decision-making. Due to the limitations of manufacturers and suppliers in their perception and prediction of unpredicted problems, it is difficult for them to make accurate judgments at the initial state. The accumulation of experience is an effective way for the manufacturer and the supplier in examining and dealing with a problem timely and properly.

Secondly, the manufacturer’s initial state plays a leading role in the supplier’s final decision making. When the manufacturer is more willing to deal with the problem in the initial state, the supplier would make a faster decision to deal with the problem. Whereas, when the manufacturer is not confident in dealing with the problem in the initial state, the supplier would hesitate to make a final decision to deal with the problem. As time is precious in the R&D of the complex product, a precise judgment of the unpredicted problem processing mechanism by the manufacturer is beneficial for the efficient R&D process.

Thirdly, there exists mutual pull-in between the manufacturer and the supplier during the decision-making process regarding the initial state of one another. Neither the manufacturer nor the supplier would like to deal with the problem alone.

Fourthly, for the supplier, the initial state of its own is more likely to affect its final decision rather than that of the manufacturer. Therefore, it would be a very big challenge but an effective way for the manufacturer to better control the supplier’s idea of dealing with a problem in the initial stage. In this respect, it is not difficult to explain why the experienced Boeing and Airbus would take more initiative than the less-experienced COMAC.

Fifthly, in dealing with the problem, the supplier is more passive rather than active. This requires the manufacturer to develop a reasonable supplier management and control mechanism.

Sixthly, it is very hard for the manufacturer to make a final stable decision to deal with the unpredicted problem with the supplier in common. The cognitive limitation of the unpredicted problem challenges a lot for the manufacturer in managing the newly established R&D system.This again emphasizes the importance of the accumulated experience for the manufacturer.

4.4 Effect of income impact coefficient

This section discusses the effects of income impact coefficients as they relate to eight scenarios. For each scenario, how five different situations can affect the results is identified, as indicated by the differently colored curves in Fig. 5 and Fig. 6. (i) α=β (green); (ii) β goes up and αremains unchanged on the basis of situation (i) (orange); (ⅲ) β goes down and α remains unchanged on the basis of situation (i) (red); (iv) α goes up and β remains unchanged on the basis of situation (i) (light blue); (v)α goes down and β remains unchanged on the basis of situation (i) (blue). Dotted lines indicate the changes for x, and solid lines denote those for y. Generally, the manufacturer shows more willingness to deal with the problem in its starting state as it can benefit directly from the end product. However, suppliers are more reluctant to deal with this problem because they are afraid to share the cost in the initial stage. Besides, considering the constraints by some contracts, both are supposed to show some willingness to deal with the problem. Therefore, the starting points of x and y are set to be bigger than 0.5, and that of y is set to be greater than that of x. Moreover, to show more clearly in simulation figures, the starting points are set as x0=0.75 and y0=0.98. To show more clearly the changes of the evolution curves with different income impact coefficients in the Scenarios 1, 2 and 6, only parts of the curves in the range t ∈[0,10] are shown in Fig. 5 and Fig. 6(a) instead of the whole curves until the curves reach their own ESSs.

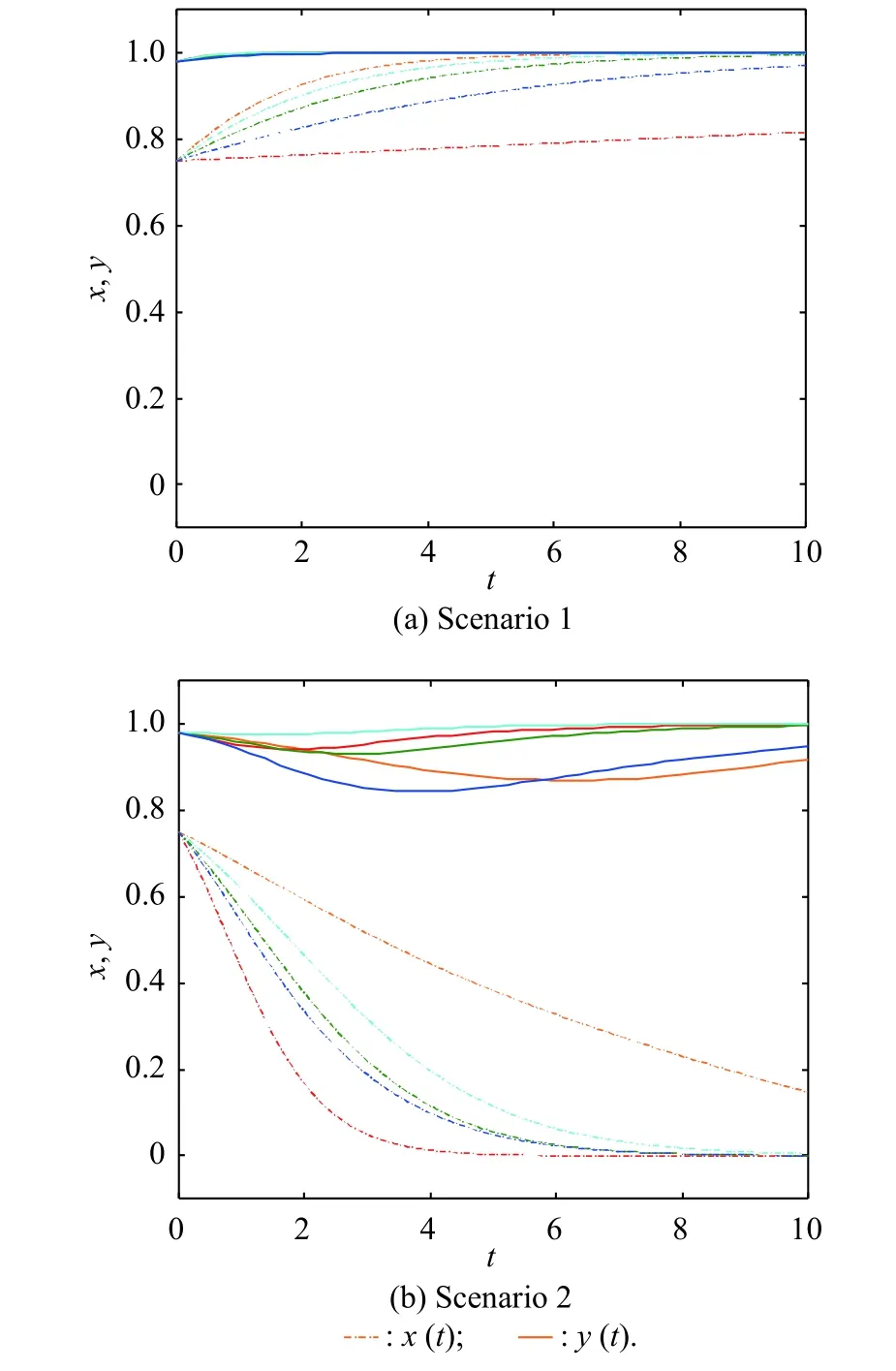
Fig. 5 Evolution curves with different income impact coefficients in the scenarios in which saddle point E5 exists

From Fig. 5(a), in which the ESSs are (0,0), (1,1), the following can be observed: (i) Changes of α and β have no effect on the final decision of either player. This means that the increased profits brought by the solved problem have no effect on either the manufacture’s or the supplier’s making decision. (ii) Changes of α and β do not influence the change rate for the manufacturer, while that of the supplier increases when either α or β becomes greater. The reaction period of the supplier becomes shorter when the increased profits brought by the solved problem for either the manufacturer or the supplier becomes bigger. However, in this situation, the reaction period remains almost unchanged for the manufacturer.

From Fig. 5(b), in which the ESSs are ( 1,0), ( 0,1), the following can be observed: (i) Changes of α and β do not affect the final decision of either player. This reflects the little effect of the increased profits brought by the solved problem on the final decisions of the manufacturer and the supplier. (ii) The change rate for the supplier declines with either α or β becoming greater. The reaction period of the supplier becomes longer when the increased profits brought by the solved problem for either the manufacturer or the supplier becomes bigger. This is contrary to the observation in Fig. 5(a), but this happens in the situation when the final decision of the supplier is not to deal with the problem, which is also contrary to that of Fig. 5(a).(iii) There is a period during which the probability for the manufacturer decreases before reaching 1. This hesitation period becomes longer as α goes down or as β goes up.This means that when the increased profit brought by the solved problem for the manufacturer becomes relatively less than before, the manufacturer would hesitate longer to decide whether or not to deal with the problem, especially when the supplier chooses not to deal with the problem. This situation is very common when the manufacturer is in a weaker position, like the less experienced COMAC, but is verified in simulation.

Fig. 6 Evolution curves with different income impact coefficients in the scenarios in which saddle point E5 does not exist

From Fig. 6(a), in which the unique ESS (0,1) exists,changes of both α and β do not influence the change rate for the manufacturer, but the change rate for the supplier increases as either α or β goes down. This means that the increased profits brought by the solved problems do not affect the reaction period of the manufacturer when only the manufacturer tends to deal with the problem. When the increased profit brought by the solved problem for either the manufacturer or the supplier becomes less, the supplier would make a quicker decision not to deal with the problem.

From Fig. 6(b), in which the unique ESS (1,1) exists,the following can be observed: (i) The change rate for the manufacturer is almost the same no matter how α orβ changes. (ii) The increase rate for the supplier increases with either α or β increases. These two phenomena are similar to what happens in Scenario 1 when the final decisions of both are to deal with the problem.

The following conclusions therefore can be drawn from the observations about Fig. 5 and Fig. 6.

Firstly, the income impact coefficients affect little on the final decisions of the manufacturer and the supplier.No matter who can get more from the profit brought by the solved problem than one another, it has little influence on either the manufacturer or the supplier making the decision.

Secondly, the income impact coefficients affect the reaction periods of the manufacturer and the supplier before making final decisions. According to the observations in Fig. 5 and Fig. 6, for the supplier, the reaction period would become shorter in the following two situations: (i) When the manufacturer and the supplier decide to collaborate to deal with the problem, and the profit brought by the solved problem for either the manufacturer or the supplier increases; (ii) When the manufacturer decides to deal with the problem alone, and the profit brought by the solved problem for either the manufacturer or the supplier decreases. Thus, to save time and reduce unnecessary reaction period during the R&D process, the manufacturer should take these two kinds of situation into consideration of the supplier management.For illustration, if the profit brought by the solved problem for the supplier increases, the manufacturer should try to keep the same decision with the supplier if the quality condition of the end product allows. This conclusion is very useful in the arrangement of the R&D schedule.

Thirdly, the manufacturer would hesitate in the situation when the manufacturer would have to deal with the problem alone. The hesitation period becomes longer when the manufacturer can obtain less profit from dealing with the problem. This kind of situation is very easy to happen when the manufacturer is in a weaker position.Thus, the R&D ability of the manufacturer is very essential for the manufacture to strive for more benefit.

4.5 Impact of dealing cost

This section discusses how dealing cost affects the eight scenarios that correspond to the numbered expressions below. For each scenario, how six different situations can affect the results is determined, as indicated by the different colored curves shown in Fig. 7 and Fig. 8. (i)(purple). In fact, in the M-S mode, if both choose D through collaboration in the meantime, in most cases, they will also choose to split the dealing cost (e.g., the collaborative development of large passenger aircraft). For this reason, only situations in whichare discussed here. The dotted lines represent changes in the curves for x over time, and the solid lines signify those for y. In this section, we set the same starting points for x and y as in Subsection 4.4. Besides, to show more clearly the changes of the evolution curves with different dealing costs in Scenarios 2 and 6,only parts of the curves in the range t ∈[0,10] are shown in Fig. 7(b) and Fig. 8(a) instead of the whole curves until the curves reach their own ESSs.

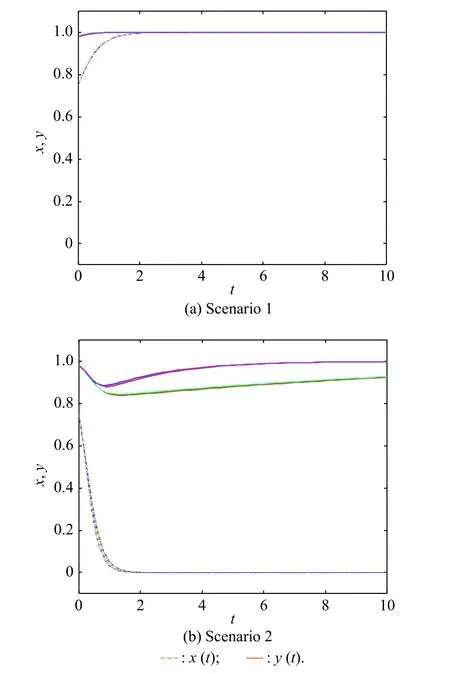
Fig. 7 Evolution curves with different dealing costs in the scenarios in which saddle point E5 exists

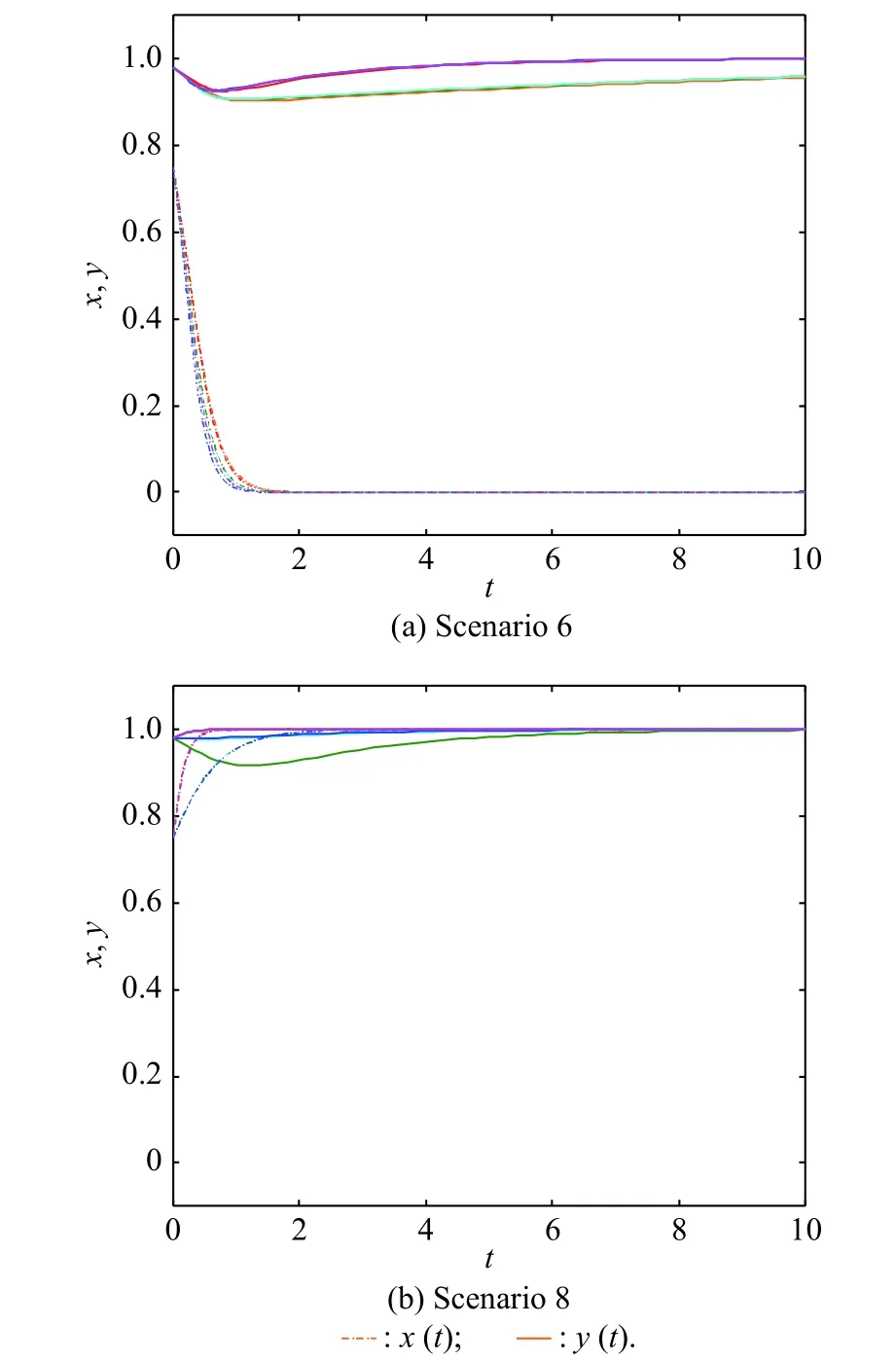
Fig. 8 Evolution curves with different dealing costs in the scenarios in which saddle point E5 does not exist

From Fig. 7(a), in which the ESSs are (0,0) and (1,1),changes in the sequence ofhave little influence on the manufacturer or the supplier making decision. Therefore, no matter how the manufacturer and the supplier choose their strategy, and no matter how much they would cost on dealing with the problem, it does not affect the final decisions.

From Fig. 7(b), in which the ESSs are ( 1,0) and (0,1),the following can be observed: (i) Change rates for the manufacturer are almost the same in situations when the sequence ofremains unchanged (e.g., sequence ofandin (1), (3), and (4) remains unchanged). Besides, when only sequence ofchanges,a tiny change occurs in the change rate of the manufacturer. The reaction period for the manufacturer keeps almost the same when its cost on dealing with the problem remains less or more than the supplier’s. (ii) The sequence of situations associated with the lowest probability of the manufacturer before reaching 1 is (1)＜(3)＜(4)＜(2)＜(6)＜(5). The manufacturer is more likely to hesitate not to deal with the problem when the manufacturer costs more on dealing with the problem alone than the supplier alone, and especially when the costs of solving problems together is the smallest in all strategic conditions. (iii) The sequence of situations associated with the change rate for the supplier is (1)＜(2)＜(3)＜(4)＜(6)＜(5). The reaction period for the supplier becomes shorter when the cost of both dealing with the problem is less than the cost of the manufacturer dealing with the problem alone, and especially when the cost of the supplier dealing with the problem alone is the smallest in all four strategy conditions.(iv) Sequence ofandhas no effect on the final state of either player. This is like the observation in Fig. 7(a).

From Fig. 8(a), in which the unique ESS (0,1) exists,interestingly, it shares identical rules with Scenario 2 when it also has an ESS of ( 0,1).

From Fig. 8(b), in which the unique ESS (1,1) exists,something different occurs: it is the sequence ofandrather than that ofandthat substantially alters the change rate for the supplier, while the sequence ofboosts the change only a little, andresults in a greater change rate. The reaction period for the supplier becomes shorter when both would cost less on dealing with the problem than the supplier alone.Thus, in this kind of situation, the supplier would be more likely to deal with the problem collaboratively instead of bearing the risk to deal with the problem alone and spend more.

Based on the above observations, the following conclusions can be drawn.

Firstly, no matter which strategy condition would cost more to deal with the problem than one another affects little on the final decisions of both players.

Secondly, the dealing costs can influence the reaction periods for the manufacturer and the supplier before they make final decisions. According to the observations in Fig. 7 and Fig. 8, for the supplier, the reaction period varies in different strategy conditions concerning the dealing cost, and the reaction period becomes shorter in the following three situations: (i) when the manufacturer decides to deal with the problem alone, and especially the dealing cost by the supplier alone is less than that by the manufacturer alone; (ii) when both decide to collaborate to deal with the problem, and the dealing cost by both collaboratively is less than that by the supplier alone.This conclusion is an inspiration for the manufacturer in the arrangement of the R&D schedule when facing with the unpredicted problem.

Thirdly, hesitation appears when the manufacturer has to deal with the problem alone. The manufacturer (the supplier) would hesitate more when the dealing cost by it alone is more than that by one another alone. However,the manufacturer would hesitate more when it has to deal with the problem alone and the dealing cost by both collaboratively is the smallest. This reflects the leading role of the manufacturer in its willingness to collaborate and the minimum cost management.

5. Conclusions and future research

This paper has investigated the ways of main manufacturers and suppliers making final decisions about processing an unpredicted problem through a process of collaborative development in a newly established collaborative system. A two-echelon supply chain is considered with an upstream supplier who is accountable for processing the problem collaboratively with the downstream manufacturer. An unpredicted problem is defined as not considered in the contract beforehand, and the manufacturer and the supplier are assumed to be boundedly rational.Then the management practices can be focused on the kind of following conditions based on conclusions regarding the impacts of the initial states, the income impact coefficients, and the dealing costs. These are also extensions of some existing literature.

Firstly, when the scenarios are settled, only the initial probabilities of the manufacturer and the supplier determine their final decisions. No matter how other factors change, they will not influence the final decisions. Therefore, the manufacturer and the supplier need to pay more attention to the initial states of each other when making decisions on processing the problem.

Secondly, [37] shows that the manufacturer’s bargaining power affects the supplier’s contract involvement.This paper shows further that both the income impact coefficients (another way of bargaining power) and the dealing costs have an effect on the decision-making processes of the manufacturer and the supplier regarding the reaction period and the hesitation period. These kinds of findings are very beneficial to the manufacturer in the management of the R&D schedule due to the precious factor of time arrangement in the R&D of the complex product. Concerning the reaction period of the supplier,the followings should be taken into full consideration in the management idea of the manufacturer when facing with the unpredicted problem including two situations in terms of the income impact coefficients as summarized in the conclusion of Subsection 4.4, and the three situations in terms of the dealing costs as summarized in the conclusion of Subsection 4.5. Besides, the hesitation period appears before one makes the final decision to deal with the problem. Moreover, both the income impact coefficient and the dealing cost affect the length of the hesitation period. Besides, it is worth noting that when the income impact coefficient of the manufacturer increases relative to that of the supplier, the manufacturer hesitates longer.That means if the manufacturer becomes more powerful in deciding the profit of the whole supply chain, such as more experienced and more capable of processing the problem, it will show more arrogance in dealing with the problem. If the manufacturer, as the general designer and inspector of the final product, is in a lower position than the supplier in the development process, such as the newly established aircraft manufacturer COMAC, it will be harder for the manufacturer to control the process, let alone the quality of the final product. Literature [37]shows that when faced with a highly competent team(supplier), the manufacturer prefers a fixed contract to a contingent contract to ensure the collaboration.

Finally, in analyzing the impact of the initial probability, the attitude of the manufacturer fluctuates a bit around dealing with the problem when the supplier has chosen to deal with the problem. In the existing analysis,the reasons cannot be found which should be dug out in the future research. This work is also applicable to the management of the unpredicted problem in an old relationship, such as the unpredicted problem in the Project 737 MAX between Boeing and its elevation sensor supplier. Although this situation may happen not that much,it would also be of great significance.

The interaction between the problem proposing mechanism and the problem processing mechanism can be further explored when considering the detailed features of the opportunity cost, such as substitutable projects, benefits of proposing the problem, scarcity of resources, and so on. Another extended research avenue is the investigation of the particular impact of a competing relationship between a collaborative manufacturer and suppliers, which is known as coopetition [48]. Suppliers can also be other manufacturers of the same equipment as the original manufacturer. The relationship between Apple, one of the biggest smartphone manufacturers, and Samsung,who is the screen supplier for Apple but also another of the largest smartphone manufacturers, provides an example of such a relationship.