Effect of surcharge loading on horseshoe-shaped tunnels excavated in saturated soft rocks

Dinchun Du, Dniel Dis, Ngocnh Do

a School of Civil Engineering, Southeast University, Nanjing, China

b School of Automotive and Transportation Engineering, Hefei University of Technology, Hefei, China

c Antea Group, Antony, France

d Department of Underground and Mining Construction, Faculty of Civil Engineering, Hanoi University of Mining and Geology, Hanoi, Viet Nam

Keywords:Surcharge loading Horseshoe-shaped tunnel Hyperstatic reaction method (HRM)Saturated soft rocks

A B S T R A C T Underground facilities are usually constructed under existing buildings,or buildings are constructed over existing underground structures. It is then imperative to account for the current overburden loads and future surface loadings in the design of tunnels. In addition, tunnels are often constructed beneath the groundwater level, such as cross-river tunnels. Therefore, it is also important to consider the water pressure impact on the tunnel lining behaviour.Tunnels excavated by a conventional tunnelling method are considered in this paper. The hyperstatic reaction method (HRM) is adopted in this study to investigate the effect of surcharge loading on a horseshoe-shaped tunnel behaviour excavated in saturated soft rocks. The results obtained from the HRM and numerical modelling are in good agreement. Parametric studies were then performed to show the effects of the water pressure, surcharge loading value and its width, and groundwater level on the behaviour of the horseshoe-shaped tunnel lining, in terms of internal forces and displacements. It displays that the bending moment, normal forces and radial displacements are more sensitive to the water pressure, surcharge loading and groundwater level.

1. Introduction

Underground structures are popular in urban areas with the increasing level of urbanization in modern societies. Underground facilities, like subway tunnels, are excavated under existing buildings,or buildings are built over underground structures.Therefore,it is important to consider the existing overburden loads and/or future surface loadings for tunnel design. On the other hand, in practical situations, tunnels have to be excavated beneath the groundwater level, like cross-river tunnels. It is also necessary to study the impact of the water table level on the tunnel lining behaviour.

According to Working Group No. 2, ITA (2000), tunnel linings should sustain the loads transferred from the surrounding soil mass and from the groundwater table. Thus, it is necessary to study the influence of these loads on the lining behaviour.Several researches have been presented to study the surface loading impact on tunnels excavated below the water table. A complete analytical solution was presented by Bobet (2001) to evaluate the lining stresses and soil deformations of a shallow circular tunnel in saturated soils.Considering the static and seismic loading conditions,Bobet(2003)derived an analytical solution for a deep tunnel excavated in a saturated poroelastic soil mass. The lining stresses were not affected by the drainage conditions. Based on the finite element method, Shin et al. (2005) investigated the pore water pressure effect on the tunnel lining,and they found that this impact depends on the lining permeability and drainage system deterioration.Katebi et al. (2013, 2015) numerically studied the surface loading impact on the lining efforts.Their results show that the geometrical and mechanical parameters of the buildings, tunnels and surrounding soils have a significant effect on the lining loads, which should be considered in the design of tunnels. By means of numerical simulations, Mirhabibi and Soroush (2012) found that the influence of surface loading on the settlement troughs induced by excavation of twin tunnels decreased as the tunnel centre-tocentre distance increased. Prassetyo and Gutierrez (2016) examined the effect of surface loading on the hydro-mechanical (HM)response of a tunnel in saturated soils. They found that the linear permeability and the soil long-term HM response have a large effect on the stability of the tunnel in saturated soils. Pan and Dias(2016) investigated the effect of pore water pressure on the tunnel face stability using the kinematic approach in combination with a groundwater flow numerical simulation. Results indicated that the critical effective pressure of the tunnel face increases with the water table elevation.Despite that the analytical solutions are very useful, they are complex to be developed when dealing with complex tunnel shapes (different from circular ones). Meanwhile,numerical simulations can overcome this difficulty but need more calculation time.Therefore,finding an accurate and efficient way is necessary to evaluate the influence of surface loading on the tunnel lining behaviour in saturated soil conditions.

This paper mainly focuses on evaluating the effects of the surface loading and pore water pressure on the lining behaviour. The hyperstatic reaction method (HRM) is adopted to estimate the internal forces and displacements of tunnel lining. Compared with the complex analytical solutions of Bobet (2001, 2003), the HRM(Duddeck and Erdmann, 1985; Oreste, 2007; Do et al., 2014; Du et al., 2018) can provide internal forces and displacements of the tunnel structure in a simpler way, which makes it suitable for tunnel design.

Tunnels excavated in saturated soils are usually subjected to the effect of groundwater(Potts and Addenbrooke,1997;Lee and Nam,2001; Shin et al., 2002, 2005; Do et al., 2019). The pore water pressure applied to the lining is zero when the lining is fully drained. In contrast, when the linings are impermeable, they should be designed to support the stresses transferred from the soil mass and the full hydrostatic pore pressure (Lee and Nam, 2001;Bobet, 2003). Therefore, to investigate the effect of pore water pressure, two extreme permeable cases are considered in this study, i.e. fully permeable (no pore water pressure) and impermeable (considering the full water loading). Then, the effects of surcharge loading value and its width, pore water pressure, and water table on the tunnel behaviour are presented.

2. Hyperstatic reaction method

As a numerical approach, the HRM was successfully used to analyse the behaviour of circular tunnels by Oreste (2007) and Do et al. (2014). This method allows one to assess the behaviour of the tunnel lining with a low computation effort.

Fig. 1. Scheme of the soil-support interaction through springs connected to the support nodes.

In this method,the tunnel lining is composed of a finite number of one-dimensional linear beam-type elements that are able to develop internal forces,as illustrated in Fig.1,where αiand αi+1are the angles of elements i and i+1 between local and global reference systems,respectively.Therefore,the direction of the normal spring attached to the node i+1 is equal to（αi+1+αi-π）/2 according to the geometric relationship. Those elements are connected to the surrounding soil through the springs at the nodes. The soil-support interaction is accomplished by means of the applied loads and the springs, as shown in Fig. 2.

According to Oreste (2007), the key to calculate the internal forces and displacements of the support is to know the displacement upof the nodes of the discretized structure, which is evaluated as

where F is the nodal force vector; and K is the global stiffness matrix, which is assembled by the local stiffness matrix of the ith element based upon the criteria of Huebner et al.(2001).The global stiffness matrix K is made up of 3n×3n elements, where n is the total number of nodes. Both the nodal force F and the unknown displacement upare composed of 3n elements.More details about the process of generating the local stiffness matrix can be found in Do et al. (2014).

The behaviour of the tunnel lining can then be evaluated once the displacement upof the node is known.

2.1. Soil-structure interaction

Neglecting the solid-fluid coupling, the soil-structure interaction is considered through normal and shear springs connected to the structural nodes. Active loads are applied to the structures by the surrounding soil mass. The values of spring stiffnesses (knfor normal springs and ksfor shear springs)are determined by means of the soil stiffnesses (ηnfor normal springs and ηsfor shear springs). According to Oreste (2007) and AFTES (1997), the soil stiffnesses (ηnand ηs) are determined by the support deformation and reaction pressure (Fig. 3).

The apparent soil stiffness η*is estimated by

Fig. 2. Schematic diagram of a horseshoe-shaped tunnel lining. σh and σv are the horizontal and vertical stresses,respectively;Ria is the radius of the inverted arch;and Rc is the crown radius.

where δ is the support deformation, η0is the initial soil stiffness(when δ is close to 0), and Plimis the maximum soil reaction pressure.Both the normal and shear spring stiffnesses in this study are determined by Eq. (2).

Based on the study in Möller (2006), the initial soil stiffness depends on the tunnel radius R, and soil Young’s modulus Esand Poisson’s ratio νs.In this case,the initial soil normal stiffness ηn，0is determined by the following empirical formula illustrated in Möller(2006):

where β is a dimensionless factor dependent on the structure geometry.Since β is not easy to be evaluated in practical engineering,previous studies (e.g. ITA, 1982; AFTES, 1997; U.S. Army Corps of Engineers, 1997; Kolymbas, 2005) took the β value of unity into consideration.However,in this study,the β value of 1 is adopted for tunnel crown and the value of 2 for the tunnel sidewall and inverted arch.

The presence of shear springs is also taken into account in the analysis.Since it is difficult to evaluate the stiffness of shear springs,a simple way,ηs= ηn/3,is adopted(Mashino and Ishimura,2005;Plizzari and Tiberti, 2006; Arnau and Molins, 2011; Barpi et al.,2011).

The maximum normal reaction pressure Pn，limcould be calculated as follows:

where c and φ are the soil cohesion and internal friction angle,respectively.

Similarly, the maximum shear reaction pressure Ps，limis estimated by the following expression:

The stiffnesses kn，iand ks，iof the ith spring can be obtained as follows:

Fig. 3. Relationship between reaction pressure P and support deformation δ.

where Liand Li+1are the lengths of elements i and i+1,respectively.

Note that the normal springs would only be active when the structure moves towards the soil, which means that only the compressive loads can be allowed in the normal direction. In contrast to the normal springs,both compressive and tensile loads are possible in the shear direction.

2.2. Active loads

The HRM requires that the active loads acting on the lining structure need to be defined.Active loads consist of the pore water pressure uf(Fig.4),soil mass gravity and additional loads induced by the surcharge loading. The surface load is assumed to be uniform, and analysis is done under plane-strain conditions. Considering the apparent soil unit weight γs, the pore water pressure uf,the surcharge loading P0, and the active vertical stress σvcan be obtained by Terzaghi’s formula (Working Group No.2, ITA, 2000):

where h denotes the overburden of a point on the tunnel lining;and Kzis the coefficient of the vertical additional load induced by surface pressure (Li et al.,2013), which is equal to

The horizontal stress σhin the HRM depends on the lateral earth pressure coefficient K0and the coefficient of the horizontal additional load (Kx) induced by the surcharge loading P0. σhcan therefore be determined as σh= K0γsh+ uf+ KxP0, where Kxis calculated as follows:

Fig. 4. Pore water pressure limit conditions.

Fig. 5. Cross-section of the numerical model and soft rock parameters.

In addition, the pore water pressure ufat the soil-structure interface should be calculated. When the tunnel lining is assumed to be impermeable,the lining has to support the full load caused by water.With respect to the pore water pressure uf,a simple way is introduced to calculate it (Bishop, 1954, 1960), i.e. uf= γwHw,where γwis the unit weight of water and Hwdenotes the distance from the groundwater level to the tunnel crown (Fig. 5).

3. Validation of the hyperstatic reaction method

To assess the accuracy of the present method,comparison of the results obtained from the numerical simulation and the HRM is presented in this section.

3.1. Design model of tunnel

Numerical simulation is performed using the fast Lagrangian analysis of continua(FLAC)software(Itasca,2012).Parameters from the Toulon tunnel in France are adopted.The Toulon tunnel allows the Toulon City to be crossed from east to west. It connects the motorways A50 and A57 from Nice to Marseille in France. The tunnel is an urban shallow tunnel with the length of 1820 m. The excavation of the tunnel started in 1993 and finished in 2002. The tunnel profile and soft rock properties from the Toulon tunnel are shown in Fig. 5 and Table 1, respectively.

As it is assumed to be a symmetrical problem, only half of the model is set up. Fig. 5 shows the two-dimensional (2D) numerical model under plane-strain conditions. The model dimensions are 10.74 m by 12.04 m. A surcharge loading P0= 0.25 MPa with a width Ldof 10 m is assumed to be applied on the soil surface. The numerical model is 120 m in x-direction,and 100 m in z-direction.This size permits to avoid boundary effects.The soil is considered as fully saturated. For the boundary conditions, the horizontal (x)displacements of the nodes in the x=0 m and x=120 m planes are fixed,while the vertical(z)displacements of the nodes at the base of the model are fixed. An impermeable lining (liner elements in FLAC) is considered in the numerical model. Along the tunnel boundary, the liner elements are attached to the soil zones. The numerical model consists of 3535 elements with a total of 7288 nodes. The dimension of the elements increases with the distance from the tunnel centre. The tunnel lining and soil geomechanical properties used in this numerical analysis are presented in Fig. 5 and Table 1, respectively. In the numerical model, it is assumed that the soft rock behaviour is governed by a linear elastic-perfectly plastic constitutive model (Mohr-Coulomb shear failure criterion).Fig. 6 illustrates the tunnel geometry considered in this study.

In this paper, the tunnel is excavated in a soft rock. The HRM could also be used to analyse the lining behaviour of the tunnel excavated in harder rock masses if their behaviour is not highly fractured.

The procedure of simulation in FLAC is illustrated as follows:

The intensive studies of objects in this meteorite have had a tremendous influence on current thinking about processes, timing, and chemistry in the primitive solar nebula and small planetary bodies, says coauthor George Rossman, the Eleanor and John R. McMillan Professor of Mineralogy at Caltech.

(1) Setup of the numerical model,such as generating grid model,setting up boundary conditions,defining material properties,setting up initial conditions,and balancing the initial state of stresses;and

(2) Tunnel excavation and liner installation,and then numerical calculations to reach the new equilibrium state.

3.2. Validation of the present method

Fig. 7 shows the mesh of the numerical model and the soil vertical displacement that occurs around the tunnel after excavation. Fig. 8 illustrates the internal forces and displacements of the tunnel lining obtained from the numerical model and the HRM.From Fig.8,it can be found that the normal forces of the HRM show a good agreement with those of the numerical model. The maximum absolute discrepancy(13%)for the normal forces occurs at the centre of the tunnel crown. Fig. 8b also shows a good agreement of the bending moments between the HRM and the numerical model. The maximum absolute discrepancy (7.2%) in terms of bending moments occurs at the connection of sidewall and inverted arch. In addition, noticeable differences in the radial displacements are observed at the tunnel crown.This could be caused by the arching effect of the tunnel lining developed in the numerical model, which is not considered in the HRM. In addition, the loading condition applied on the lining is different between both methods.The external loads in the HRM act explicitly and directly on the beam elements. The external loads in the FLAC model are instead applied on the tunnel support through the continuous soil.The values of the radial displacements obtained from the HRM are slightly higher than the numerical ones at the tunnel sidewall and inverted arch.The maximum discrepancy(31%)occurs at the centre of the tunnel crown.

According to the above-mentioned analysis, the lining displacements and internal forces obtained from the HRM are in a good agreement with the numerical ones.Therefore,the HRM can be effectively used to estimate the displacements and structural forces of lining.In the following section,by means of the HRM,the effects of the water pressure, the surcharge loading value and width,and the groundwater level are presented.

4. Parametric analysis

Fig. 9 presents the pore water pressure effect on the internal forces and radial displacements of the horseshoe-shaped tunnel support.Without considering the pore water pressure,the absolute values of the internal forces and radial displacements obtained from the HRM are always lower than the ones when considering the pore water pressure. The peak of the normal force when considering the pore water pressure occurs near the connection of the tunnel crown and sidewall,i.e.θ = 70°.This maximum normal force is approximately 57%larger than the one without considering the pore water pressure. The pore water pressure impact on the bending moments at the tunnel sidewall is significant;however,its influence on the bending moments at the tunnel crown could beneglected.A maximum bending moment of 2.45 MN m/m occurs at the corner of the lining(θ = 32°)when considering the pore water pressure, which is about 76% larger than the one without considering the pore water pressure.In contrast,the influence of the pore water pressure on the radial displacement along the whole tunnel,except at the tunnel crown centre,is significant,as shown in Fig.8c.The maximum radial displacement of 0.057 m occurs near the spring line.Fig.10 shows the impact of the surcharge loading on the internal forces and radial displacement of the horseshoe-shaped tunnel lining considering the pore water pressure. One can find that the surcharge loading has a great influence on the radial displacements and normal forces at the tunnel crown and sidewall.However, the effect of the surcharge loading on the bending moment could be neglected. No matter what the value of the surcharge loading is, the maximum normal force always occurs near the connection of the tunnel crown and sidewall. In contrast, the maximum radial displacement is observed at the tunnel spring line.

Table 1 Tunnel lining parameters.

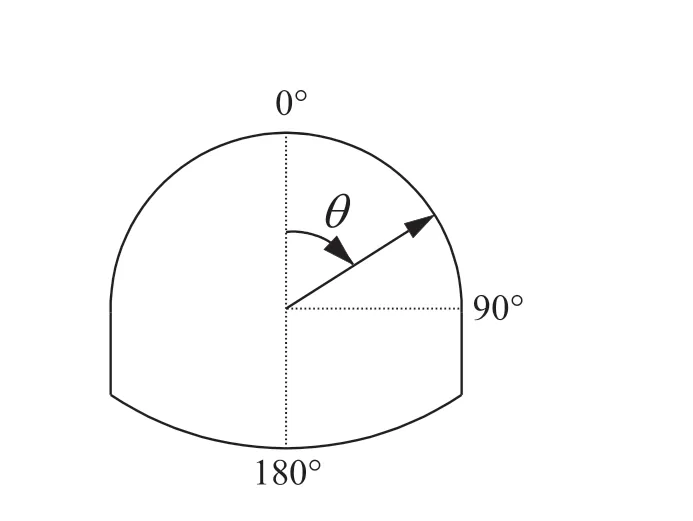
Fig. 6. Geometry of the studied tunnel.

Fig. 11 displays the effect of the surcharge loading on the internal forces and radial displacement without considering the pore water pressure.It can be clearly observed that the surcharge loading has a great influence on the normal force at the tunnel crown and sidewall. Although the differences in the bending moment appear to be small, significant changes in the normal force and radial displacement are induced at the tunnel crown and sidewall.The normal forces at the tunnel crown and sidewall increase significantly as the increase of the surcharge loading.The impact of the surcharge loading on the normal force at the tunnel inverted arch could be neglected.However,the increase of the surcharge loading leads to an increase of the radial displacement at the tunnel crown and inverted arch, but a decrease of the radial displacement at the tunnel sidewall.Unlike the results of Fig.10c, the maximum radial displacement shown in Fig. 11c occurs at the tunnel inverted arch rather than at the tunnel sidewall.

Fig. 7. Numerical model in FLAC.

Fig. 8. Comparison of internal forces and displacement obtained from the numerical model and the HRM.

Fig. 9. Behaviour of the tunnel lining with and without considering the pore water pressure.

Fig.10. Behaviour of tunnel lining considering both the surcharge loading P0 and the pore water pressure.

Fig.11. Behaviour of tunnel lining only considering the surcharge loading.

Fig.12. Effect of the surcharge loading width on the lining behaviour without considering the pore water pressure.

Fig.13. Effect of groundwater level on tunnel behaviour.

Comparing Figs.10 and 11, the effects of the surcharge loading on the normal force and displacement are significantly different.When considering the pore water pressure, the absolute values of internal forces and radial displacement are always greater than those without considering the pore water pressure. This is consistent with the numerical analysis results of Shin et al. (2002), who found that the magnitudes of lining internal forces increased as the permeability of the drainage layer was reduced.

Fig. 12 reveals the influence of applied surcharge loading width Ldon the tunnel behaviour. While fixing the surcharge loading at P0= 0.25 MPa, the surcharge loading width Ldof the half model is varied from 10 m to 40 m. Fig.12 indicates that the surcharge loading width has a great influence on the lining normal force. As the surcharge loading width becomes greater,the normal force increases. The bending moment is not affected by the surcharge loading width changes as compared to the radial displacement.

Fig.13 presents the effects of the groundwater level on the internal forces and radial displacement of the horseshoe-shaped tunnel under conditions of Hw/H = 0-0.6 and P0= 0.25 MPa. As expected,the Hw/H ratio significantly influences the internal forces and radial displacement of the tunnel lining. It can be observed from Fig.13 that the normal force increases with the increase of the Hw/H ratio along the whole tunnel.Although the effect of the Hw/H ratio on the bending moment at the tunnel sidewall is obvious,this effect at the tunnel crown and inverted arch is not significant.The absolute values of bending moment increase with the increase of the Hw/H ratio at the tunnel sidewall.A significant influence of the Hw/H ratio on the radial displacement can also be found near the connection of the tunnel crown and sidewall. The radial displacement increases as the Hw/H ratio increases.

5. Conclusions

In this paper, the HRM is presented to calculate the internal forces and displacements of tunnel lining considering the effects of surface loading and pore water pressure.Comparison between the results obtained from the HRM and numerical simulation is conducted. Although some discrepancies remain between the results from these two methods,the differences are admissible for an engineering design,which has permitted to validate the HRM.Then, the effects of the surcharge loading value and width, the pore water pressure, and the groundwater level on the tunnel behaviour are investigated. Some conclusions are drawn as follows:

(1) The surcharge loading has a significant impact on the lining normal forces when the tunnel buried-depth is approximately four times its height. However, the effect of the surcharge loading on the lining bending moment is negligible.

(2) The surcharge loading width has a considerable effect on the lining normal forces for the considered depth. As the surcharge loading width becomes greater, the lining normal force values increase.However,the lining bending moments and radial displacements are not sensitive to the surcharge loading width changes.

(3) The groundwater level greatly influences the displacements and internal forces of lining. The lining normal forces along the whole tunnel decrease with the increase of the groundwater level. The impact of the groundwater level on the lining bending moments is only observed at the tunnel sidewall.

In conclusion, compared to the bending moments, the normal forces and radial displacements of tunnel lining are more sensitive to the water pressure, surcharge loading value and its width, and groundwater level when the buried-depth of tunnel is approximately four times its height.

Declaration of competing interest

The authors confirm that there are no known conflicts of interest associated with this publication, and there has been no significant financial support for this work that could have influenced its outcome.

Acknowledgments

The first author is supported by the Fundamental Research Funds for the Central Universities in China. The third author is supported by the Vietnam Ministry of Education and Training under grant number B2020-MDA-15. These funds are greatly appreciated.