Ultrasonic imaging of microscale processes in quartz gouge during compression and shearing

Amin Gheibi, Ahmadreza Hedayat

Colorado School of Mines, Golden, USA

Keywords:Granular material Ultrasonic investigation Particle comminution Compaction and dilation

A B S T R A C T Active ultrasonic monitoring in rock joints and gouge materials has the potential to detect the signatures of shear failure for a wide range of sliding modes, from slow and stable movements to fast and unstable sliding. While these collected measurements currently are being used to identify the seismic precursors to shear failure in rock joints and gouge materials, the underlying physical processes and contact scale mechanisms that control the changes in ultrasonic wave attributes are still poorly understood.To address this knowledge gap, this paper aims to investigate the relationship between the variations in ultrasonic wave attributes and the underlying particle scale mechanisms during both compression and shearing.Our double direct shear experiments were coupled with ultrasonic wave propagation measurements on granular quartz gouges, in which the gouge layers were sheared under different sliding velocities and constant normal stress conditions. Simultaneously, ultrasonic waveforms were continuously recorded during shearing with a fast data acquisition system and three pairs of ultrasonic wave transducers embedded at the two sides of the gouge layers. Different particle comminution mechanisms were observed from the non-uniform distribution of normal and shear stresses through the changes in ultrasonic transmissivity and scanning electron microscope (SEM) images. Our results show that the signatures of the geometry- and time-dependent variations of the inter-particle contact quality and pore volume changes with sliding velocity and slip accumulation were clearly captured from the variations in the transmitted wave amplitude and the dominant frequency, respectively. In addition, we found that variations in dominant frequency corresponded to dilation and compaction of the granular gouge layer during compression as well as stable and unstable sliding. Our results therefore confirmed that nondestructive acoustic techniques are capable of capturing a variety of micromechanical processes during fault gouge deformation and may prove useful in natural settings.

1. Introduction

The widespread devastating impacts of earthquakes on society and human life have motivated many researchers to focus on the mechanisms of the origin of fault movements in order to better understand earthquake nucleation. Tectonic faults are generally filled with a thin layer of gouge material, which has an important role in sliding stability and accommodating shear displacement(e.g.Anthony and Marone,2005;Leeman et al.,2016;Scuderi et al.,2016).Fault motion can occur in a spectrum of sliding modes:from creeping at very small sliding velocities(10-4m/a)to fast dynamic slip observed during high magnitude earthquakes(1 m/s)(e.g.Peng and Gomberg,2010;Beroza and Ide,2011;Kato et al.,2012;Scuderi et al., 2016). Both stable and unstable sliding scenarios have been observed to occur on the same fault patch(Kato et al.,2012).Hence,understanding and monitoring the sliding mechanisms involved in the transition from stable to unstable is of primary importance.This transition has been investigated through both experimental and analytical studies which have identified sliding velocity and changes in gouge fabric as the main factors affecting the sliding stability (e.g. Ampuero and Rubin, 2008; Kaproth and Marone,2014; Scuderi et al., 2017).

The frictional stability in granular materials has been interpreted through either the concept of the interaction between the rheological fault critical stiffness and surrounding elastic stiffness(Rice and Ruina,1983;Gu et al.,1984)or the theory of force chains and the micro-mechanisms between the particles (Rechenmacher et al., 2010; Tordesillas et al., 2011). In the context of rate and state friction constitutive equations (e.g. Marone,1998), it is well established that the sliding mode is controlled by the ratio of elastic loading stiffness (k) to the rheological critical stiffness (kc). In this context,the system may become unstable for critical stiffness ratio,κ,less than unity(κ=k/kc<1)(Gu et al.,1984).On the other hand,in the context of the theory of force chains and inter-particle micromechanisms,the frictional behavior is related to the occurrence of a variety of time- and slip-dependent physical and chemical processes with slip accumulation and changes in the boundary stress conditions. These processes (e.g. particle comminution, thermal activation,and variation of contact point properties) play a critical role in the theory of force chains and the micro-mechanisms between the particles, in which the stability and the macro-scale gouge frictional behavior are positively related to the evolution of the weakening or strengthening mechanisms at the particle contacts (Rice and Ruina, 1983; Shimamoto, 1986; Bar-Sinai et al.,2014). In fact, the crossover between velocity weakening friction and velocity strengthening is affected by several factors including the competition between two mechanisms at the grain contacts:(i)variation of the particle to particle shear strength (σs) and (ii)changes in the state of the inter-particle contact points (Heslot et al., 1994; Baumberger et al., 1999; Bureau et al., 2002;Baumberger and Caroli, 2006; Bar-Sinai et al., 2014). The contact shear strength (σs) increases logarithmically with sliding velocity according to the thermal activation model, which assumes an energy barrier resisting slippage between the contact points (Bar-Sinai et al., 2014). The state of inter-particle contact points in granular materials is significantly influenced by the particle comminution processes.These processes(e.g.abrasion and particle splitting) during compression and shearing can change the shape(Heilbronner and Keulen, 2006; Storti et al., 2007), particle size distribution (Rawling and Goodwin, 2003; Keulen et al., 2007;Sammis and King,2007),and force chain arrangement,and thereby significantly affect the macro-scale frictional behavior of the granular gouge layer.

Geophysical methods,such as ultrasonic wave monitoring,have proven to be successful for remotely detecting and illuminating the micro-mechanisms occurring within the gouge layer during compression and frictional sliding (Erickson and Jarrard, 1998;Fortin et al.,2007;Lee et al.,2010;Knuth et al.,2013;Kaproth and Maorne,2014).The ultrasonic waves transmit through the contact points between the particles;therefore,the amount of transmitted ultrasonic energy is dependent on the area and quality of interparticle contacts as well as the volume and distribution of the inter-particle voids (i.e. porosity) (Nagata et al., 2008, 2012, 2014;Knuth et al., 2013; Dehghani et al., 2020). In rock joints, the transmitted amplitude has been considered a reliable proxy for the evolution of the real contact area in the stable sliding mode(Shreedharan et al., 2019). However, the amplitude variations are not clearly understood in granular materials with irreversible deformation mechanisms and porosity variations due to high external stresses.

Porosity and particle shapes were originally considered as the parameters controlling the dilative or contractive behavior of the gouge layers during both stable sliding and stick-slip cycles(Marone et al.,1990;Scuderi et al.,2015).The volume and porosity changes in the granular gouge layer result from the rearrangement or collapse of force chains. These changes in pore volume could affect the gouge structure and subsequently the ultrasonic measurements (Schubnel et al., 2003; Fortin et al., 2005; Croize et al.,2010). In rock materials, several studies focused on the propagation of ultrasonic waves through rock fractures (joints) and observed that wave propagation, especially the changes in the dominant frequency of transmitted waves, was highly affected by void and fracture properties which include opening distribution and asperity stiffness (Pyrak-Nolte et al., 1990; Pyrak-Nolte and Nolte, 1992; Resende, 2010). However, more complicated mechanisms are involved in the compression and shear deformation of granular materials that can affect ultrasonic wave propagation.While the majority of experimental studies conducted in the past years focused on documenting wave velocity variation with stiffness evolution in granular materials (Mousavi and Ghayoomi,2018), less attention was paid to variations of wave frequency and amplitude with gouge fabric evolution and particle scale mechanisms.

In this paper, we investigated the correlation between the micromechanical deformation and ultrasonic attributes in the granular quartz gouge layers during compression and shearing.We utilized a double direct shear device and a fast ultrasonic wave propagation system to monitor, remotely and non-destructively,the local changes in the gouge layers at different locations. Three different ultrasonic transducers were utilized in this setup to evaluate the impact of non-uniform distribution of normal and shear stresses on the gouge fabric evolution.The gouge layers were sheared under different values of normal stresses and slip velocities in order to explore variation in the ultrasonic wave attributes for a wide range of sliding modes.We identified different comminution mechanisms along the fault surface that were promoting different acoustic transmissivity and found that the amplitude and frequency of the transmitted ultrasonic waves can provide valuable insights into the deformation processes in the granular soil layers.

2. Experimental methods

2.1. Testing material and specimen preparation

Double direct shear experiments were performed on gouge layers composed of granular quartz material known as Ottawa sand,which was acquired from US Silica Company.Ottawa sand F75 was selected because it was widely used in previous laboratory studies(e.g.Mair et al.,2002;Hong and Marone,2005;Ikari et al.,2007;Gheibi and Hedayat,2018).The F75 sand initially included a wide range of grain sizes, which were sieved according to ASTM C136/C136M-19 (2019) in order to obtain uniform grain distribution with a mean grain size of 0.58 mm. This uniform particle size distribution was beneficial in our study to better evaluate the particle scale mechanisms and subsequent ultrasonic changes.The particles were considered sub-angular according to our scanning electron microscope(SEM)images(Gheibi and Hedayat,2018).The particle size distribution curve and the SEM images of the quartz particles used in our study are shown in Fig. 1, and the general properties are summarized in Table 1.

Specimens were prepared using oven-dried quartz particles mixed with de-aired water to achieve the water content of 10%which was selected experimentally to obtain uniform and homogenous specimens with repeatable mechanical and ultrasonic behaviors. To produce specimens with uniform moisture content,the moist material was kept in a sealed plastic bag for 24 h.Thereafter, 6 mm thick gouge layers were prepared using two 10 cm × 10 cm (i.e. nominal contact area) stainless steel forcing blocks.A leveling lab-jack and four plastic bars were used to control the specimen height and construct the specimen with the desired initial porosity (n) of 38%, given the volume of void space and the total volume of the gouge layers.

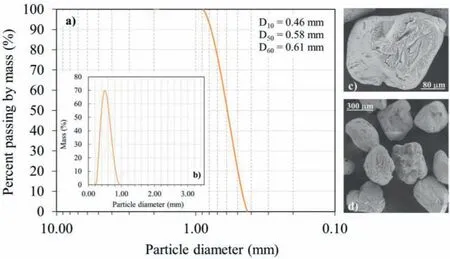
Fig.1. The Ottawa sand used in this study:(a,b)Particle size distribution data in logarithmic and linear axes;and(c,d)SEM images of particles illustrating the particle shape before the experiments.

The surfaces of the forcing blocks were grooved in a triangular pattern 0.5 mm deep with 1.5 mm spacing to minimize particle slippage at the boundaries and to localize shearing within the gouge layer.Cellophane tape was applied around the forcing blocks to prevent loss of material and moisture after the specimen was prepared.In addition to the cellophane tape,two stiff plastic plates were screwed to the sides of the forcing blocks to prevent any side extrusion of material during shearing, which was utilized successfully in similar studies (e.g. Knuth and Marone, 2007; Leeman et al., 2015).

2.2. Double direct shear device

The experimental setup consisted of a horizontal loading frame that applied the normal stress, an assembly of transducer holder plates to place the ultrasonic transducers, a fast data acquisition system to record shear waveforms, and an automated vertical loading piston that applied forces and induced shear displacement within the gouge layers (acquisition frequency of 100 Hz). Normal stress was applied on the specimen using a flat jack connected to an automated servo-controlled hydraulic pump that was capable of applying normal stress up to 30 MPa. The schematic configuration of the double direct shear device and the ultrasonic transducers are shown in Fig. 2.

2.3. Ultrasonic measurement system

A fast ultrasonic wave propagation system was used to monitor the gouge layers during application of compressive and shear loadings. In this setup, two arrays, each including three transducers, were used to record the shear waveforms. A 12.7 mm cylindrical contact transducers (Olympus V103) with a central frequency of 1 MHz was used. An Olympus pulser-receiver (Panametric 5077PR) was used to generate signals with amplitude of 300 V at a repetition rate of 5 kHz. The waveforms were digitized with a National Instruments digitizer with a sampling rate of 100 million samples per second.To reduce the noise effect and increase the signal-to-noise ratio,20 signals were averaged and stacked for each transducer recording. All averaged and stacked transmitted waveforms for all the transducers were recorded every 0.5 s with a fast LabVIEW data acquisition system.

The location of the ultrasonic transducers and their labels are shown in Fig.2c.The transducers were placed in a diagonal pattern with the source and receiver transducers located, and thus they were exact mirror images of each other.The diagonal arrangement of transducers would enable one to extrapolate the localized ultrasonic data to other areas of the gouge layer, given the symmetrical distribution of normal stress on the gouge layer. The accuracy and resolution of ultrasonic measurements are known to be dependent on the acoustic properties of the material through which the waves are traveling, such as the gouge layer and the forcing blocks as well as the coupling material.A thin layer of ovenbaked honey at 90°was used as the coupling material between the surface of the transducers and the forcing blocks to improve transmission of the ultrasonic waves (Hedayat, 2013; Gheibi and Hedayat, 2018, 2019; Shirole et al., 2019). The other factor influencing the resolution of recorded waveforms is known to be the contact stress between the transducers and forcing blocks.To keep the contact stress constant for all the transducers throughout the test, a spring washer and a number of stainless steel shims were embedded under each transducer in a way that the final surface of all transducers was 2 mm higher than the final surface of the aluminum cover plate (Fig. 2b). The washer acted as a spring and became flat under the preloaded stress, which ensured that the transducer surface was at the same height as the aluminum cover plate.

2.4. Wave analysis method

We used the same ultrasonic monitoring technique throughout different stages in the experiments to investigate the link between the changes in the ultrasonic wave attributes and the occurrence of inter-particle mechanisms. The waveforms were continuously recorded for three transducers during the initial compression stage as well as the shear deformation. Fig. 3 shows the typical waveforms recorded during compression and shearing at different values of normal stresses and shear displacements. For the transducers with this level of central frequency,it was expected that the wave amplitude would be very sensitive to the changes in the state of the contact between particles and inter-particle forces(Hedayat et al.,2018).The waveforms recorded during compression showed considerably more changes in peak-to-peak amplitude and frequency than the waveforms recorded during shearing due to the increase in normal stress as well as the changes in the gouge layer structure (Fig. 3).

The main focus of our study was to detect the variation of the transmitted amplitude and the dominant frequency of the shear waves traveling through the granular materials. The maximumpeak-to-peak amplitude was determined as the sum of the maximum and minimum values in the received waveform in the time domain. For dominant frequency determination, the time domain wavelet needed to be transferred into its frequency domain components. However, in granular materials such as quartz sand, only the portion of the waveform immediately following the arrival of the wave needed to be considered to evaluate the changes in dominant frequency (Mirchandani and Sharma, 2010). Thus, as illustrated in Fig. 4, we defined a window and selected the initial part of the wave. Then, we used the taper function with a half cosine shape (Pyrak-Nolte et al., 1990;Gheibi and Hedayat,2018;Shirole et al.,2020)to isolate the initial pulse from the subsequent variations. The selected window was moving for each waveform with respect to the wave arrival.Several iterations were conducted to obtain the right length for the taper function, in a way that neither the shape of the spectral amplitude nor the frequency contents of the waves were affected(Hedayat et al., 2018). Fig. 4 shows the selection of the window and taper function for the waveforms recorded at 15 MPa and 20 MPa normal stresses.

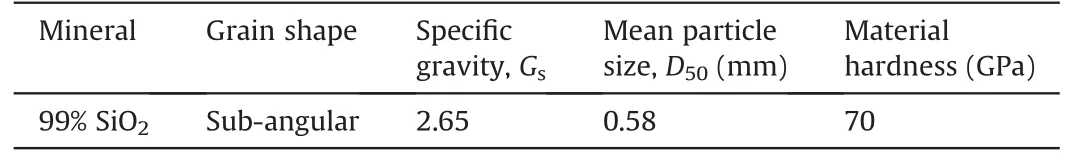
Table 1 General properties of the quartz sand particles(Dutta and Penumadu,2007;Gheibi and Hedayat, 2018).

We applied a 5.12 μs taper to the original waveform and then performed the fast Fourier transform (FFT) on the tapered waveforms. The FFT yielded the wave spectra for the range of frequencies, from which we selected the frequency corresponding to the peak spectral amplitude as the dominant frequency. The obtained wave spectra for the waveforms shown in Fig. 3 are presented in Fig. 5. The evolution of the maximum wave energy as well as the corresponding frequency is demonstrated with increases in the normal stress and shear accumulation. It is noteworthy to mention that the original waves were sent with a dominant frequency of 1 MHz, while it was decreased to 0.3-0.4 MHz for the received signals. More details of the methodology used for the determination of dominant frequency can be found in Gheibi and Hedayat (2018)and Hedayat et al. (2018).

Fig.3. Recorded shear waveforms during(a)the initial compression at different values of normal stress and (b) application of shear displacement at different values of shear displacement.

Fig. 2. Double direct shear setup used in this study: (a) Schematic side view of the double direct shear device with embedded ultrasonic transducers imaging the gouge layers sandwiched between the side and middle forcing blocks;(b)Schematic presentation of the details of the transducer,spring washer,and steel shims embedded in the transducers holder plates; and (c) Arrangement of the ultrasonic transducers in the transducer holder plate.

Fig. 4. Illustration of using moving windows and taper function to isolate the appropriate section of the waveforms for dominant frequency determination.

2.5. Testing procedure

We performed two types of double direct shear experiments to investigate the frictional micro-mechanisms in granular quartz gouge in different modes of sliding.Ultrasonic measurements were carried out during all the experiments to explore the link between the ultrasonic wave attributes and the frictional mechanisms. The first set of experiments included double direct shear tests on the granular quartz layers under constant normal stress and constant load point velocity to study the occurrence of particle scale mechanisms during unstable sliding with different sliding velocities. The second set of experiments involved a series of velocity step tests on granular quartz layers using the double direct shear device at four different normal stresses of 15 MPa,20 MPa,25 MPa and 30 MPa (Table 2). Load point velocities were intentionally varied to explore the changes in frictional behavior and ultrasonic attributes due to velocity variations.

All the experiments were initiated by applying the desired level of normal stress, which was kept constant throughout the test. At the compression stage, the normal stress was increased at a constant rate of 0.2 MPa/s as previously practiced by McDowell and Humphreys (2002) and Knuth et al. (2013). Following the compression stage, the shear load was applied at a constant displacement rate on the middle steel plate in the double direct shear setup while the other two sides of the specimen were supported and fixed by the steel spacers, as shown in Fig. 2a. For thesecond set of experiments, the specimens were sheared first for about 4 mm at a sliding velocity of 5 μm/s to pass the initial strengthening and then to reach a steady state frictional sliding regime.Once steady state sliding was achieved,a series of velocity step tests was then performed.

Table 2 Summary of experiments conducted in this study.

After each experiment, using the SEM and sieve analysis, the material under each ultrasonic transducer was carefully collected so as to observe and quantify the amount of particle comminution and the developed structure. All the experiments were conducted at room temperature and humidity.Each experiment was repeated at least twice to assure that repeatable results were obtained.Table 2 lists the experiments conducted in this study.

3. Results

The macroscale compression and shear deformations in granular assemblies are the result of grain movement at the particle scale, which are accompanied with frequent cycles of formation and breakage of the contact networks and force chains(Salman and Gorham,2000;Hartley and Behriger,2002).Force chains have been investigated through several experimental and numerical studies,which support the notion that most of the external loads are carried through the network of multiple and non-homogenous distributed force chains (Oda and Kazama, 1998; Iwashita and Oda, 2000;Rechenmacher, 2006). The inter-particle contact area, force, and stiffness within force chains may change significantly due to changes in the boundary stresses or the structure of the force chains. Evolution in characteristics of the inter-particle contact points, particularly the contact stiffness and contact area, may affect the transmission of ultrasonic waves. Hence, these methods have been considered as successful experimental approaches for evaluation of the inter-particle contact properties in granular materials. In the following sections, the results of ultrasonic measurements during compression and shearing of granular quartz sand are discussed in detail.

Fig. 5. Evolution of spectral amplitude for the waveforms at different levels of (a) normal stress and (b) shear displacement.

3.1. Compression

During compression,the normal stress applied on the specimen increased linearly from the preloaded value to the desired normal stress defined for each experiment at the rate of 0.2 MPa/s.Simultaneously, the ultrasonic waveforms traveling through the materials were recorded at a frequency of 1 Hz. Our results from experiment VS-30 are shown in Fig.6.At the target normal stress of 30 MPa,the gouge layer thickness decreased from 12 mm to 8 mm.It was observed that the normal deformation in the gouge layer did not change linearly with evolution of the normal stress.Instead,the gouge layers experienced higher values of normal deformation at the early stage of loading and then gradually decreased with the evolution of normal stress,indicating a gradual increase in normal stiffness with increase in normal stress. This observation is consistent with the findings of Nakata et al. (2001) and Mesri and Vardhanabhuti (2009). During compression, the peak-to-peak amplitude and dominant frequency increased with normal stress;however,the amount of evolution was not equal for the transducers imaging different locations,as shown in Fig.6.It was observed that the absolute values, as well as the amount of evolution in the transmitted amplitude and dominant frequency, increased from transducers 1S to 3S, indicating the possibility of more stress concentration and compaction in the central area. The values of the peak-to-peak amplitude obtained at normal stresses of 30 MPa versus 5 MPa were 27,35,and 45 times greater for transducers 1S,2S, and 3S, respectively, while the amount of evolution for dominant frequency was much less than the transmitted amplitude and the values were 1.4, 1.6 and 1.8 for the three transducers,respectively.

As observed in Fig. 6, for the granular quartz material and the range of stresses applied in this study, the transmitted amplitude did not show a linear relation with the normal stress.However,the measurements reported for rock and plastic materials(Nagata et al.,2014;Shreedharan et al.,2019)showed almost a linear correlation between amplitude and normal stress which could be due to either the shorter range of normal stresses applied in those studies or the different mechanisms involved. We observed that the transmitted amplitude evolved slightly at the early stage of compression and then gradually increased at a higher rate. In fact, the transmitted amplitude showed a bilinear trend with a middle transitional area with increases in the slope of the curve.The transmitted amplitude is known to be related to the changes in the amount of real contact area,and the inter-particle contact force and stiffness(Kendall and Tabor,1971; Nagata et al., 2008, 2014). The changes in the rate of increase in the amplitude with the normal stress showed that the porosity of the gouge layer also influenced the rate of increase in addition to the normal stress effect. This can explain the influence of different mechanisms at different levels of normal stress (e.g.elastic deformation of particles, breakage and rearrangement of force chains, and particle comminution). The sensitivity of the transmitted amplitude to the normal stress was also observed at the normal stress of 30 MPa. When the normal stress became constant at 30 MPa,the rate of change in the transmitted amplitude significantly decreased, which was consistent with the normal stress variation, but no similar trends were observed for the dominant frequency values.

The trends observed for the evolution of dominant frequency were different from those for the transmitted amplitude. The dominant frequency increased significantly at the early stage of compression in contrast to the transmitted amplitude and then followed an increasing trend at a decreasing rate. The variation of dominant frequency followed a trend more similar to the deformation in the granular layer than to the normal stress.This was also observed as the normal stress became constant at 30 MPa, where no noticeable change was observed in the rate of dominant frequency evolution.

3.2. Shear experiments

In order to understand the evolution of the elastic properties of the granular quartz gouge material with shear displacement, double direct shear experiments were conducted under different normal stresses and sliding velocities. During the direct shear experiments, we continuously monitored the variations of shear stress and layer thickness due to shear displacement as well as the waveforms transmitting through the gouge layer.The evolutions of shear stress and shear displacement with time are shown in Fig.7a.Shear displacement of a few millimeters was enough to pass the initial strengthening and reach the steady state condition. During initial sliding, the shear stress was stable and increased significantly with slippage. However, the rate of increase in shear stress decreased and small stick-slip events gradually appeared for a limited number of cycles as the shear slippage continued. The small stick-slip events gradually grew with shear displacement and turned to repeatable unstable stick-slip events as similarly observed in Leeman et al. (2018). Gouge layer thickness decreased continuously with slippage during both the initial stable sliding and the later unstable sliding.

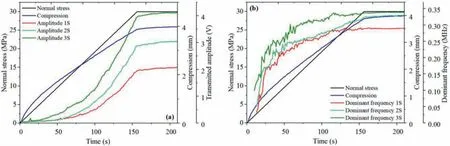
Fig. 6. Data showing the gouge layer compression and evolution of ultrasonic wave attributes with application of the normal stress up to 30 MPa during the initial compression stage: Variations of (a) transmitted peak-to-peak amplitude and (b) dominant frequency with normal stress for three ultrasonic transducers. Determination of the dominant frequency was not possible for waveforms collected at low normal stress values (<2 MPa).

Fig.7. Representative curves showing experimental data during shearing of the gouge layer under normal stress of 20 MPa and sliding velocity of 10 μm/s: (a) Shear stress and shear displacement evolutions, and (b) changes in gouge layer thickness and porosity. The porosity values were corrected in accordance with the geometrical thinning phenomena.

In the direct shear experiments, it was difficult to continuously measure porosity variations due to the geometrical thinning phenomenon and mass loss.Geometrical thinning due to mass loss evolves with increases in layer thickness and accumulation of shear displacement in direct shear experiments.The traditional method to evaluate geometrical thinning assumes a triangular shape for the mass loss;however, Kaproth and Marone (2014) found that assuming a rectangularshapewouldbetterestimatetheamountofextrudedmaterial.We utilized the equation below as the suggested method by Kaproth and Marone(2014)to evaluate the geometrical thinning in our study:

where h is the layer thickness,δx is the shear displacement increment,L isthespecimenlength,andδhistheincrementalthickness.Theporosity values were corrected with respect to layer thickness and sliding velocity using Eq.(1).The applied correction reduced the porosity variations with the evolution of shear displacement.The measured values of layer thickness and corrected porosity are shown in Fig.7b.

Fig. 8. Corresponding ultrasonic wave attributes obtained from three ultrasonic transducers during the experiment M20-10 shown in Fig. 7: (a) Variation of transmitted amplitude, and (b) variation of dominant frequency.

The results of our ultrasonic measurements from representative double direct shear experiment(M20-10)on granular quartz gouge are shown in Fig. 8. The changes in transmitted amplitude and dominant frequency recorded by the three transducers are presented.It can be seen clearly from the figure that the data obtained from the transducers imaging different locations followed different trends, indicating differences in the type, sequence, and level of intensity of the frictional particle scale mechanisms.

We observed that the absolute values of the transmitted amplitude and dominant frequency were higher for the transducers located closer to the center.Based on the results observed in the previous section and also in similar studies(Nagata et al.,2014;Hedayat et al., 2018), the transmission of ultrasonic energy(amplitude) was highly dependent on the boundary stresses or inter-particle forces and the state of inter-particle contact. Therefore, the systematic additional increases in the ultrasonic transmissivity of the central areas show higher values of inter-particle stresses and possible formation of a more compacted and tighter structure in the center (under transducer 3S).

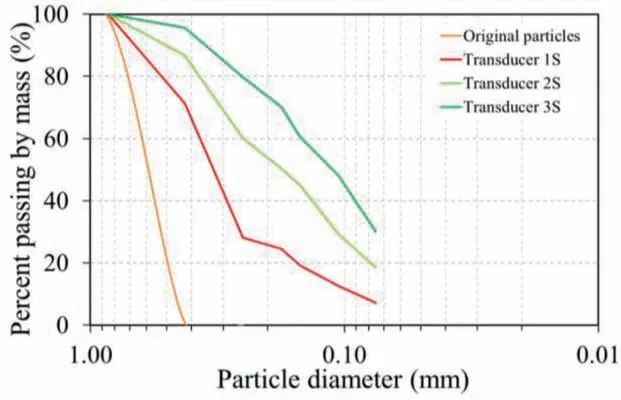
The transmitted amplitude obtained from transducer 1S increased by about 20%during the initial loading,up to the steady state condition (t = 400 s), but beyond this point, it decreased slightly to a residual value, which remained almost constant with further application of shear displacement. This observation was in contrast to the results reported by Kaproth and Marone (2014).However,the initial decreasing trend(0 s

The dominant frequency values for transducer 1S slowly and continuously increased by about 30% throughout the experiment.Similar to the variation of amplitude, the dominant frequency variations for transducers 2S and 3S were not identical to that for transducer 1S. For these transducers, in the initial part of loading(t < 200 s), the dominant frequency values showed a decreasing trend and then gradually increased.The total amount of increase in the dominant frequency for transducers 2S and 3S was 8%and 13%,respectively, less than that for transducer 1S.

The higher ultrasonic transmissivity in the central area can be seen in Fig. 8, indicating that during compression, a denser and tighter localized structure was developed in the center which favored the transmission of ultrasonic waves with higher frequency and energy. The developed structure and contact networks may have been disturbed due to the applied initial shear displacement.This disturbance could have occurred in the form of particle sliding and rolling, which led to particle rearrangement within the force chains. Dilation and compaction of granular materials have been extensively studied under different stress conditions, with the observation that in dense and compacted structures, the movements of particles and subsequent rearrangement in force chains can be accompanied by localized dilation and increases in interparticle pore volume; while in loose structures, it may lead to further layer compaction (Bolton,1986; Wood,1991). However, in double direct shear tests, depending on the normal stress, level of densification, sliding velocity, and size of the specimen, the localized dilative mechanisms may or may not be represented in the global specimen behavior as contrastive observations have been reported in the literature (Samuelson et al., 2009; Kaproth and Marone, 2014). In our experiments, all the specimens showed continuous contractive behavior during initial shear.However,this does not necessarily imply that the compaction was occurring in all the areas. In fact, the decreases in the amplitude and dominant frequency of transducers 2S and 3S could be an indication of localized dilation in a highly densified central area.However,since the dilation was not observed in the global behavior of the specimen, there is a possibility that dilation occurred laterally in the gouge layer plane perpendicular to the direction of normal stress.

The results obtained from experiments VS-25 and M20-10 are respectively presented in Figs.9 and 10 as representative results for better understanding the variations in ultrasonic wave attributes in response to velocity steps and stick-slips. The gouge material showed stable sliding when sheared with a velocity of 1 μm/s. In the stable sliding regime, the displacement rate in the gouge layer and the load point velocity were constant and equal; but during unstable sliding, the sliding velocity at the interface was not constant and fluctuated between lower and higher values than the load point velocity (as similarly reported in Brace and Byerlee (1966)).Fig. 9a presents the data of shear stress, amplitude, and sliding velocity obtained for a velocity step from 5 μm/s to 1 μm/s.During stable sliding, the gouge layer response to a velocity perturbation first resulted in a drop in friction, followed by a gradual decline to the steady state with slip accumulation.The transmitted amplitude for all three transducers increased suddenly with reduction in sliding velocity.After this point(t=562 s),the sliding velocity and,subsequently the transmitted amplitude, were almost constant while the shear stress was experiencing decreasing and increasing trends due to the velocity step.

Fig.9. Variations of shear stress,sliding velocity, and transmitted amplitude for three ultrasonic transducers during(a)velocity step from 5 μm/s to 1 μm/s and(b)stick-slip cycles at sliding velocity of 10 μm/s. The dashed line in Fig. 9a shows the time of change in the sliding velocity.

Fig.10. Variations of shear stress,layer porosity and dominant frequency for three ultrasonic transducers during(a)velocity step from 5 μm/s to 1 μm/s and(b)stick-slip cycles at sliding velocity of 10μm/s.The dashed line in Fig.10a shows the time of change in the sliding velocity.

The shear data for the gouge layer in the unstable sliding mode are shown in Fig. 9b. The values obtained for sliding velocity in different cycles were very repeatable with an equal recurrence time to the amplitude and dominant frequency.Based on the variation of shear stress and particularly the sliding velocity, the variation of transmitted amplitude was divided into separate increasing and decreasing phases.In the first phase,when the sliding velocity was almost zero (t = 710 s to t = 715 s), the transmitted amplitude increased because the particles were almost under stationary contact and the quality of the contact at the particle surface grew with time as described by Dieterich and Kilgore (1994). With acceleration in the sliding velocity (t = 718 s), the transmitted amplitude for all three transducers slightly decreased, which was associated with the initiation of slippage between the particles.This decreasing trend in amplitude continued more with increases in sliding velocity.However,with reduction in sliding velocity with the initiation of the next cycle, the transmitted amplitude again followed an increasing trend.

The results presented in Fig. 9 indicate that a systematic correlation may exist between sliding velocity and transmitted amplitude. In our study, a decrease in sliding velocity resulted in the evolution of transmitted amplitude and vice versa. Similar observations were made during the shearing of rock joints reported by Nagata et al. (2014) and Shreedharan et al. (2019), where the transmitted amplitude was found to be a reliable proxy for the variations in the contact area in the stable sliding mode.

The porosity changes in the gouge layers, either compaction or dilation,can influence frictional behavior(Marone et al.,1990).The frequency of transmission for ultrasonic waves is dependent on the parameters related to the gouge structure, including the particle size, particle shape,density and the amount of inter-particle voids(Pyrak-Nolte et al.,1990;Pyrak-Nolte and Nolte,1992;Pyrak-Nolte,1996; Gheibi and Hedayat, 2018). In our experiments, we continuously monitored the changes in gouge porosity and dominant frequency during stable and unstable sliding,and those results are presented in Fig.10.

For the velocity step from 5 μm/s to 1 μm/s(Fig.10a),the gouge porosity decreased suddenly as similarly observed in previous studies(Samuelson et al.,2009)and became almost constant as the sliding velocity remained equal to 1 μm/s. Correspondingly, the dominant frequency values experienced a sudden increase followed by constant values with the continuation of shear displacement. During the stable sliding regime with constant sliding velocity, the gouge layer experienced compaction mainly due to particle movement, force chain re-organization, and particle comminution, resulting in a tighter and more dense structure(Santamarina, 2003; Gheibi and Hedayat, 2018).

The cyclic trends observed during unstable sliding were also observed in the dominant frequency values obtained from all the transducers. Based on the porosity changes, different phases were observed for dominant frequency. After the peak shear stress, the gouge layer porosity gradually decreased and reached the minimum value in that specific stick-slip cycle. During this phase(t = 688 s to t = 697 s), the dominant frequency grew with a reduction in porosity and reached its maximum value at the same time corresponding to the minimum in porosity (t = 697 s). However, with increases in the shear stress and the initiation of slippage, the gouge layer gradually dilated due to the movements of particles and correspondingly, the dominant frequency values decreased. This reduction continued to the point where the maximum dilation was achieved (t = 705.5 s). Beyond this point,the dominant frequency increased again with compaction of the gouge layer in the next cycle.

4. Discussion

4.1. The role of particle comminution in ultrasonic measurements

Our experimental results demonstrated that the comminution intensity was not uniform along the surface and was generally higher for the central areas. The particle comminution in granular materials is one of the main mechanisms that control the shear strength, hydraulic conductivity, and the sliding stability in rock joints and tectonic faults with granular filling materials (Gu and Wong, 1994; Guo and Morgan, 2006). Grain comminution has been described through either grain splitting or grain abrasion mechanisms,which are both favored with increases in particle size,shear strain accumulation, and external stress (Hattori and Yamamoto, 1999; Storti et al., 2003). Abrasion is defined as the crushing of particles at the surface while splitting is referred to as the fracture of the body of particles into two or more pieces(Nakata et al., 1999; Yoshida, 2005). Depending on the stress distribution and gouge porosity, particle splitting and abrasion may take place at different locations along the fault, resulting in different gouge structures and particle size distributions (Fukumoto,1992).

Mair and Abe (2011) indicated that at the early stage of loading when the gouge fabric is not fully developed,the dominant mechanism contributing to particle comminution is the particle splitting.However, in laboratory direct shear experiments or similar natural conditions, the type of comminution may gradually switch from splitting to abrasion-dominated mechanisms with shear accumulation.Withinabrasion-dominatedmechanisms,more fineparticlesare generated and the amount of inter-particle surface area increases significantly,but when particle splitting is dominant,less fine particles are generated and the load is carried through the matrix of particles(Henderson et al.,2010;Mair and Abe,2011).

Our SEM images showed the changes in the shape and structure of the gouge layer at three different locations under the ultrasonic transducers. A significant reduction in particle size was clearly shown in the gouge layer; however, the amount of comminution and the developed structure type were different. The particles in the central area(transducers 3S,Fig.11c)experienced a significant amount of comminution with a fully-dominated abrasion mechanism.However,at the top corner of the specimen(Fig.11a),particle splitting was apparent as the dominant mechanism with detectable inter-particle voids.In the central area where particle abrasion was dominant, the generated fine particles fully covered the surface of the coarse particles and filled the inter-particle voids, which significantly influenced the inter-particle contact area and ultrasonic transmission.

Fig.11. Illustration of different particle comminution mechanisms and developed structures in the gouge layer after shear experiments at three different locations.The SEM images were obtained from the areas under transducers (a) 1S, (b) 2S, and (c) 3S.

The particle size distribution of the gouge layer for the areas under different transducers also supports the SEM image observations (see Fig.12). The particles, under transducer 1S, showed a concave distribution curve with relatively smaller particles(d < 0.1 mm). Moving toward the center of the gouge layer, the shape of the particle size distribution curve for the material under transducer 2S showed an almost linear trend while this trend became a slightly convex form for the material in the center(transducer 3S).The typical shape of the particle distribution curves shown in Fig. 12 is consistent with the results reported by Henderson et al. (2010), where the concave distribution curve(transducer 1S) was identified as the indication of particle supported structure while the convex curve was considered the matrix supported structure (abrasion-dominated) and the linear middle curve was a mix of grain-matrix supported fabric(Henderson et al.,2010).

Differences in the developed structures in gouge material along the surface are known to affect the acoustic transmissivity of the material and wave transmission significantly. Our analysis of ultrasonic wave attributes during compression and shearing showed that the results obtained from different transducers do not always follow similar trends. During compression, we observed that the amount of evolution and subsequently the absolute values of the ultrasonic elastic energy transmitted through the gouge layer were higher for the center of the layer. Similar observations were made during shearing,except during the initial part,where the amplitude and dominant frequency decreased slightly due to the disturbance of the gouge structure developed during compression. The transmission of ultrasonic waves through granular materials is well known to be dependent on the state of the contact between particles, which is a function of several factors including the contact force,area,and stiffness.Recent numerical simulations of granular assemblies considering the particle crushing process suggest that the changes in the mechanical properties of granular assemblies can be described with the changes in the particle coordination number, which is the average number of contact points for each particle(e.g.Yang and Cheng,2015;Shi et al.,2016).An increase in the particle coordination number tends to increase the number of inter-particle contact points and leads to higher acoustic transmissivity (Knuth et al., 2013). It is clear that the increase in the coordination number under abrasion-dominated comminution is much higher than that in the particle splitting process, which explains why the transmitted amplitude increases at a higher rate in the central area.It also points out the importance of considering the role of particle comminution in ultrasonic wave transmission.

Fig. 12. Comparison of the evolution of the particle size distribution for the gouge material at different locations.

4.2. Attributes of ultrasonic waves as indicators of particle scale mechanisms

Variation in the quality and quantity of the contacts in granular materials is one of the main parameters directly linked to the behavior of granular materials (Anthony and Marone, 2005; Mair and Abe, 2011; Kim et al., 2016). During shearing, the sliding stability in granular materials is highly dependent on the combined variation of the state of the contact and the true contact area as well as the shear strength at the particle scale(σs)(Kuwano et al.,2013;Bar-Sinai et al., 2014; Aragon et al., 2018). The following equation provides the variation of the true contact area with changes in sliding velocity and normal stress(Bar-Sinai et al., 2014):

where σ is the normal stress, σHis the material hardness, V is the sliding velocity, D is the typical slip distance, and b and φ are the positive material constants.Eq.(2)shows that the true contact area in granular materials is a function of the material hardness,particle size,normal stress,and sliding velocity.Based on this equation,the true contact area can change linearly with particle hardness and normal stress and has a nonlinear reverse relation with the sliding velocity.

Experimental results reported by Nagata et al. (2014) and Shreedharan et al. (2019) showed that an increase in the true contact area due to increases in the normal stress or the stationary contact time with zero slip velocity condition may be captured by an increase in the transmitted amplitude exhibited over elapsed time.Furthermore,during stable sliding,it has been observed that the transmitted amplitude decreases logarithmically with the slip velocity,similar to the changes in the real contact area,as estimated by Eq. (2) as well as the rate-state models (Dieterich, 1972;Dieterich and Kilgore,1994). Observation of the link between ultrasonic wave amplitude, sliding velocity and true contact area in transparent material and rock joints has suggested that the transmitted amplitude could be considered as a proxy to monitor the changes in the real contact area under limited stress conditions and a stable sliding mode(Nagata et al.,2014;Shreedharan et al.,2019).Our results obtained from velocity step tests and also stick-slip cycles showed a similar correlation between transmitted amplitude and slip velocity. We quantified the average values of the transmitted amplitude as a function of the slip velocity variations over the range of normal stresses explored in our study. Fig. 13 shows that the amplitude decreases logarithmically with increases in the sliding velocity,similar to that predicted for variation of the true contact area by Eq. (2) and rate-state friction laws.The observed trends can be explained using the average contact time between the particles, which is known to decrease with the evolution of sliding velocity and causes the logarithmic reduction in the contact area(Dieterich and Kilgore,1994;Gheibi et al.,2018).It should be noted that the trends presented in Fig.13 are observed only in the data obtained from transducer 1S, where the abrasion type of comminution is minimal compared to the other areas of the gouge layer. The relations between the slip velocity and the transmitted amplitude for the other transducers were observed only during the velocity steps and each individual stick-slip for short-range slip accumulation and not for the general trend throughout the experiment. In fact, for the wide range of slip accumulation, the roles of porosity changes and particle comminution mechanisms were more significant than that of the slip velocity,which indicated that it would be necessary to consider the impact of the comminution and porosity changes in interpretation of the ultrasonic measurements in granular materials.

Fig. 13. Variations in the averaged values of the transmitted amplitude versus the logarithm of the sliding velocity for a series of experiments at different normal stresses. The dashed lines represent the least square best fit to each data set.

Our results for the variation of transmitted amplitude with the evolution of normal stress for different sliding velocities indicate a nonlinear trend, as shown in Fig. 14. Granular assemblies were generally referred to as elastoplastic materials in the sense that inelastic compression and shear deformation are likely to develop in granular materials under a given loading path,resulting in plastic(irreversible) deformations and nonlinear evolution of acoustic transmissivity. However, previous experimental studies on rock joints or similar non-welded interfaces have documented that the transmitted amplitude is linearly proportional to the normal stress or contact stiffness as similarly predicted with the elastic solutions for the deformation of multi-contact interfaces(Nagata et al.,2014;Shreedharan et al.,2019).However,these elastic solutions may not accurately model the inelastic behavior of granular materials.

Fig.14. Evolution of the averaged values of transmitted amplitude with normal stress for a series of experiments at different sliding velocities. For a given sliding velocity,the transmitted amplitude evolution was nonlinearly consistent with nonlinear deformation in the granular layers.

The nonlinearity of the amplitude variations observed in Fig.14 was due to the nature and the transition of the mechanisms involved in the deformation of granular materials as well as the increase in the normal stress.In order to separate the impact of the normal stress evolution and deformation mechanisms, the transmitted amplitude values were linearly normalized with the normal stress as suggested by Shreedharan et al.(2019)and plotted versus the normal deformation in Fig.15.Fig.15 shows that in addition to the normal stress, a reduction in porosity would also facilitate the transmission of ultrasonic waves, particularly in a nonlinear increasing trend. The nonlinear trend in Fig. 15 was due to the evolution of particle comminution,which significantly contributed to the amount of particle surface areas and showed the nonlinear increase in the amount of inter-particle contact areas.

In addition to the quality of the inter-particle contact points,frictional strength and stability are also inter-related with dilation and compaction in the granular gouge layers (van den Ende and Niemeijer, 2018). Dilation and compaction and the related porosity changes can be described as the work done by normal stress,which results in changes in the friction as well as the fabric and development of shear localization(Marone et al.,1990;Kaproth and Marone, 2014). Volume changes in granular materials are basically due to the collapse and deformation of the force chains which are directly related to the pore volume changes at the particle scale (Zhang et al., 2017). Inter-particle pore volumes can be imaged by relative changes in ultrasonic wave attributes, particularly the dominant frequency of the transmitted waves. The link between dominant frequency changes and inter-particle void volumes can be explained through the displacement discontinuity theory (Pyrak-Nolte et al.,1990; Pyrak-Nolte,1996). Based on this theory, the size and distribution of fractures and voids in geomaterials can impact the frequency of ultrasonic waves traveling through the material.The openings and voids act as barriers which tend to filter the high-frequency content of the waves(Pyrak-Nolte,1996; Hedayat et al., 2018). With increases in normal stress and compression,the sizes of the voids decrease,but the frequencies at which the waves can travel increase. Hence, during dilation, an opposite trend is expected.

During the initial compression stage, our results show that the dominant frequency increased at a decreasing rate with normal stress. Similar to the amplitude variations, the external force and porosity are the two main factors that significantly affect the dominant frequency values. We normalized the variation of dominant frequency with normal stress in order to better evaluate the relation between porosity and dominant frequency. Similarly,the porosity values were normalized with respect to their corresponding normal stress to solely examine the link between gouge structure and dominant frequency. Fig.16 shows the variation of normalized porosity and normalized dominant frequency during compression.The trends shown in Fig.16 for the normalized dominant frequency closely follow a nonlinear trend similar to that of the normalized porosity, indicating a close correlation between dominant frequency and the volume of inter-particle voids during compression.

Fig. 15. Nonlinear variation of normalized transmitted amplitude with respect to normal stress versus compression illustrating the impact of porosity changes and particle comminution on the evolution of transmitted amplitude.

During stick-slip cycles, a combination of compaction and dilation with a net amount of compaction occurs (Fig. 7b). In our experiments, we documented the variation of layer porosity(compaction/dilation)and dominant frequency for different values of normal stress and sliding velocity during unstable sliding.Dominant frequency and porosity follow cyclic trends with identical recurrence time as shear stress does.Details on the variation of dominant frequency in each single stick-slip cycle reveal that dominant frequency decreases with increase in porosity and vice versa (see Fig. 10b). The scaled dominant frequency and porosity variations during the stick-slip cycles under different sliding velocities are shown in Fig.17. In contrast to the initial compression stage,the normal stress was constant throughout the shear process.Hence, there was no need to normalize the values with respect to normal stress. However, we scaled the values in order to have all the curves started from unity. It is very interesting that although several different particle scale mechanisms were involved during shearing and stick-slip cycles, the dominant frequency followed a trend identical to the porosity trend for multiple stick-slip cycles for different sliding velocities. This trend showed that the link observed between the dominant frequency and porosity during compression was also valid during shearing even when more complicated deformation mechanisms were involved.

We monitored the variation of layer porosity and the corresponding changes in dominant frequency for different experiments to examine whether the consistency observed in Figs.17 and 18 was valid for different stress conditions. Fig.18 quantifies the averaged variations in layer porosity and dominant frequency in multiple stick-slip cycles for sliding velocities of 20 μm/s, 40 μm/s and 80 μm/s. For normal stress of 15 MPa, stick-slips were not clearly observed with a sliding velocity of 20 μm/s,but for higher values of normal stress,compaction and dilation in gouge layers were easily detectable. We observed that in agreement with the data in the literature (Marone et al., 1990; Karner and Marone, 2001), both layer compaction and dilation increased with the sliding velocity,due to the larger amount of kinetic energy provided by the higher sliding velocity which acted against the normal stress (Scuderi et al., 2015). This increase in the amount of compaction and dilation with the sliding velocity was also observed with similar trends in the evolution of dominant frequency. The impact of normal stress on volume changes was different from that of sliding velocity and had an opposite impact on dilation and compaction,as shown in Fig.18. The variation of dominant frequency due to variations in normal stress was consistent with the changes in layer thickness. Fig. 18 illustrates that the frequency of the ultrasonic waves transmitted through the gouge layer is sensitive to the changes in the volume of inter-particle voids and subsequently can be considered a good measure to monitor the changes in layer porosity and structure.This sensitivity is due to the dependency of ultrasonic wavelengths to the volume and distribution of interparticle voids (Pyrak-Nolte and Nolte,1992; Pyrak-Nolte,1996).

Fig. 16. Comparing the trends for normalized porosity and normalized dominant frequency with normal stress during compression. The observed consistency emphasizes the inter-relation between dominant frequency and gouge porosity.

Fig. 17. Similarity in the evolutions of dominant frequency and porosity changes during multiple stick-slip cycles for different sliding velocities at normal stress of 20 MPa.

Fig.18. Comparing the trends of volume changes with the variations in dominant frequency during the unstable sliding: Amount of (a) compaction and (b) dilation and corresponding changes in dominant frequency with evolution of sliding velocity under four different normal stresses.

Our observations provided insights into the variations of ultrasonic wave amplitude and dominant frequency in different modes of sliding of granular quartz gouges.The transmitted amplitude was shown to be dependent on multiple factors in granular materials such as normal stress, sliding velocity, porosity, and particle comminution mechanisms. We observed an opposite trend between the sliding velocity and transmitted amplitude which could provide information about the contact area variation during shearing. However, since porosity changes are associated with shearing in granular materials,the interpretation of the changes in ultrasonic amplitude should be conducted carefully to distinguish between the types of particle scale mechanisms and porosity variations.This is particularly the case because transmitted amplitude and porosity have a nonlinear correlation. Dominant frequency in our experiments was found to be a good measure to qualitatively monitor the changes in porosity and structure of granular materials. Although our observations were made based on laboratory experiments on standard materials, the microscale mechanisms involved in laboratory and natural faults would share similar fundamental characteristics. Hence, our findings afford possible new avenues in application of ultrasonic waves to better monitor the frictional mechanisms and the changes in characteristics of tectonic faults. Our observations indicate additional potentials for individualizing different mechanisms through the simultaneous analysis of ultrasonic wave amplitude and frequency. The findings could also improve the methodologies for detecting seismic precursor prior to shear failure in tectonic faults and landslides.However, additional field scale studies are required to better understand the impact of scale difference between laboratory and natural faults.

5. Conclusions

The results presented in this paper provided insights into the changes in the ultrasonic wave attributes that correspond with particle scale mechanisms during compression and shearing of granular materials under a wide range of external stresses and sliding modes. We monitored the gouge layer itself as well as the changes in the transmitted amplitude and dominant frequency of the ultrasonic waves at different locations while the gouge layers were being subjected to compressive and shear loads.We observed that non-uniformity along the fault could have promoted different comminution mechanisms and ultrasonic transmissivity values.Throughout the stable sliding phase, the variation of the transmitted amplitude was observed as opposite to the sliding velocity,and the rates of changes in the dominant frequency and layer porosity were closely related. During unstable sliding, the ultrasonic wave attributes followed the same cyclic behavior as that of shear stress.Similar to the observations during stable sliding mode,we found that variation of the sliding velocity was closely linked to changes in transmitted amplitude, which was due to the interrelation between the transmitted amplitude, inter-particle contact properties, and sliding velocity. However, the occurrence of different comminution mechanisms due to the non-uniform distribution of normal and shear stresses may have resulted in various general trends in transmitted amplitude and dominant frequency evolutions throughout our experiments.Our results also suggested that the ultrasonic wave transmission frequency through granular materials may be a function of the inter-particle pore volume, and hence the dominant frequency could be a valuable measure for assessing the porosity changes within the granular gouge layers.

Declaration of competing interest

The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.

Acknowledgments

The authors would like to acknowledge the supports provided by the Southern California Earthquake Center (Grant No. 17242),and the U.S. Department of Energy (Grant No.DE-SC0019117).