Effect of fatigue loading-confining stress unloading rate on marble mechanical behaviors: An insight into fracture evolution analyses
Yu Wng, Dongqio Liu, Jinqing Hn, Chnghong Li, Ho Liu
a Beijing Key Laboratory of Urban Underground Space Engineering,Department of Civil Engineering,School of Civil and Resource Engineering,University of Science and Technology Beijing, Beijing,100083, China
b State Key Laboratory for GeoMechanics and Deep Underground Engineering, China University of Mining and Technology, Beijing,100083, China
c Institute of Acoustics, Chinese Academy of Sciences, Beijing,100190, China
Keywords:Fatigue loading Confining stress unloading Unloading rate Energy evolution Computed tomography (CT) scanning
A B S T R A C T Rocks in underground works usually experience rather complex stress disturbance. For this, their fracture mechanism is significantly different from rocks subjected to conventional triaxial compression conditions. The effects of stress disturbances on rock geomechanical behaviors under fatigue loading conditions and triaxial unloading conditions have been reported in previous studies. However, little is known about the dependence of the unloading rate on fatigue loading and confining stress unloading(FL-CSU) conditions that influence rock failure. In this paper, we aimed at investigating the fracture behaviors of marble under FL-CSU conditions using the post-test X-ray computed tomography (CT)scanning technique and the GCTS RTR 2000 rock mechanics system. Results show that damage accumulation at the fatigue stage can influence the final fracture behaviors of marble. The stored elastic energy for rock samples under FL-CSU tests is relatively larger compared to those under conventional triaxial tests, and the dissipated energy used to drive damage evolution and crack propagation is larger for FL-CSU tests.In FL-CSU tests,as the unloading rate increases,the dissipated energy grows and elastic energy reduces.CT scanning after the test reveals the impacts of the unloading rate on the crack pattern and a fracture degree index is therein defined in this context to represent the crack dimension.It shows that the crack pattern after FL-CSU tests depends on the unloading rate, and the fracture degree is in agreement with the analysis of both the energy dissipation and the amount of energy released.The effect of unloading rate on fracture evolution characteristics of marble is revealed by a series of FL-CSU tests.
1. Introduction
The engineered rocks usually experience stress disturbance during the construction stage of underground rock structures.Various types of disturbances (e.g. excavation, rock blasting,explosive fracturing, and vibration) could disturb the original in situ stress field.The rock mass in the vicinity of the working face is generally in the fatigue stress state during and after excavation. In this sense,the excavation zone composed of an original stress zone,a stress-increasing zone,and a stress-decreasing zone exists in the vicinity of the excavation surface (Bagde and Petroˇs, 2005;Fuenkajorn and Phueakphum,2010; Cerfontaine and Collin,2018).The stress disturbance on rock structures is crucial to its long-time stability, and as a result, investigation of the geomechanical properties of rocks under fatigue loading and confining stress unloading(FL-CSU) conditions is of significant importance in the subject of the rock geomechanics investigation and rock engineering stability prediction.
Rocks undergoing fatigue disturbance and triaxial unloading,respectively, have been intensively investigated. The influence of triaxial loading and unloading paths on rock fracture has attracted wide attentions(Zhao et al.,2014;He et al.,2015;Ding et al.,2016;Guo et al., 2017; Xu et al., 2017; Yang et al., 2019). Under varying loading and unloading conditions, new triaxial test and conventional triaxial test were performed. By adopting conventional triaxial unloading test, the damage evolution or rock treated with high temperature was studied(Ding et al.,2016),and it was found that the failure pattern of sandstone was influenced by both the temperature and the initial confining stress. Brittle failure was much more obvious for rock under relatively high confining stress.Triaxial loading and unloading tests were conducted on granite samples in order to understand the effect of the unloading rate on rock mechanical responses(Li et al.,2017).He et al.(2015)proposed that rock is affected by the shear traction in the direction of intermediate stress during the rockburst process based on the analyses of the microscopic and macroscopic fracture processes of granite samples under true triaxial unloading paths. The failure mechanism of rock under true triaxial unloading paths is totally different from those under uniaxial compression and confined compression tests. Zhao et al.(2014)carried out true triaxial unloading tests on granite samples and concluded that strain detonation easily occurred when the unloading rate was high. Takeda and Manaka(2018) experimentally studied the stress dependence of shale semi-permeability during loading and unloading stresses. Liu and He (2012) proposed a residual strain method that describes the initial fatigue damage and damage propagation after studying the influence of confining stress on the mechanical properties of intact rock samples under cyclic loading. Their results showed that the shear fracture surface becomes wider with increasing triaxial confining stress. Wang et al. (2013) used the triaxial compression test to study the fatigue behavior of granite. They suggested that the fatigue behavior of rocks can be described by the residual axial strain. The creep and expansion behaviors of salt rocks under fatigue loading were investigated by Roberts et al.(2015),and Taheri et al. (2017) performed cyclic loading test on brown coal and suggested that the mechanical properties of lignite were not greatly affected by cyclic loading. They also demonstrated that lignite accumulated irreversible axial strain. Furthermore, the local damage and progressive localization of porous sandstone during cyclic loading and the strength and deformation characteristics of the sandstone were revealed by Munoz and Taheri (2017a).Voznesenskii et al. (2017) showed the relationship between ultrasonic quality factor and strength of rock salt under cyclic fatigue loading.He et al.(2019)experimentally studied salts with different loading frequencies, stress amplitudes and loading rates, and proposed a relationship between fatigue life and fatigue loading conditions. Munoz and Taheri (2018) investigated effects of cyclic loading on the deformational characteristics of both the nonlocalized damage zone(NLDZ)and the localized damage zone(LDZ)of rock in the post-peak regime,and found that the material located in the NLDZ experiences much less damage in contrast to material located in the LDZ. Zhang et al. (2019) conducted triaxial cyclic loading test to reveal the pre- and post-peak damage characteristics of sandstone, and found that the maximum dissipated energy occurs after the peak stress.
Investigations concerning the cyclic dynamic loading on rock geomechanical properties have also been intensively studied. For example,the influences of stress amplitude and loading frequency on fatigue damage of rocks have been investigated(Ray et al.,1999;Li et al.,2001,2009;2019;Bagde and Petroˇs,2005;Ren et al.,2013;He et al., 2019). Bagde and Petroˇs (2005, 2009) conducted cyclic tests on intact sandstone samples under fatigue loading, and pointed out that the applied loading frequency and amplitude have a major influence on the rock dynamic behaviors. Fuenkajorn and Phueakphum (2010) conducted triaxial cyclic tests and determined the relationship between the rock strength and cyclic loading number(N),and suggested that the elastic modulus almost remains constant until rock failure. Wang et al. (2020) conducted stress disturbance experiment with FL-CSU path, and the anisotropic energy and fracture behaviors of interbedded marbles were studied. They found that rock structure has obvious influence on rock failure and energy release.
The previous studies mainly focused on the effects of triaxial unloading and cyclic fatigue loading on rock geomechanical properties. Although these two loading paths have been widely studied individually, investigations on rock fracturing that first underwent fatigue loading and then unloading conditions are rare.In this study,a series of triaxial mechanical tests is conducted under these conditions using the GCTS RTR 2000 rock mechanics testing system to study the deformation and energy evolution of marble experiencing fatigue loading and unloading. Different stress paths are used to test the marble samples:the conventional monotonous triaxial compression tests (TC), conventional triaxial unloading tests(TCU),and FL-CSU tests.The purpose of this investigation is to understand the effects of the unloading rates of FL-CSU tests on the fracture behaviors of marble. The axial, lateral and volumetric deformations of marble samples are analyzed.In addition,the fatigue damage evolution and energy evolution characteristics to reveal the fracture mechanism of marble are discussed. After the FL-CSU test, the crack pattern in marble samples is visualized and the relationships among the fracture degree, energy dissipation, and energy release characteristics are discussed. The experimental results are likely to provide a theoretical basis for studying the fracture mechanism of marble during underground metal mining under site-specific fatigue and unloading conditions.
2. Experimental methods
2.1. Rock materials
The rock cores were sampled at 600 m depth in the Lilou iron mine located in Anhui Province, China, with an obviously interlayered structure (Fig. 1a). During the mining process, the mine pillars and chambers were subjected to repeated stress disturbance activities,such as rock excavation and blasting.In this case,the rock pillars basically experienced fatigue dynamic disturbances prior to excavation in terms of a two-step mining operations. The marble used is composed of a cyan rock matrix and interbedded white bands(see Fig.1c).X-ray powder diffraction(XRD)analysis results indicate that the rock matrix is mostly composed of quartz(63.12%),hematite (16.84%), calcite (11.11%), and amphibole (8.93%). The mineral content of the white band is dominated by dolomite.Scanning electron microscope (SEM) imaging analysis (Fig. 2)shows micro-cracks at the interface of marble and dolomite, and various dissolved voids in the dolomite are found as well.
Cylindrical rock cores were made with a diameter(D)of 50 mm and a height (H) of 100 mm according to the ISRM suggested methods (Ulusay, 2014). For all of the tested samples, the samples come from larger cores that have a diameter of 130 mm, and the samples apparently display strong interbedded structure,having an orientation of 15°with respect to the drilling direction,as shown in Fig.1b. Both ends of the samples were then polished with a precision within±0.05 mm,and both ends have precise parallelism of less than 0.1 mm. The average dry density of the marble cores is 2.86 g/cm3. The mean P- and S-wave velocities of the tested samples are 6043 m/s and 3647 m/s, respectively.
2.2. Laboratory test apparatus
A GCTS RTR 2000 rock mechanics testing system with a loading capacity of 4500 kN was used for triaxial compression tests,with a loading frequency of 0-10 Hz. One circumferential sensor (linear variable differential transformer(LVDT)system)and a pair of axial sensors were used during testing to measure the axial and lateral strains of the rock samples simultaneously during the FL-CSU test.The rock strength,axial strain, axial stress and transverse strain at the same sampling frequency were recorded by the central computer. At the end of the FL-CSU test, X-ray computed tomography(CT)scanning was performed on each sample to obtain the internal crack pattern of the marble sample. In this study, CT images were recorded by a high-resolution industrial CT machine that has a source voltage of 450 kV and a source current of 2 mA. Five CT images obtained for each sample were taken from the top,middle and bottom of the sample corresponding to the following positions:70 mm, 60 mm, 50 mm, 40 mm, and 30 mm, respectively. The internal failure morphology of the marble samples can be seen based on the CT images.The testing apparatus used in this study is shown in Fig. 3.

Fig.1. Location of the Lilou iron mine and preparation of tested marble samples: (a) Sampling location; (b)Field drilling of marble cores that have a diameter of 130 mm;and (c)Marble samples with an interbedded structure.

Fig. 2. SEM images of marble with magnifications of (a) 200, (b) 500, (c) 2000, and (d) 5000 times. (b)-(d) show the magnification of the yellow region in (a).
2.3. Test scheme
The tested marble samples were basically divided into three groups: group I used to perform the monotonous triaxial compression test,group II for the triaxial confining stress unloading test, and group III for FL-CSU tests. Ultrasonic P- and S-wave velocities of the marble samples were measured in advance in order to ensure the consistency of the rock structure and composition.Triaxial compression tests on group I samples were conducted to determine the peak stress. For group III, the marble samples generally went through three loading stages(see Fig.4):the static loading stage, the fatigue loading stage, and the confining stress unloading stage. At the first stage, an axial strain loading rate of 0.06 mm/min was maintained until the axial stress was equal to the mean stress(an equivalent strain rate of 1×10-5s-1)of 110 MPa.At the second stage,the upper limit stress was set to be ~0.98 times that in the triaxial compression test for rock subjected to a confining stress of 20 MPa(i.e.170 MPa) for this low-cycle fatigue loading test. The stress-controlled stage had a load-controlled loading rate of 20 kN/s, and cyclic loading started when the triaxial stress was equal to 110 MPa (Fig. 4). The axial fatigue loading was specified to be a sinusoidal cyclic compressive load with a frequency of 0.05 Hz(i.e. the loading-unloading duration is 10 s).The loading path chosen for this study is also shown in Fig.4.At the last stage, to simulate excavation unloading, the confining stress unloading was performed with unloading rates of 0.02 MPa/s, 0.05 MPa/s, 0.2 MPa/s, and 0.4 MPa/s, respectively. The basic physico-mechanical parameters of the tested marble samples are summarized in Table 1.The purpose of confining stress unloading is to investigate the effects of the unloading rate on the fracture of marble. The detailed loading paths are tabulated in Table 2.

Fig.3. Testing system for marble samples subjected to FL-CSV loading paths:(a)GCTS RTR 2000 rock mechanics apparatus; (b) Axial and lateral deformation measurement device; and (c) The 450 kV industrial CT system employed to visualize crack pattern.
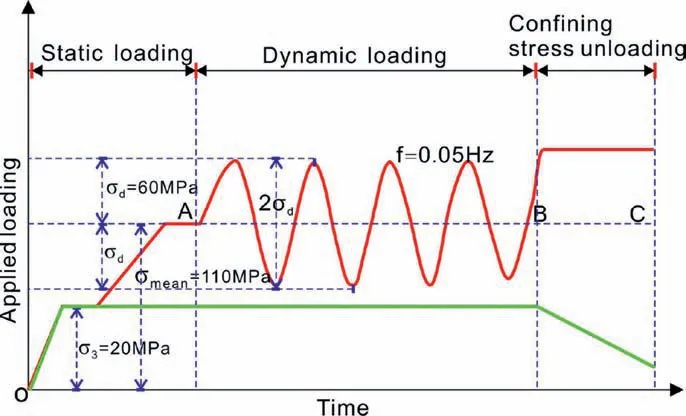
Fig.4. Scheme of the FL-CSU loading path for the marble samples.OA:Sketch of static loading stage;AB:Sketch of dynamic loading stage;and BC:Confining stress unloading stage. In this figure, σ3 is the confining stress, σmean is the mean stress, and σd is the cyclic stress.The red line shows the change of axial stress and the green line represents the change of confining stress.
2.4. Energy calculation
In the triaxial test,both the axial stress σ1and confining stress σ3respectively provide positive work for the samples during the deformation process and under hydrostatic loading stress.Increasing axial stress can increase the energy input of the sample until the maximum differential stress is obtained. The radial expansion of the deformed specimen causes negative work when the confining stress is applied. Therefore,the total strain energy U for the triaxial test can be expressed as (Li et al., 2017):

where U1is the absorbed strain energy of axial compression by σ1after application of hydrostatic stress, U3is the consumed strain energy due to negative work for σ3for radical expansion after hydrostatic stress, and U0is the absorbed strain energy due to hydrostatic stress.
At any time during the triaxial test,the absorbed and consumed strain energies (U1and U3) can be calculated by integrating the stress-strain curve as follows:



Table 1 Basic physico-mechanical parameters of marble samples.

Table 2 Testing scheme of triaxial unloading test for marble samples.

where n is the total number of trapezoids for the stress-strain curves, and i is the segmentation points.
The absorbed strain energy due to hydrostatic stress loading U0can be calculated with the following elastic equation:

where E is the rock elastic modulus,ν is the initial Poisson’s ratio,andis the initial confining stress.
The energy absorbed in any physical process without heat exchange can be regarded as the sum of the elastic energy Ueand the dissipated energy Udaccording to the energy conservation principle, i.e.

According to Xie et al. (2009, 2011), Huang and Li (2014) and Wang et al.(2020),the elastic strain energy Uecan be shown at any time during the experimental process as
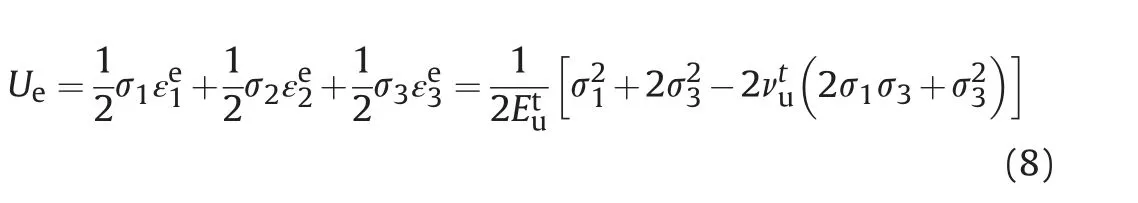
3. Results and discussion
3.1. Conventional triaxial stress-strain responses
Fig. 5 plots the complete stress-strain responses of marble under continuous static loading and unloading confining stress conditions. In Fig. 5a, the peak deviatoric stress is 173.52 MPa and the peak strain is 0.195%.Relatively large plastic deformation occurs for marble under triaxial compression. After the peak stress, the stress-strain curves drop gradually with a certain residual strength. Fig. 5b shows that the lateral strain begins to increase quickly for the marble sample after the unloading point. The volumetric dilatation effect is obvious compared to the sample subjected to conventional triaxial test (the strain is about 0.2% in Fig. 5a). At the failure point, the axial strain, lateral strain and volumetric strain are 0.306%, 0.357%, and -0.457%, respectively.However, for the sample under continuous triaxial stress loading path, the corresponding strains are 0.191%, 0.097%, and -0.015%,respectively. The strain in continuous loading stress conditions is smaller than that in unloading conditions.The result indicates that the unloading confining stress has a potential damage to rock stability. The axial deviatoric stress continuously increases as confining stress decreases, and the deformation of the marble sample increases suddenly till rock failure.
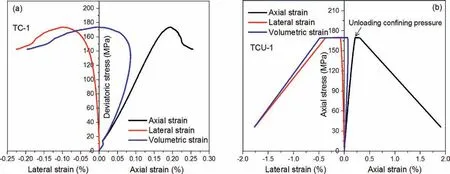
Fig.5. Representative stress-strain curves for the tested samples under conventional triaxial compression test:(a)Continuous triaxial loading test;and(b)Triaxial confining stress unloading test with an unloading rate of 0.05 MPa/s.
3.2. Triaxial FL-CSU stress-strain responses
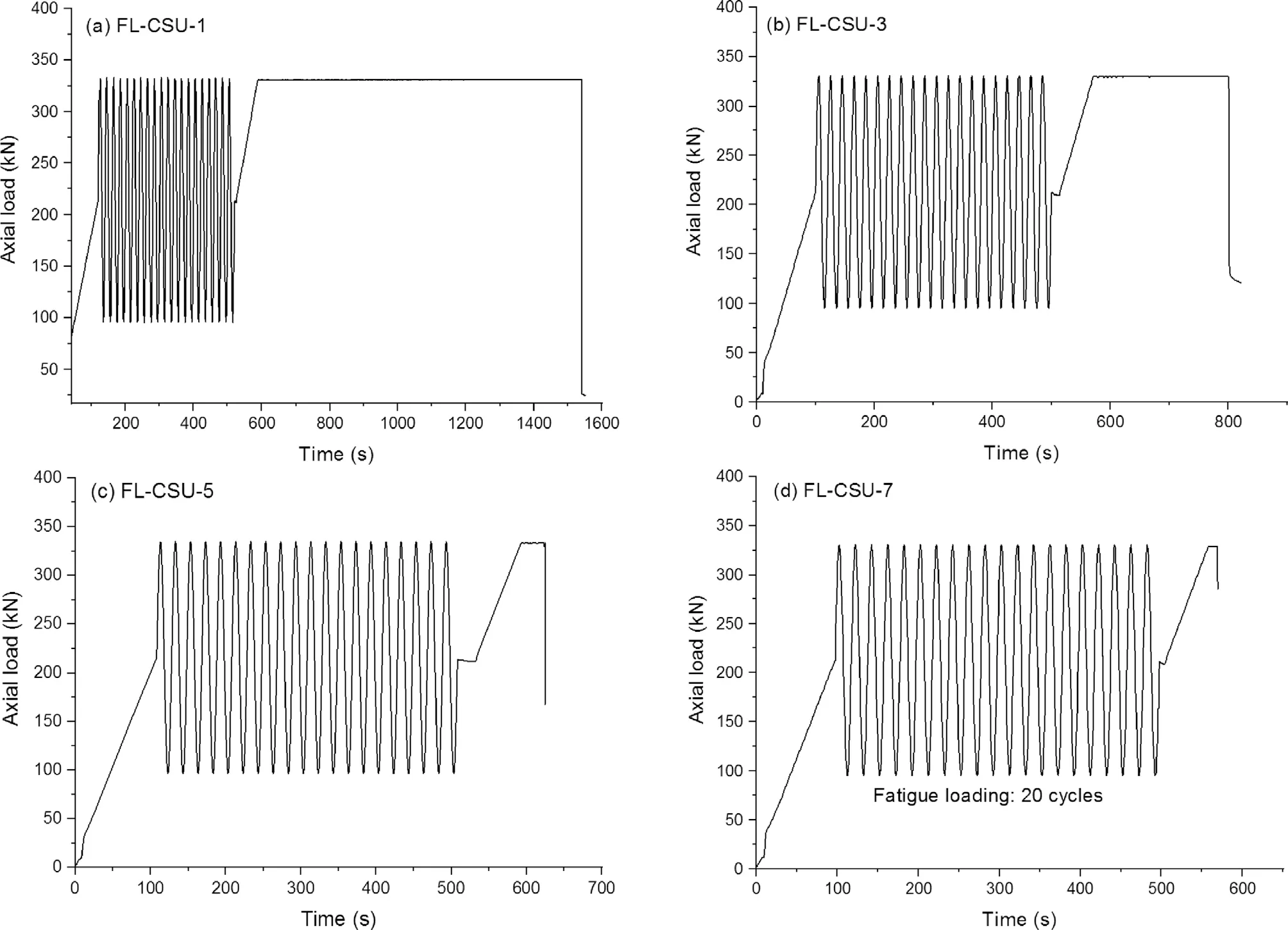
Fig. 6. The relationship between axial load and time for marble under FL-CSU test with confining stress unloading rates of (a) 0.02 MPa/s, (b) 0.05 MPa/s, (c) 0.2 MPa/s, and (d)0.4 MPa/s.
The typical loading-time curves for marble under FL-CSU test are shown in Fig. 6. It shows that during the confining stress unloading stages, the axial stress is kept constant as a stresscontrolled mode was used. At the sample failure point, a sudden failure occurs and the axial force drops sharply to a minimum.It can also be noticed that the time duration decreases with the increase in stress unloading rate.For a sample under a stress unloading rate of 0.02 MPa/s, the time before sample failure is the longest(about 592 s),while it is the shortest for a sample under an unloading rate of 0.4 MPa/s, with the time period before sample failure of about 14 s. The result indicates that increasing the confining stress unloading rate can result in earlier failure of the marble sample.During the FL-CSU experiment,we can also observe that a sudden failure occurs, but the stress drop degree is different from the samples under different stress unloading rates - the stress drop amplitude is the largest for the sample under a relatively low unloading rate. During the experiments, it has also been noticed that the sound generated in rocks is the largest for a sample with the highest unloading rate, and it implies that the elastic energy stored in the sample is suddenly released after failure.
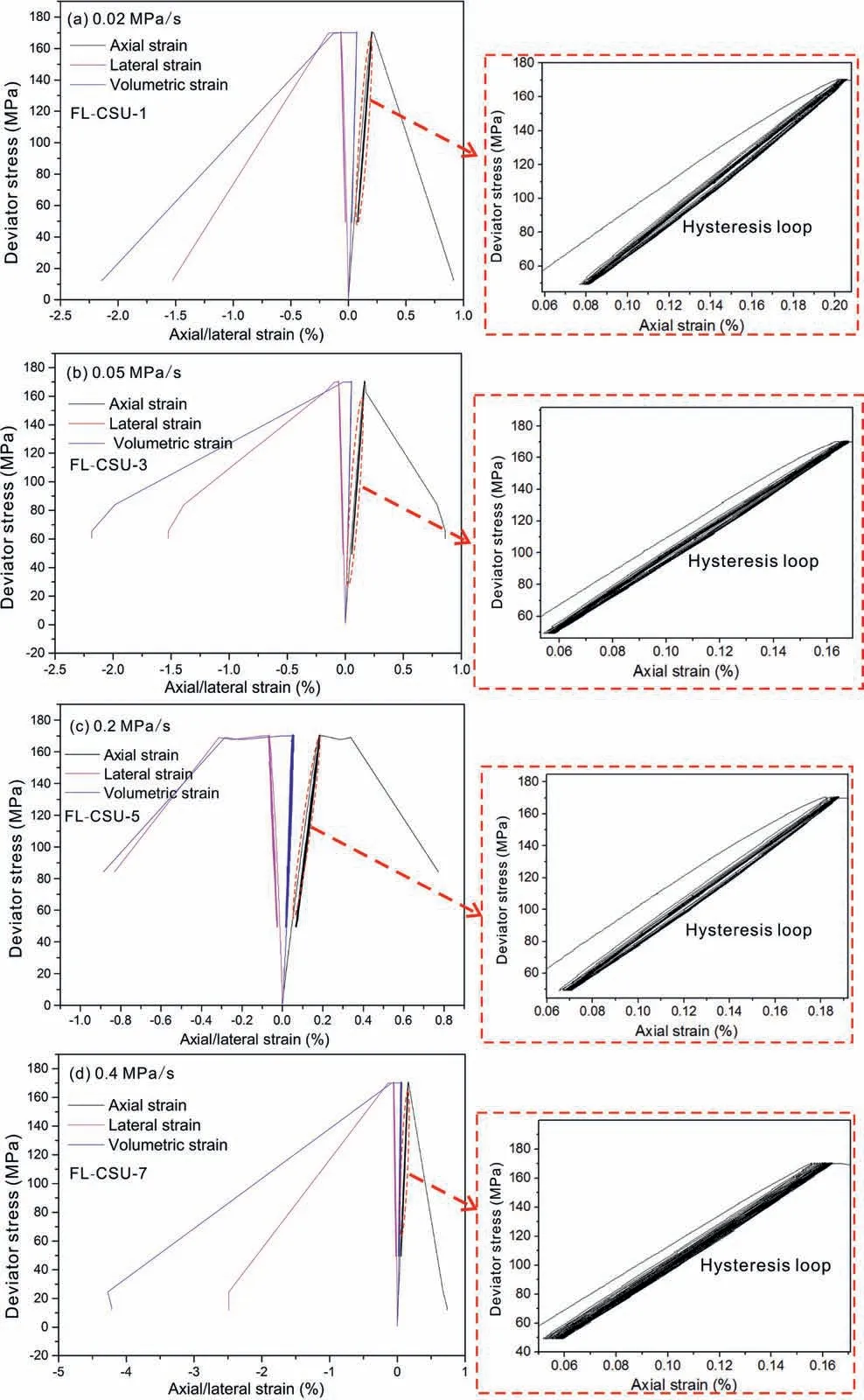
Fig. 7. The axial, lateral and volumetric stress-strain responses for marble samples under different confining stress unloading rates: (a)0.02 MPa/s, (b) 0.05 MPa/s,(c) 0.2 MPa/s,and (d) 0.4 MPa/s, respectively.
The complete stress-strain curves during the FL-CSU test are shown in Fig. 7. To observe the morphology of the rock sample under hysteresis stress loop, the axial stress-strain curve corresponding to the cyclic dynamic stage is magnified in this figure.During the stress distribution stage(i.e.fatigue loading stage),the samples generally undergo irreversible plastic deformation and the loading curve does not overlap with the unloading curve with increase in cycle number. In this case, a hysteresis loop forms during low-cycle loading conditions. The reason to apply cyclic loading is to simulate stress disturbance. In this work, 20 cycles are applied to the marble sample.Although damage occurs in the marble sample at the fatigue loading stage,failure does not occur for all the tested samples. Followed by the fatigue stage is the confining stress unloading applied to the marble sample, and failure occurs abruptly at some moment (567 s, 623 s, 808 s, and 1556 s, respectively). The lateral and volumetric deformations grow faster than the axial deformation during the unloading stages. The axial stress is kept the same during the stress unloading stage due to the decrease in confining stress.Although the testing machine does not add strain energy to the sample,the deviatoric stress continues to increase until rock failure.
3.3. Deformation in the FL-CSU tests
The deformation characteristics of the marble sample in our tests are basically composed of three parts,i.e.deformations in the static stress loading,fatigue,and confining stress unloading stages.In order to describe the whole deformation process during the FLCSU tests, the relationship between the axial strain and time is plotted, as shown in Fig. 8. For the four unloading cases, the axial strain continuously increases, and the increasing rate becomes steady at the fatigue stage and then grows faster until failure of the marble.The lateral strain exhibits a similar trend as that of the axial deformation. The volumetric strain first increases with elapsed time, and then the incremental rate becomes larger. The result indicates that the compressive deformation dominates the volumetric changes. Then, the volumetric strain decreases and volume expansion plays a significant role in sample deformation. Some scholars(e.g.Bagde and Petroˇs,2005;Liu and He,2012)studied the strain variation law of rocks under fatigue load,and they proposed that rocks will undergo three stages under fatigue load: initial deformation stage, stable deformation stage and accelerated deformation stage.In this work,we chose a low-cycle loading type during the cyclic stage. The damage to the rock sample generally accumulates in this stage, and it belongs to the second stage according to the loading path shown in Fig.6.For the samples at the four confining stress unloading rates, the axial strain increases abruptly at some moment, and then sudden failure occurs.

Fig. 8. Plots of deformation characteristics of the tested marble samples subjected to FL-CSU tests with confining stress unloading rates of (a) 0.02 MPa/s, (b) 0.05 MPa/s, (c)0.2 MPa/s, and (d) 0.4 MPa/s.
In order to reveal the accumulative damage evolution characteristics at the second loading stage(i.e.fatigue loading stage),the axial, lateral and volumetric strains against the cycle are shown in Fig. 9. In this figure,the axial strain first shows a fast increase and then a stable increase pattern (see Fig. 9a). The lateral strain also shows a similar increase pattern (see Fig. 9b). The sample volume experiences a translation from compression to dilation(see Fig.9c).From the results,it also can be observed that the three strain curves are apparently different even for the same kind of marble samples used, indicating the effect of rock internal structure on the deformation characteristics.Although the values of the three strains vary individually,the axial,lateral and volumetric strain results are close to each other at the four unloading stress rates.
To reveal the damage accumulation of marble that has experienced 20 cycles at the second stage numerically,the damage index which is defined using the cyclic axial strain is introduced herein.A one-dimensional (1D) damage constitutive equation based on the continuum damage mechanics was proposed according to the basic principle of hypothesis strain equivalence mechanism,i.e.

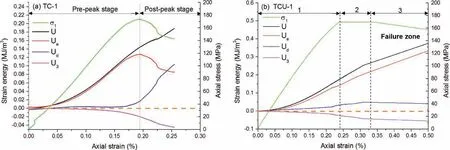
where σ and ε are the stress and strain of the undamaged marble,respectively; and D is the damage index. When the rock is undamaged,D=0;when the marble is completely destroyed,D=1;and at the fatigue loading stage,we have 0 If the damage in a single cycle is not considered,σ is assumed to be a constant. The constitutive equation can be written as (Ren et al., 2013): Assuming that ε0is the initial strain before fatigue loading and εdis the final strain after sample fatigue failure,the damage index D would be equal to 0 and 1,respectively.By integrating Eq.(11),we have From Eq. (6), the damage index D can be obtained as follows: where C2is a constant.When D=0 and ε=ε0in view of Eq.(13),we have C2= 0. When D = 1 and ε = εd, we can rewrite Eq. (13) as Fig. 9. Evolutions of (a) axial strain, (b) lateral strain, (c) volumetric strain, and (d) damage index against fatigue cycles under different confining stress unloading rates of 0.02 MPa/s, 0.05 MPa/s, 0.2 MPa/s, and 0.5 MPa/s for the marble samples. The damage index D of marble can be written by combining Eqs.(13) and (14) as follows: The damage evolution characteristics can be obtained during fatigue loading by using the definition of the damage index in Eq.(15).The relationship between the cycle number and damage index is shown in Fig. 9d. It displays that the damage increases with increasing cycle number; however, the increasing rate becomes slower, and the damage accumulation for the tested sample is almost the same after the 20th cycle.As damage inside the sample is roughly the same after stress redistribution,the influence factor to marble fracture can be only attributed to the applied confining stress unloading rate after fatigue loading stage. Based on Eqs. (4), (5), (7) and (8), the strain energy evolution characteristics can be obtained and are shown in Fig.10. The total strain energy(U)shows a similar trend as the axial strain increases for the samples under different unloading conditions. The difference for the total strain energy (U) for the four cases is that the change in the energy for the fatigue loading stage is different.With increase in the stress unloading rate, the variation of total strain energy (U) becomes smaller, and the fluctuation degree of U reduces.The elastic energy(Ue)curve also shows a similar pattern as the deformation increases and the accumulated energy is released at the point of failure. However, the dissipated energy (Ud) curve shows different trends,especially during the fatigue loading stage.When the unloading rates are 0.02 MPa/s and 0.05 MPa/s, Udpresents a similar growth trend with the increase in axial strain of marble. However, when the unloading rates are 0.2 MPa/s and 0.4 MPa/s, the unloading rate of marble shows a downward trend.The results show that the stress unloading rate has a great influence on energy dissipation and release,which may lead to the difference in failure modes of samples. For the strain energy (U3) curve, it decreases with increase in axial strain. Sample’s lateral expansion consumes a part of the energy provided by the testing machine,and it rapidly decreases at the unloading stage. One can see from the energy curves shown in Fig. 10, at the initial loading stage, Ueis almost the same as U. Therefore, the absorbed energy from the testing machine transfers almost completely into elastic energy, and therefore, no Udis generated.With increase in axial deformation, in particular at the fatigue loading stage, Udincreases rapidly and U and Uecurves do not overlap.At the confining stress unloading stage,Uedoes not change much and Udcontinues to increase.Although the axial stress is kept at a constant rate, the axial deviatoric stress on the rock sample increases with decrease of the confining stress.This effect leads to a continuous growth of Ueand continuous conversion of total energy into energy dissipation. After the sample is damaged, Ueis significantly released,and the total energy is converted into the dynamic energy and friction energy of the broken rock block. Fig.10. The variation law of dissipated energy Ud, elastic energy Ue, total energy U, and lateral energy U3 with axial deformation during deformation of marble under confining stress unloading rates of(a)0.02 MPa/s,(b)0.05 MPa/s,(c)0.2 MPa/s and(d)0.4 MPa/s.The numbers labeled 1,2,3,and 4 represent the different loading stages on the energy curve:1 - Static loading stage; 2 - Fatigue loading stage; 3 - Confining stress unloading stage; 4 - Post-failure stage. Fig.11. Evolutions of the total energy,elastic energy,dissipated energy,and circumferential energy against axial strain for the sample under(a)conventional monotonous triaxial test and(b)conventional confining stress unloading test.Labels 1,2,and 3 indicate different loading stages:1-Monotonic loading stage;2-Confining stress unloading stage;and 3- Post-peak stage. As per the experimental scheme, three groups of marble samples are tested.Groups I and II are designed to conduct continuous triaxial loading and triaxial unloading tests in order to compare with the results of the FL-CSU tests. At the critical failure point(which is defined as the sudden failure point in the stress-strain curves, and at this time, the sample failure and the stress-strain curves drop sharply), under the same confining stress of 20 MPa,in the conventional triaxial test, the samples in groups I and II absorb more energy along the axis, while the samples in group II absorb less energy along the axis after unloading. The energy evolution characteristics of marble samples in groups I and II are shown in Fig.11.In Fig.11a,under triaxial continuous loading test,the elastic strain energy is larger than that under FL-CSU conditions;in addition,the dissipated energy is less than that under FLCSU conditions. This result indicates that damage to the samples experiencing fatigue distribution is relatively large,and the energy used to drive crack propagation is larger consequently.In Fig.11b,in the triaxial unloading test and at the critical failure point,it can be also seen that the absorbed elastic energy is larger than that under FL-CSU conditions. Table 3 summarizes the energy evolution of the marble samples in groups I, II, and III. Fig.12 depicts the elastic and dissipated energies of group III at the confining stress unloading point. The results show that the energy values are very close before unloading.Fig. 13 depicts the evolutions of elastic energy, dissipated energy and unloading rate.The Uedecreases with the increase in unloading rate; however, the Udpresents an opposite trend. It indicates that for the sample under low unloading stress rate,the energy used todrive crack initiation and propagation is much more than that in the cases under high unloading stress rate. Table 3 Energy values of U,Ue,Ud,and U3(in MJ/m3)for the marble samples under various loading and unloading paths. Fig. 12. Evolutions of elastic energy and dissipated energy before confining stress unloading point. Fig. 13. Change rule of dissipated energy and elastic energy with confining stress unloading rate. The failure morphology of marble samples can only be observed at the external surface. However, by using the highresolution X-ray CT technique, the crack pattern inside the sample can be observed (Van Geet et al., 2000; Viggiani et al., 2004;Lenoir et al., 2007). In this study, the post-test CT scanning is performed on the marble samples in group III. The fractured marble samples after FL-CSU tests are performed by X-ray CT scanning with a scanning interval of 10 mm (the smaller the scanning interval, the better the crack pattern that can be captured), and five slices are observed for each sample. The reconstructed CT images show low-density regions that can be observed in response to cracks, as seen in Fig.14. The cracks are identified and extracted using a series of digital imaging methods(Wang et al., 2018a, b). As shown in Fig. 14, the crack pattern is strongly influenced by the unloading rate. For all the tested samples under FL-CSU test, the failure morphology of the marble sample is controlled by the interbedded structure of the rocks,and almost all the cracks are parallel to the interbedded layer. The failure mode is,however,different for the marble as it is related to the confining stress when the sample fails. For the sample under an unloading rate of 0.02 MPa/s, the applied confining stress almost drops to zero, which is characterized by a tensile failure mode. For the samples under unloading rates of 0.05 MPa/s,0.2 MPa/s, and 0.4 MPa/s, they exhibit the shear failure mode.Influenced by the interbedded structure, the failure surfaces are along the interbedded layer. Interestingly, the crack pattern is affected by the applied unloading rate - the crack density and scale decrease with an increase in unloading rate. The crack pattern indicates that the deformation of marble is affected by its internal structure. An index of simulated fracture degree, defined as the ratio of the total crack area to the sample’s cross-sectional area, is used to indicate the size of the crack in the marble sample. The degree of fracture for the tested rock sample is 0.111,0.0681, 0.0409, and 0.0327, respectively. To simulate the stress disturbance condition of mining rocks subjected to FL-CSU paths, triaxial compression tests that include fatigue loading and then confining stress unloading conditions are performed. Previous studies discussed the triaxial loading and unloading paths and also the fatigue dynamic paths on rocks (e.g.Guo et al.,2017;Zhao et al.,2014;He et al.,2015;Li et al.,2017;Xu et al., 2017). However, the FL-CSU paths are not well considered.Actually, during mining exploitation (metal and nonmetal mines),the surrounding rock mass often undergoes stress distribution with cyclic loading before excavation. The accumulation of damage caused by stress disturbances (such as blasting, variation and earthquake) is critical to the stability of underground rock engineering.In this study,we investigated the strength,deformation and fracture behaviors of marble under cyclic loading and confining stress unloading.The experimental results confirm the influence of cyclic loading and unloading on rock damage and energy evolution.For samples experiencing fatigue loading and at the critical failure point, the elastic energy is lower than that of the samples under continuous triaxial compression;furthermore,the dissipated energy used to drive crack initiation and propagation is also relatively low.Although the axial, lateral and volumetric deformations are varied due to the heterogeneity of marble,the damage accumulation after cyclic loading is roughly the same.The damage at the second loading stage ensures the comparison of the effect of unloading rate at the third stage on the fracture characteristics of marble. Fig.14. Reconstructed CT images of the marble samples after FL-CSU tests. Fig. 15. Relationships between confining stress and elapsed time during sample deformation under different confining stress unloading rates. Fig. 16. Relationships of elastic strain energy, dissipated energy and fracture degree with confining stress unloading rate. For the samples in group III in this context, the energy dissipation and release are greatly affected by the unloading rate,which then influences how the crack pattern after sample failure forms.For a sample with a low confining stress unloading rate,the damage and cracking behaviors can fully develop in marble, the absorbed energy during the first and second loading stages is transferred mostly to the sample, and the crack dimension is relatively large. However, for a sample under a high unloading rate,a relatively small part of the absorbed energy is transferred to generate cracks in the marble. The elastic energy at the critical failure point is the largest compared to that in other cases. The energy release at the post-peak stage of the third stages is much more severe compared to that in the uniaxial compression stress paths (Munoz et al., 2016; Munoz and Taheri, 2017b), and the stored elastic energy releases suddenly at high confining stress when rock fails. On the post-peak stress-strain curves, Munoz et al. (2016) proposed an energy-based brittleness index to clarify the fracture mechanism of rock, and it was proved to be better than the brittleness obtained from pre-peak stage. For the experimental data,the brittleness evaluation for rock subjected to the FL-CSU conditions at the post-peak stage can be utilized using the method of Munoz et al. (2016) in further studies. The energy release and dissipation characteristics can be well clarified at the third loading stage for the FL-CSU paths. X-ray CT scanning shows the influences of the unloading rate on the energy evolution and fracture degree.One can see from the CT images that a different failure mode for marble samples under different unloading rates is found, and the failure mode of marble can also be inferred from the changes between confining stress and elapsed time, as plotted in Fig. 15. This result shows that the confining stress almost drops to zero for the sample under the minimum unloading rate, the sample is close to the uniaxial compression state, and the failure mode is typically of tensile failure. The other samples are still under the triaxial compression stage,and the failure mode is typically of shear failure.In Fig.16,the dissipated energy shows a consistent changing trend as that of the fracture degree, both decreasing with increasing unloading rate.However,the stored elastic energy shows an opposite trend to the fracture degree and dissipated energy. This implies that failure of the sample under a high unloading rate is more severe, and the stored energy is suddenly released at the critical failure point. This study investigates the effects of triaxial confining stress unloading rate on the fracturing evolution behaviors of marble samples that undergo triaxial fatigue loading and unloading conditions. The test results reveal the macro- and mesomechanism of unloading rate on the deformation, damage and energy evolution characteristics of marble. The following conclusions can be drawn: (1) For marble that experiences FL-CSU conditions, it is shown that the volumetric strain increases more quickly than that under conventional triaxial deformation.The fatigue loading causes damage to the marble and damage accumulation has a large impact on the plastic deformation and also the final crack morphology. (2) The confining stress unloading rate in triaxial conditions affects the dissipation and release of energy.Compared with the conventional triaxial test,the accumulated strain energy of the marble samples in FL-CSU tests is a kind of larger elastic energy, as more strain energy is needed to drive damage evolution and crack propagation.It is observed that dissipated energy decreases and elastic energy increases with increasing unloading rate. (3) From the energy evolution results during the FL-CSU tests,the micro-fracturing process is found to be the main cause of rock failure. This result suggests that the larger damage begins during the cyclic fatigue loading stage. The damage accumulation and the associated fracture behaviors are the key to predict the stability of rock mass,such as rockburst or rock collapse. (4) CT imaging after the FL-CSU tests reveals different crack patterns for the marble samples. The CT images show a different failure mechanism, and the crack pattern in the marble is influenced by the unloading rate. It is also shown that the fracture degree corresponds well with energy dissipation and release. It is suggested that the confining stress unloading rate has potential influences on energy evolution and associated macroscopic fracture morphology. Declaration of competing interest The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper. Acknowledgments The authors would like to thank the editors and the anonymous reviewers for their helpful and constructive comments. This study was supported by National Key Technologies Research & Development Program (Grant No. 2018YFC0808402), State Key Laboratory for GeoMechanics and Deep Underground Engineering, China University of Mining and Technology (Grant No. SKLGDUEK1824),and the Fundamental Research Funds for the Central Universities(Grant No.FRF-TP-20-004A2).






3.4. Energy evolution during the test


3.5. Post-test CT imaging analysis


4. Discussion



5. Conclusions
 Journal of Rock Mechanics and Geotechnical Engineering2020年6期
Journal of Rock Mechanics and Geotechnical Engineering2020年6期
- Journal of Rock Mechanics and Geotechnical Engineering的其它文章
- Ultrasonic imaging of microscale processes in quartz gouge during compression and shearing
- Influence of degree of interlock on confined strength of jointed hard rock masses
- Crack dynamic propagation properties and arrest mechanism under impact loading
- Micro-annulus generation under downhole conditions: Insights from three-dimensional staged finite element analysis of cement hardening and wellbore operations
- Excavation of underground research laboratory ramp in granite using tunnel boring machine: Feasibility study
- Strength and dilatancy behaviors of deep sands in Shanghai with a focus on grain size and shape effect
