刚性挡墙位移土压力的数学拟合公式研究综述

张常光 单冶鹏 高本贤 吴凯

摘 要：在研究土压力与挡墙位移关系时，可采用数学拟合方法表征土压力随挡墙位移的变化规律。数学拟合方法常以静止土压力、主/被动土压力为基础，通过构造数学函数来描述挡墙位移土压力，但所采用的数学函数形式各不相同。将挡墙位移土压力的数学拟合公式按函数形式分为：三角函数、指数函数、双曲线函数、幂函数、S型函数以及其他函数等6大类，总结了位移土压力数学拟合公式的特点与不足，并指出需进一步研究的方向。结果表明：数学拟合公式的主要差异在于函数形式选择和待定参数及取值不同，导致了数学拟合公式的多样性与研究的广泛性。合理实用的位移土压力数学拟合公式需具备3方面特征：边界条件与初值满足、参数含义明确以及能反映挡墙与土体之間的相互作用。在试验方面，应持续对挡墙不同位移模式开展针对性研究，并进行黏性土、非饱和土、湿陷性黄土、膨胀土等的土压力试验;在理论计算方面，应加强位移土压力数学拟合公式间对比分析，探究各自的合理性及适用性，揭示土压力与挡墙位移关系的内在机理。拓展对非饱和土挡墙的位移土压力研究，完善参数选择、模型验证，以加快工程应用进程。

关键词：挡墙位移;位移土压力;数学拟合公式;位移模式;非饱和土

中图分类号：TU432 文献标志码：R 文章编号：2096-6717（2020）06-0019-12

Abstract： Variation laws of earth pressure accounting for the displacement of a retaining wall can be well described by mathematical fitting， which is usually based on the earth pressure at rest or the active and passive earth pressures to illustrate the displacement-earth pressure of retaining walls through constructing various mathematical functions. This study subdivides displacement-dependent earth pressure formulations into six categories according to different functional forms， including trigonometric， exponential， hyperbolic， power， sigmoid and other functions. Characteristics and deficiencies of displacement-dependent earth pressure formulations are summarized， and future researches are provided. The findings of this study show that main differences of mathematical fitting are attributed to determination of function forms as well as undetermined parameters as well as their magnitudes， which results in the uncertainty of mathematical fitting and the generallity of research. A reasonable and practical mathematical fitting function has three features： boundary condition and initial value satisfied， parameters with clear meaning and representing the interaction between a retaining wall and soils. In terms of test studies， it is necessary to perform targeted research on different movement modes of a retaining wall， and model tests of earth pressure are conducted on clay， unsaturated soil， collapsible loess， expansive soil， among others. In terms of theoretical calculations， displacement-dependent earth pressure formulations using different mathematical fitting functions are compared to explore their rationality and applicability as well as to reveal intrinsic mechanisms between earth pressure of a retaining wall and its displacement. Displacement-dependent earth pressure of a retaining wall in unsaturated soil needs to be paid more attention. The choice and measurement of different parameters are improved and validated by model tests in order to accelerate the process of engineering applications for mathematical fitting functions.

Keywords：displacement of retaining walls; displacement-dependent earth pressure; mathematical fitting; movement mode; unsaturated soil

土压力大小及其分布在挡墙和地下设施设计中占有十分重要的地位，Coulomb、Rankine基于挡墙平移极限状态建立了土压力公式并形成两类经典极限土压力理论，文献[1-4]对经典极限土压力理论进行了扩展研究。经典极限土压力的形式简单、物理意义明确，至今仍被广泛应用。但要达到极限土压力的状态，所对应的挡墙位移多数超过实际设计允许位移，特别是对于被动状态，土压力的极限平衡状态往往不会出现。因此，经典极限土压力理论不能反映土压力随挡墙位移变化的中间性态，有必要研究土压力的整个变化过程以及挡墙位移效应的影响。

土压力是土体与挡墙相互作用的结果，不仅与土体性质、挡墙高度、挡墙刚度、时间效应等有关，还与挡墙的位移有着密切关联，这些因素对土压力的影响程度各不相同，不现实也没必要把所有影响因素一起考虑，笔者将重点探讨刚性挡墙位移对某点处土压力的影响。文献[5-11]的土压力模型试验结果均表明：挡墙位移效应（包括位移状态、位移模式和位移大小）对土压力的影响显著，且不同位移模式下土压力沿深度均为非线性分布。

在研究土压力与挡墙位移关系的计算方法中，可采用数学函数来表征土压力随挡墙位移的变化关系。笔者针对挡墙位移土压力的众多数学拟合公式，依据函数形式的不同分为三角函数、指数函数、双曲线函数、幂函数、S型函数以及其他函数等6大类，总结这些数学拟合公式的特点与不足，并指出进一步研究的方向。

1 土压力及挡墙位移效应

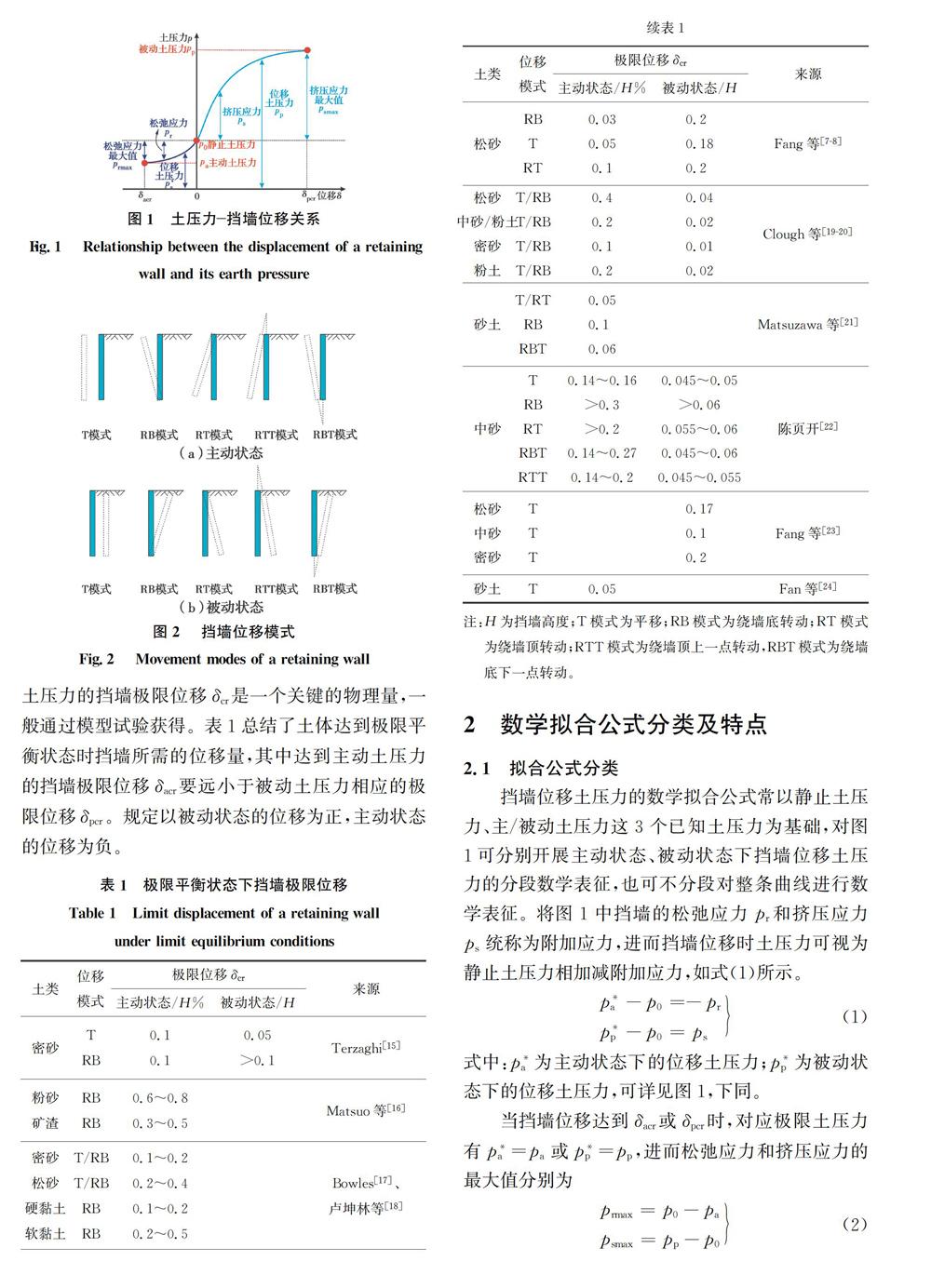
文献[12-14]指出土压力随挡墙位移变化而变化，实际挡墙土压力介于静止土压力和极限土压力之间，并与挡墙位移存在非线性关系，如图1所示。挡墙不发生移动时的土压力为静止土压力p0，背离土体移动时挡墙土压力随之衰减并终止于主动极限状态，相应的土压力衰减称为松弛应力pr、主动极限状态土压力为主动土压力pa即土压力的最小值。同理，朝向土體一侧移动时挡墙土压力随之增长并终止于被动极限状态，相应的土压力增长称为挤压应力ps、被动极限状态土压力为被动土压力pp即土压力的最大值。

除图1挡墙位移状态（主动、被动）、位移大小显著影响土压力以外，图2中的挡墙位移模式也对土压力具有重要影响。当刚性挡墙发生平移而处于非极限状态时，挡墙沿深度方向各点的位移相同，即研究某点处土压力与挡墙位移的函数变化关系可用于挡墙上的所有点，这使得挡墙位移土压力求解大为简化，故现有文献采用数学拟合方法研究挡墙位移土压力p*时均基于挡墙的平移T模式。

在位移土压力的数学拟合公式中，对应主/被动土压力的挡墙极限位移δcr是一个关键的物理量，一般通过模型试验获得。表1总结了土体达到极限平衡状态时挡墙所需的位移量，其中达到主动土压力的挡墙极限位移δacr要远小于被动土压力相应的极限位移δpcr。规定以被动状态的位移为正，主动状态的位移为负。

2 数学拟合公式分类及特点

2.1 拟合公式分类

挡墙位移土压力的数学拟合公式常以静止土压力、主/被动土压力这3个已知土压力为基础，对图1可分别开展主动状态、被动状态下挡墙位移土压力的分段数学表征，也可不分段对整条曲线进行数学表征。将图1中挡墙的松弛应力pr和挤压应力ps统称为附加应力，进而挡墙位移时土压力可视为静止土压力相加减附加应力，如式（1）所示。

可以看出：位移函数kδ1、kδ2、kδ3的量纲为1，对应位移函数kδ的3种构造方式，用于描述土压力随挡墙位移的变化关系。当构造位移函数的方式确定后，可选用不同形式的数学函数对位移函数进行表征，并着重研究其待定参数及取值。因土体类型复杂且土压力数据少，某种数学拟合公式仅在文献个别土体类型下的预测效果较好，不同土体类型下的拟合效果不尽一致，故数学拟合公式的适用性难以准确评价。但构造位移函数最突出的特点就是每个数学拟合公式都有较为明确的函数形式，决定挡墙位移土压力曲线的变化趋势。

因此，按照文献所用拟合函数的不同数学形式，将位移土压力的代表性数学拟合公式大致分为6大类。需说明的是，如果同时涉及2种函数形式，则以主函数形式进行分类，并按出现年代依次列出;若位移函数在主动状态、被动状态中采用同一函数，则用一个公式表示整条曲线;本文研究对象为刚性挡墙，未考虑挡墙自身的变形影响。

2.1.1 三角函数

2.2 拟合公式特点

结合挡墙位移土压力数学拟合公式的构造方式及函数形式分类可知，同类数学拟合公式保留了该类函数的数学特征，继而土压力呈现相似的变化趋势。现从数学拟合公式的目的、构造方式、合理性以及参数确定等方面，对代表性位移土压力公式进行总结：

1）这6类位移土压力公式的目的相同，即采用某一种数学函数描述挡墙在表1极限状态范围内土压力随挡墙位移的变化规律，可获得挡墙非极限状态下的位移土压力，故数学拟合方法的途径明了、简单易用，不过多探究挡墙位移土压力的内在机理，在工程设计中已得到一定应用。

2）构造位移土压力的数学拟合公式存在4种方式，前3种以静止土压力、主/被动土压力为基础，通过附加应力作比或已知土压力直接作比定义了无量纲的位移函数，尤以附加应力作比的构造方式应用最为广泛，可分别对主动状态、被动状态下挡墙的位移土压力进行分段数学表征，也可不分段对整条曲线进行数学表征。以已知土压力直接作比的构造方式，需综合考虑位移土压力的整体变化规律。

3）结合图1和6类位移土压力公式的数学特征可知，合理实用的位移土压力数学拟合公式需具备3方面特征：边界条件与初值满足、参数含义明确以及能反映挡墙与土体之间的相互作用。位移土压力公式的适用性需经多种类型土体工程实测、模型试验和数值模拟的系统性验证。

4）位移土压力数学拟合公式重点表征了刚性挡墙位移对土压力的影响，其待定参数常与土体内摩擦角或黏聚力等土性因素有关，部分参数需结合工程实测或模型试验确定;土体流变时间效应、基坑开挖空间效应以及多因素耦合作用等影响，一般是借助不同类型的数学函数相复合得以实现。

5）位移土压力数学拟合公式大多基于某一类明确具体的函数形式，其差异主要体现在函数形式选择和待定参数及取值不同，导致了数学拟合公式的多样性、可选性，同时也表明当前位移土压力数学拟合方法研究的广泛性与非系统性，这实际反映的是对挡墙位移土压力的认知局限性。

3 存在的不足及进一步研究方向

目前，数学拟合方法在土压力与挡墙位移关系研究中已占据重要地位，但土压力的影响因素众多且错综复杂，使得很多数学拟合公式仍停留在理论层面，存在研究不足并需进一步完善。

1）6类数学拟合公式重点描述了土压力随挡墙位移的变化特征，很大程度上未对墙后土体、挡墙以及土体扰动的相互作用进行阐述，仅针对土压力有限数据做了宏观规律性对比验证，虽在某些条件下说明了数学拟合公式的正确性，但只适用于对比所用的特定土，对其他土体的拟合效果不尽理想。

研究方向：应将挡墙位移土压力的形成机理、物理解释和理论分析与数学拟合方法相结合。另外，数学拟合公式提出后，需经大量土压力实测、试验或模拟的适用性验证，进而界定公式的适用范围、应用步骤，或以分段函数形式获得更具针对性的拟合公式。加强各数学拟合公式间的比较和参数定量化描述。

2）土压力是土体与挡墙相互作用的结果，其他影响因素，如温度、时空效应、施工工序、渗流等，有时一同归并为对挡墙位移的影响，这样虽然可简化实际问题，但并不一定客观。图2挡墙位移模式对土压力大小及分布具有显著影响，而数学拟合方法却均假定挡墙为平移T模式。

研究方向：应针对不同位移模式的挡墙，建立相应的位移土压力数学拟合公式，并适当兼顾其他因素的影响。例如，邾祝融等[68]考虑开挖顺序及时间因素，将时空效应影响与位移函数相复合。如何将其他因素影响也转化成数学函数表达，是对现有位移土压力数学拟合公式进行修正的一个重要方向。

3）位移土壓力数学拟合公式大多针对的是干土或饱和土，表1中模型试验的土体也多为干砂、密砂、粉砂、粉土和矿渣，鲜有黏性土，针对不良特殊土体（湿陷性黄土、膨胀土等）的研究也不充分，且尚未开展基于非饱和土力学原理的挡墙位移土压力研究。

研究方向：开展各类土体挡墙土压力的模型试验或数值模拟以及现场试验，特别是黏性土、非饱和土、湿陷性黄土、膨胀土等。工程实践中遇到的土体大多为非饱和土，与饱和土最大的区别是基质吸力的存在及其产生的吸附强度，可构建基于非饱和土力学原理的挡墙位移土压力数学拟合公式。

4）已有的土体真三轴试验结果表明[69-70]，中间主应力对土体强度的影响明显。土体强度的提高必然会对挡墙土压力产生全方位影响，包括墙后土体滑裂面、土压力大小和分布，甚至是挡墙的位移模式。现有位移土压力的数学拟合公式均没有体现中间主应力的影响，获得的土压力难以反映土体实际强度。

研究方向：对墙后土体开展真三轴试验研究，探讨中间主应力对土体黏聚力及内摩擦角的影响规律，或者基于合理考虑中间主应力影响的土体真三轴强度准则与土体常规轴对称压缩试验，获得平面应变条件下土体的真实强度参数用于位移土压力计算，并加强对墙后土体滑裂面和挡墙位移模式的试验观测。

4 结论

1）挡墙位移土压力常以静止土压力、主/被动土压力为基础，存在4种数学函数构造方式，尤以第1种附加应力作比构造位移函数的应用最为广泛，可相应对主动或被动状态下挡墙的位移土压力进行分段数学表征，也可不分段对整条曲线进行数学表征，目的都是更准确地描述土压力随挡墙位移的变化规律。

2）将挡墙位移土压力数学拟合公式按函数形式分为6大类：三角函数、指数函数、双曲线函数、幂函数、S型函数以及其他函数，可利用某类数学函数应具备的3方面特征，确定其适用范围，以便更好地表达土压力随挡墙位移变化的非线性特征。

3）应将挡墙位移土压力的形成机理、物理解释和理论分析与数学拟合方法相结合，加强位移土压力数学拟合公式间的对比分析，探究各自的合理性及适用性。对挡墙不同位移模式开展全面分析，并进行黏性土、非饱和土、湿陷性黄土、膨胀土等的土压力试验和中间主应力效应研究。

参考文献：

[1] TERZAGHI K. Theoretical soil mechanics [M]. New York： John Wiley & Sons， 1943.

[2] ROWE P W， PEAKER K. Passive earth pressure measurements [J]. Géotechnique， 1965， 15（1）： 57-78.

[3] 顾慰慈. 挡土墙土压力计算[M]. 北京： 中国建筑材料工业出版社， 2001.

GU W C. Calculation of earth pressure on retaining wall [M]. Beijing： China Building Materials Press， 2001.（in Chinese）

[4] HAN S， GONG J X， ZHANG Y Q. Earth pressure of layered soil on retaining structures [J]. Soil Dynamics and Earthquake Engineering， 2016， 83： 33-52.

[5] TERZAGHI K， PECK R B， MESRI G. Soil mechanics in engineering practice [M]. New York： John Wiley & Sons， 1996.

[6] SHERIF M A， FANG Y S， SHERIF R I. ka and k0 behind rotating and non-yielding walls [J]. Journal of Geotechnical Engineering， 1984， 110（1）： 41-56.

[7] FANG Y S， ISHIBASHI I. Static earth pressures with various wall movements [J]. Journal of Geotechnical Engineering， 1986， 112（3）： 317-333.

[8] FANG Y S， CHEN T J， WU B F. Passive earth pressures with various wall movements [J]. Journal of Geotechnical Engineering， 1994， 120（8）： 1307-1323.

[9] 岳祖润， 彭胤宗， 张师德. 压实粘性填土挡土墙土压力离心模型试验[J]. 岩土工程学报， 1992， 14（6）： 90-96.

YUE Z R， PENG Y Z， ZHANG S D. Centrifuge model tests on lateral pressure on walls retaining compacted clayey backfill [J]. Chinese Journal of Geotechnical Engineering， 1992， 14（6）： 90-96.（in Chinese）

[10] 杨斌， 胡立强. 挡土结构侧土压力与水平位移关系的试验研究[J]. 建筑科学， 2000， 16（2）： 14-20.

YANG B， HU L Q. Test study on relationship between lateral earth pressure acting on retaining structures and horizontal displacement [J]. Building Science， 2000， 16（2）： 14-20.（in Chinese）

[11] 徐日庆， 陈页开， 杨仲轩， 等. 刚性挡墙被动土压力模型试验研究[J]. 岩土工程学报， 2002， 24（5）： 569-575.

XU R Q， CHEN Y K， YANG Z X， et al. Experimental research on the passive earth pressure acting on a rigid wall [J]. Chinese Journal of Geotechnical Engineering， 2002， 24（5）： 569-575.（in Chinese）

[12] BANG S. Active earth pressure behind retaining walls [J]. Journal of Geotechnical Engineering， 1985， 111（3）： 407-412.

[13] 殷德順， 王保田， 李向凤. 土压力位移关系的应力路径试验研究[J]. 岩土力学， 2008， 29（10）： 2769-2774.

YIN D S， WANG B T， LI X F. Research on relation between soil pressure and displacement by tests of stress paths [J]. Rock and Soil Mechanics， 2008， 29（10）： 2769-2774.（in Chinese）

[14] MEI G X， CHEN Q M， SONG L H. Model for predicting displacement-dependent lateral earth pressure [J]. Canadian Geotechnical Journal， 2009， 46（8）： 969-975.

[15] TERZAGHI K. Large retaining-wall tests： I-pressure of dry sand [J]. Engineering News Record， 1934， 112（February 1）： 136-140.

[16] MATSUO M， KENMOCHI S， YAGI H. Experimental study on earth pressure of retaining wall by field tests [J]. Soils and Foundations， 1978， 18（3）： 27-41.

[17] BOWLES J E. Foundation analysis and design [M]. New Delhi： McGraw-hill Education （India） Private Limited， 1996.

[18] 卢坤林， 朱大勇， 杨扬. 位移及拱效应下的土压力计算方法[M]. 北京： 国防工业出版社， 2012.

LU K L， ZHU D Y， YANG Y. Calculation method of earth pressure under displacement and arching effect [M]. Beijing： National Defense Industry Press， 2012.（in Chinese）

[19] CLOUGH G W， DUNCAN J M. Finite element analyses of retaining wall behavior [J]. Journal of Soil Mechanics & Foundations Division， 1971， 97（12）： 1657-1673.

[20] CLOUGH G W， DUNCAN J M. Earth pressures [M]. Boston： Foundation Engineering Handbook， 1991.

[21] MATSUZAWA H， HAZARIKA H. Analyses of active earth pressure against rigid retaining wall subjected to different modes of movement [J]. Soils and Foundations， 1996， 36（3）： 51-65.

[22] 陳页开. 挡土墙上土压力的试验研究与数值分析[D]. 杭州： 浙江大学， 2001.

CHEN Y K. Model test and numerical analysis of earth pressures on retaining wall [D]. Hangzhou： Zhejiang University， 2001.（in Chinese）

[23] FANG Y S， HO Y C， CHEN T J. Passive earth pressure with critical state concept [J]. Journal of Geotechnical and Geoenvironmental Engineering， 2002， 128（8）： 651-659.

[24] FAN C C， FANG Y S. Numerical solution of active earth pressures on rigid retaining walls built near rock faces [J]. Computers and Geotechnics， 2010， 37（7/8）： 1023-1029.

[25] 徐日庆， 杨仲轩， 龚晓南， 等. 考虑位移和时间效应的土压力计算方法 [C]//浙江省第八届土力学及基础工程学术讨论会论文集， 上海： 上海交通大学出版社， 1998： 9-14.

XU R Q， YANG Z X， GONG X N， et al. The calculation method of earth pressure considering space-time effort [C]// Proceealing of the Eigth Zhejiang conference on Soil Mechanics and Foundation Engineering， Shanghai Jiao Tong University Press， 1998：9-14.

[26] 张吾渝， 李宁波. 非极限状态下的土压力计算方法研究[J]. 青海大学学报（自然科学版）， 1999， 17（4）： 8-11， 46.

ZHANG W Y， LI N B. The method of earth pressure calculation under the state of unlimited equilibrium [J].Journal of Qinghai University （Natural Sciences）， 1999， 17（4）： 8-11， 46.（in Chinese）

[27] 徐日庆. 考虑位移和时间的土压力计算方法[J]. 浙江大学学报（工学版）， 2000， 34（4）： 370-375.

XU R Q. Methods of earth pressure calculation for excavation [J]. Journal of Zhejiang University （Engineering Science）， 2000， 34（4）： 370-375.（in Chinese）

[28] 张燕凯， 桂国庆， 赵抚民. 深基坑工程中考虑开挖深度和时间效应的土压力计算公式的探讨[J]. 南昌大学学报（工科版）， 2002， 24（1）： 85-89.

ZHANG Y K， GUI G Q， ZHAO F M. Study on time-space effect on earth pressure in deep foundation pits [J]. Journal of Nanchang University （Engineering & Technology）， 2002， 24（1）： 85-89.（in Chinese）

[29] 喻军， 鲁嘉， 龚晓南. 考虑围护结构位移的非对称基坑土压力分析[J]. 岩土工程学报， 2012， 34（Sup1）： 24-27.

YU J， LU J， GONG X N. Earth pressure on supporting structures of asymmetric excavations considering their displacement [J]. Chinese Journal of Geotechnical Engineering， 2012， 34（Sup1）： 24-27.（in Chinese）

[30] 刘一俊. 基于位移-土压力模型基坑支护受力变形分析[D]. 长沙： 湖南大学， 2018.

LIU Y J. Analysis of force and deformation of foundation pit support based on displacement-earth pressure model [D]. Changsha： Hunan University， 2018.（in Chinese）

[31] 陈页开， 徐日庆， 杨晓军， 等. 基坑工程柔性挡墙土压力计算方法[J]. 工业建筑， 2001， 31（3）： 1-4.

CHEN Y K， XU R Q， YANG X J， et al. A new method calculating earth pressure on flexible structures for excavation work [J]. Industrial Construction， 2001， 31（3）： 1-4.（in Chinese）

[32] 田培先. 考慮位移非线性影响的深基坑土压力计算方法研究[D]. 广州： 广东工业大学， 2007.

TIAN P X. Research on the soil pressure calculation method to think of the nonlinear effect of displacement in deep foundation pit [D]. Guangzhou： Guangdong University of Technology， 2007.（in Chinese）

[33] 李德贤. 考虑位移影响的朗肯土压力计算模型[J]. 土工基础， 2008， 22（5）： 48-50.

LI D X. A calculation model of Rankine earth pressure considering displacement [J]. Soil Engineering and Foundation， 2008， 22（5）： 48-50.（in Chinese）

[34] 赵新益. 非极限状态下刚性墙主动土压力的模拟[J]. 土工基础， 2009， 23（4）： 69-72.

ZHAO X Y. Simulation on active earth pressure of rigid wall under non-limit state [J]. Soil Engineering and Foundation， 2009， 23（4）： 69-72.（in Chinese）

[35] 柯才桐， 陈奕柏， 高洪波， 等. 考虑支护结构位移影响的非极限土压力计算模型及应用[J]. 水电能源科学， 2013， 31（11）： 127-131.

KE C T， CHEN Y B， GAO H B， et al. Calculation model of unlimited earth pressure and its application considering displacement effect of supporting structure [J]. Water Resources and Power， 2013， 31（11）： 127-131.（in Chinese）

[36] 陆瑞明， 赵锡宏. 挡土拱圈的内力和变形计算[J]. 岩土工程学报， 1997， 19（6）： 36-42.

LU R M， ZHAO X H. Computation of inner force and deformation of arch retaining structure [J]. Chinese Journal of Geotechnical Engineering， 1997， 19（6）： 36-42.（in Chinese）

[37] DUNCAN J M， MOKWA R L. Passive earth pressures： theories and tests [J]. Journal of Geotechnical and Geoenvironmental Engineering， 2001， 127（3）： 248-257.

[38] 夏唐代， 徐肖华， 孙苗苗， 等. 基坑工程中双曲线土压力模型研究[J]. 地下空间与工程学报， 2009， 5（5）： 893-896， 955.

XIA T D， XU X H， SUN M M， et al. Analysis of hyperbolic earth pressure model for excavation engineering [J]. Chinese Journal of Underground Space and Engineering， 2009， 5（5）： 893-896， 955.（in Chinese）

[39] 姜志强， 孙树林， 李磊. 基坑开挖中土压力计算模型探讨[J]. 河海大学学报（自然科学版）， 2003， 31（3）： 303-306.

JIANG Z Q， SUN S L， LI L. Calculation model for earth pressure during foundation pit excavation [J]. Journal of Hohai University （Natural Sciences）， 2003， 31（3）： 303-306.（in Chinese）

[40] 姚海明. 优化方法在地下工程设计中的应用[D]. 上海： 同济大学， 2004.

YAO H M. Application of optimization method in underground engineering design [D]. Shanghai： Tongji University， 2004.（in Chinese）

[41] 胡志平， 姚海明， 罗丽娟. 考虑位移的非极限土压力计算[J]. 西安科技大学学报， 2005， 25（3）： 296-300.

HU Z P， YAO H M， LUO L J. Calculation of unlimited earth pressure [J]. Journal of Xian University of Science and Technology， 2005， 25（3）： 296-300.（in Chinese）

[42] 张文慧， 田军， 王保田， 等. 基坑围护结构上的土压力与土体位移关系分析[J]. 河海大学学报（自然科学版）， 2005， 33（5）： 575-579.

ZHANG W H， TIAN J， WANG B T， et al. Relationship between earth pressure on support structure of foundation pits and displacement of earth mass [J]. Journal of Hohai University （Natural Sciences）， 2005， 33（5）： 575-579.（in Chinese）

[43] 易南概， 吴大群， 谢志， 等. 基于支护结构位移计算土压力的方法[J]. 沈阳建筑大学学报（自然科学版）， 2005， 21（1）： 26-28.

YI N G， WU D Q， XIE Z， et al. A method of lateral earth pressure calculation consequent upon displacement for excavation [J]. Journal of Shenyang Jianzhu University （Natural Sciences）， 2005， 21（1）： 26-28.（in Chinese）

[44] 李青. 软土深基坑变形性状的现场试验研究[D]. 上海： 同济大学， 2008.

LI Q. Field test research on deformation behavior of deep foudation pit in soft clay area [D]. Shanghai： Tongji University， 2008.（in Chinese）

[45] 刘国彬. 软土卸荷变形特性的试验研究[D]. 上海： 同济大学， 1993.

LIU G B. Experimental research on the soft clay under unloading [D]. Shanghai： Tongji University， 1993.（in Chinese）

[46] 胡晓虎. 上海软土卸荷的时间效应试验研究[D]. 上海： 同济大学， 1999.

HU X H. Experimental research on the Shanghai soft clay under unloading considering time effort[D]. Shanghai： Tongji University， 1999.（in Chinese）

[47] 卢坤林， 杨扬. 非极限土压力的近似计算方法[J]. 合肥工业大学学报（自然科学版）， 2008， 31（6）： 946-949.

LU K L， YANG Y. Approximate mathematic expression of unlimited earth pressure [J]. Journal of Hefei University of Technology （Natural Science）， 2008， 31（6）： 946-949.（in Chinese）

[48] 卢坤林， 杨扬. 考虑位移影响的主动土压力近似计算方法[J]. 岩土力学， 2009， 30（2）： 553-557.

LU K L， YANG Y. Approximate calculation method of active earth pressure considering displacement [J]. Rock and Soil Mechanics， 2009， 30（2）： 553-557.（in Chinese）

[49] 刘昌清， 陶志平， 彭胤宗. 墙背填料为砂性土的短卸荷板式挡土墙离心模型试验[J]. 西南交通大学学報， 1996， 31（1）： 81-85.

LIU C Q， TAO Z P， PENG Y Z. Centrifugal model test of retaining wall with short relieving plate on sand backfill [J]. Journal of Southwest Jiaotong University， 1996， 31（1）： 81-85.（in Chinese）

[50] 李海珍， 李永刚， 王河. 基于有限元的挡土墙主动土压力分析及经验计算[J]. 水电能源科学， 2017， 35（9）： 93-96.

LI H Z， LI Y G， WANG H. Active earth pressure analysis and experience calculation of retaining wall based on finite element [J]. Water Resources and Power， 2017， 35（9）： 93-96.（in Chinese）

[51] 聂宗泉， 汪清河， 周奇， 等. 软土基坑主动区土压力与位移关系实测研究[J]. 施工技术， 2011， 40（24）： 43-46.

NIE Z Q， WANG Q H， ZHOU Q， et al. Monitoring and research on relationship between earth pressure in active area and displacement of foundation excavation in soft soil [J]. Construction Technology， 2011， 40（24）： 43-46.（in Chinese）

[52] 韩勤， 徐立， 刘干斌， 等. 软土基坑土压力测试及分布模式研究[J]. 宁波大学学报（理工版）， 2014， 27（4）： 127-132.

HAN Q， XU L， LIU G B， et al. Earth pressure measurement and distribution for soft soil foundation pit [J]. Journal of Ningbo University （Natural Science & Engineering Edition）， 2014， 27（4）： 127-132.（in Chinese）

[53] 伍露露. 挡土结构附加土压力与位移关系的试验研究与数值分析[D]. 天津： 天津大学， 2016.

WU L L. An experimental study and numerical analyses of the relationship between additional earth pressure and displacement for retaining structures [D]. Tianjin： Tianjin University， 2016.（in Chinese）

[54] 吴伟强. 基坑支护结构计算的位移土压力法[J]. 建筑结构， 1997（9）： 19-21.

WU W Q. The calculation method of earth pressure considering displacement in supporting of foundation pit [J]. Building Structure， 1997（9）： 19-21.（in Chinese）

[55] 唐孟雄， 赵锡宏. 基坑开挖挡土墙的有限元模型[J]. 同济大学学报（自然科学版）， 1998， 26（5）： 516-521.

TANG M X， ZHAO X H. Finite element model of retaining wall in deep excavation [J]. Journal of Tongji University （Natural Sciences）， 1998， 26（5）： 516-521.（in Chinese）

[56] 高文华， 杨林德. 软土深基坑围护结构变形的三维有限元分析[J]. 工程力学， 2000， 17（2）： 134-141.

GAO W H， YANG L D. Three-dimensional finite element analysis of deformation of the retaining structure of deep foundation pit in soft-clay [J]. Engineering Mechanics， 2000， 17（2）： 134-141.（in Chinese）

[57] 蒋鹏， 杨淑碧. 支护结构位移对侧土压力影响的计算分析[J]. 地质灾害与环境保护， 1998， 9（2）： 61-64.

JIANG P， YANG S B. Computation of effect of supporting structure displacement upon earth pressure [J]. Journal of Geological Hazards and Environment Preservation， 1998， 9（2）： 61-64.（in Chinese）

[58] 谭志勇， 王余庆， 周根寿. 软土地层中考虑土与结构共同作用的支护结构设计计算方法[J]. 工业建筑， 1999， 29（5）： 8-11，15.

TAN Z Y， WANG Y Q， ZHOU G S. Design and calculation method of support structure taking account of soil-structure interaction in soft soil stratum [J]. Industrial Construction， 1999， 29（5）： 8-11，15.（in Chinese）

[59] 梁洪水. “兩墙合一”支护结构的变形及土压力研究[D]. 昆明： 昆明理工大学， 2017.

LIANG H S. Deformation and earth pressure research on the supporting structure of dual-purpose diaphragm wall [D]. Kunming： Kunming University of Science and Technology， 2017.（in Chinese）

[60] 梅国雄， 宰金珉. 现场监测实时分析中的土压力计算公式[J]. 土木工程学报， 2000， 33（5）： 79-82.

MEI G X， ZAI J M. A new formula for earth pressure [J]. China Civil Engineering Journal， 2000， 33（5）： 79-82.（in Chinese）

[61] NI P P， MANGALATHU S， SONG L H， et al. Displacement-dependent lateral earth pressure models [J]. Journal of Engineering Mechanics， 2018， 144（6）： 04018032.

[62] 梅国雄， 宰金珉. 考虑位移影响的土压力近似计算方法[J]. 岩土力学， 2001， 22（1）： 83-85.

MEI G X， ZAI J M. Earth pressure calculating method considering displacement [J]. Rock and Soil Mechanics， 2001， 22（1）： 83-85.（in Chinese）

[63] 赵建平， 余闯， 陈国兴， 等. 考虑位移影响的Rankine土压力模型及有限元计算分析[J]. 工程勘察， 2006， 34（10）： 13-16， 21.

ZHAO J P， YU C， CHEN G X， et al. Rankine earth presure model and FEM analysis considering displacement. [J]. Journal of Geotechnical Investigation & Surveying， 2006， 34（10）： 13-16， 21.（in Chinese）

[64] 张春会， 郭海燕， 于广明. 考虑挡墙位移非线性影响的土压力计算模型[J]. 岩土力学， 2006， 27（Sup1）： 171-174.

ZHANG C H， GUO H Y， YU G M. Earth pressure calculating model considering the nonlinear influence of displacement of retaining wall [J]. Rock and Soil Mechanics， 2006， 27（Sup1）： 171-174.（in Chinese）

[65] 罗伟， 刘章顺， 沈红萍， 等. 考虑变形的土压力计算新方法[J]. 中国水运， 2009， 9（10）： 161-162.

LUO W， LIU Z S， SHEN H P， et al. A new method for calculating earth pressure considering deformation [J]. China Water Transport， 2009， 9（10）： 161-162.（in Chinese）

[66] 卢国胜. 考虑位移的土压力计算方法[J]. 岩土力学， 2004， 25（4）： 586-589.

LU G S. A calculation method of earth pressure considering displacement [J]. Rock and Soil Mechanics， 2004， 25（4）： 586-589.（in Chinese）

[67] 王培清， 何强， 其米旺姆， 等. 桥台填土土压力计算方法[J]. 工业建筑， 2010， 40（Sup1）： 600-603.

WANG P Q， HE Q， QI M W M， et al. Earth pressure calculation of abutment fill [J]. Industrial Construction， 2010， 40（Sup1）： 600-603.（in Chinese）

[68] 邾祝融， 刘影， 刘保战. 深基坑开挖中考虑时空效应的土压力计算方法研究[J]. 四川建筑科学研究， 2014， 40（4）： 153-155.

ZHU Z R， LIU Y， LIU B Z. The research of time-space effect on earth pressure in deep excavation [J]. Sichuan Building Science， 2014， 40（4）： 153-155.（in Chinese）

[69] ZHANG C G， CHEN X D， FAN W， et al. A new unified failure criterion for unsaturated soils [J]. Environmental Earth Sciences， 2015， 74（4）： 3345-3356.

[70] 方瑾瑾， 邵生俊， 李荣， 等. 真三轴条件下Q3黄土的屈服特性研究[J]. 岩石力学与工程学报， 2016， 35（9）： 1936-1944.

FANG J J， SHAO S J， LI R， et al. Yield characteristics of Q3 loess in true triaxial tests [J]. Chinese Journal of Rock Mechanics and Engineering， 2016， 35（9）： 1936-1944.（in Chinese）

（編辑 章润红）