Study of Shaking Table Test on Dynamic Response Characteristics and Failure Mechanism of the Loess Slope1

PU Xiaowu,WANG Lanmin,WANG Ping,CHAI Shaofeng and XU Shiyang

1)Key Laboratory of Loess Earthquake Engineering,Lanzhou Institute of Seismology,CEA,Lanzhou 730000,China

2)Key Laboratory of Mechanics on Disaster and Environment in Western China,Lanzhou University,Ministry of Education,Lanzhou 730000,China

3)College of Civil Engineering and Mechanics,Lanzhou University,Lanzhou 730000,China

The natural loess that covers the ground surface has good stability due to its low water content.However,when violent earthquakes occur,the strong dynamic stress generated in the slope may induce landslide disasters with different sizes.In this paper,a large-scale shaking table model test is used to reveal the dynamic response and instability failure process of the loess slope.The test results show that different parts of the slope have different vibration characteristics and the first natural frequency in the model increases with the increase of the slope height.The response acceleration of different parts may change due to the coupling relationship between the spectral characteristics of input wave and the natural frequencies of different parts of slope,suggesting the characteristics of regional differential dynamic response.Under the condition of different dynamic response,stress state and boundary conditions of different parts of slope,a rapid microstructural damage,cumulative residual deformation evolution,and tension-shear coupling instability failure process may appear at the top of the slope with the strong dynamic response associated with the increase of dynamic loading intensity.The Sd values presented in this paper may reflect soil damage and slope instability and failure.

Key words:Loess slope;Shaking table;Dynamic response;Microstructure damage;Failure

INTRODUCTION

In nature,loess that covers the earth's surface is a porous multi-phase system composed of solid,liquid and gas phases.Loess in China has been developing as aeolian sediment since Quaternary.Because of its unique material source,special occurrence environment and stress history of low overburden pressure,the pore structure of metastable scaffolds is well developed and has strong seismic vulnerability(Gao Guorui,1994;Wang Lanmin,2003).When it is subjected to moderately strong earthquakes,its microstructures may collapse,leading to devastating geotechnical disasters(Zhang Zhenzhong,1999).

Loess,the cover widely distributed in north China,is the substandial carrier of the production,living and development of people in loess area.As the main area of loess distribution in China,Loess Plateau is characterized by broad width and thickness,strong geotectonic activities and frequent strong earthquakes.In addition to its hilly-gullied and fragmented topography,the combination of several other factors has resulted in widely distributed,frequent,and serious geological hazards in Loess Plateau.(Zhang Zhenzhong,1999;Wu Weijiang et al.,2006).Among them,landslide is the most destructive one which always causes huge casualties and property losses.The TongweiMS7.5 earthquake in 1718 and the HaiyuanMS8.5 earthquake in 1920 triggered massive loess landslides in the Loess Plateau,leading to serious disasters to the people in those areas(Wang Lanmin,2003).

Based on the practical requirement of preventing and mitigating earthquake-induced loess landslides,numerous experts and scholars have conducted research works regarding earthquakeinduced loess landslides and have made great progresses.For example,the dynamic characteristics of loess,the distribution characteristics,morphological characteristics,sliding mechanism and stability evaluation of landslides,etc.(Chen Yongming et al.,2006;Huang Yahong,1998;Wang Lanmin et al.,1999).At present,the main methods for loess seismic landslide research include field investigation,indoor dynamic triaxial test,theoretical calculation,numerical simulation and physical simulation.Compared with other methods,largescale shaking table physical simulation test is one of the most powerful means to reveal the dynamic response and failure process of slopes(Lin M.L.et al.,2006;Huang Runqiu et al.,2013).However,in general,the shaking table model test for loess slope is less carried out,and research results are far from meeting the requirements of landslide disaster prevention and control.The high-stability loess slopes with a gradient of 20 degrees or less are often neglected by people in earthquake disaster prevention.In order to distinguish them from those with higher steep loess slope which have gradients of more than tens of degrees,we named them as low angle slope in this paper.

At present,the seismic hazards of these low-angle loess slopes are not well understood by people.In high earthquake-prone areas,many new villages and towns are built near the feet of low-angle slopes.However,the investigation after the earthquake shows that the original slope angle of the loess landslide induced by the earthquake is between several degrees and dozens of degrees,and the slope gradient mostly concentrates within the range of 10°-30°(Zhang Zhenzhong,1999;Wang Lanmin,2003;Chen Yongming et al.,2006).Therefore,we intend to conduct shaking table model test of low-angle natural loess slope,visually reproduce the deformation evolution and instability failure process of loess slope under strong earthquake,study the dynamic response characteristics and deformation evolution law of the slope,and determine the dynamic instability mode and mechanism of the slope.The research results may provide theoretical support for the stability evaluation and disaster prevention of loess seismic landslides.

1 METHODOLOGY

According to the test purpose and actual conditions,a typical Q3loess gentle slope with a height of 7 m and a gradient of 20° in Shanzidun Village of Lanzhou New Distirct is selected as the prototype to establish a generalized model through field survey.The high stability of low angle slopes in natural state renders negligible seismic safety problems as well as less related research.

1.1 Similarity Relation and Material Parameters

The key point of this test simulation is to reproduce the process of deformation,instability and failure of loess slope.Therefore,gravity and resistance similarity are mainly considered,and design similarity relation based on Lin Gao's gravity similarity law(Lin Gao et al.,2000).Besides,the geometric size,cohesion and density similarity ratio are determined as the main control variables.The similarity relationship and the soil material parameters are shown in Table 1.In order to ensure a certain degree of similarity of soil micro-structure between prototype and model(Shao Shengjun et al.,2004),the remolded loess of the prototype site is considered as the model material.

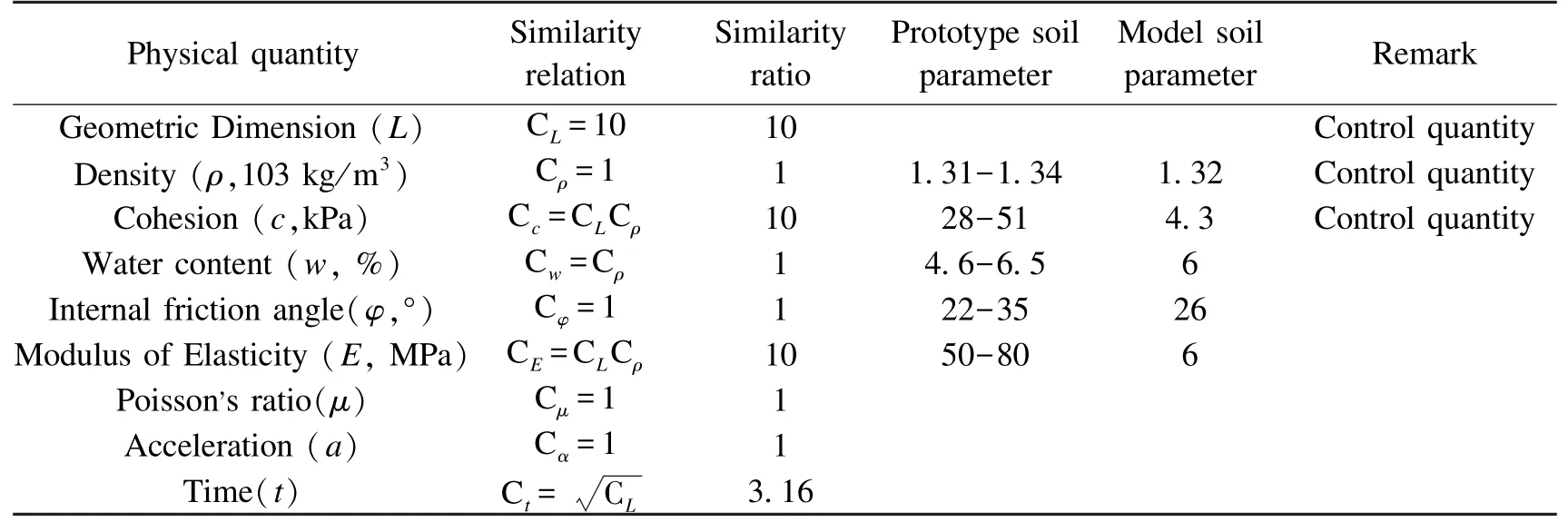
Table 1 Similarity relation and soil parameters

1.2 Input Seismic Waves

Substantial seismic loess landslides mostly occur in the areas with seismic intensity ofⅧdegrees or above(Zhang Zhenzhong,1999),and the slope failure is generally recognized to be caused by horizontal shear waves.Therefore,in this experiment,the NSdirection acceleration time history record(Fig.1)measured at the Minxian Seismic Station during theMS6.6 Minxian-Zhangxian earthquake on July 22nd,2013 is selected.The PGA of the seismic wave is approximately 220 Gal(Ⅷdegrees),which is near-field wave recorded by bedrock base.The loading waves include the original wave(predominant frequency 4.2 Hz)and the compressed wave(predominant frequency 23.06 Hz).The loading mode follows the steps below:The original wave is loaded at initial,followed by the compressed wave with similar amplitudes.Finally,the loading amplitude is increased step by step until the mode is failed.

1.3 Test Equipment

A large bi-directional electro-servo shaking table is used as a loading device(Fig.2).The size of the shaking table is 4 m×6 m,the maximum acceleration is 1.7g,and the frequency range is 0.1-70 Hz.A rigid model box with a length,width and height of 2.82 m×1.42 m×1.1 m is used,its main materials are carbon steel plates and both long sides are plexiglass plates.

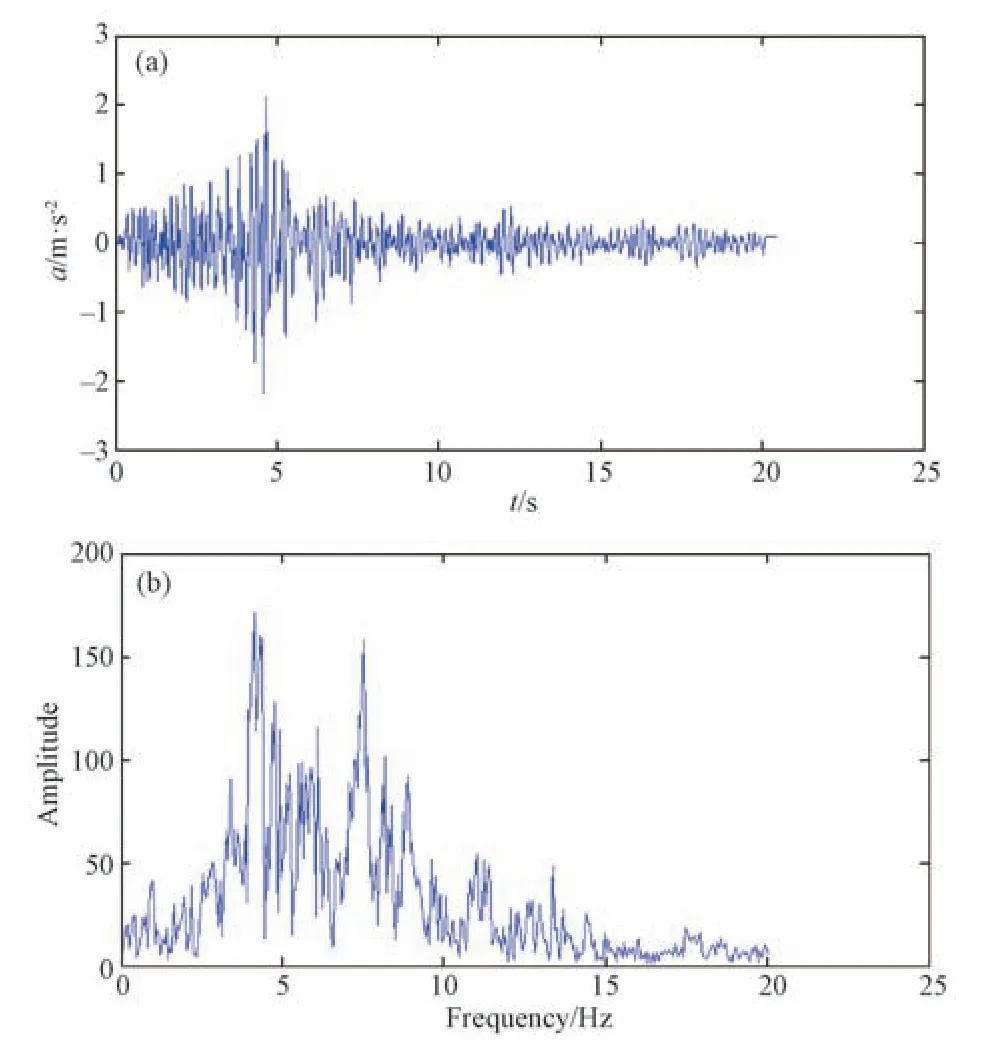
Figure 1 Time history curve and fourier spectrum of loading seismic wave

Figure 2 Large-scale shaking table

1.4 Model Making and Sensors Layout

To reduce the vibration boundary effect,the two 3 cm thick plastic foam boards are placed inside the front and back steel plates of the model box.After crushing and sieving the undisturbed loess,the soil layers of the model are paved and compacted with an interval of 10 cm.Fifteen accelerometers from A1 to E5 are installed,the model size and sensor position are shown in Fig.3.

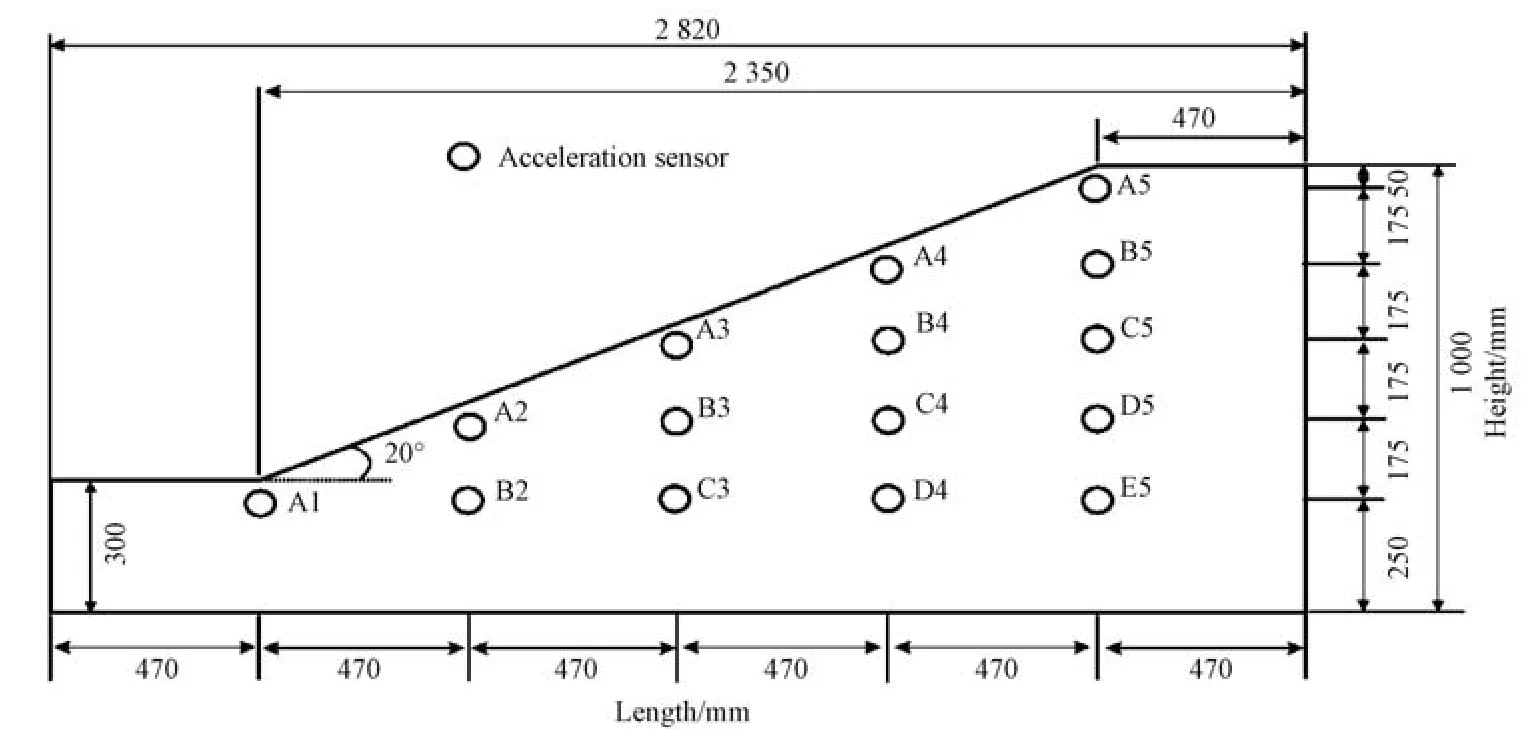
Figure 3 Geometric dimensions of the model and sensors location

2 ANALYSIS OF TEST RESULTS

2.1 Macro Deformation and Failure Phenomena of the Model

As shown in Fig.4,when the dynamic loading intensity is less than 247 Gal,there is no residual deformation that can be identified by naked eyes;when the value equals to 247 Gal,several small cracks appear in the shoulder;when the value equals to 464 Gal,the small cracks are widened and enlarged;when the value equals to 708 Gal,a cut-through tensile crack of approximately 60 cm deep appeared at about 10 cm behind the shoulder,the widest part of the crack is about 2 cm.There are also several large transverse cracks appear in the area below the shoulder and above the middle of the slope surface,the total permanent displacement of the slope shoulder is about 2-3 cm.To conclude,these macro phenomena indicate that the upper part of the model has been failed.

2.2 Spatial-Temporal Variations of Dynamic Characteristics of the Slope Model

2.2.1 Calculation Method of Model Dynamic Characteristics

In the slope model test of the shaking table,the dynamic parameters of the model can be calculated by transfer function(Jiang Liangwei et al.,2010).If the input acceleration isag(t),and the absolute acceleration of the response of thejpoint isaj(t),the relative acceleration of thej-point isarj(t)=aj(t)-ag(t),and its transfer function is:

WhereGxxis the self-power spectrum andGxyis the cross-power spectrum ofarj(t),zjis the position ofjpoint,ωis angular frequency.The frequency corresponding to the peak value of the transfer function spectrum is the natural frequency of the model.

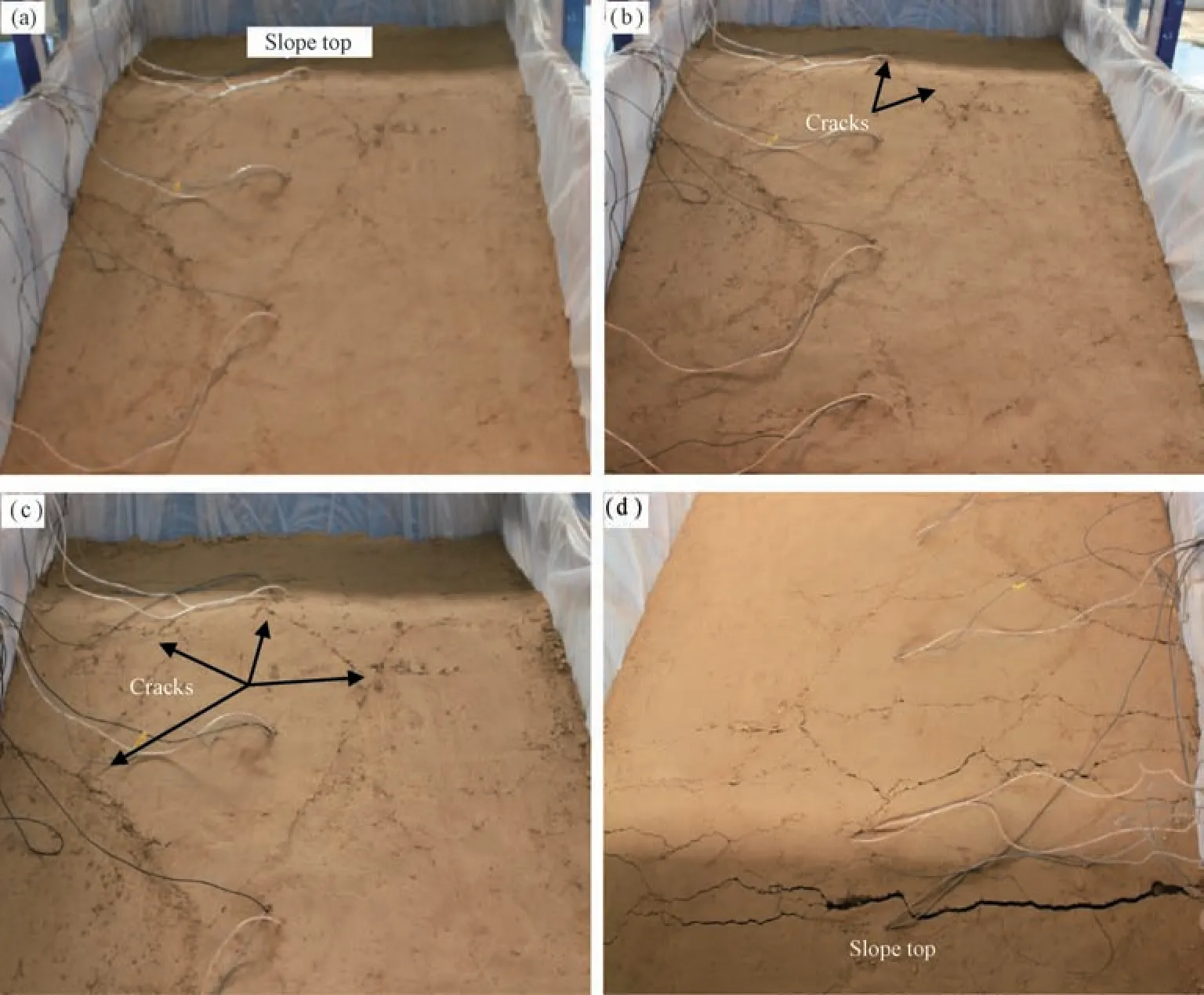
Figure 4 Model photos under different loading conditions

2.2.2 Variation of Natural Frequency with Spatial Positions

Soil cannot be damaged and deformed under the activity of seismic ground motion with a strength of 75 cm/s-2(Wang Yushi et al.,2017).Therefore,the natural frequencies of the slope model obtained in this experiment under 67 Gal seismic ground motion are considered as the natural frequencies of the initial stage of the slope model.

As shown in Fig.5(a),even if the slope is composed of homogeneous loess,different parts of the slope model also have different natural frequencies because of the difference in spatial location.With the increase of elevation,the natural frequency decreases gradually.However,at the same height,the natural frequency reduces with the decrease of the distance to the slope surface.For example,the natural frequencies of A1(slope foot),E5(inside the slope)and A5(slope shoulder)are 81.25 Hz,129.5 Hz and 32.25 Hz respectively.

2.2.3 Variation of Natural Frequency with Dynamic Loading Intensity

With the increase of dynamic loading intensity from 67 Gal to 247 Gal,the natural frequencies of different parts of the model gradually decrease,even though the change is not significant.Under 464 Gal loading condition(Fig.5(c)),the natural frequencies of different parts of the slope model decrease greatly.Under 708 Gal loading intensity(Fig.5(d)),the natural frequency decreases significantly in the vertical tension failure zone from A5 to E5,indicting that the model has been destabilized.

Figure 5 Variation of natural frequency of different parts of the model under different dynamic loading intensity

2.3 Dynamic Response Characteristics

A large number of field investigation and monitoring results show that the amplification effect of seismic ground motion may occur in loess site(Pu Xiaowu,2016).The results of shaking table tests also suggest that the amplification effect of peak ground acceleration(PGA)is extremely significant(Wang Lanmin et al.,2017,2018).

(1)PGA amplification effect of slope topography

Amplification effect is mainly presented by the change of amplification coefficient of PGA,which is the ratio of PGA of response on slope to PGA inputting from shaking table.

Fig.6 and Fig.7 show that along the slope surface up,with the increase of elevation,the amplification coefficient of PGA increases significantly and the most significant amplification effect of PGA appears at the slope shoulder and its adjacent area.

(2)PGA amplification effect of seismic wave of different predominant frequency

In this test,the amplification effect of PGA is significantly different between the original wave(4.2 Hz)and the compressed wave(23.06 Hz)with different predominant frequencies.When the slope model is not destabilized,the compression wave has stronger PGA amplification effect than the original wave.The compressed wave amplification factor at 99 Gal is 5.9 times,while the original wave amplification factor at the similar load condition is only 2.4 times.

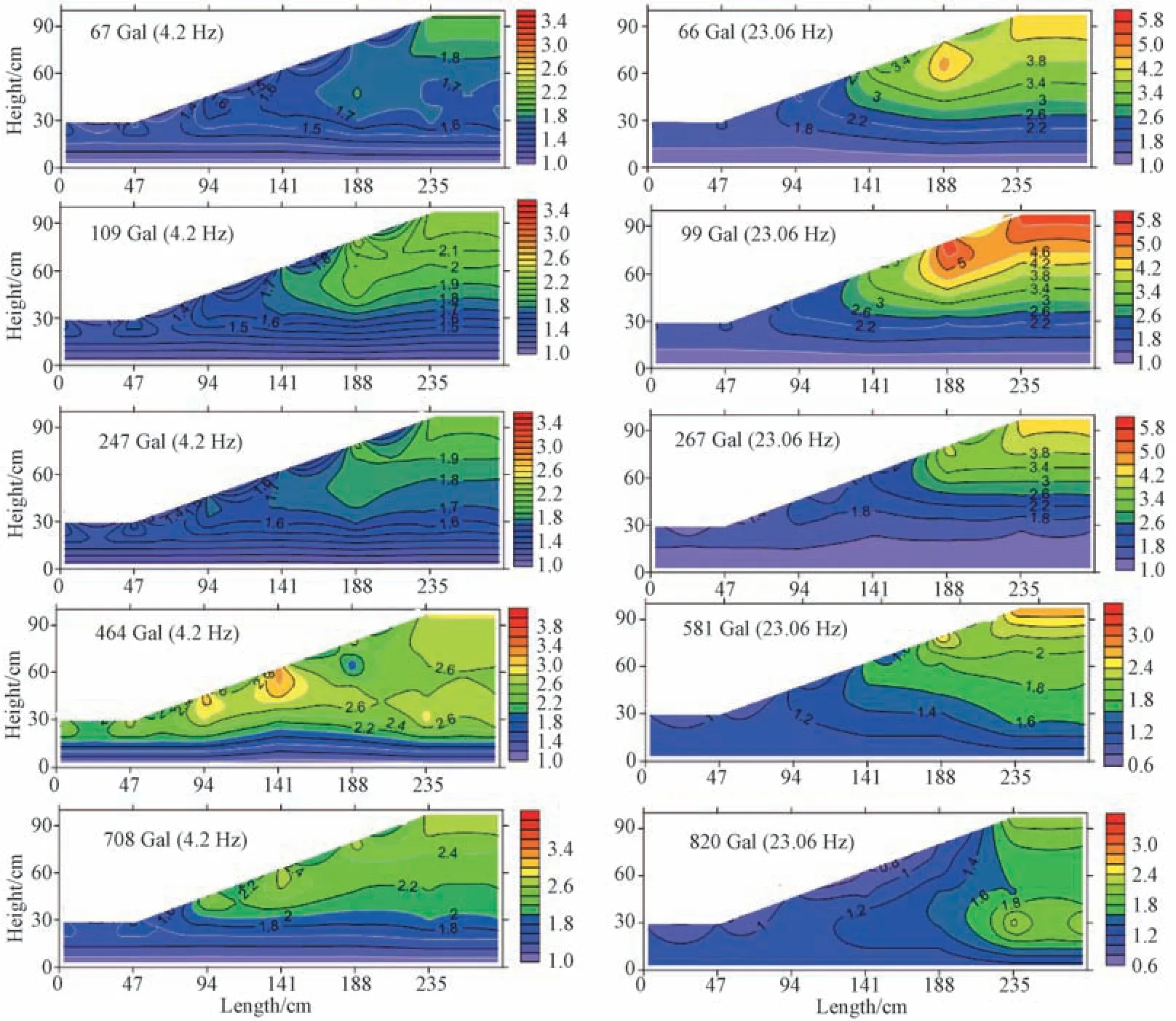
Figure 6 Nephogram of PGA amplification coefficient of the model under different dynamic loading intensity

(3)Slope dynamic response change with dynamic loading intensity

With the increase of dynamic loading intensity step by step,the slope dynamic response of two kinds of input waves has a greatly different variation.Under the activity of the original wave,the slope PGA amplification effect increases with the increase of dynamic loading intensity,and the strongest amplification effect occurs under 464 Gal load condition,and the amplification effect decreases under 708 Gal loading condition.For the compression wave,the slope dynamic response reaches its maximum at 99 Gal,PGA amplification effect attenuates step by step under subsequent loading conditions.

(4)The PGA amplification effect of slopes and change reasons

The dynamic response of slope is the result of the coupling effect of input wave characteristics and slope own dynamic characteristics.Because the natural frequencies of different parts of the slope are different,the dynamic responses on different parts of the slope show different states when the input waves of a certain frequency spectrum,amplitude and duration are transmitted from bottom to top.The amplification effect enhances with the decrease of the difference between the predominant frequency of the input wave and the natural frequency of different parts of the slope.For example,the 23.06 Hz compression wave in this test has stronger PGA amplification effect in the slope top region(31.25 Hz natural frequency).

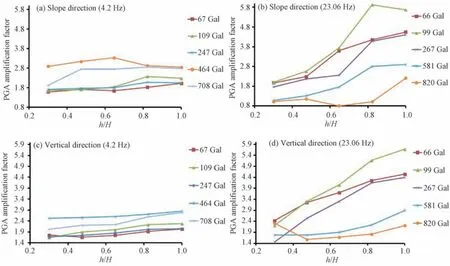
Figure 7 Amplification coefficient varies with model elevation under different dynamic loading intensity

With the increase of loading intensity,the microstructures of slope soil body are gradually damaged under the activity of dynamic stress,the mechanical properties are decreased,and the natural frequencies are changed.The greater the damage of soil microstructures and the faster the natural frequencies decrease in the regions with stronger dynamic amplification effect,such as slope shoulders.When the natural frequency decreases and tends to be the predominant frequency of the input wave,the amplification effect increases,and vice versa.

2.4 Damage Evolution of Slope Soil

Loess is a special kind of soil with well-developed overhead pore micro-structure(Deng Jin et al.,2013;Wang Qiang et al.,2013).The overhead pore structure formed by the overlapping large solid particles belongs to metastable structure system.In addition,the overlapping particles is mainly connected by the contact of the tip of particles,resulting in low dynamic strength.Under the activity of reciprocating dynamic loads such as seismic waves,the weak parts of particle connection are easy to be destroyed and further cause soil damage and deformation(Wang Lanmin et al.,2007;An Liang et al.,2019).When the loess slope is subjected to seismic activities,shear wave transfers from bottom to top in the form of stress wave,causing tension,compression and shear between solid media in different parts of the slope.For the weakly cemented loess with porous support,the damage and deformation of soil microstructures may occur even under lower dynamic stress,which may change the natural frequency of slope soil mass and dynamic response of slope.

In the shaking table model test,the natural frequencies,amplification coefficients and TF spectrum amplitudes of different parts of the slope change with the increase of dynamic loading intensity.The first-order natural frequency and peak value of TF spectrum change regularly with the loading intensity,reflecting that certain damage and deformation of slope soil body may take place.Because the peak value of TF spectrum decreases regularly with the increase of dynamic loading intensity in all loading conditions of model test,and can credibly reflect the damage and deformation of soil body,the normalized peak value of TF spectrum is proposed to express the damage and deformation of soil.The formula is shown below:

whereirepresents thei-th loading condition,Ainiis the TF peak value of the first loading condition,Aultis the TF peak value of the last loading condition,Aiis the peak value of thei-th loading condition,andSdicharaterizes the degree of soil damage of thei-th loading condition.

Figure 8 Change of Sd value characterizing soil damage under different dynamic laoding intensity

As shown in Fig.8,theSdcurves of different locations show different changing patterns with the increase of loading intensity.In the lower parts of slope,no obvious macrodeformation and instability failure phenomena are observed under all loading conditions(Fig.4),indicating that the damage of soil is slight and thus the variation range ofSdcurves of A1 and A2 points is small.In the upper part of the slope,with the increase of dynamic load intensity,obvious tensile and shear failure phenomena appear in macro-scale,especially in 708 gal loading condition.Instability failure appears in the model area andSdvalues of A5,A4 and B5 increases sharply up to 95%.It also shows thatSdcan reflect the damage change of soil body with the increase of dynamic load strength credibly.IfSdvalue reaches 95%,it can be judged as soil failure.If theSdvalue of continuous multi-points of slope reaches 95%,it can be judged as instability failure of slope model.

3 DISCUSSION ON FAILURE MODES AND MECHANISMS OF LOW ANGLE LOESS SLOPE

Natural low-angle loess slope is highly stable under static condition because of low water content,large matrix suction and high shear strength of loess than loess with high water content(Wang Jun et al.,2012;Wang Lanmin,2003).When slope is subjected to seismic activities,in the process of vertical upward propagation where seismic waves propagate from the bottom of slope,seismic wave is transmitted through layers in the form of stress waves due to the interaction between soil particles(Fig.9).As shown in Fig.10,the relative acceleration curves of the soil elements adjacent to C4 show several differences in the spectrum and amplitude(“left”is the difference of acceleration between B3 and C4,“right”is the difference of acceleration between C4 and D5,“up”is the difference of acceleration between B4 and C4,“down”is the difference of acceleration between C4 and D4),indicating that the soil elements in slope is mainly subjected to the strong dynamic shear stress of upper and lower soil elements.Besides,it is also subjected to tensile and compressive stresses of soil elements on the left and right sides.Therefore,based on the relevant study(Wu Shiming,2000),the dynamic equation at C4 point is concluded as follows:

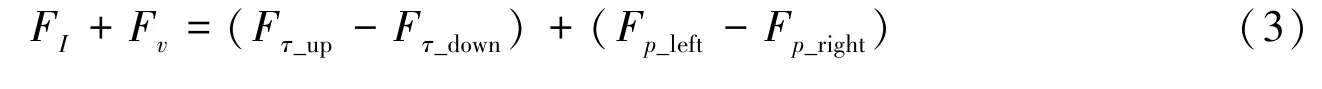
WhereFIis inertia force,Fvis viscous force,Fτ_upandFτ_downare upper and lower surface shear stress,Fp_leftandFp_rightare left and right surface tension and compressive stress.If stress is expressed in the form of displacement,the upper formula is as follows:

In the equations above,∂,v,urepresent acceleration,velocity and displacement,respectively,ρis the oil density,GandEare shear and elastic modulus of soil,cis viscousity damping coefficient.

From Eq.(5),it can be concluded that the soil particles in slope can be pulled,compressed and sheared when seismic wave activities occur.Because of the strong PGA amplification effect of loess slope,the dynamic stress activity increases with the increase of elevation.However,the dynamic shear stress at slope top changes to 0 because the confining pressure changes to 0 at the top of slope,horizontal tension and compression stress become the main activity forces between soil particles.As shown in Fig.9,the stress-strain relationship of loess is closely related to the stress state and water content of loess.When it is in natural state(low water content)and under low confining pressure,brittle failure usually occurs in loess at strain rate of 2%～3%(Wang Lanmin,2003).For the soil body of slope shoulder,when the dynamic loading intensity is increased to a certain extent,brittle tensile failure(Lupsection of Fig.9(a))may occur,seismic ground motion may further trigger a large number of slope cracks,which is confirmed by post-earthquake site surveys(Xu Chong et al.,2013).The soil body of slope shoulder divided by the cracks lacks the pulling force of the soil body of slope back edge.Under the reciprocating activity of seismic ground motion,the swing amplitude may increase,and the pulling and pressing activity between soil particles and the collision with the back edge soil body may become stronger.Also,the soil body of slope shoulder can possibly be further divided into several small blocks.The generation of cracks may transfer the dynamic stress,Lmidsection andLdownsection dynamic shear stress may strengthen,and ultimately,strong dynamic stress reciprocating activity may cause shear failure and induce instability sliding in the upper part of slope.Field investigation shows that the failure mode of top tension crack and shear sliding is one of the main failure modes of landslide induced by Wenchuan earthquake(Huang Runqiu,2009),Historic earthquake investigation in the Loess Plateau shows that tension failure occurs at the top of the slope under seismic inertia force,and then shear sliding occurs under the combined activities of seismic inertia force and gravity.This kind of tension-shear failure is also very common.Numerous experts' numerical and physical simulation experiments also prove the failure mechanism of slope instability caused by the tension-shear coupling effect(Zheng Yingren et al.,2009;Che Ailan et al.,2016).

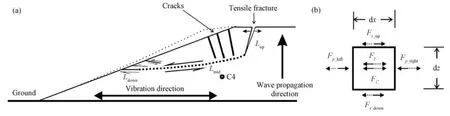
Figure 9 Diagram of stress state and instability failure of slope under the activity of seismic gound motion

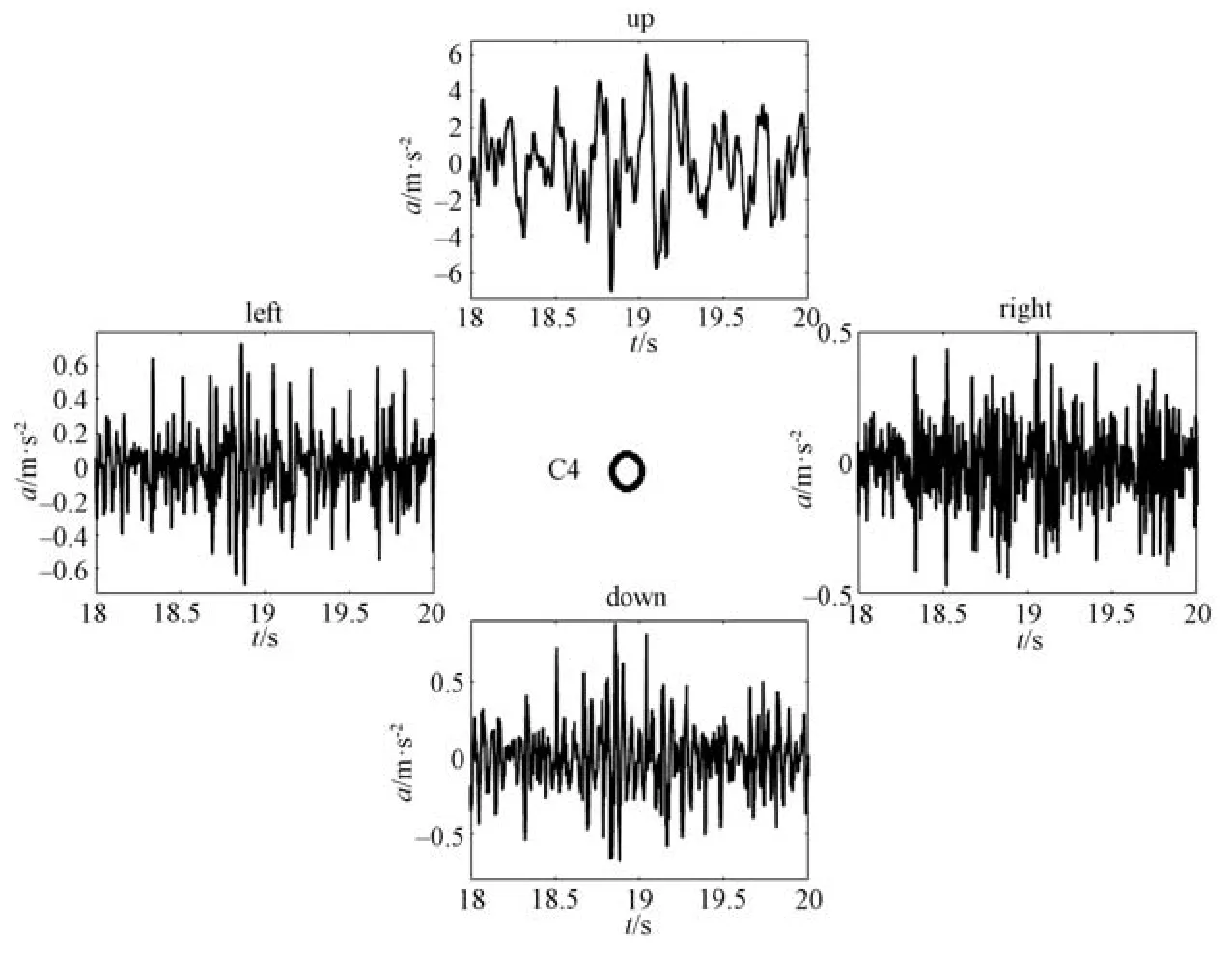
Figure 10 Local time history of acceleration difference between C4 and adjacent points

4 DISCUSSION AND CONCLUSION

(1)When loess slope is subjected to seismic ground motion,soil particle of slope is randomly subjected to strong shearing activities between the vertically adjacent particles.It is also subjected to pulling and pressing forces between horizontal adjacent points.Due to the dynamic amplification effect of loess slope,dynamic activity may increase with the elevation.Because the overburden pressure at the top surface of slope approaches 0,the dynamic shearing stress of slope top also approaches 0 correspondingly.Horizontal tension and compression forces are the main forces between soil particles,thus,tensile failure may occur firstly at the top of slope when loading intensity reaches a certain extent.Under subsequent seismic ground motion,the swing amplitude of the soil body divided by cracks may increase,and the pulling and pressing activities between soil particles and the collision with the back edge soil body may become stronger.The soil body divided by tensile cracks may then be cut apart into smaller blocks.Finally,an unstable slip may occur in the region where the upper part of slope is divided by a large number of cracks,thereby may induce the loess landslide of tensile-shear coupling type.

(2)The dynamic response of slope is the result of the coupling effect of input wave characteristics and slope own dynamic characteristics.Because the natural frequencies of different parts of slope are different,the dynamic responses of different parts of slope show different states when the input waves of a certain frequency spectrum,amplitude and duration are transmitted from bottom to top.The amplification effect may be highly different.The amplification effect is larger when the two kinds of characteristics are more similar.For example,the 23.06 Hz compression wave input in this test has strong PGA amplification effect in the slope top region(31.25 Hz natural frequency).With the increase of loading intensity,the microstructures of slope soil body are gradually damaged with the activity of dynamic stress,the mechanical properties are decreased,and the natural frequencies of different parts of slopes are changed differently.The damage level of soil microstructures increases with the enhancement of the dynamic amplification effect,however,the natural frequency decreases.When the natural frequency decreases and tends to be the main frequency of the input wave,the amplification effect increases and vice versa.

(3)When loess slope is subjected to seismic ground motion,the damage of soil microstructures may occur with lower dynamic stress.The damage of soil body may cause the amplitude of transfer function spectrum to change regularly with the increase of loading intensity,reflecting the degree of damage and deformation of soil body in shaking table test.The expression ofSdproposed in this paper can stably represent the damage state of slope soil body.It can be seen from the test results that when theSdvalue of a certain region reaches 95%,we can determine that the region is instable and may fail.Thus,Sdvalue is also able to determine whether the slope model has been damaged.

(4)Shaking table test has inevitable adverse effects such as scale effect and boundary effect.However,the high similarity between our test results and those of previous landslide field investigating as well as numerical investigating suggests the results are credible.

ACKNOWLEDGMENT

Some colleagues have helped directly and indirectly in the preparation of the paper,particularly Dr.Wang Lili,and Mr.Xu Shuhua,the authors appreciate for their supports and assistances.