非线性保守摆的研究
郑冕 李亮


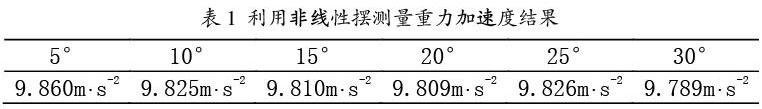
摘 要:文章采用Jacobi椭圆函数描述保守摆动系统的非线性运动学方程,研究了非线性摆的角位移随时间及振幅的变化,计算了不同振幅对应的振动周期,并同实验结果进行比较,发现理论值和实验值符一致。同时用大振幅摆的周期间接测量了重力加速度,测量结果与重力加速度的精确结果9.8m/s2符合得很好。
关键词:非线性;复摆;重力加速度
中图分类号:O314 文献标志码:A 文章编号:2095-2945(2019)14-0023-03
Abstract: In this paper, Jacobi functions were used to describe the nonlinear vibrations of the conservative pendulum and the variation of the angular displacement with time and amplitudes was studied theoretically. The periods under different angular amplitudes were calculated and theoretical results agree with experimental values. Finally, this nonlinear pendulum was used to measure the gravitational acceleration, and the experimental results are in good agreement with the accurate value 9.8m/s2.
Keywords: non-linear; physical pendulum; gravitational acceleration
作為振动的范例,“摆”是最为人熟知的振子,实验室经常用单摆或复摆测量重力加速度。但单摆或复摆是一种线性化模型[1,2],它们具有固定的周期,而实际的摆并不像线性摆那样具有固定的周期,它们的周期与振幅相关。本文将利用Jacobi椭圆函数研究保守的非线性摆动。
1 线性摆和非线性摆
2 非线性摆理论
3 非线性摆周期的测量结果与理论结果
4 结论
非线性摆可以利用Jacobi椭圆函数给出精确解,同时摆动周期也可以给出严格的理论公式。理论分析结果表明,摆动周期随摆角增大而加速上升,只有在摆角十分小时才满足单摆的周期公式,这一结论在实验室中得到精确验证。利用非线性摆的周期公式也可以测量重力加速度,测量结果与精确结果十分接近,可见非线性摆也是一种行之有效的测量重力加速度的方法。
参考文献:
[1]刘正成,李青松,孙迎春.单摆非线性特征的研究[J].物理实验,2018,38(1):56-59.
[2]陈大伟,斯小琴.非线性单摆周期的数值解[J].邵阳师范学院学报(自然科学版),201714(6):1-4.
[3]曹刚,任晓荣,王桂珍,等.单摆的非线性运动[J].山东轻工业学报(自然科学版)200620(2):82-86.
[4]T.V.卡曼,M.A.比奥.工程中的数学方法[M].科学出版社,1962.
[5]王竹溪,郭敦仁.特殊函数概论[M].科学出版社,1979.
[6]黄卫立,龚善初.非线性单摆的微扰解[J].江西师范大学学报(自然科学版),2000,24(2):188-189.
[7]乔文华.大摆幅单摆的运动研究[J].聊城师院学报(自然科学版),2002,15(4):88-89.

