Magnesium nanocomposites:An overview on time-dependent plastic(creep)deformation

M.Haghshenas ,M.Gupta

a Department of Mechanical Engineering,University of North Dakota,ND,USA

b Department of Mechanical Engineering,National University of Singapore,Singapore

Keywords:Mg nanocomposite Creep Strength Reinforcement Nanoparticles

A B S T R A C T Magnesium(Mg)nanocomposites are created w hen nano-size particles are embedded into the Mg(or Mg alloy)matrix.The Mg nanocomposites,cited as high-strength energy-saving materials of future,are a group of emerging materials w ith excellent combination of strength and ductility and superior speci f i c strength property(strength-to-weight ratio).Having said this,Mg nanocomposites are considered as promising replacement for other structural alloys(i.e.aluminum and titanium)wherever low density and high strength are required,i.e.transportation,aerospace,defense,etc.To be able to apply this group of materials for real components,different failure mechanisms at ambient and elevated temperatures under static and dynamic loading condition must be w ell documented.Compared w ith other metals and alloys,rate-dependent plastic deformation(creep),at ambient and elevated temperatures,of these novel materials is not yet well studied w hich seems a tangible lack of knowledge.This is required since the materials in service are often exposed to medium and elevated temperatures and/or static loads for long duration of time and this encourages creep failure on them.To this end,the information and the controlling mechanisms on time/temperature-dependent response of the material need to be developed to be able to predict the response of the Mg nanocomposites w here the materials are under creep conditions.This paper aims at providing an overview on(i)creep-resistant Mg alloys(as matrix)and their chemical compositions,and(ii)responses of the Mg nanocomposites at different creep conditions(time and temperature).The controlling mechanisms contributing to the strength and ductility of the Mg nanocomposites due to the presence of the nanoparticles have been reviewed brie f l y in the present article.In this paper both traditional(uniaxial)and depth-sensing indentation creep of Mg nanocomposites are review ed.Also,some fundamental questions and possible explanations have been raised on the creep characteristics of Mg nanocomposites and the contribution of microstructural features(i.e.grain boundaries,tw ins,precipitates,nanoparticles).This overview article provides a comprehensive summary to understand one of the failure modes(creep)at ambient and elevated temperature in the energy saving Mg nanocomposites that w ould be of interest for those in academia who explore novel nanocomposites.

1.The concept of Mg nanocomposites

When nanoscale(＜100 nm)particles,as reinforcement constituents,are incorporated into a matrix of a metallic material,either a pure metal or an alloy,a metal matrix nanocomposite(MMN)is born.The key feature of the nanoparticles is that they possess great surface to volume ratio w hich noticeably alter their response compared w ith the bulk sized counterparts.The end product is a MMN that could be signi f i cantly stronger than the matrix material.

If the matrix is pure Mg or a Mg alloy,the produced material w ill be called Mg nanocomposite.Compared w ith other types of metal matrix nanocomposites,in recent years,tremendous attentions have been devoted to Mg nanocomposite considering the fact that Mg is the lightest structural metal;it is the sixth most abundant element and constitutes about 2%of the Earth's crust,and it is the third most plentiful element dissolved in seaw ater.However,Mg is not a perfect material.As the lightest metallic material w ith great potential for various applications including transportation,defense and aerospace,Mg and its alloys suffer from low strength and ductility,low creep resistant,and low fatigue life.This is mainly due to the hexagonal close packed(hcp)crystalline structure of Mg w ith limited number of active slip systems at ambient temperature.

Nanocomposites based on the Mg matrix could be a potential solution to the mentioned challenges,w hich have limited the application of Mg and Mg alloys for different critical components.Various types of nanoparticles could be employed toward the manufacturing of the Mg nanocomposites including oxides,carbides,nitrides,borides,and carbonaceous materials(i.e.carbon nanotubes and graphene nanoplatelets).The result of the addition of nanoparticles is a great improvement in properties including mechanical strength and toughness.In a Nature publication by Chen et al.[1]a Mg-4Zn-3Gd-1Ca Mg alloy reinforced w ith 2 w t%Zn O nanoparticles,diameters 90-200 nm,w as introduced that possesses compressive strengths matching/exceeding that of mild steel.

The controlling mechanisms contributing to the strength of Mg nanocomposites due to the presence of the nanoparticles could be listed as follow s[2]:

·Effective load transfer from the matrix to the nanoparticles:The load transfer improvement factor,fLT,is w ritten as-P,w here Vp,and P are the volume fraction of the nanoparticles and porosity in the matrix.

·Hall-Petch strengthening due to grain re f i nement:The Hall-Petch strengthening can be w ritten asw here K is a constant,GMis the shear modulus,b is the burgers vector,Vpand dpare the volume fraction and size of the nanoparticles.

·Orowan strengthening due to the presence of nanoparticles:the improvement factor due to the Orow an strengthening,fOrowan,can be expressed a,w hereλis the inter-particle spacing,and YMis the yield strength of matrix.

·Generation of dislocations due to the thermal expansion mismatch betw een reinforcements and matrix:The improvement factor related to the dislocation density,fDD,in the matrix,can be expressed as fDD,w hereρin w hichρis the enhanced dislocation density due to the difference in the coef f i cients of thermal expansion betw een the reinforcement phase and the matrix(Δα),andΔT is the difference betw een the processing and test temperatures.

In addition to the strength,ductility of Mg nanocomposites could be superior to the Mg matrix.The improvement could be due to[3-7]:

·grain re f i nement(grain boundaries'pinning effect due to the presence of nanoparticles)

·the activation of non-basal slip systems(i.e.＜c+a＞)

·texture modi f i cation(change in crystallographic orientation)

·reduction in the size and sharpness of second phase due to the presence of nanoparticles and therefore reducing the amount of localized stress buildup around the intermetallic particles

·change in the stress state from plane strain to plane stress near the crack tip due to even dispersion of the nanoparticles w ithin the matrix

In particular,ceramic-based nanoparticles(i.e.Al2O3,Y2O3,SiC,SiO2)provide promising results in enhancing strength and ductility,simultaneously.

Fig.1 show s bobble charts of yield strength versus ductility for pure Mg and various Mg nanocomposites produced w ith different manufacturing methods.

The effectiveness of the nanoparticles is such that the amount of material added is normally only betw een 0.25 and 3.00 vol%w hich produces results comparable or even superior to that of metal matrix microcomposites reinforced w ith much larger amount of micron size constituents w ithout any w eight penalty.

Rate-dependent plastic deformation,w hich occurs under stresses low er than the yield stress of materials at ambient and/or elevated temperatures(creep),stands for one of the major failures associated w ith the selection and use of engineering materials for various applications.For instance,poor creep resistance of pure Mg and some Mg alloys is considered as an important barrier for further expansion of these lightweight materials for hightemperature applications in different sectors and components.

As potential structural materials for vehicles,aircraft and military armor,creep and fatigue response of Mg alloys(and therefore Mg matrix nanocomposites)require in-depth investigations in order to understand their rate-dependent characteristics.In recent years,research efforts on the strain rate dependence of the mechanical properties of Mg alloys have signi f i cantly increased[9-20].Conventional Mg alloys soften at about 120°C-150°C[21,22];that is,increase in temperature in Mg and its alloys results in quick loss of strength and limited creep resistance[23-26].Improving the elevated temperature mechanical properties(i.e.creep)has become a critical issue to enable applications of Mg alloys at higher temperatures.Therefore,the primary focus currently is to develop new generation of magnesium nanocomposites,capable of exhibiting good combination of thermal and mechanical properties.Hence,researchers attempt to manufacture Mg-matrix nanocomposites,employing different types and volume fraction of the nanoparticles,to obtain such lightw eight materials along w ith acceptable strength and ductility even at elevated temperatures[27-34].How ever,creep mechanisms for the Mg nanocomposite materials,as emerging high-strength lightw eight materials,have not been w ell documented w hich seems a tangible lack of science.This paper aims at providing an overview on creep-resistant Mg alloys and creep response of some Mg-nanocomposites that have been studied so far.Some fundamental questions that must be considered w hen studying creep response of Mg nanocomposites are as follow s:

·Would Mg and Mg alloys reinforced w ith the nanoparticles(i.e.ceramic particles)provide enhanced creep resistance compared w ith the matrix materials?

·What are the contributions of the nanoparticles and the matrix(i.e.Mg and/Mg alloy)precipitates on creep response of the nanocomposites?Would they change w ith temperature and strain rate?

·How do tw ins in the Mg matrix interact w ith the nanoparticles and the precipitates during creep testing at ambient and elevated temperatures?How these interactions vary w ith temperatures?

·What are the possible effect of nanoparticles'volume fraction(i.e.0.5 to 3.0 vol%)on the questions raised above?

2.Creep resistant Mg alloys(matrix material)

Among different Mg alloys,those based on Mg-Al,Mg-Sn-Ca,and Mg-Sc systems are the ones applicable for moderate to high temperature uses.For instance,addition of alloying elements such as Ca,Sr,Si,Sn and rare earth elements in the Mg-Al system could produce thermally stable intermetallic compounds(i.e.Mg2Si,Al11RE3,(Mg,Al)2Ca,Al2Ca,Mg-Al-Sr,Al-Ce-Mg-Ca and Mg14Nd2Y,etc.).These intermetallics directly affect the creep resistance of the alloy through strengthening the matrix(i.e.impede dislocation motion and/or grain boundary sliding).

Table 1 show s a list of creep resistant Mg alloys for various nonambient temperatures.For producing creep-resistant Mg nanocomposites,these alloys could be considered as promising matrix materials[35].

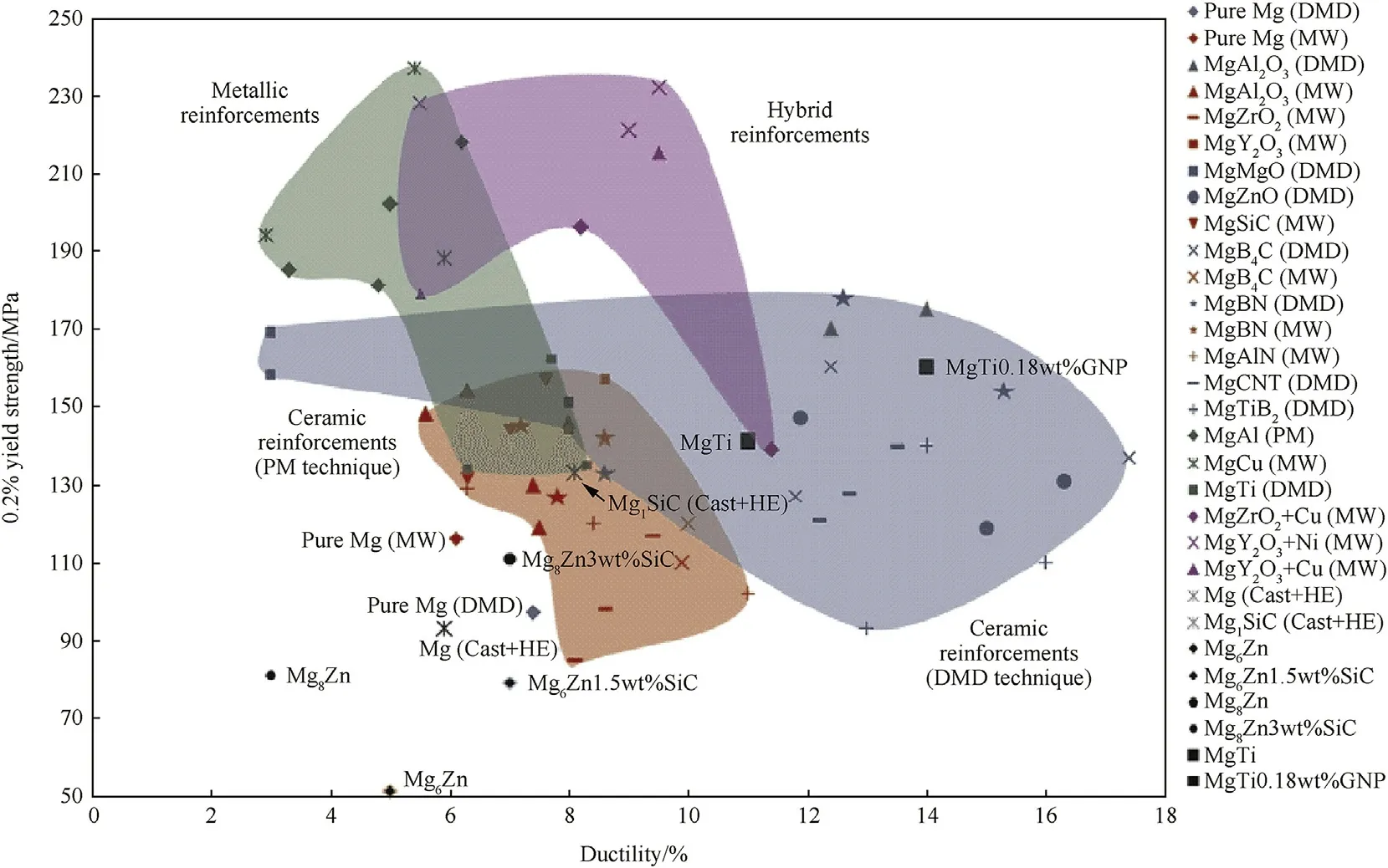
Fig.1.Bubble chart show ing yield strength versus ductility for various types of Mg nanocomposites made using disintegrated melt deposition(DMD),microwave sintering(MW)assisted pow der metallurgy(PM),and cast and hipped(Cast+HE)techniques[8].

3.Uniaxial tensile(conventional)creep test of Mg nanocomp osites

In a conventional creep test,steady-state creep rate can be w ritten as:

w here A is a constant,σis the(uniaxial)stress,n is the stress exponent,T is the absolute temperature and Q is an activation energy.The stress exponent,n,can be obtained from a plot of ln˙ε against lnσ.The value of Q can be found from a plot of ln˙εagainst 1/T.

A limited number of researchers have studied creep response of Mg composites(nano-and micro-)through conventional creep testing approach.Ferkel and Mordike[36]studied creep behavior of Mg reinforced w ith 3 vol%of SiCnanoparticlesproduced by pow der metallurgical technique.They used uniaxial tension testing w ith strain rate of 3.3×10-4s-1at 200 and 300°C.They prepared one set of samples just mixed(matrix pow der and nanoparticles)and a separate set of samples milled.They show ed that the nanocomposite material and the pure Mg sample have completely different creep responses.For instance,they showed that grain boundary sliding mechanism of creep is limited in the nanocomposite materials,compared w ith the pure Mg,due to the presence of the SiCnanoparticles decorating and pinning the matrix grain boundaries.

The minimum creep ratesare plotted in Fig.2 and it can be seen that composite synthesized using milling show s the best creep resistance,even if the grain size is smaller compared to the others,w hich should enhance grain boundary sliding(GBS).The SiC particles are potentially responsible for hindering this phenomenon.Comparing the creep results w ith those of creep-resistant magnesium alloys(WE43,WE54 and QE22),it is evident that even a small amount of nano-SiCleads to similar or even better creep resistance.

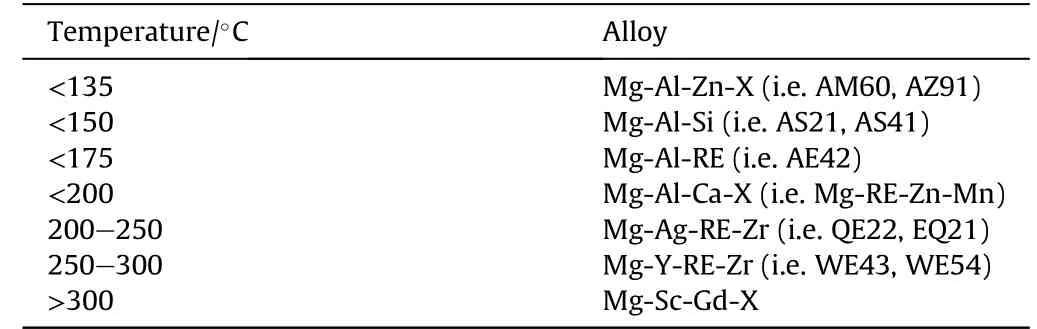
Table 1Creep resistant Mg alloys.

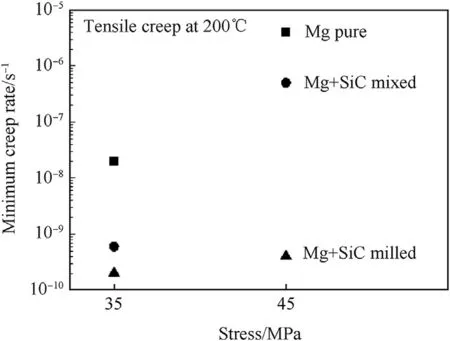
Fig.2.Minimum creep rates of creep tests performed at 200°C,35 MPa and 45 MPa[36,37].

Fig.3.log（dε/d t） versus logσplot of the steady-state creep rates recorded at different stresses in tests carried out at 200°C(circles)and 300°C(triangles)for the milled Mg-3vol%n-SiCcomposite[36].

Fig.3 show s a pow er law representation recorded at different stresses in tests carried out at 200 and 300°Cfor the milled Mg-SiC composite.Note that the date points in particular for the creep test carried out at 300°Callow s only a rough estimation of n.From the plot the stress exponents w ere determined to be～10 and～3 for the experiments done at 200 and 300°C,respectively.

Katsarou et al.[38]studied creep response of magnesium alloy Elektron 21(El21)reinforced w ith AlN nanoparticles(El21+1%w t AlN Mg nanocomposites)manufactured by ultrasound-assisted stirring.They did compression creep tests at 240°C at constant stresses betw een 70 and 200 MPa on lever-arm testing machine.Fig.4 show s a double logarithmic plot of minimum creep rate versus applied stress from tests performed at 240°C on the El21 alloy and the El21+AlN nanocomposite.A bilinear trend is observed;at low stresses up to 120 MPa the stress exponent n is 4.8 and 5.0 for Elektron 21 and Elektron 21+AlN,respectively.At higher stresses it is 6.6 and 8.3,respectively.The reinforced Elektron 21 show s a lower minimum creep rate compared to the unreinforced material at all applied stresses.The difference decreases w ith increasing stress.

Han and Dunand[39]studied creep of magnesium strengthened w ith high volume fractions of micron-sized yttria dispersoids(dispersion-strengthened-cast magnesium,DSC-Mg).Their test material w as magnesium w ith 1μm grain size containing 30 vol%of 330 nm yttrium particles.Constant-load compression tests w ere performed in air at engineering stresses from 7 to 125 MPa and at temperatures from 573 to 723 K(T/Tm=0.62-0.79).Fig.5 show s the minimum strain rate˙εas a function of the true stressσ(calculated at the strain w here the minimum strain rate w as achieved),plotted in a double logarithmic manner according to the general pow er-law constitutive creep equation.

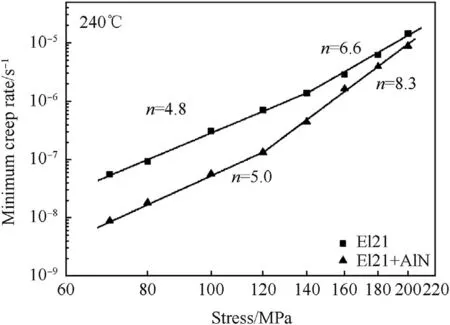
Fig.4.Double logarithmic plot of minimum creep rate versus applied stress from tests performed at 240°Con the El21 alloy and the El21+AlN nanocomposite[38].

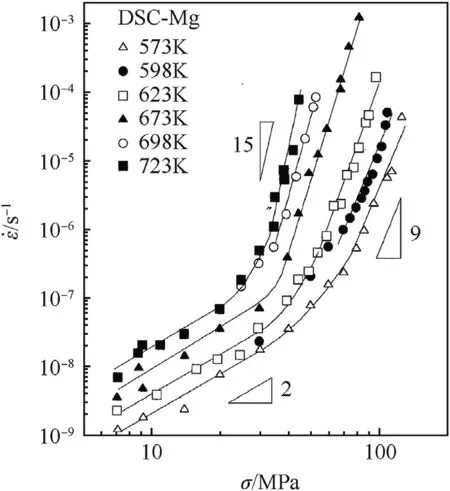
Fig.5.Strain rate as a function of true stress for DSC-Mg at temperatures from 573 to 723 K[39].

They observed that in the low-stress regime(σ＜30 MPa),the apparent stress exponent is about 2 at all temperatures and the apparent activation energy is 48 k J·mol-1,about half the activation energy of grain boundary diffusion in pure magnesium.In the highstress regime(σ＞34 MPa),the apparent stress exponent increases w ith increasing temperatures from 9 to 15 betw een 573 and 723 K.The apparent activation energy increases w ith increasing stress from 229 k J·mol-1at 40 MPa to 325 k J·mol-1at 80 MPa.

Ganguly and Mondal studied the microstructure and impression creep behavior of an AZ91-2.0Ca-0.3 Sb alloy and its three nanocomposites i.e.,AZ91-2.0Ca-0.3 Sb-x SiC(x=0.5,1.0 and 2.0 w t%)[40].They conducted the impression creep tests in the stress and temperature range of 300-480 MPa and 448-523 K,respectively for a duration of 2 h.They observed better creep resistance in the nanocomposites than the unreinforced AZ91-2.0Ca-0.3 Sb alloy w ith the optimum creep resistance in the nanocomposite pertaining to 2.0 w t%SiCnanoparticles(Fig.6).They also reported the controlling creep mechanism for the alloy and nanocomposites as dislocation climb controlled by pipe diffusion.

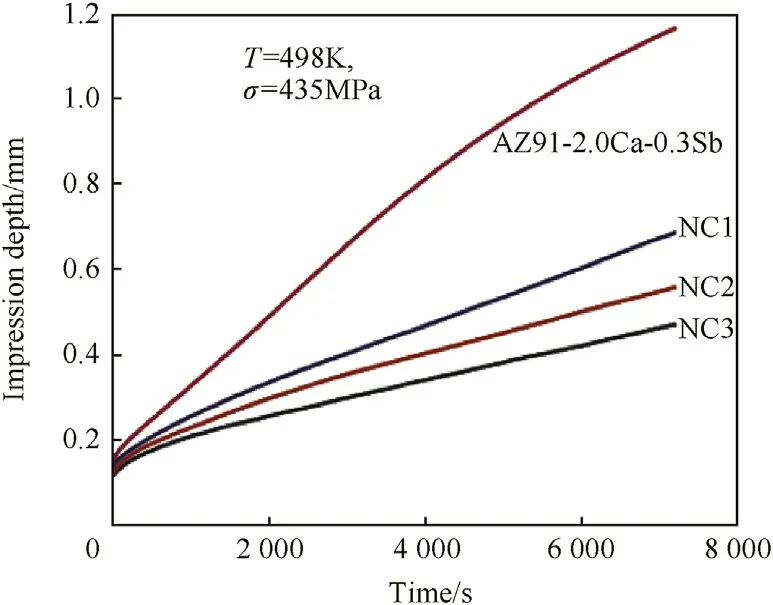
Fig.6.Typical impression creep curves for the alloy(AZ91-2.0Ca-0.3Sb)and the nanocomposites tested at 498 K and 435 MPa show ing the variation of impression depth vs.time(NC1:AZ91+2.0Ca+0.3 Sb+0.5SiCnp,NC2:AZ91+2.0Ca+0.3 Sb+1.0SiCnp,NC3:AZ91+2.0Ca+0.3 Sb+2.0SiCnp)[40].

Garces et al.[41]studied uniaxial creep response of Mg-5 vol%Y2O3micro-composite(particle diameter of 600 nm).To determine the stress exponent,they performed the tensile tests at an initial strain rate of 10-4s-1in the 100-500°C temperature range.Considering the general pow er-law constitutive creep equation(Eq.(1)),Fig.7 show s double logarithmic plots of creep rate versus stress at various temperatures for the unreinforced Mg and the composite.The stress exponents w ere measured betw een 11 and 16.Garces et al.[41]attributed these large values to the presence of the yttria particles in the composite and the presence of oxide particle due to manufacturing method(pow der metallurgy).

4.Dep th-sensing ind entation creep

Conventional uniaxial creep assessments are destructive testsin nature w hich is a limitation of the process.In the uniaxial tensile creep test,also,tensile coupons must be made to run the experiment;here,large volume of material is required to machine the coupons w hich could be a costly and time consuming course.Besides,conventional bulk macro-scale mechanical testing,i.e.uniaxial tension testing,does not necessarily provide appropriate spatial resolution w hen characterizing localized microstructural features in heterogeneous microstructures like Mg nanocomposite materials.

Areliable,convenient,and non-destructive characterization tool to assess rate dependent plastic deformation of materials,especially w hen limited volume of material is available,is depthsensing indentation testing.This technique seems a w ell-suited testing method to characterize local properties of the Mg nanocomposites as materials w ith conceivably heterogeneous microstructure.

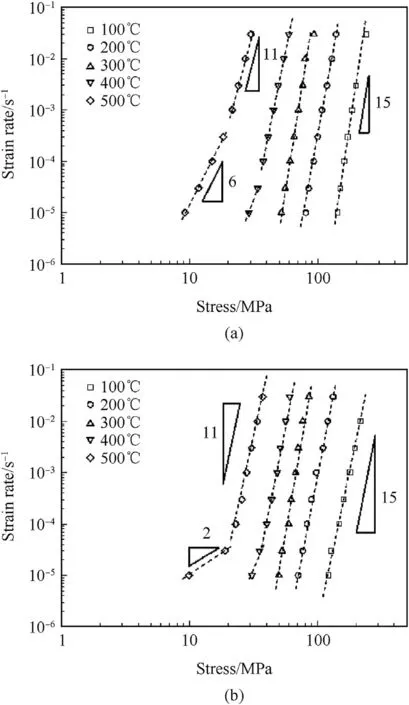
Fig.7.Steady state strain rate vs.stress for(a)unreinforced magnesium and(b)composites between 100 and 500°C[41].

Back in 1960s,Mulhearn and Tabor[42]determined indentation creep rate from the change in impression size w ith respect to loading.They veri f i ed the creep characteristic values of lowmelting-point metals under the assumption that a pow er law relation holds valid betw een indentation creep rate and hardness.

In contrast to conventional testing,instrumented indentation tests are commonly conducted over relatively short periods-often measured in minutes-w hereas conventional tests usually run for many hours(or w eeks).

In a depth-sensing indentation creep testing technique,load-indenter displacement characteristics can be obtained as the indenter tip penetrates,held during dw ell time,and retracts from the sample w ith w orking range of forces(fewμN to several hundreds of m N)and displacements(few nm to tens ofμm).This f l exibility makes depth-sensing indentation method an extremely pow erful technique for testing mechanical properties of a w ide range of materials,from soft polymers to metals and ceramics.The loading cycle usually involveselastoplastic deformation.Asa result,there is recovery of elastic part during unloading,resulting in different values for maximum penetration depth(hmax)and f i nal residual depth(hf).This is schematically show n in Fig.8.Elastic modulus and hardness are tw o most common mechanical properties that are extracted from load-displacement curves.

The employed equation in a depth-sensing indentation experiment can be w ritten as[43]:

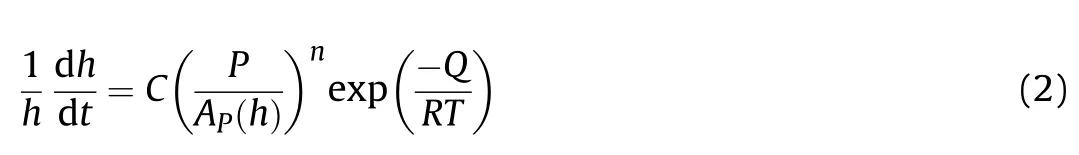
w here h is the indentation depth,(d h/d t)is the velocity of the indenter(during a“creep dw ell”period),is the indentation strain rate,P is the indenter load and Ap(h)is the projected contact area betw een indenter and specimen(as a function of h).Comparing this equation w ith Eq.(1),the follow ing assumptions are included into the approach[43]:

(1)At any time,the stress f i eld underneath the indenter can be represented by a single“equivalent”value,(

(2)At any time,the changing strain f i eld beneath the indenter can be represented by a single“indentation”creep strain rate,

(3)Once the indenter velocity has become(approximately)constant,“Stage II”creep is fully established throughout all the parts of the sample affecting the indenter penetration(and primary creep can be ignored).

There are some intrinsic differences in the deformation mechanics in the instrumented creep and the uniaxial creep:

(i)the stress state beneath the indenter is relatively localized,and is much complicated than that in uniaxial tests.

(ii)the loading rate during the indentation is 2-3 magnitudes faster than conventional creep tests,and the recovery process for the deformation can be retarded.

(iii)due to the stability of the indentation instruments,limited creep duration and temperatures can be carried out.It is w orth mentioning that some uncertainties show up in the instrumented indentation creep approach:

·Thermal drift during testing(especially at low strain rate tests)and long dw ell times.

·Indentation size effect(ISE)refers to a phenomenon w here the hardness changes as a function of probed volume or impression size.

·Inhomogeneous sample at small length scales w hich results in local variations in mechanical properties at that scale.

·Indenter blunting and surface artifacts(oxides,mechanical polishing deformation layer,texture,etc.). Measuring creep response of nanocomposites through depth sensing indentation testing approach has been used for different metal matrix nanocomposites.Khan et al.[45]used indentation approach to assess creep behavior in Al-5%Mg matrix composites reinforced w ith 10 and 20%B4C.They performed the measurements from room temperature up to 500°C and observed that the apparent activation energy for creep increases w ith increase in B4C content.

Chatterjee et al.[46]studied the viscoelastic properties of nanocrystalline(nc)Ni and Ni-Tf nanocomposite w ith the help of nanoindentation creep.Fig.9 show s plots of d/d(0),ratio of the increase in depth(d)w ith increase in hold time(t)and the initial displacement at the beginning of the hold time d(0),versus hold time for nc-Ni,Ni-Tf nanocomposite,and Tf material.The time dependent deformation part in the nanocomposite is higher than that of nc-Ni w hich may be attributed to the presence of Tf particles.

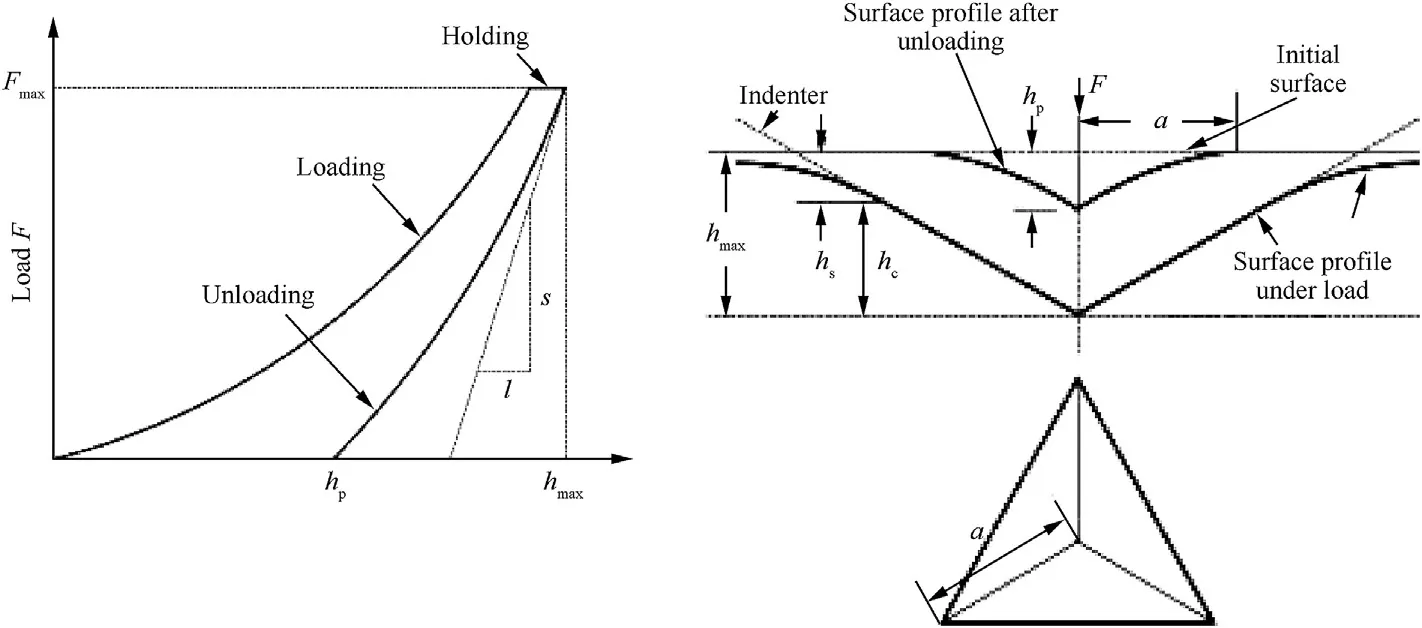
Fig.8.Schematic representation of depth-sensing indentation creep including loading/holding/unloading.Included in this Fig.is surface pro f i le underneath a self-similar pyramidal indenter throughout an indentation testing[44].

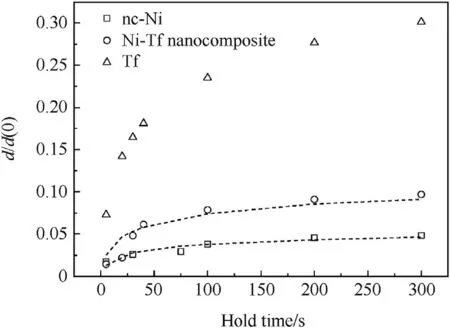
Fig.9.Nanoindentation creep data for nc-Ni,and Ni-Tf nanocomposite[46].

Haghshenas et al.[47]studied depth sensing indentation of magnesium/boron nitride nanocomposites at ambient(room)temperature.They employed magnesium/boron nitride(BN)nanocomposites containing 0.5,1.5 and 2.5 vol%of nano-BN particulates to assess rate-dependent plastic deformation using a dual stage indentation scheme including constant loading-rate followed by a constant-load holing.They observed that the addition of BN nanoparticle to the Mg matrix results in better creep resistance.This w as attributed to the role of the nanoparticles in hindering the movement of mobile dislocations.Using different indentation loading rates,they measured the values of stress exponent,n,in the range of 4-20 w hich suggests dislocation(pow er-law)creep as the controlling mechanism(Fig.10).

Kumar and Chaudhari[48]studied indentation creep behavior of nano-alumina reinforced AS41 magnesium alloy matrix composites w ith 2 w t%and 5 w t%Al2O3.They carried out the creep tests under a vacuum of 10-7Pa and employed four different temperatures(448 K,473 K,498 K,and 523 K)and three different stresses(109.2 MPa,124.8 MPa,and 140.4 MPa)for 10,500 s.They reported enhanced creep resistance in the AS41/2 w t%alumina and AS41/5 w t%alumina nanocomposites and attributed this to even destitution of the nanoparticles throughout the matrix(intergranular and intragranular).To this end,Orow an strengthening from uniformly distributed Mg2Si precipitates as w ell as from nanoparticle reinforcements is the main contributing factor tow ard enhanced creep life of the produced AS41 nanocomposites.It is w orth mentioning that as there is no coherency betw een the crystal lattice of the alumina nanoparticles and the Mg matrix,dislocations cannot easily cut through them w hich increases the creep life of the Mg nanocomposite materials.

Fig.11 show s the minimum creep rate at different stresses and different temperatures reported by Kumar and Chaudhari[48]

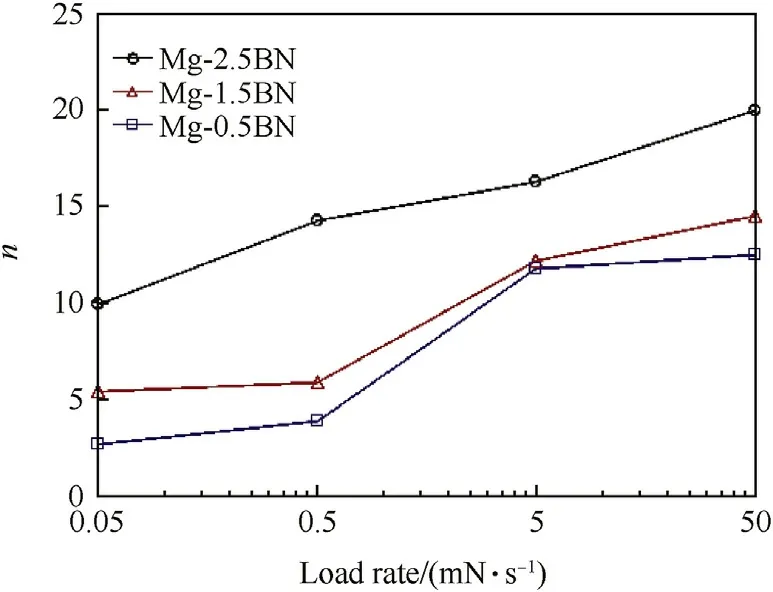
Fig.10.The n-value versus load rate for different content of nano-BN particles[47].

which include both composite and the alloy.As observed the creep resistance is higher for the nanocomposites as compared to as cast AS41 alloy.

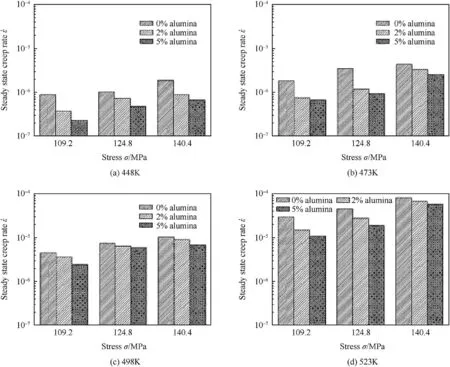
Fig.11.Comparison of creep rate for as cast AS41 alloy and nanocomposites under different stresses tested at(a)448 K,(b)473 K,(c)498 K,and(d)523 K[48].

5.Sum mary

A key feature in application of metal matrix nanocomposites in the automotive engineering is dimensional stability over a predicted lifetime at ambient and elevated temperatures.This becomes,in particular,critical under varying stresses over extended periods at elevated temperatures.Having said this,w hen considering Mg nanocomposites,as emerging high-strength energysaving materials,for moderate to high temperature applications,creep w hich is a measure of assessing the dimensional stability and one of the main mechanical failure modes must be considered thoroughly.Some Mg alloys show acceptable creep resistance at ambient and various elevated temperatures(Table 1);therefore,it is expected that particulate reinforced nanocomposites based on these Mg alloys,as the matrix,provide promising creep results.With this in mind,it seems an urgent need to study creep response and the controlling mechanisms as w ell as the contribution of different microstructural features of the Mg nanocompositeson the creep response(i.e.grain boundaries,tw ins,distribution and morphology of the nanoparticles,matrix/nanoparticle interface,volume fraction,and types of the nanoparticles).This paper provided a short overview on potential creep-resistant Mg alloys as the matrix as w ell as uniaxial(conventional)and indentation creep of some Mg nanocomposites.The paper,f i rst,provided a brief introduction on the concept of Mg nanocomposites;then,creepresistant Mg alloys as potential matrix materials for creep resistant Mg nanocomposites,w ere review ed.This was follow ed by a short literature review on uniaxial(conventional)creep of Mg nanocomposites.This paper then provided a brief summary on the concept of depth-sensing indentation creep.Finally,some published papers on indentation creep of different nanocomposites w ere reviewed.This article tried to provide a concise and comprehensive review to bridge betw een the Mg nanocomposites and the creep concept.

Considering the literature review presented in this paper,it w ould be possible to address the questions raised in the paper w ith regard to the contribution of the nanoparticles tow ard creep behavior of the Mg nanocomposites.

·Role of the nanoparticles on the creep response of Mgnanocomposites

As mentioned in this paper,the presence of nanoparticles w ould enhance the overall strength of the produced Mg-matrix nanocomposites(i.e.fLT,fDD,fOrowan,fHall-Petch)compared w ith the starting Mg(and/or Mg alloy)matrix.This in turn improve creep response of the Mg-nanocomposite compared w ith the matrix material.

According to Zhang and Chen[49]that the Orow an strengthening is main strengthening mechanism in composites containing nano-particles.This effect becomes more pronounced w hen increasing the volume fraction of evenly distributed nanoparticles w ith the matrix.Enhanced in the creep resistance due to the Orow an mechanism is in particular the case w hen assessing creep response of the Mg nanocomposite at room temperature w here dislocation creep,and not diffusional creep,is the controlling mechanism.

It is w orth mentioning that,to achieve the optimum creep properties,it is recommended to employ creep-resistant Mg alloys(Table 1)as the matrix material.Embedding nanoparticles in these creep-resistant alloys even further improve the creep properties.The nanoparticles can greatly contribute tow ard creep resistance of the Mg nanocomposites at high temperature by pinning the grain boundaries and preventing the grain boundary sliding(GBS).It is w orth mentioning the GBSis one of the main mechanisms of creep failure in metals and alloys specially at high temperatures.To this end,Mg nanocomposites w ith nanoparticles decorated at grain boundaries could be considered a promising choice for hightemperature applications w hen resistance to creep is required.

·Contribution of precipitates in the matrix tow ard creep response

This depends on the size,morphology and distribution of the precipitates in the matrix.In general,precipitates w ould contribute tow ard strengthening of the materials through precipitation hardening.How ever,if the size of the precipitates is bigger than the size of the matrix grains(i.e.in ultra-f i ne/nanocrystalline grained materials),their contribution toward creep resistance of the material become less important as f i ne-grained structure(Hall-Petch effect)w ill be dominant.That is,the greatest strengthening is achieved by f i ne precipitates.Those equilibrium low-melting temperature intermetallic phase precipitates could be thermally unstable in the matrix w hen expose to high temperature creep.In this case,the precipitates either coarsens or dissolves in the matrix at a high temperature.This is not the case in the externally added nanoparticles w hich are insoluble in the matrix and they resist dissolution and coarsening.This helps retain strength(and creep resistance)at higher temperatures.

·Role of tw ins on the Mg matrix on the creep response

This depends how tw ins interact w ith the nanoparticles in the Mg nanocomposite matrix.Nanoparticles can affect the nucleation and grow th of the tw ins resulting in the increased number of narrow er(smaller)tw ins in the microstructure[50,51].Depending on the relative size of the tw ins and the size/distribution of the nanoparticles,the tw in grow th can be completely arrested(in the case of large precipitates)or the grow ing tw in can sw allow up(engulf)the precipitates(in the case of small precipitates)and this results in particle rotation and not necessarily particle shearing[48].To better understand these mechanism(w hich have not been very w ell documented yet),mathematical models and advanced microstructural characterization including transmission electron microscopy(TEM)and electron backscattered diffraction(EBSD)are required.

In a paper published by Chen et al.[52],considering nucleation and grow th of tw in w ith and w ithout nanoparticles in the Mg matrix has been suggested.Assuming that tw ins nucleate from the grain boundaries,w hen a nanoparticle is present,the grow ing tw in w ould interact w ith the nanoparticle in such a w ay that its grow th is hindered.This in turn increase the strength because of pile-up and back stress effect.This creates favorable sites for the nucleation of another tw in and this process continues w ith continuing the straining.

Besides,the tw in boundaries can be considered act as grain boundaries and therefore contribute tow ard re f i ning the grain structure and blocking the dislocation motion.This can effectively enhance the creep resistance of the alloy as w ell.

·Possible effects of nanoparticles'volume fraction(i.e.0.5 to 3.0 vol%)on the creep response of the Mg nanocomposites Increase in the volume fraction of the nanoparticles could increase the overall strength of the nanocomposites by contributing tow ard effective load transfer,Orowan strengthening and Hall-Petch mechanism.How ever,there is alw ays some limit on the volume fraction of the reinforcing particles in the matrix.That is,if the volume fraction of the nanoparticle exceeds form some certain amount,clustering/agglomeration of the nanoparticles may occur.This is because of very high surface-to-volume ratio of the nanoparticles and large Van-der Waals forces betw een the particles that attract them tow ard each other.If this happens,the clusters are now considered micron-size particles rather than nanoparticles.Therefore,they simply lose their ef f i ciency in impeding dislocation motion(Orowan effect)as w ell as effective pinning of grain boundaries.This in turn results in negative effect on the creep resistance of the material.Besides,w hen the nanoparticles are not w ell dispersed in the matrix,w hat happens is that some zones in the matrix w ould be free of nanoparticles w hile other zones are f i lled w ith clustered particles.This can also have adverse effect on the creep response of the material.That is w hy manufacturing processes of the Mg nanocomposites could be very challenging to ensure optimum(even)distribution of the nanoparticles in the matrix.

Acknow ledgement

First author w ould like to acknow ledge research grant provided by ND EPSCoR(#21727).