NEW PROOFS OF THE DECAY ESTIMATE WITH SHARP RATE OF THE GLOBAL WEAK SOLUTION OF THE n-DIMENSIONAL INCOMPRESSIBLE NAVIER-STOKES EQUATIONS∗

Linghai Zhang

(Dept.of Math.,Lehigh University,14 East Packer Avenue,Bethlehem,Pennsylvania 18015,USA)

Abstract Consider the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations:−α△u+(u·∇)u+∇p=f(x,t),with the initial condition u(x,0)=u0(x)and with the incompressible conditions∇ ·u=0,∇ ·f=0 and∇·u0=0.The spatial dimension n≥2.Suppose that the initial function u0∈L1(Rn)∩L2(Rn)and the external force f∈L1(Rn×R+)∩L1(R+,L2(Rn)).It is∫ well known that there holds the decay estimate with sharp rate:(1+t)1+n/2 Rn|u(x,t)|2dx≤C,for all time t>0,where the dimension n≥2,C>0 is a positive constant,independent of u and(x,t).The main purpose of this paper is to provide two independent proofs of the decay estimate with sharp rate,both are complete,systematic,simplified proofs,under a weaker condition on the external force.The ideas and methods introduced in this paper may have strong in fluence on the decay estimates with sharp rates of the global weak solutions or the global smooth solutions of similar equations,such as the n-dimensional magnetohydrodynamics equations,where the dimension n≥2.

Keywords n-dimensional incompressible Navier-Stokes equations;global weak solution;decay estimate with sharp rate;Fourier transformation;Parseval’s identity;Gronwall’s inequality

1 Introduction

1.1 The mathematical model equations

Consider the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations

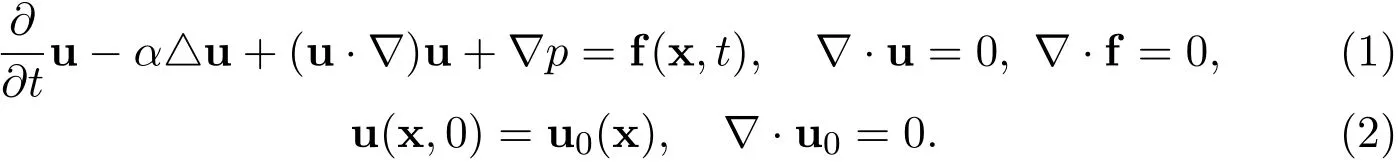
The real vector valued function u=u(x,t)represents the velocity of the fluid at position x and time t.The real scalar function p=p(x,t)represents the pressure of the fluid at x and t.The positive constant α>0 represents the diffusion coefficient.

1.2 Previous related results

The existence of a global weak solution or a global smooth solution:Suppose that the initial function u0∈L2(Rn)and the external force f∈L1(R+,L2(Rn)).Then there exists a global weak solution u∈L∞(R+,L2(Rn)),such that∇u∈L2(R+,L2(Rn)).Moreover,for the spatial dimension n=2,if the initial function u0∈L1(R2)∩H2m+1(R2)and the external force f∈L1(R2×R+)∩L1(R+,L2(R2))∩L2(R+,H2m(R2)),then there exists a global smooth solution u∈L∞(R+,H2m+1(R2)),such that∇u∈L2(R+,H2m+1(R2)),for any positive integer m≥1.Under additional conditions,the solution is in finitely smooth:u∈C∞(R2×R+).There holds the following solution representation

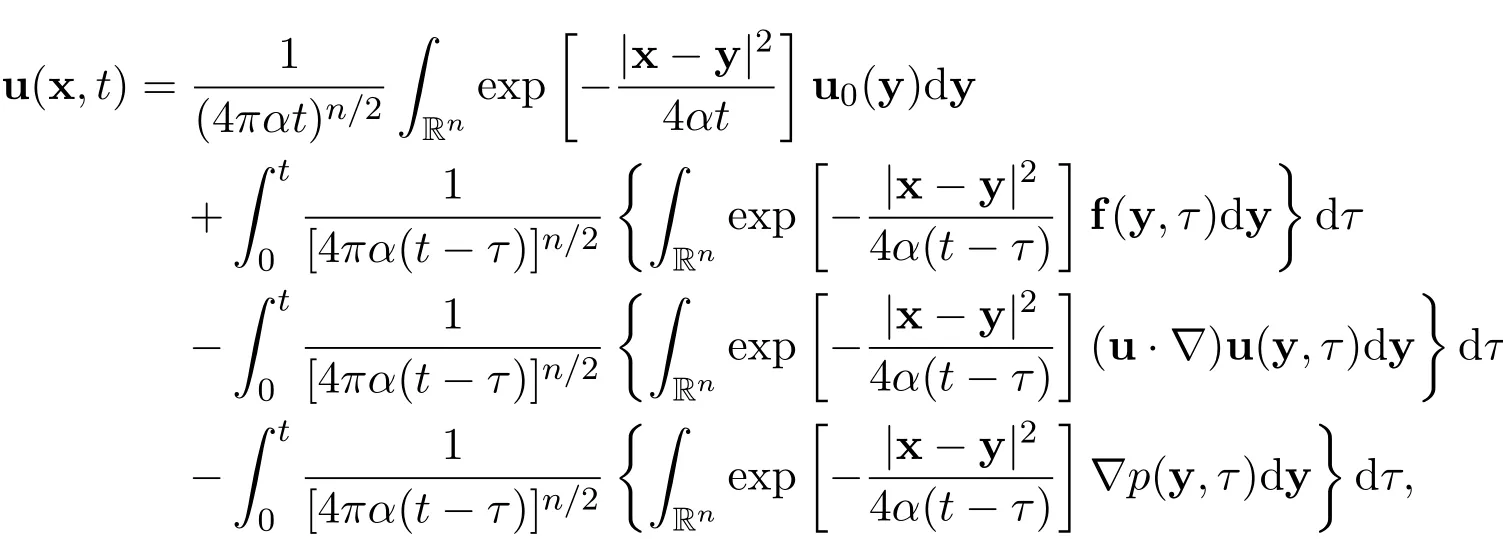
where

for all x∈Rnand t>0.

The decay estimates with sharp rates:Suppose that the initial function u0∈L1(Rn)∩L2(Rn)and the external force f∈L1(Rn×R+)∩L1(R+,L2(Rn)).Then there holds the following decay estimate with sharp rate

for all t>0,where C>0 is a positive constant,independent of u and(x,t).

1.3 The mathematical assumptions

Suppose that the initial function u0∈L1(Rn)∩L2(Rn)and the external force f∈L1(Rn×R+)∩L1(R+,L2(Rn)).Suppose that there exist real scalar functions ϕkl∈ C1(Rn)∩L1(Rn)and ψkl∈ C1(Rn× R+)∩L1(Rn×R+),such that

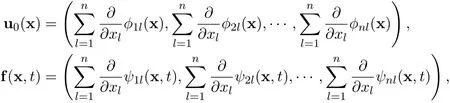
and that

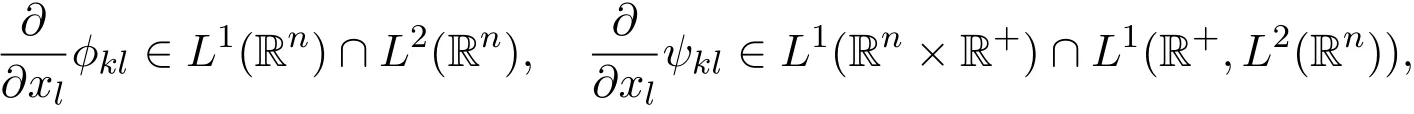
for all positive integers k=1,2,3,···,n and l=1,2,3,···,n.

These assumptions are motivated by the special structures of the nonlinear functions(u·∇)u and∇p in the n-dimensional incompressible Navier-Stokes equations,due to the incompressible condition∇·u=0.They are also motivated by the following facts

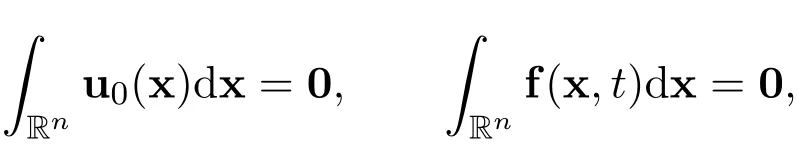
for all t>0.

Suppose that there exists the following integral

We allow the initial function and the external force to have some lower order singularity,such as

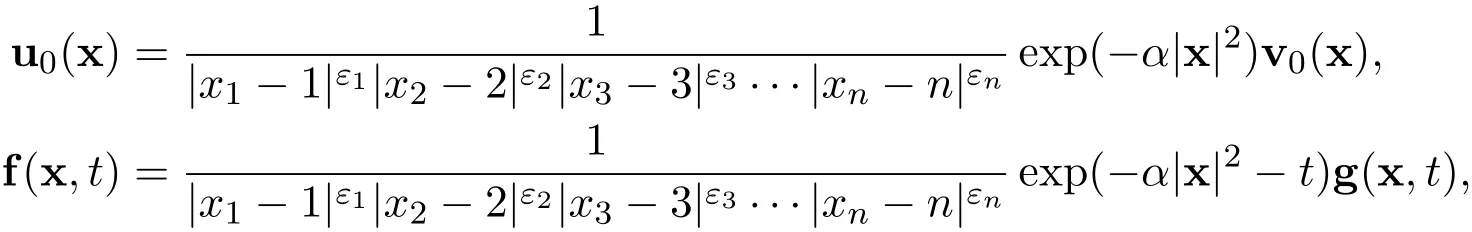
where ε1, ε2, ε3, ···, εnare sufficiently small positive constants,such that 0<2ε1<1,0<2ε2<1,0<2ε3<1,···,0<2εn<1.The functions v0=v0(x)and g=g(x,t)are chosen in such a way that∇·u0=0 and ∇·f=0.

1.4 The main results

There holds the following decay estimate with sharp rate for the global weak solution of the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations(1)-(2):

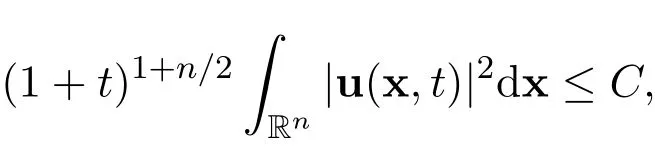
for all time t>0,where the dimension n≥2,C>0 is a positive constant,independent of u and(x,t).

2 The Mathematical Analysis and the Proofs of the Main Result

For the decay estimate with sharp rate of the global weak solution of(1)-(2),we will provide two independent proofs:one proof for the case n≥2 and another proof for the case n≥3.Both proofs are complete,systematic and simpler than before.

2.1 The proof of the decay estimate with sharp rate for the case n≥2

We will provide the proof of the decay estimate with sharp rate for the global weak solution of(1)-(2),where the dimension n≥2.

Theorem 1Suppose that the initial functionu0∈L1(Rn)∩L2(Rn)and the external forcef∈L1(Rn×R+)∩L1(R+,L2(Rn)).Suppose that there exists the following integral

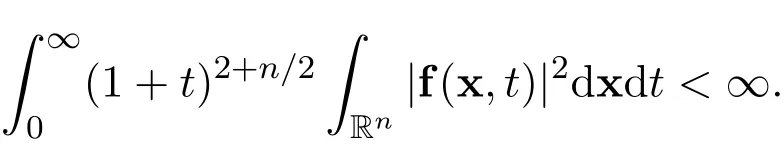
Then there holds the following decay estimate with sharp rate

for all timet>0,where the dimensionn≥2,C>0is a positive constant,independent ofuand(x,t).

Proof There are three main steps in the proof of Theorem 1.

Step 1The proof of the elementary decay estimate

Multiplying the n-dimensional incompressible Navier-Stokes equations by 2u and integrating the result with respect to x over Rnyield

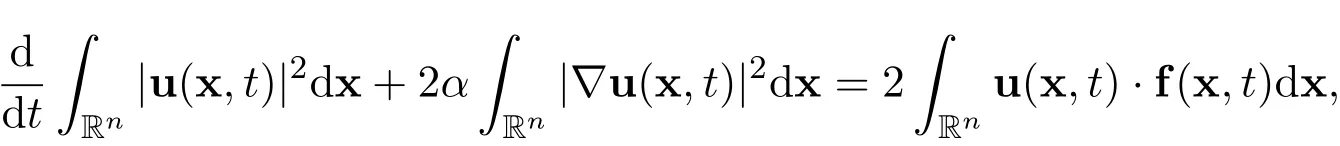
where

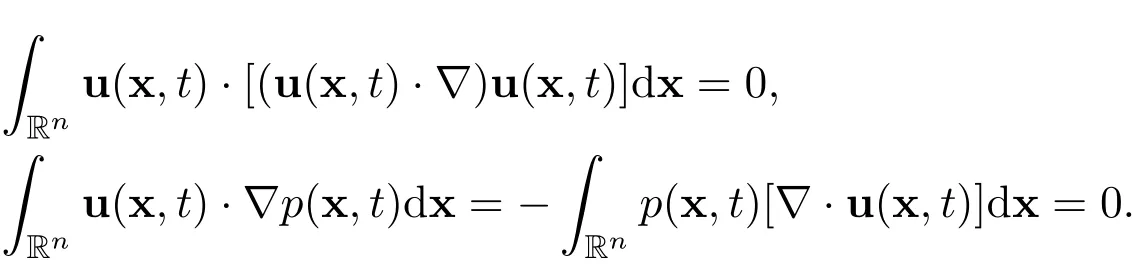
Applying the Parseval’s identity to the last energy equation leads to

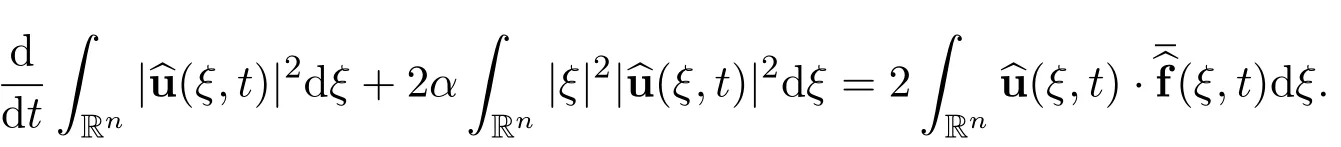
Multiplying this equation by[ln(e+t)]2ngives the new energy equation

By using Cauchy-Schwartz inequality,we have the following estimate

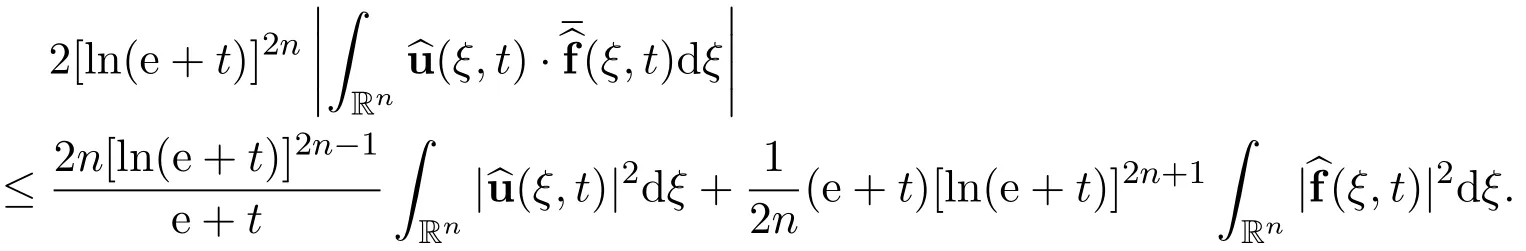
Therefore,the above energy equation becomes the differential inequality

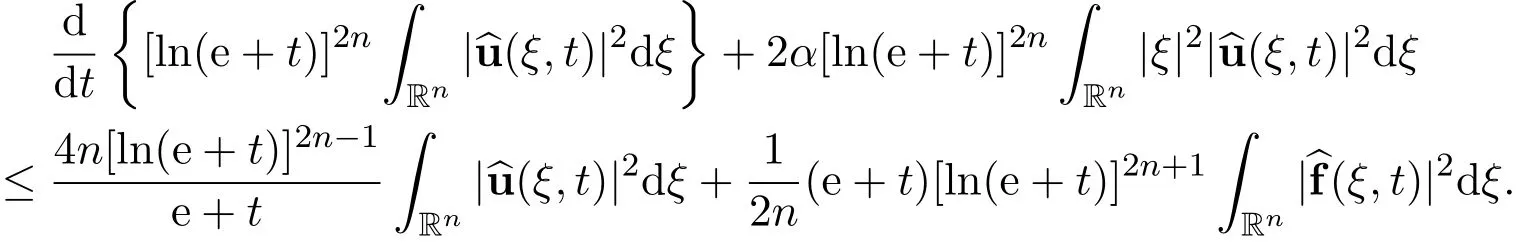
Let us split the frequency space as the union of a small ball and its complementary.Define

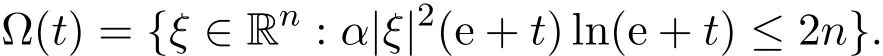
Then there hold the following estimates

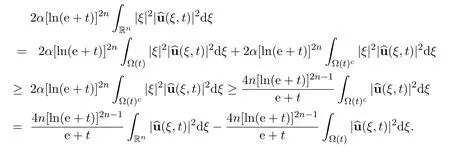
Therefore,the above differential inequality reduces

Applications of standard ideas and methods in differential equations lead to the following representation of the Fourier transformation

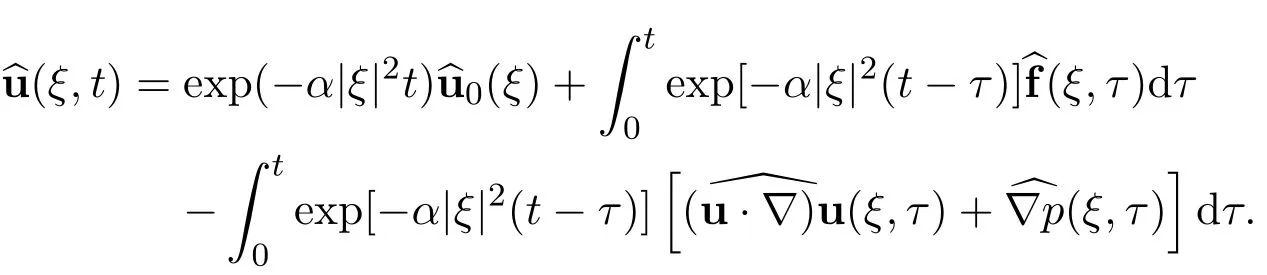
Note that

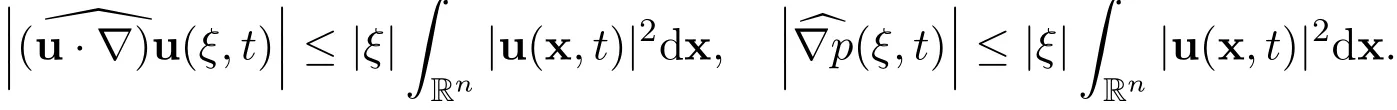
Now we have

Multiplying the n-dimensional incompressible Navier-Stokes equations(1)by 2u and integrating the result with respect to x over Rn,using Cauchy-Schwartz’s inequality and integrating the differential inequality with respect to time t leads to the following elementary uniform energy estimate

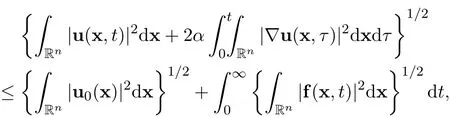
for all t>0.

Therefore,there hold the following estimates

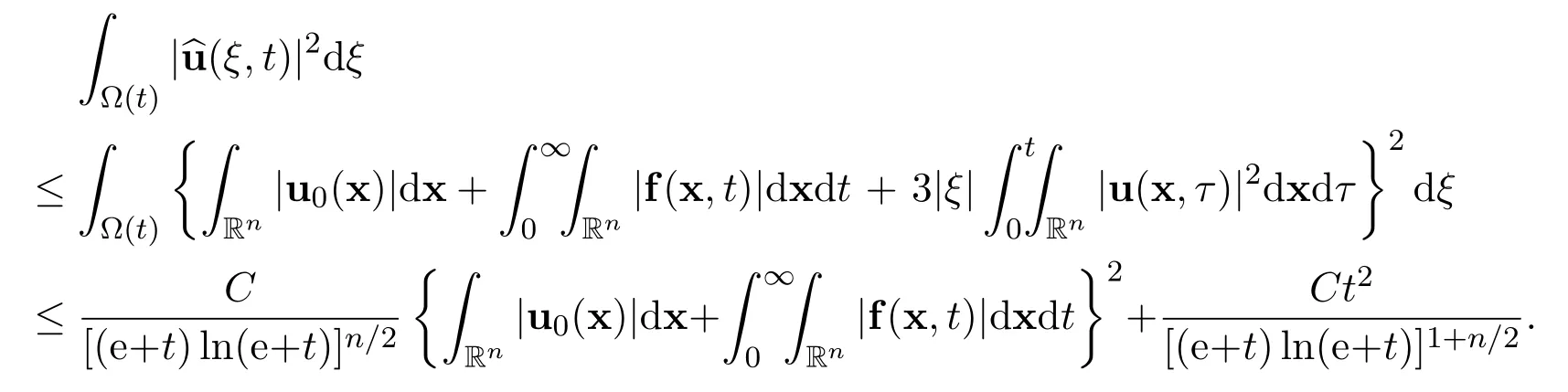
Now the above differential inequality becomes

where C>0 is a positive constant,independent of u and(x,t).Integrating the inequality with respect to time t leads to

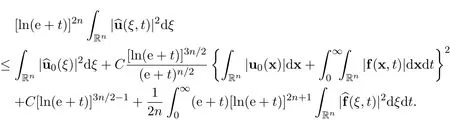
Therefore,there holds the following elementary decay estimate

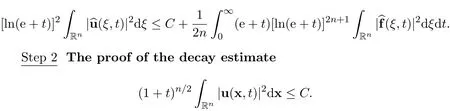
Multiplying the n-dimensional incompressible Navier-Stoke equations by 2u and integrating the result with respect to x over Rnyield

where

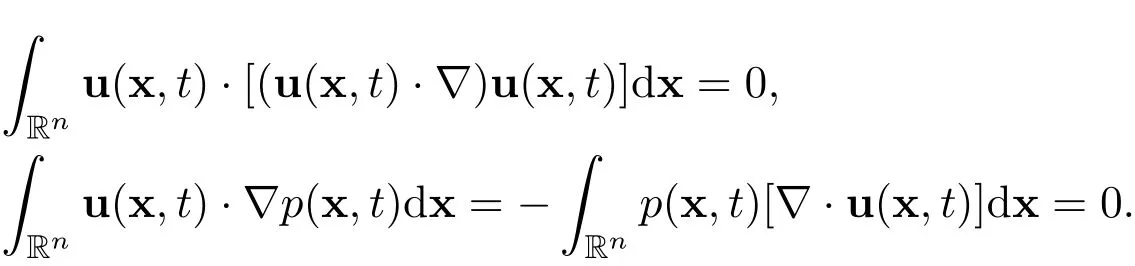
Applying the Parseval’s identity to the energy equation leads to

Multiplying this equation by(1+t)2n,we get

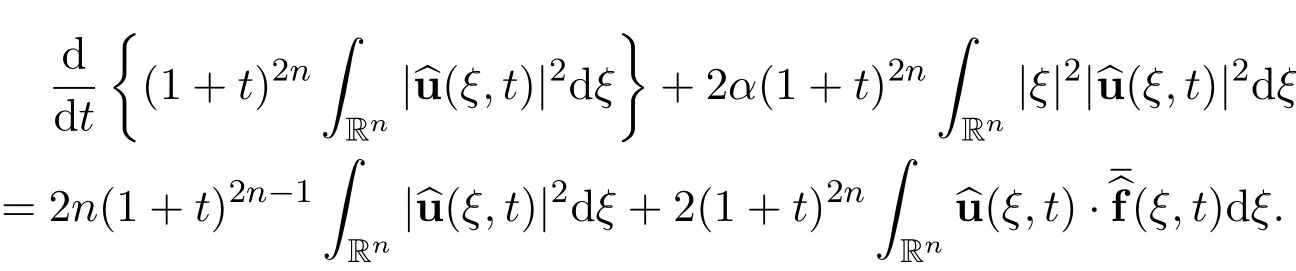
By using Cauchy-Schwartz’s inequality,we have

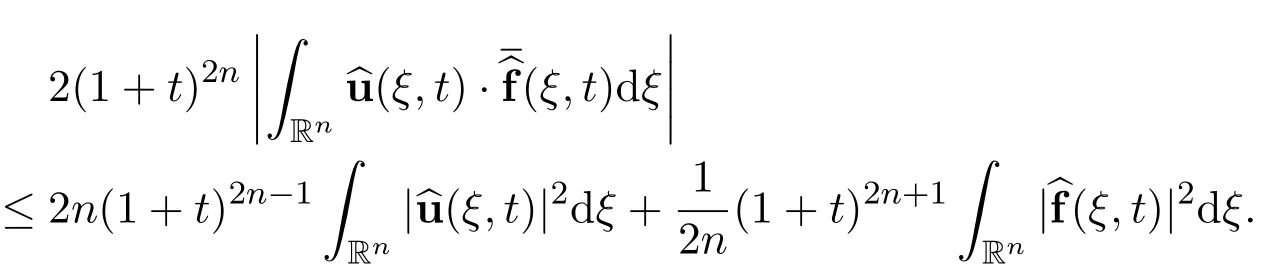
Now the above energy equation becomes a differential inequality

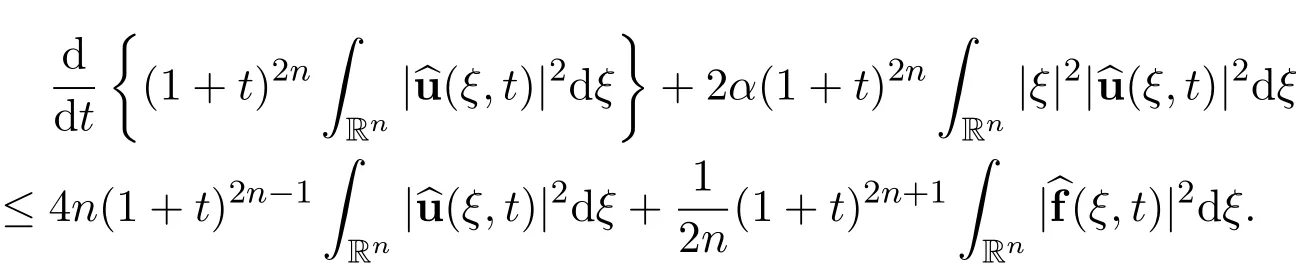
Define

Then there hold the following estimates

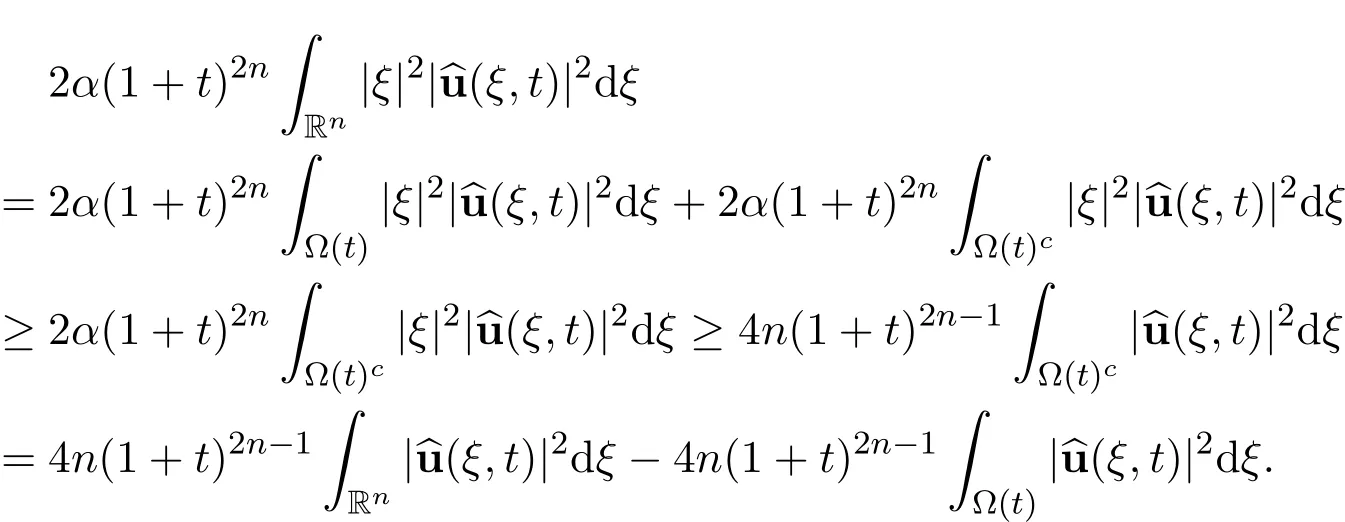
Now the differential inequality reduces

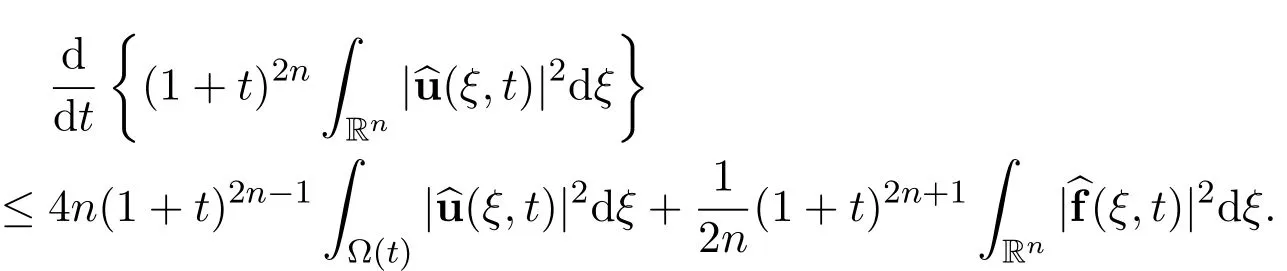
By using the representation of the Fourier transformation

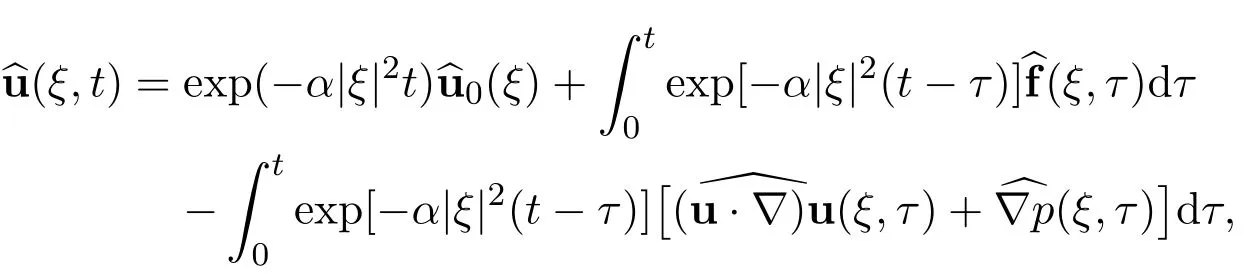
we have the estimate

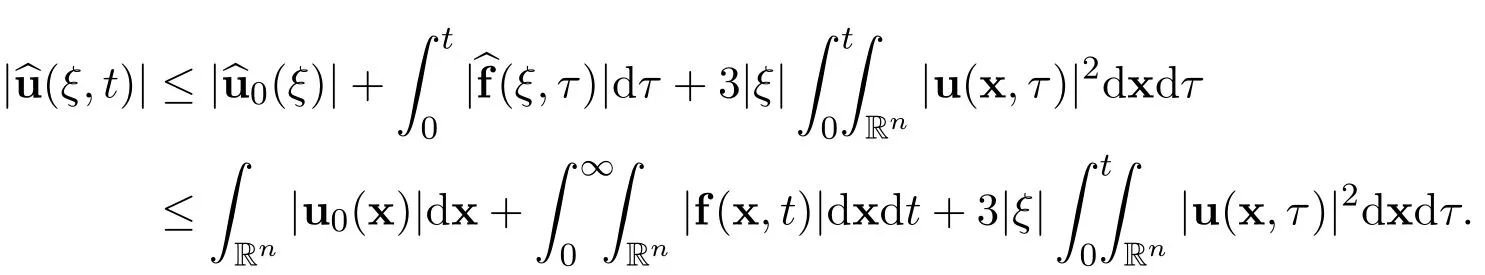
Now there hold the following estimates

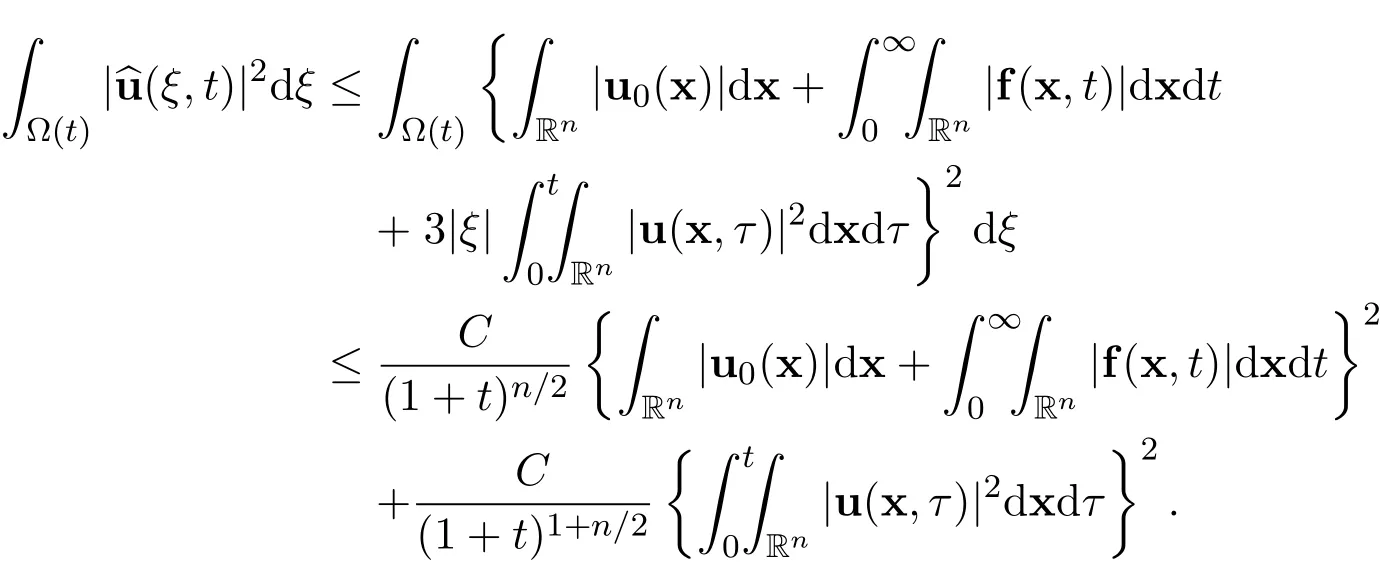
Therefore,we have the following inequality

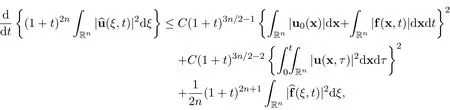
where C>0 is a positive constant,independent of u and(x,t).Integrating this inequality with respect to time t yields

That is

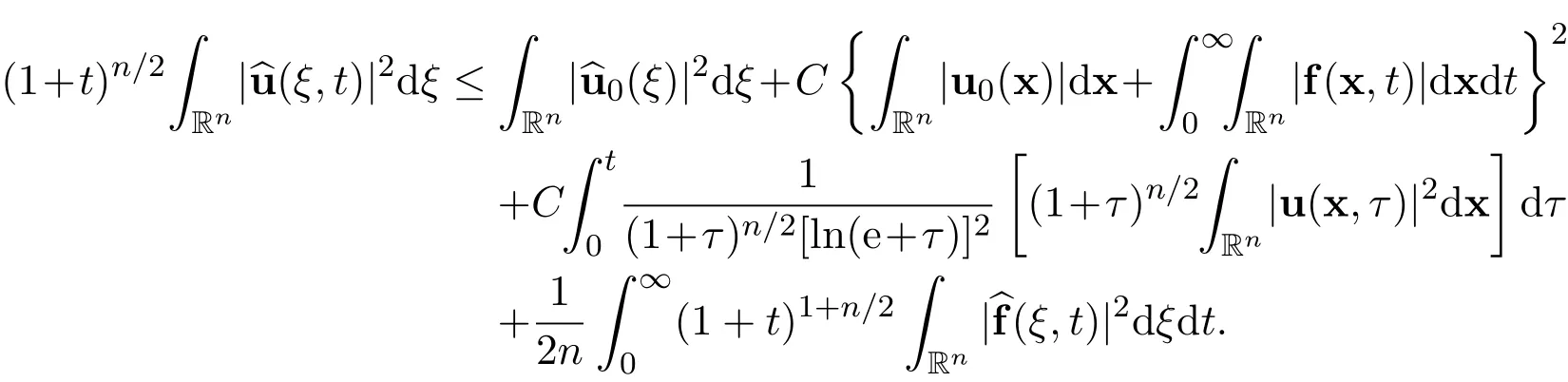
By using Gronwall’s inequality,we obtain the decay estimate

where C>0 is a positive constant,independent of u and(x,t).

Step 3The proof of the decay estimate with sharp rate

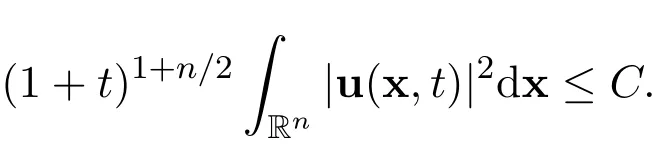
Recall that we have derived the representation for the Fourier transformation

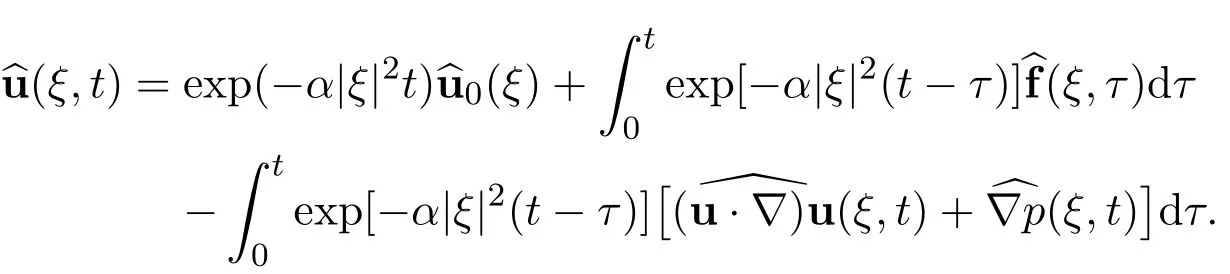
Suppose that there exist real scalar functions ϕkl∈ C1(Rn)∩ L1(Rn)and ψkl∈C1(Rn× R+)∩ L1(Rn× R+),for all k=1,2,3,···,n and l=1,2,3,···,n,such that

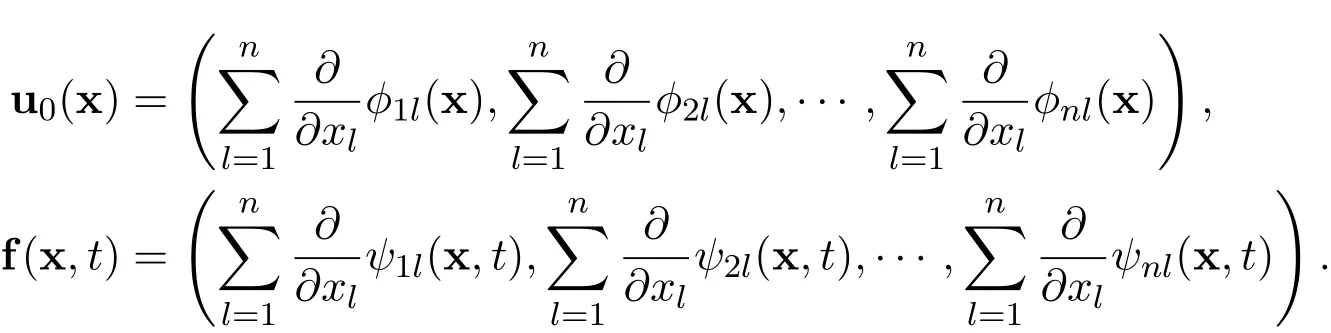
Performing Fourier transformation to the initial function and the external force yields the following representation

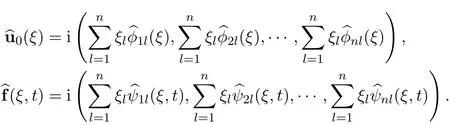
The following estimates follow from the Cauchy-Schwartz’s inequality

Therefore

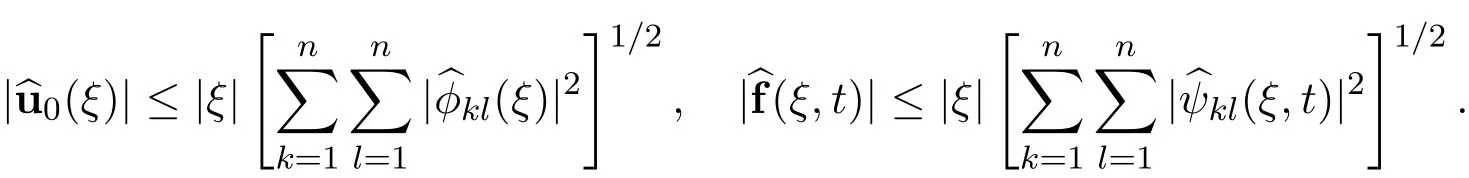
Taking the divergence of the n-dimensional incompressible Navier-Stokes equations leads to

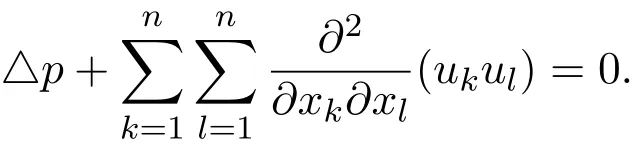
Performing the Fourier transformation to this equation yields

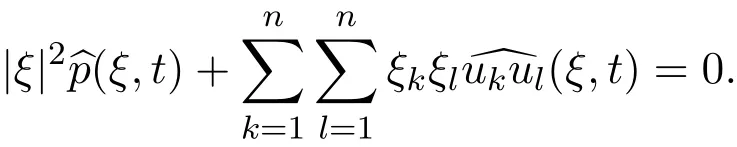
By using the Cauchy-Schwartz’s inequality,we can easily establish the following estimates

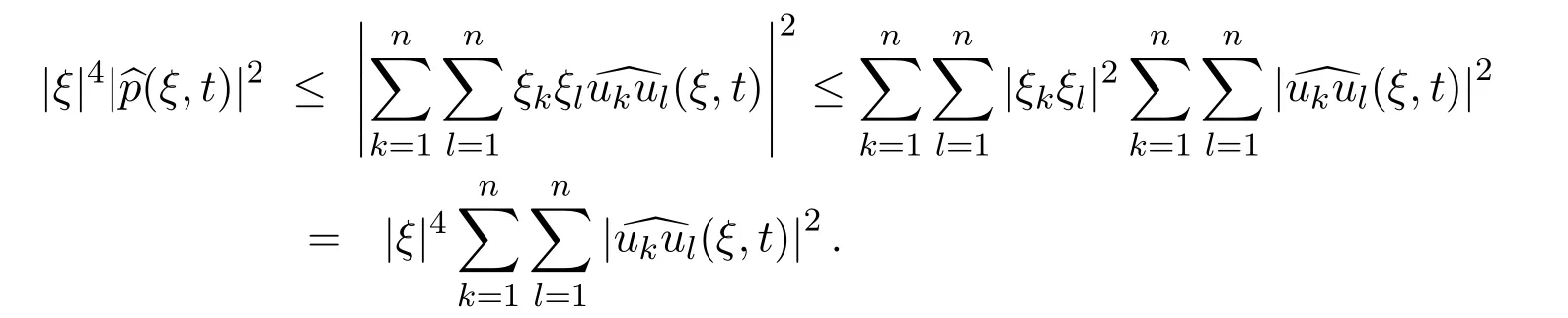
Therefore

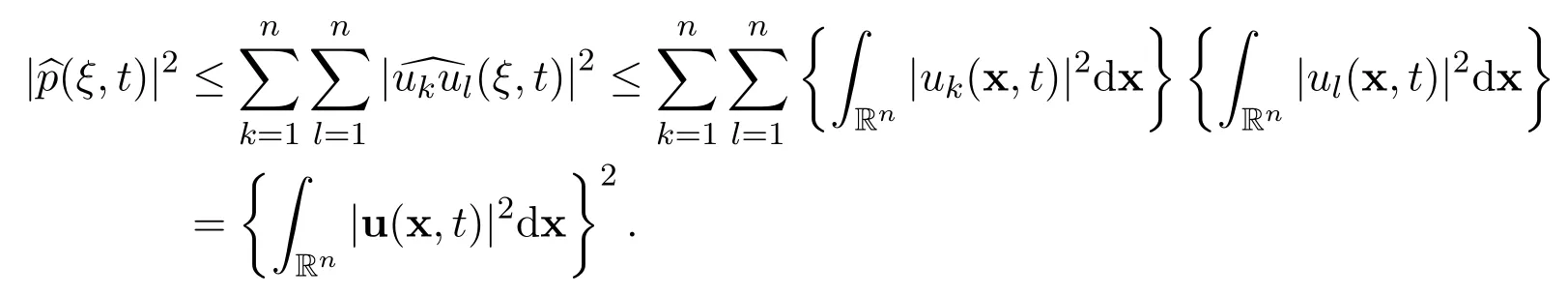
Now there hold the following estimates

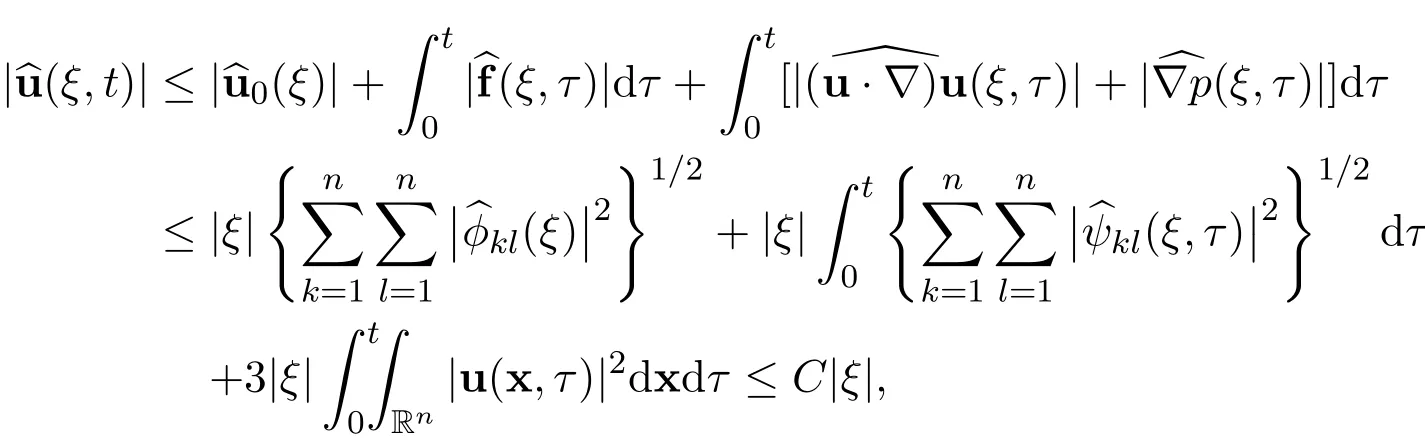
and then

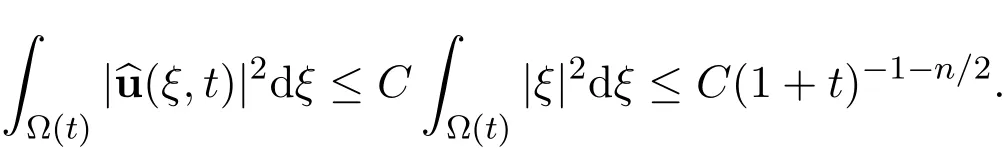
Therefore

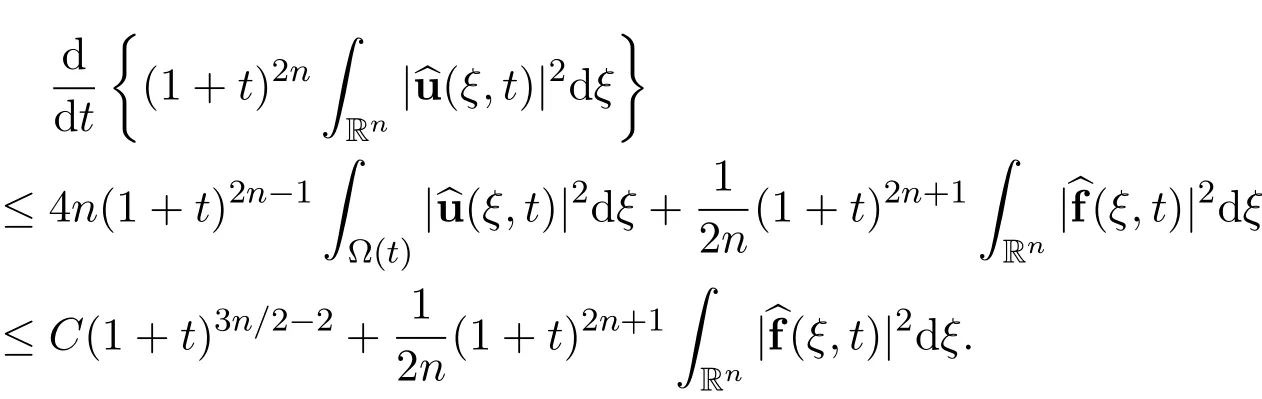
Upon integrating this inequality with respect to time t,there hold the following estimates

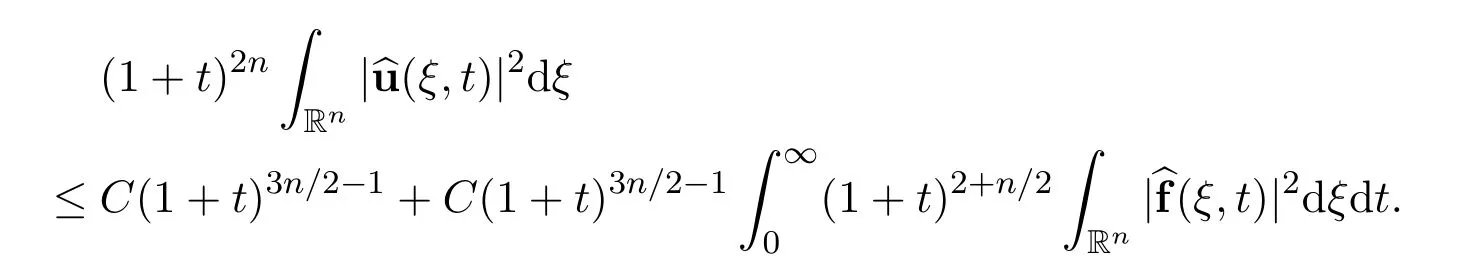
Finally

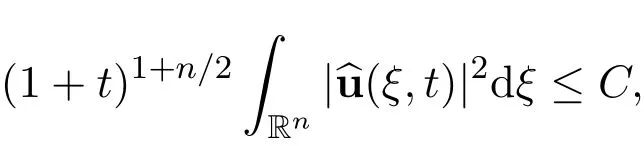
where C>0 is a positive constant,independent of u and(x,t).

The proof of Theorem 1 is completed finished.

Remark 1 For the two-dimensional incompressible Navier-Stokes equations

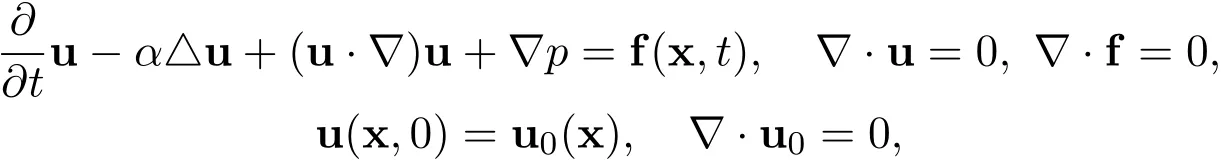
where

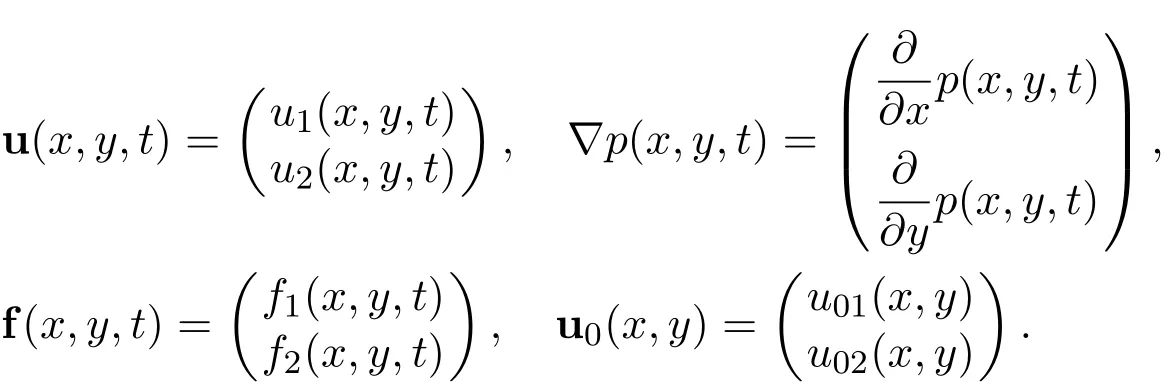
Let

Then

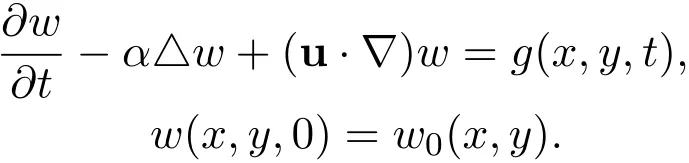
Multiplying this equation by 2w and integrating the result with respect to(x,y)over R2leads to

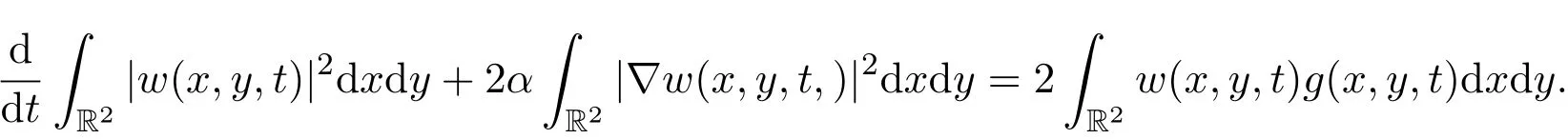
That is

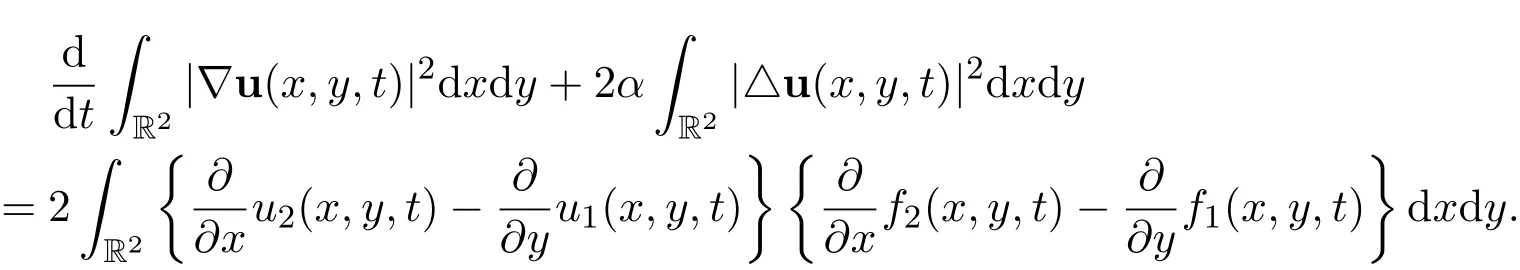
Then we may use very similar ideas to establish the decay estimate with sharp rate.For higher order derivatives of the global smooth solution,we may use very similar ideas to establish the decay estimates with sharp rates,provided that there hold the corresponding uniform energy estimates for the derivatives.

2.2 The proof of the decay estimate with sharp rate for the case n≥3

The proof of the decay estimate with sharp rate of the global weak solution of the case n≥3 is independent of the proof of the case n≥2.

Theorem 2Suppose that the initial functionu0∈L1(Rn)∩L2(Rn)and the external forcef∈L1(Rn×R+)∩L1(R+,L2(Rn)).Suppose that there exists the following integral

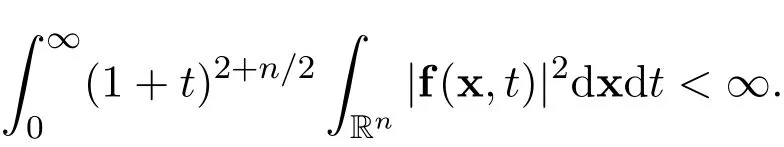
Then there holds the following decay estimate with sharp rate

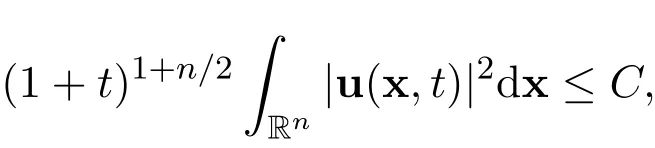
for allt>0,where the dimensionn≥3,C>0is a positive constant,independent ofuand(x,t).

Proof There are twelve simple steps in the proof.

Step 1A differential inequality.Multiplying the n-dimensional incompressible Navier-Stokes equations(1)by 2u and integrating the result with respect to x over Rnyields the energy equation

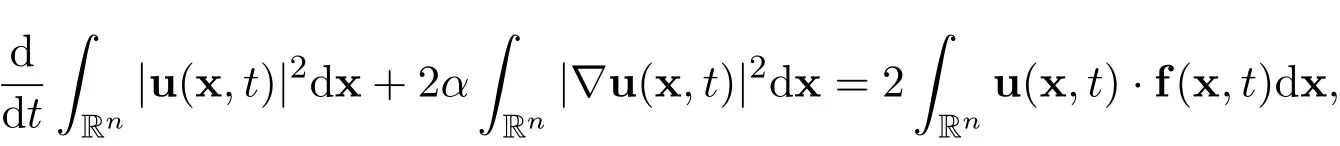
where

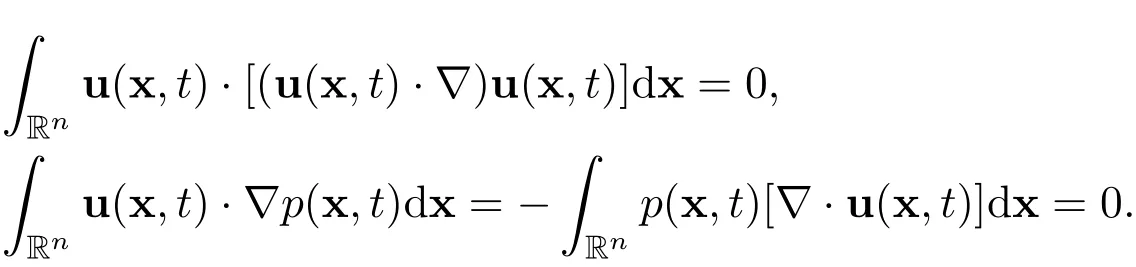
Applying the Parseval’s identity to the energy equation leads to

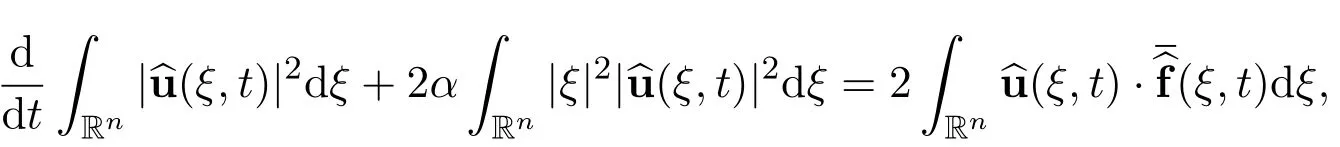
Multiplying this equation by(1+t)2n,we get

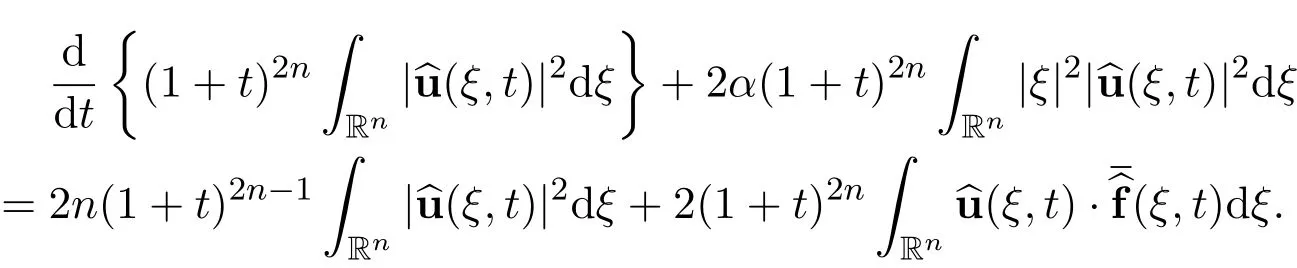
Applying Cauchy-Schwartz’s inequality to the last integral term,we get

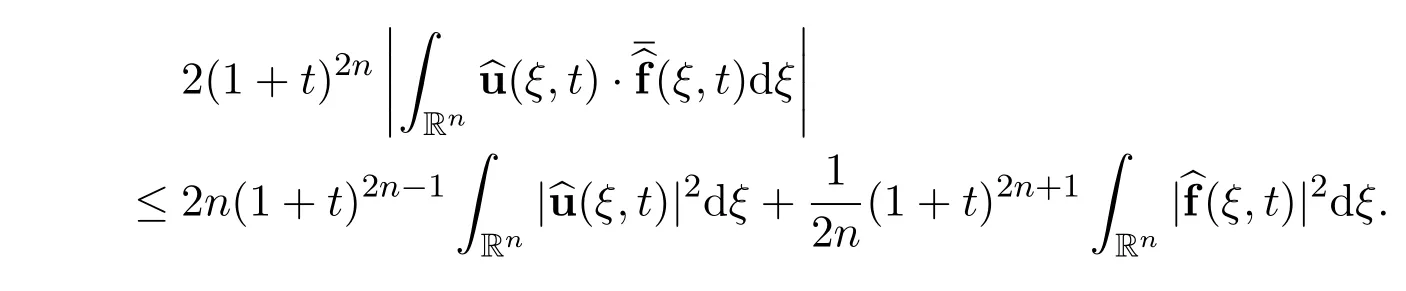
Now we have the differential inequality

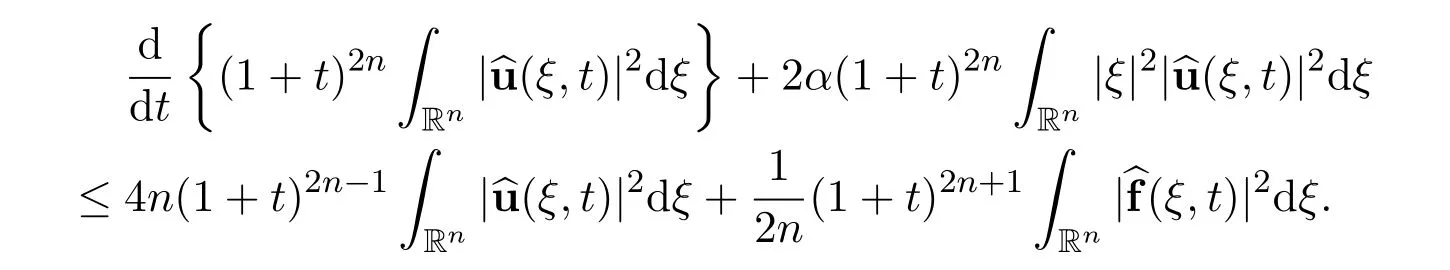
Step 2The splitting of the frequency space.Define

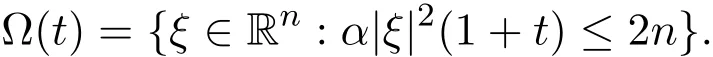
Then there hold the following estimates

Now the above differential inequality becomes

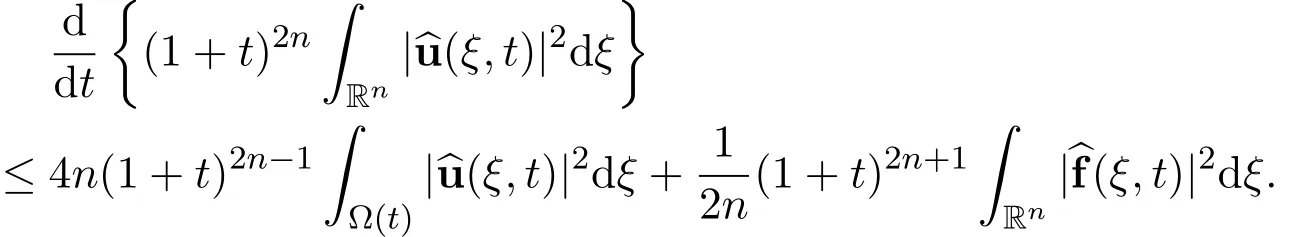
Step 3The elementary uniform energy estimate.There holds the following uniform energy estimate for the global weak solution

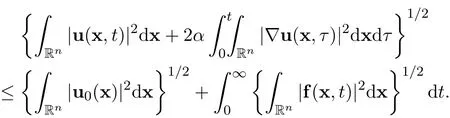
Step 4The representation of the Fourier transformation ub(ξ,t).There holds the following representation for the Fourier transformation

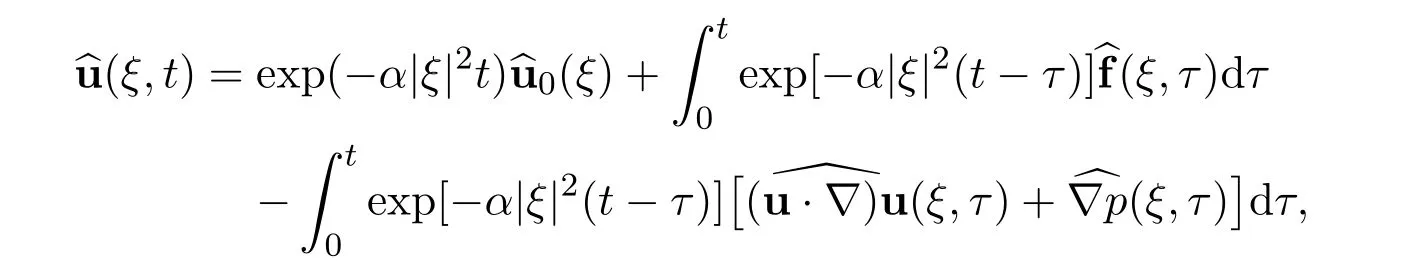
for all(ξ,t)∈ Rn×R+.

Step 5The elementary estimates ofand its integral

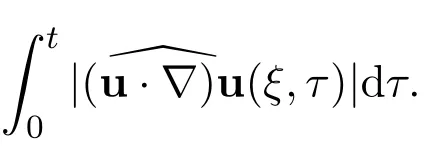
By definition of the Fourier transformation,we have

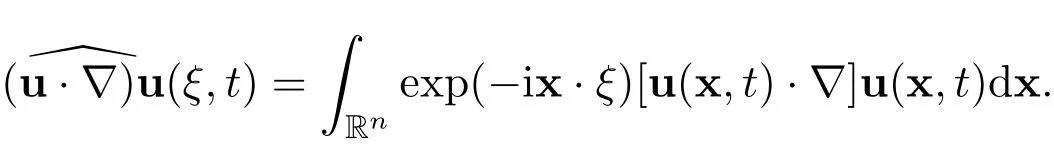
Then there hold the following estimates

Therefore,there holds the following estimate

for all(ξ,t)∈ Rn×R+.

Step 6The elementary estimates of the Fourier transformationand its integralTaking the divergence of the n-dimensional incompressible Navier-Stokes equations leads to

Performing the Fourier transform gives

There hold the following estimates and

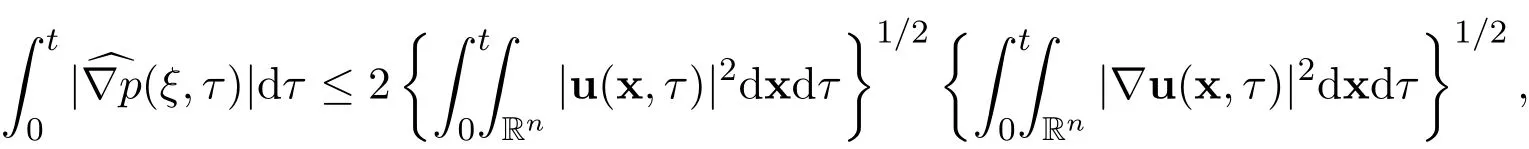
for all ξ∈Rnand t>0.

Step 7The elementary estimate of the Fourier transformation ub(ξ,t).By using the representation of the Fourier transformation

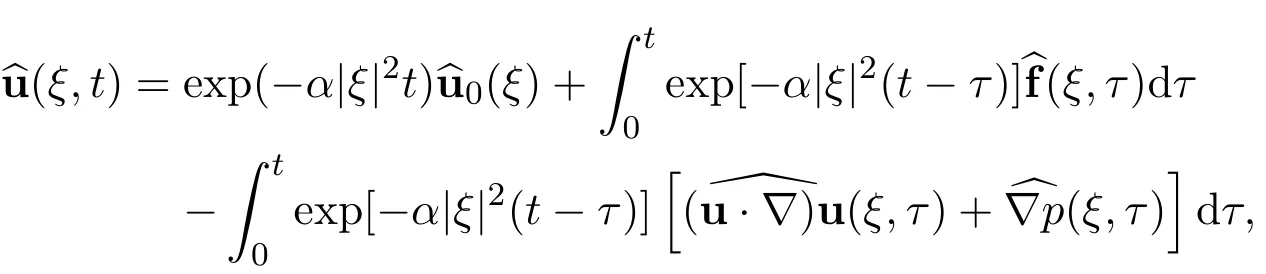
and the above estimates,we have the following estimates

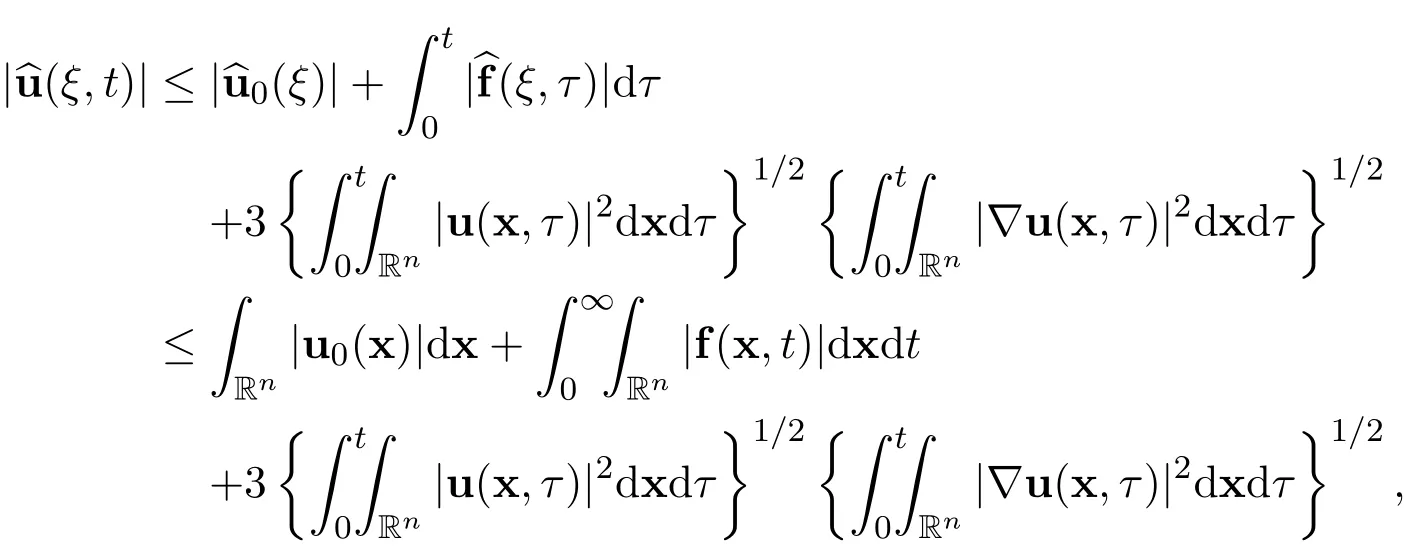
for all(ξ,t)∈ Rn×R+.

Step 8The estimate of the integralThere hold the following estimates

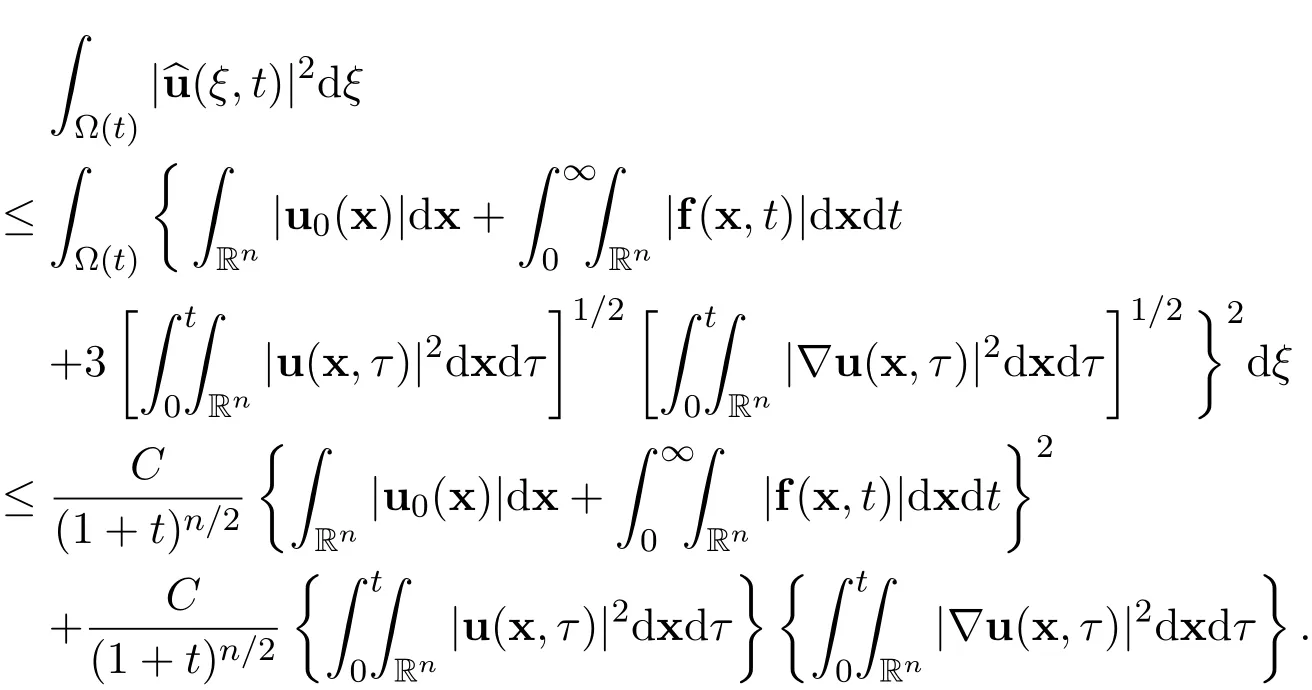
Step 9An energy inequality.By coupling together Steps 2 and 8,there hold the following estimates

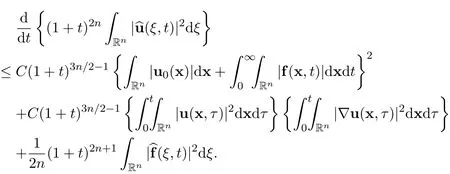
Integrating it with respect to time t gives

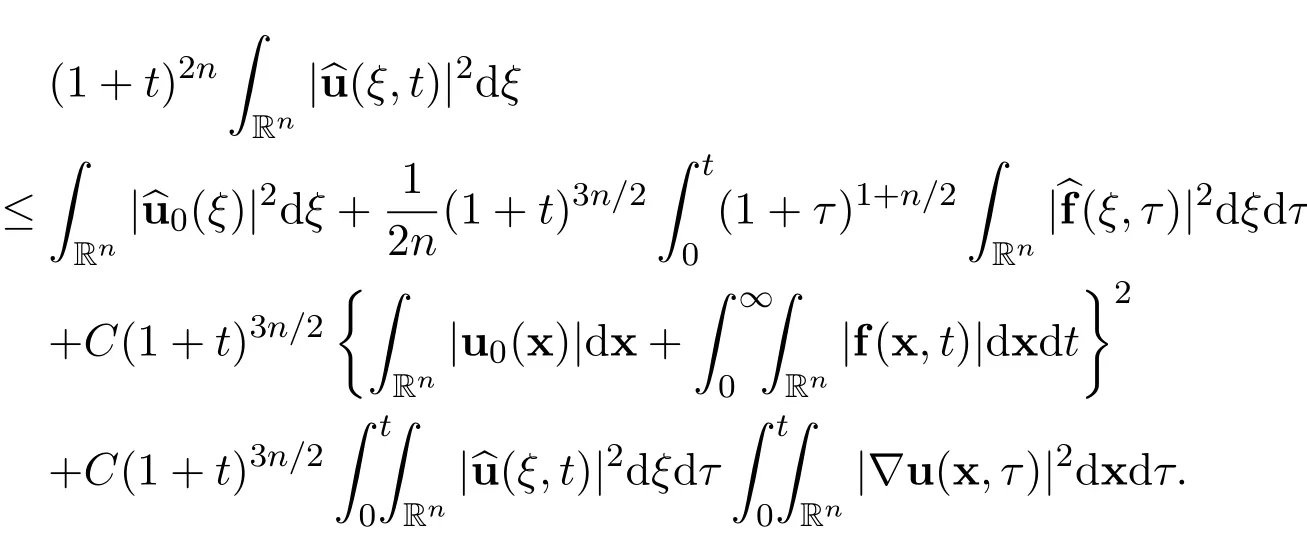
Step 10The application of a Gronwall’s inequality.Now there hold the following estimates

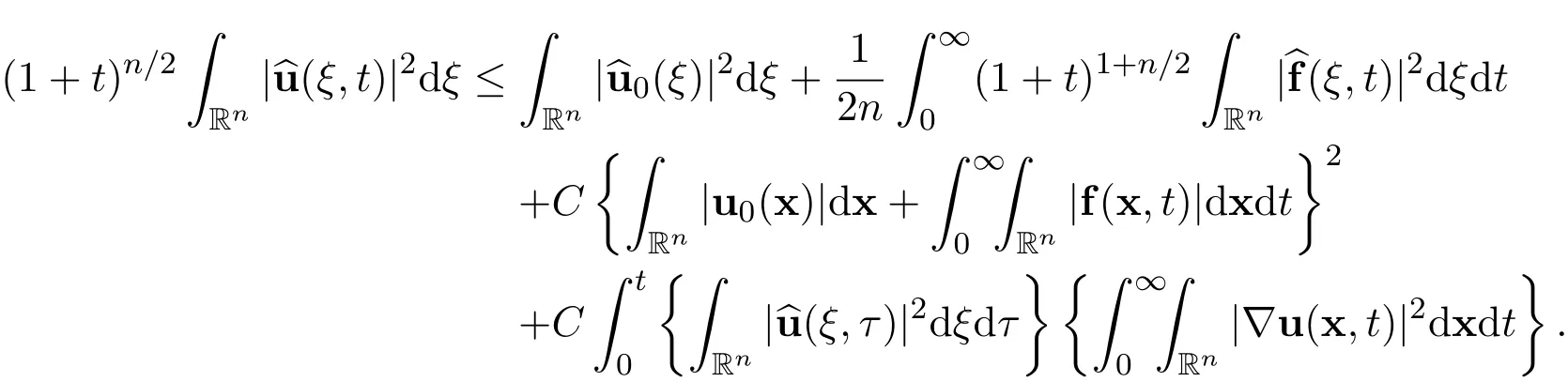
If we apply the Gronwall’s inequality,then we obtain the decay estimate for all n≥3 and for all time t>0.

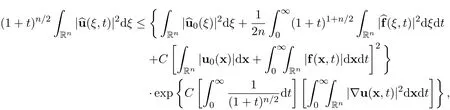
Step 11The elementary estimates of the Fourier transformations ub0(ξ),and.Suppose that there exist real scalar functions ϕkl∈ C1(Rn)∩L1(Rn)and ψkl∈ C1(Rn×R+)∩L1(Rn×R+),such that the initial function u0and the external force f satisfy

By using the definition of Fourier transform and Cauchy-Schwartz’s inequality,we have the following estimates

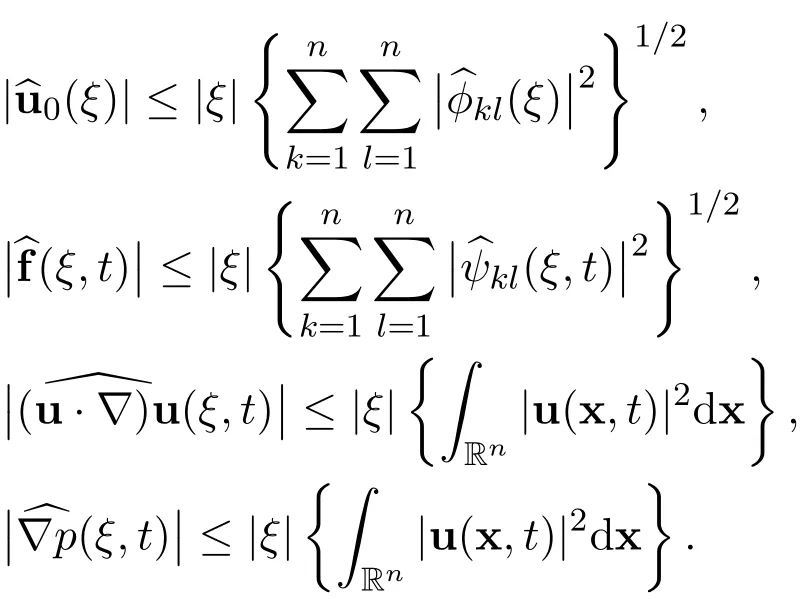
Step 12The decay estimate with sharp rate.By coupling together these estimates,Steps 4 and 11,we have the following estimates

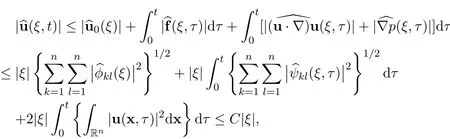
and

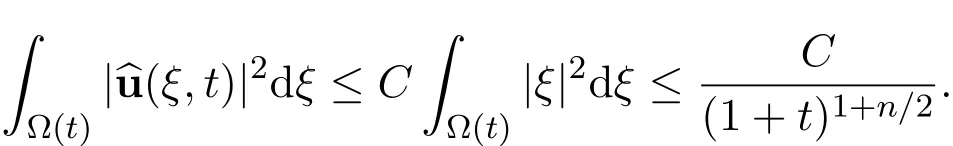
Now the above energy equation becomes

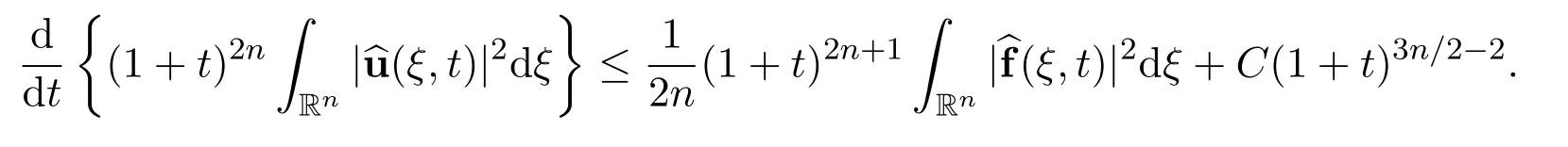
Integrating it with respect to time t gives

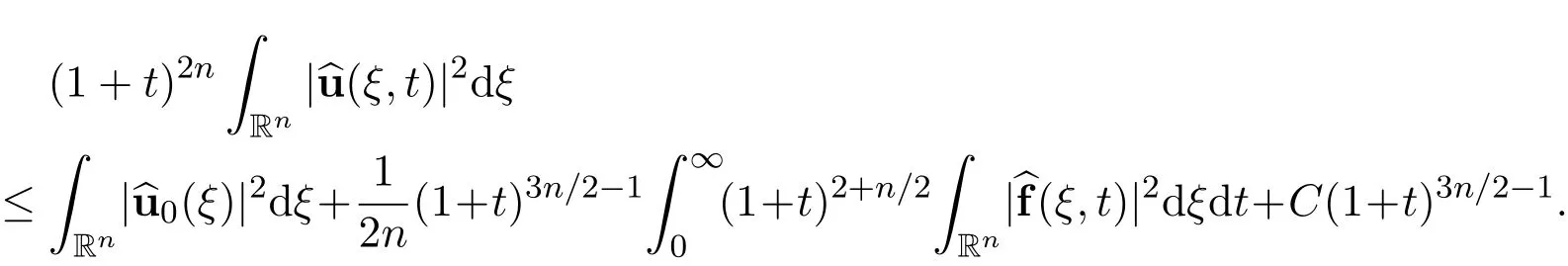
Therefore,we obtain the desired decay estimate with sharp rate

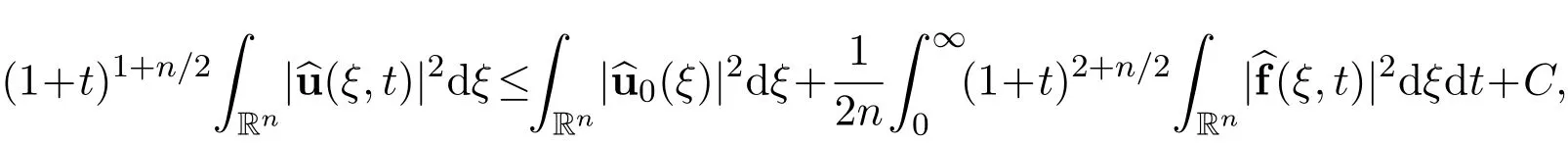
where C>0 is a positive constant,independent u and(x,t).The proof of Theorem 2 is completed.

3 Conclusions and Remarks

3.1Summary

Consider the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations

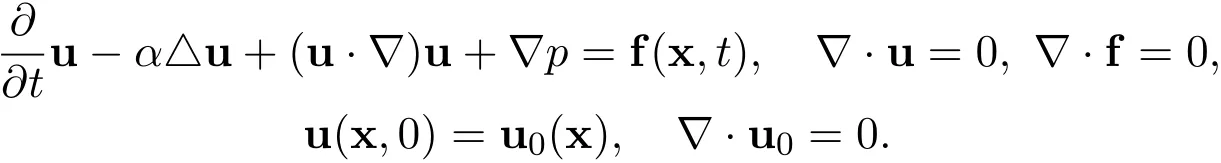
Suppose that the initial function u0∈ L1(Rn)∩L2(Rn)and the external force f∈L1(Rn×R+)∩L1(R+,L2(Rn)).Then there exists a global weak solution u∈L∞(R+,L2(Rn)),such that∇u∈L2(R+,L2(Rn)).Moreover,after a sufficiently long time,the global weak solution becomes small and smooth u∈L∞((T,∞),H2m+1(Rn)),such that∇u∈L2((T,∞),H2m+1(Rn)),where m≫1 is a sufficiently large positive integer and T≫1 is a sufficiently large positive constant,provided that the external force is also sufficiently small and smooth after a long time.Moreover,if the dimension n=2,if the initial function u0∈L1(R2)∩H2m+1(R2)and the external force f∈ L1(R2×R+)∩L1(R+,L2(R2))∩L2(R+,H2m(R2)),then there exists a global smooth solution u∈L∞(R+,H2m+1(R2)),such that∇u∈L2(R+,H2m+1(R2)),where m≥1 is a positive integer.Suppose that there exists the following integral

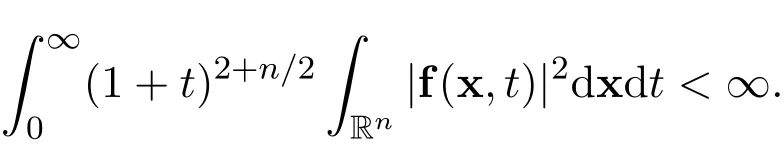
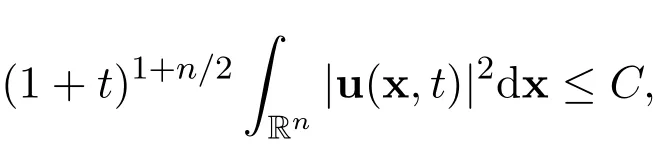
Then there holds the following decay estimate with sharp rate for all t>0,where C>0 is a positive constant,independent of u and(x,t).

The ideas and methods developed in this paper may be used to study the decay estimates with sharp rates of the global weak solutions of many other very interesting equations with dissipations.The rigorous mathematical analysis of the decay estimate with sharp rate of the global weak solution of the nonhomogeneous incompressible Navier-Stokes equations has never been established before,though the same results for the homogeneous Navier-Stokes equations has been established.

3.2 Remarks

Let the initial function u0∈L1(Rn)∩H2m+1(Rn)and the external force f∈L1(Rn×R+)∩L1(R+,L2(Rn))∩L2(R+,H2m+1(Rn)).Motivated by the existence and uniqueness of the local smooth solution u ∈ L∞((T,∞),H2m+1(Rn)),such that∇u∈ L2((T,∞),H2m+1(Rn)),without loss of generality,suppose that there exists a global smooth solution to the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations(1)-(2):u∈L∞(R+,H2m+1(Rn)),such that∇u∈L2(R+,H2m+1(Rn)),where m≥1 is a positive integer.

Suppose that there exist the following integrals

Suppose that there exist real scalar functions ϕkl∈ C1(Rn)∩ L1(Rn)and ψkl∈C1(Rn× R+)∩ L1(Rn× R+),for all k=1,2,···,n and l=1,2,···,n,such that

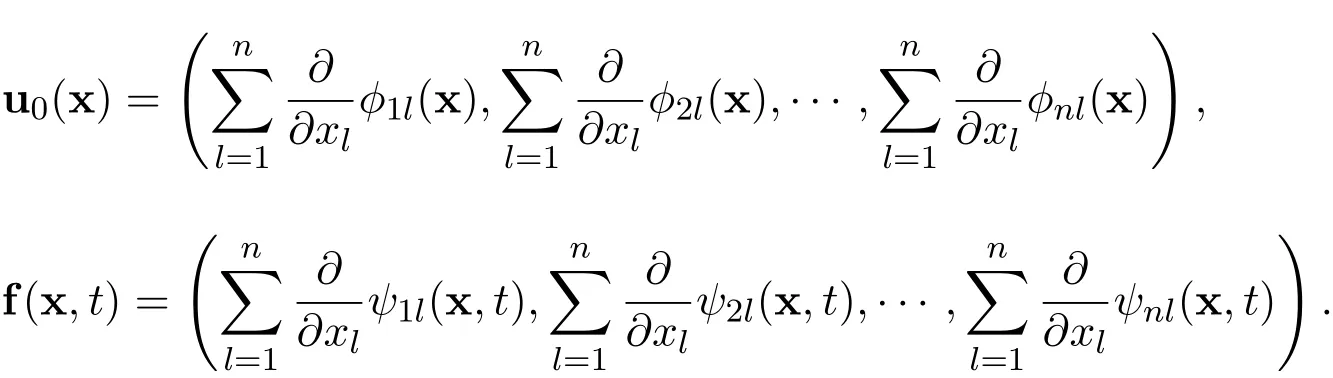
These assumptions are motivated by the structures of the nonlinear functions(u·∇)u and∇p,and by the following facts

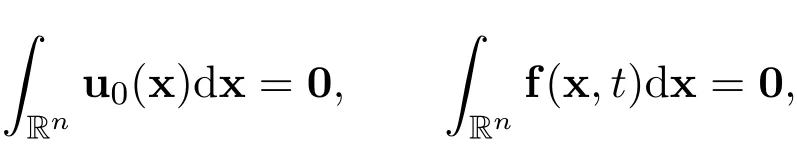
for all t>0.

Note that

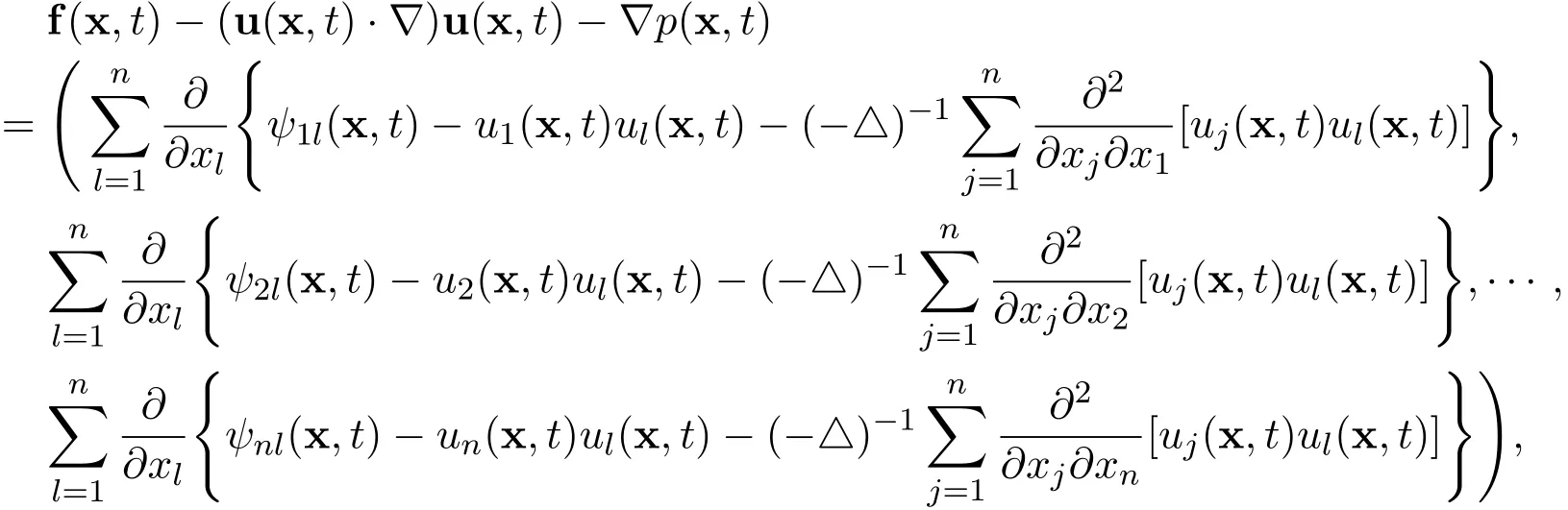
where

We will establish the following exact limits of the global smooth solution of the Cauchy problem for the n-dimensional incompressible Navier-Stokes equations

in terms of α,m,n,the integrals of the initial function and the external force

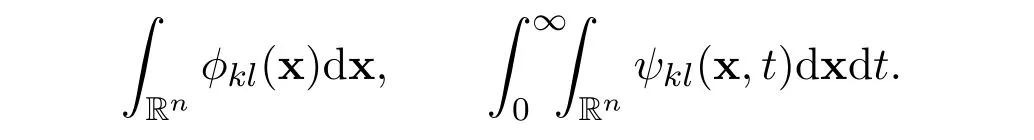
3.3 Some technical lemmas

Recall that the Fourier transformation and the inverse Fourier transformation of u∈L2(Rn)is defined by

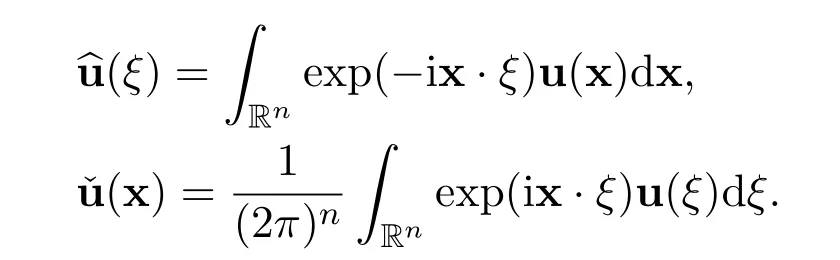
Lemma 1(Parseval’s identity)There holds the following identity

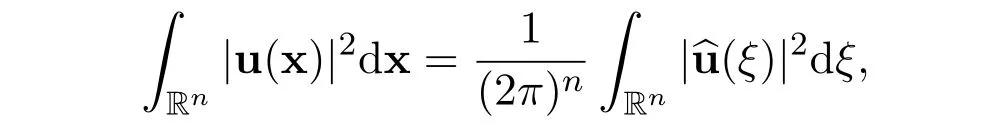
for all functionsu∈L2(Rn).

Lemma 2(The general Gronwall’s inequality)Let the nonnegative continuous functionsg≥0andh≥0satisfy the following inequality

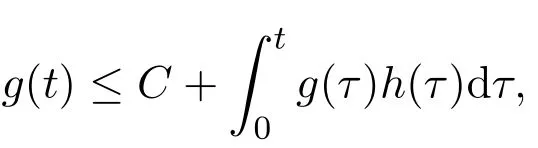
for allt>0,whereC>0is a positive constant,the functionh∈L1(0,∞).Then there holds the following estimate

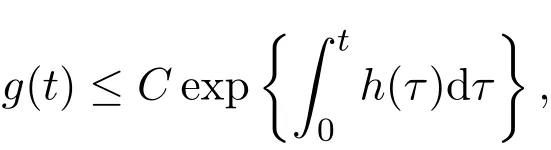
for allt>0.