A Preliminary Study on the Buoyancy Materials for the Use in Full Ocean Depth Manned Submersibles
CUI Wei-cheng,GUO Jian,PAN Bin-bin
(Hadal Science and Technology Research Center(Shanghai Engineering Research Center of Hadal Science and Technology),Shanghai Ocean University,Shanghai 201306,China)
0 Introduction
The hadal zones(6 500-11 000 m)represent the largest poorly understood areas in the ocean on earth.The remoteness and isolation of hadal zones makes these regions of inner space a particularly fertile field for scientific inquiry.Fundamental contributions to the understanding of processes responsible for plate tectonics and ocean chemistry,as well as the origins of life and mechanisms for speciation,have been made by scientists working at hadal zones in the past sev-eral decades[1].There is reason to believe that further discoveries can be made if adequate access to the deepest ocean depths can be provided.
The study of hadal science needs the special technology support such as landers[2],unmanned submersibles[3]and manned submersibles[4].Sending deployments to hadal zones are technically challenging and expensive,making it difficult to collect the biological samples necessary to generate a thorough species list.Within all the deep sea investigation platforms,manned submersible represents the highest technology challenge.Up to now,no operational manned submersibles exist which can dive to FOD.
After the success of the development of 7 000 m class manned submersible JIAOLONG[5-6],the first author of this paper strongly pushed forward the development of a FOD manned submersible in China.A new system called a movable laboratory for hadal trenches was defined which includes 3 FOD landers,1 FOD autonomous and remotely operated vehicle(ARV),1 FOD human occupied vehicle(HOV)and a special mothership of 4 800 ton displacement[7].In that paper,the technical issues encountered in the development of the FOD HOV were analyzed and later in another paper[8],the technical issues encountered in the design and manufacture of the manned cabin were discussed.The project for constructing the movable laboratory was jointly funded in 2014 by Shanghai Municipal government,Shanghai Rainbowfish O-cean Technology Co.Ltd and Shanghai Ocean University.
According to the first author’s judgment in 2012,the FOD manned and unmanned submersibles will be in the sponsor list of projects in the thirteenth five-year plan(2016-2020)and this has now been confirmed[9].The Chinese Ministry of Science and Technology had sponsored two FOD unmanned submersibles and one FOD manned submersible in 2016.In the design of the FOD HOV,the issue how to select the buoyancy material became one of the main obstacles.The purpose of this paper is to carry out a preliminary study on the buoyancy materials for the use in FOD manned submersibles.
1 An overview of syntactic foams
1.1 Basic concepts and brief history
Hollow Glass Microspheres(HGM)filled polymer has been developed as buoyancy-aid materials in deep-sea applications as early as 1950s[10].After the initial development,a more general class of materials called syntactic foams have been gradually developed[11-12].From many references,one can find that syntactic foam can be defined as a material consisting of hollow sphere fillers in a resin matrix,e.g.Ref.[13].The term ‘syntactic’comes from the Greek word‘syntaktikos’,which means ‘to arrange together’[14].Syntactic foams are a special kind of particulate composite materials made by a matrix(‘binder’)and a gas-filled aggregate(‘filler’)consisting of hollow spherical microspheres(often called microballoons(MBs))[15].These composite materials are classified as foams since they possess a structure formally similar to that of a cellular,gas-expanded,solidified liquid.The term syntactic indicates a constructed foam,in the sense that the material is manufactured by a specific mixing procedure of fillers and binders with appropriate volume fractions.However,the filler is randomly dispersed in the matrix,in a way to obtain a homogeneous and isotropic macroscopic behavior.
Shutov[13]had carried out a comprehensive and detailed survey on syntactic foams before 1986 with an emphasis on USSR.Other general introduction materials include John and Nair[14]and Pinisetty et al[15].The filler microspheres may be glass,polymeric,carbon,ceramic,or metallic.Microspheres are 1-500 μm in diameter,have a wall thickness of 1~4 μm,bulk densities of 70-500 kg/m3,and apparent densities of 50-250 kg/m3.Hollow macrospheres(1-100 mm in diameter)are also used as fillers for some syntactic foams[13].
The binder(or matrix)used in syntactic foams can be polymers,metals,or ceramics.Polymer matrices have been the most extensively used in syntactic foams.The polymer materials used in syntactic foams must have low viscosity,easily controlled gelation time,a small exothermal effect during curing,low curing shrinkage,good adhesion and wettability to the filler,and compatibility with modifiers and fillers,including diluents,plasticizers,dyes,and flame retardants[13].Polymeric materials are generally classified into thermoplastics and thermosets.Thermoplastics are polymeric materials that soften upon heating and harden upon cooling.The process is reversible and the material can be remolded by heating.Thermosetting polymers are polymeric materials that ‘set’ or cross-link upon heating.The cross-linking process is the formation of chemical bonds between the long carbon chains.The cross-linking process is irreversible.Once set,the thermosetting plastic cannot revert to its prior stage.The important thermosetting polymers used in syntactic foams are epoxies,phenolics,cyanate esters,etc.Thermosetting matrices have been the most widely used because of their availability,well-established processing technologies,existence of large databases,and low material cost[14].Thermosetting syntactic foams have many advantages compared to thermoplastic ones.The most important one is that thermosetting syntactic foams can be processed at much lower temperatures compared to thermoplastic syntactic foams,thereby reducing the material and energy costs for processing.Moreover,thermosetting resins have less solvent sensitivity and are not negatively affected by cleaning solutions.
Conventional plastic foams,wood,glass foam,and plastics cannot withstand pressures of the order of 60 MPa,and metal foams corrode.Thus only syntactic foams meet all the specifications for deep sea manned submersibles,and those of epoxy and glass,or epoxy and carbon,are the best.The low water absorptivity and good resistance to hydrostatic pressure make syntactic foams very useful for marine and submarine construction.Materials to be used for deepsea application must have[11]:(1)Low compressibilities at high hydrostatic pressure;(2)Low thermal expansion coefficients;(3)Low water absorption;and(4)Good fire resistance.More comprehensively,the following should be taken into consideration when choosing the type of solid syntactic foams for deep manned submersibles[17]:
·Specific gravity of the material
·Crush point and safety factor
·Shrinkage due to pressure,i.e.,loss of buoyancy with depth
·Abrasion resistance,brittleness
·Potential protective coatings
·Available shapes and machinability,including hazardous material requirements
·Water absorption and thus loss of buoyancy
·Placement and stability considerations
·Ability to modify in the future
Up to now,for all the deep manned submersibles such as AINVIN(4 500 m)and SEACLIFF(6 000 m)of USA,MIR I(6 000 m),MIR II(6 000 m),CONSUL(6 000 m)and RUS(6 000 m)of Russia,NAUTILE(6 000 m)of France,SHINKIN6500(6 500 m)of Japan,JIAOLONG(7 000 m)and SHEN HAI YONG SHI(4 500 m)of China,the currently used syntactic foams together with the currently available syntactic foams for full ocean depth manned submersibles are all made of HGM filled epoxy and therefore,only these two are further discussed in this paper.
Glass microspheres are widely used because of the strength of the glass and the substantial difference between the elasticities of glass and polymers.Fig.1 shows macroscopic(a)as well as microscopic;(b)views of glass microspheres.Sodium and borosilicate glasses are generally used,but some low-cost‘Sirasu’ brand microspheres have started to be manufactured in Japan using volcanic glass and ashes[13].

Fig.1 Macroscopic(a)As well as microscopic;(b)Views of glass microspheres[17,Courtesy Trelleborg]
The mechanical properties for glass material are given in Tab.1[18],and the average tensile strength compares favorably to the well-documented tensile strengths of glass fibers,e.g.BRATT et al[19].

Tab.1 Basic properties of various glasses[18]
Based on the glass properties,one can design or predict the mechanical properties of hollow glass microspheres(HGMs).The HGM model is shown in Fig.2 and the physical and mechanical parameters or properties include internal radiusri(),outer radiusro(),medium ra-dius (rm),radius ratio ( )η,density of the microballoon (ρmb),uniaxial compressive strength(σcmb),hydrostatic compressive strength (σpmb).
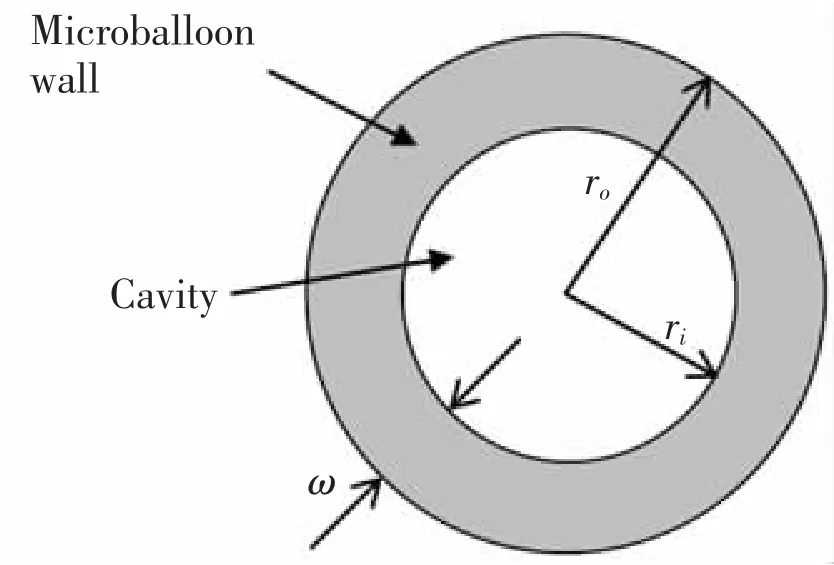
Fig.2 Notations used to define various physical parameters for microballoons[20]

The density of MBs is of interest in determining other mechanical properties or can be used to distinguish between grades of MBs.The comparison between the calculated density by Eq.(5)and the measured density of actual microballoons produced by 3M company from reference[20]is given in Tab.2.It can be seen that the calculated density is about 98%of the measured density.

Tab.2 Comparison between the calculated density by Eq.(5)and the measured density of actual microballoons produced by 3M company[20]
With the growth in the use of MBs and the technology associated with their production,so too must the ways of characterizing MBs grow.Carlisle et al[18]discussed extensively the structure and mechanical properties of micro and macro balloons,with a particular emphasis on the test techniques.In that paper,the measurement and prediction of the uniaxial compressive strength of a MB (σcmb)is given.However,for the syntactic foam used in a deep manned submersible,we are more interested in its compressive strength under hydrostatic pressure (σpmb).
Let us first treat it as the same as a perfect spherical sphere shown in Fig.2,there are two possible failure modes.One is the maximum stresses reaching the tensile strength(the same as the yield strength since glass is a very brittle material)and the other is elastic buckling leading to collapse.The minimal external pressure of these two failure modes is defined as the ultimate compressive strength of this sphere (σpmb).For the first problem it is mainly a stress analysis while for the second problem it is a stability analysis.
The stresses in a perfect spherical shell under uniform external pressure can be calculated by the following formula:

If the tensile strength of the glass is regarded to be the maximum stress allowed in the sphere,then the pressure at stress collapse of the sphere can be expressed as

For perfect spherical shells,the critical elastic buckling load of a complete sphere under external pressure was first derived by Zoelly in 1915[21].His equation is

From Eq.(7)and Eq.(8),one can see that the minimal external pressure from the two failure modes depends on material property and the ratio of t/rm.If we take glass as an example,and assuming the mechanical properties of Tab.1,then one can plot two curves of py~t/rmand pcr~t/rm,as shown in Pan and Cui[21].From the figure,one can see that when t/rmsatisfies the following condition,

then the tensile strength is higher than the elastic buckling strength.This requires that η should not exceed 0.936(or t/rmis greater than 0.066).Otherwise,it is a thinner sphere and it will fail by elastic buckling.
From Tab.2,one can see that the actual η will always be greater than this critical value,thus,the failure mode will be elastic buckling dominated and the ultimate pressure the HGM can resist can be calculated by Eq.(8).Bratt et al[19]have tested a series of HGMs,the average experimental results are compared with Eq.(8)in Fig.3.It can be seen that the agreement is very good especially for thinner spheres.However,for HGMs used for FOD,they may approach or slightly exceed the critical value of ηc=0.936 or t/rm=0.066.From Fig.3 one can see that in this region,the strength predicted by Eq.(8)is on the conservative side.

Fig.3 Comparison of experimental isostatic buckling pressure for individual HGMs with Eq.(8)
The experimental data is the average curve from Fig.1 of Ref.[19].
In all HGMs,defects either on a macroscopic or microscopic scale act as strength limiting flaws.These flaws are either in the initially formed spheres or are created by the foam bonding process.Thus,variation of sphere properties caused by defects resulting from the forming process must be considered as an integral part of the success of the application.This may explain why the formula used by Carlisle et al[22]is only 31%of Eq.(8).
Epoxy resins are characterized by the presence of at least two epoxy groups within their molecular structure.The chemistry of epoxies and the range of commercially available variations allow cure polymers to be produced with a very broad range of properties[14]and a set of typical values are given in Tab.3.
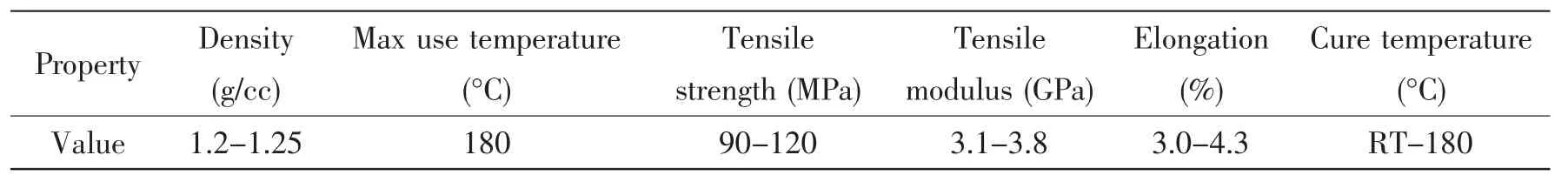
Tab.3 Typical properties of a representative epoxy[14]
1.2 Property
Syntactic foam is known to possess low density,high stiffness,excellent compressive and hydrostatic strength and good impact behavior[13].Unlike most other foams,syntactic foam is a material whose density before curing is the same as that after curing.Such predictability and attribute are advantageous in the manufacturing process.
The properties of a syntactic material can be varied quite significantly by changing the filler type,the binder-filler ratio,the process and hardening conditions,and the physicochemical processes at the binder/filler interface.HGM filled epoxy has been widely investigated due to its high specific strength,relatively high thermal stability,low thermal conductivity,and excellent damage tolerance,which are desirable properties of composites[16].
The properties of HGM/epoxy concerned include density,tensile strength,compressive strength,elastic modulus,Poisson’s ratio,flexural strength,crushing pressure under hydrostatic pressure,water absorption rate,etc and their measurement methods have all been standardized as various ASTM standards.In deep sea,the main loading is hydrostatic compression.However,as can be seen from these standards,there is no standard procedure for testing material under pure hydrostatic pressure.Ref.[23]has presented a new characterization technique based on buoyancy loss measurement under hydrostatic pressure and this can be acted as a starter for the further study.
The materials made from epoxy binders and glass microspheres were usually believed to be the strongest syntactic foams.However,several papers,e.g Refs.[24-25]have shown that,when carbon microspheres replace those of glass,the material could become stronger,more water resistant,and more capable to withstand hydrostatic pressure.However,up to now,there is no commercial product available.
Two major factors governing the strength and elasticity of a syntactic foam are the residual internal stresses in its bulk and the adhesion strength between the binder and the filler.Accordingly,an obvious way of improving these parameters is to use strong binders and to strengthen the microspheres.Simply rejecting defective spheres can increase a syntactic foam’s strength by 15%-20%.Kenyon[26]applied an elastic adhesive to the filler which not only reduced the stresses at the binder-filler interface but also altered the way the material strength depended on the microsphere concentration.Elastic binders increase the unit elongation of a syntactic foam from 1%to 10%,and its ultimate tensile strength to 30 MPa.
Rizzi et al[27]carried out a study on the mechanical behavior of a syntactic foam made with an epoxy resin matrix embedding randomly dispersed hollow glass microspheres.The stress/strain curves of the uniaxial tests are reported in Fig.4.Tensile strength σt=15.63 MPa is about 55%of the compressive strength σc=28.39 MPa.The observed responses are in qualitative agreement with the behavior normally displayed by syntactic foams with glass microspheres[15].The response under tension is perfectly brittle with rupture on a section perpendicular to the loading axis.Compression behavior is rather ductile,with a softening post-peak branch which tends to stabilize on a horizontal plateau at residual strength.Loading/unloading paths performed in some of the compression tests showed that elastic stiffness degradation is not particularly significant[27].The collapse mechanism is due to strain localization along a shear band inclined to an angle of about 45°with respect to the loading axis.The band separates the specimen into two parts that glide with respect to each other:interlocking and friction govern the behavior after the onset of strain localization and may explain the residual strength observed for the nominal stress/strain curves.
Experimental results in Fig.4 also showed that different elastic stiffnesses in tension and compression(bimodulus material):Young’s modulus in tension Et=2 200 MPa is about 35%greater than that in compression Ec=1 600 MPa.This phenomenological feature is not normally reported in the literature on syntactic foams.Poisson’s ratio was rather unaffected by the sign of the applied stress:the average value of ν=0.34 was recorded.

Fig.4 Uniaxial nominal stress/strain curves of syntactic foam Tencara 2000TMin tension and compression(traction positive)[27]
The shear modulus of about G=780 MPa,which was measured on torsion tests on speci-mens of the same type as those of the tensile tests,is quite in agreement with a shear modulus evaluated with the classical formula for isotropic linear elastic materials where the tensile modulus is taken as Young’s modulus would be the intrinsic elastic stiffness of a syntactic foam without unintentionally included voids.Hypothesis of isotropy seems consistent for that case.
Concerning the mechanical behavior,syntactic foams display at best uniaxial compression strengths of about 100 MPa,hydrostatic compression strengths around 150 MPa,uniaxial tension strengths of about 25-30 MPa,tensile Young’s modulus around 2 500-3 000 MPa.The behavior in compression is quite ductile,whereas tensile response is rather brittle[15].DeRuntz and Hoffman[28]reported an experimental investigation on an epoxy matrix/hollow glass microspheres foam with low degree of dispersed air bubbles,tested under biaxial and triaxial stress states:the failure locus is closed in the principal stress space so that the material exhibits a finite strength under triaxial compression.The hydrostatic pressure/volumetric strain diagram takes a typical sigmoidal form,with a central part at almost constant pressure which corresponds to progressive sphere crushing[15].Other experimental investigations on the mechanical behavior of syntactic foams are available in the literature;most of them are devoted to the determination of Young’s moduli and uniaxial tension and compression strengths,often seen as a function of the weight fractions of the constituents;the experimental results are frequently compared to predictions based on analytical and FE micromechanical computations.This will be further discussed in section 1.3.
The water absorption and resistance to hydrostatic pressure are one of the most concerned properties for syntactic foams.The differences in the absorptivities of different syntactic foams are due to the binder chemistry,filler type,and filler concentration.Hobaica and Cook[11]demonstrated that for microsphere concentrations below 67 vol%,syntactic-foam water absorptivities are virtually independent of the apparent density,but above that concentration the absorptivities rise rapidly due to the loss of binder integrity and the appearance of cavities and ducts.Therefore,67 vol%can be regarded as the upper limit of the microsphere volume fraction in a syntactic foam.
Syntactic foams absorb considerable amounts of water only at hydrostatic pressures above a certain value,but absorb very little below that value.A good feature of syntactic materials is their stability in cyclic hydrostatic tests.They can withstand many cycles of alternating pressure under that value.Experience has shown that,even under hydrostatic pressure up to 75%of the collapsing pressure,syntactic foams do not absorb large amounts of water[13].Epoxy and glass syntactic foams resist water the best,but after prolonged exposure they weaken considerably,as the binder-filler adhesion fails.Filler dressing improves the water resistance.
Other properties of less concern are thermal properties,dielectric properties and other properties discussed in Shutov[13]and they are not further discussed in that paper.
1.3 Modeling of mechanical properties
In order to improve the properties of HGM filled epoxy syntactic foams,a general theory covering their deterioration and deformation should be developed.Establishing a quantitative understanding of the structure-property relationships for syntactic foams under hydrostatic loading condition would be very important for the proper design of buoyancy materials for a deep manned submersible.In order to establish a reliable mathematical model for the structure of a syntactic foam which should be reasonably simple and accurate,many attempts have been made.Basically,they can be divided into either macrostructural models or microstructural models.
For a macrostructural model,the syntactic foam is treated as a homogeneous uniform material.General theory of composite materials may be used to develop a macrostructural model.Dementyev and Tarakanov[29]proposed a macrostructural model of syntactic foam morphology to calculate the strength properties of an epoxy foam with phenolic microspheres.They made two restrictive assumptions,i.e.that the mechanical properties of the microsphere walls and the binder are the same,and that the volume fraction of filler is substantially smaller than that of the matrix.These two assumptions are certainly not valid for HGM/epoxy syntactic foams.It is our firm belief that the mechanical parameters of a highly filled syntactic foam must in general be analyzed taking into account the interactions at the binder-filler interface and the system’s stresses since both of these factors are important for highly filled systems.Therefore,microstructural models are more appropriate.
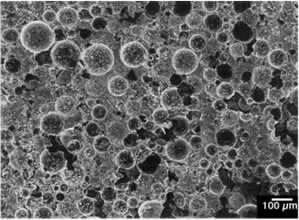
Fig.5 Scanning electron micrograph of three-phase structure of syntactic foam[30]
Syntactic foams are normally classified into two-phase and three-phase systems.The closely packed arrangement of microspheres leads to two-phase syntactic foams.However,this is only a hypothetical situation since during the processing of syntactic foams,entrapment of air is inevitable,which leads to three-phase syntactic foams.Sometimes,the voids are intentionally created to reduce density of syntactic foams[13].Fig.5 shows a photograph of a real HGM/epoxy where the voids can be clearly identified.A schematic of two-phase and three-phase syntactic foams is shown in Fig.6 while in this section,only the microstructural models for three-phase syntactic foams are reviewed.
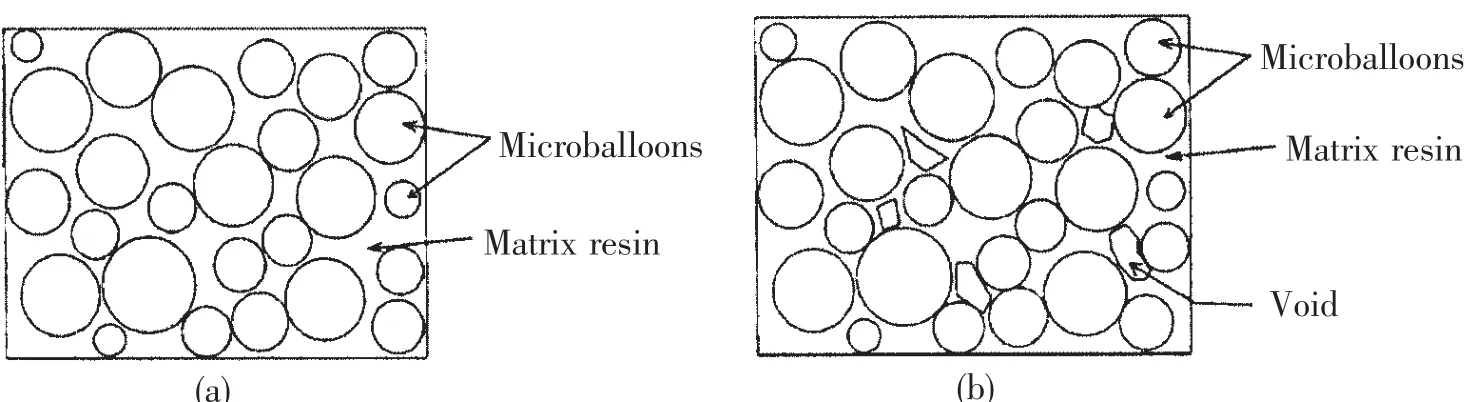
Fig.6 Schematic showing the features in(a)Two phase and(b)Three phase structures ina syntactic foam material[31]

Fig.7 Schematic structure of syntactic foams showing microballoon and matrix porosities[20]
Fig.7 is the actual picture for establishing the microstructural model of a three-phase syntactic foam where the wall thicknesses of microballoons are shown[20].
DeRuntz and Hoffman[28]have made an interesting and promising attempt of estimating the strength parameters of syntactic foams.They applied the concepts and mathematical apparatus of the mechanics of discontinuous media and the theory of plasticity.Basically,these models are all based on the same(microstructural)principle,viz.that there is an elementary structural unit which is called a representative elementary volume(REV)[32]that can be described and then used for calculation.Microstructural models are a first approximation to a general theory describing the deformation and failure of gas-filled materials.Marur[33]has thoroughly studied the FE unit cell methods used in the study of micromechanics of particulate composites.The unit cell methods presume that a periodic repetition of a representative volume of the material would represent the bulk material.These repetitive unit cells can be two-dimensional plane strain,axisymmetric cylindrical,axisymmetric spherical or 3D cubic array idealizations.The choice of a particular unit cell for the analysis of a composite is a trade-off between the cost of computation and the closeness of the numerical representation with the real material.Typical REVs proposed in the literature are shown in Figs.8-10.

Fig.9 Representative elementary volume(REV)of multiple MBs[37-45]

Fig.10 General model consisting of cubic cells.Three types of unit cells as building blocks for the general model:(a)Simple cubic(SC);(b)Face centered cubic(FCC);and(c)Body centered cubic(BCC)unit cells with diagonal cross sectional view.Each unit cell contains one large sphere representing a group of large microspheres[46]
Some of the complicated microstructural models such as Fig.9 and Fig.10 have been found not only to predict the strength parameters of a syntactic foam,but also to calculate some of its other properties,from the known properties of the foam’s main constituents,i.e.the binder and filler.Gupta et al[45]showed their solid model of syntactic foam microstructure as in Fig.9 is useful in obtaining higher mechanical properties and lower moisture absorption.
Let us now introduce some methods for calculating the strength properties of syntactic foams.
The density of the three-phase syntactic foam (ρsf)can be calculated by the following formula:

where ρmb,ρrare the densities of microballoons and resin respectively,φmb,φvoidare the volume fractions of microballoons and voids respectively.For a normal HGM/resin,φvoidis about 1%-6%[47].
Turcsanyi et al[48]proposed the following formula to calculate the uniaxial tensile strength of syntactic foams,

where σtSF,σtmare the uniaxial tensile strengths of syntactic foam and matrix respectively,Vfis the volume fraction of HGMs,A is the packing factor for spherical microballoons,its value is about 2.5,B is a parameter reflecting the bonding strength between filler and matrix.
Lavrenyuk suggested the following expression for the strength of a syntactic foam in axial compression[13].

where σcsfis the ultimate strength of syntactic foam in axial compression,σcylis the cylindrical strength of the filler,and Esf,Ef,Emare the elastic moduli of the syntactic foam,the filler,and the matrix,respectively.
Krzhechkovsky et al have calculated the deformability and long term strength of syntactic foams under external hydrostatic pressures[13].Their deformability ε()τas a function of time is given by:

where τ is the duration of pressure hardening,rmis mean radius of sphere,P is the external pressure,G and J are the instantaneous and long term shear moduli,K is the bulk elastic modulus, τ*is the relaxation time, χ1and χ2are dimensionless numbers that depend on the filler concentration φmbin the foam,as shown in Eqs.(14).

Elastic deformations are found if τ*=0.The ultimate long term strength can be obtained from criteria given in Ref.[13].The hydrostatic pressure that the foam can withstand for an infinitely long time,P∞is given as

where Pcolis the collapsing pressure,v is Poisson’s ratio,and Emand Hmare the instantaneous and long term elastic moduli of the matrix.
The dimensionless numbers χ3and χ4are determined as:

where σtSF, σcSFand σshearare those stresses that in axial tension,compression,and shear,respectively,cause the syntactic foam to fail in the same lifetime;here ηPand φmbmay vary only from 0.5 to 0.65.

Fig.11 Calculated relative collapsing pressure of syntactic foams (Pr /σc )versus volume fraction of filler,φmb[38]
The influence of the volumetric concentration of the filler φmbon the hydrostatic strength has been discussed in Ref.[13].They assumed that the filler particles were spheres,had all the same dimension,and that φmbdid not exceed 30%.The relative concentration in a working cell is assumed to be C=σmb/D,where D is the packing factor.Values for the relative collapsing pressure (Pr),as it depends on C,were found by solving numerically and simultaneously the equilibrium equation for a spherical element,given a filler concentration C,and the equation that gives the onset of the limiting strength of the syntactic foam[13].Fig.11 shows the results for a syntactic foam with σcaxial/σchydro=0.657,where σcaxialand σchydroare the ultimate stresses for axial and hydrostatic compressions.The collapsing pressure is given by
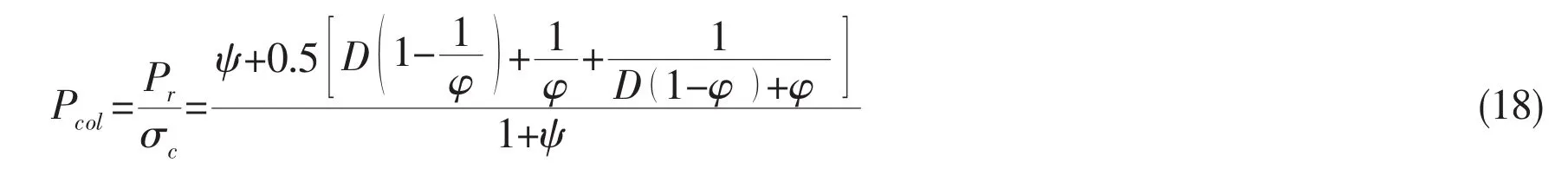
where

where Kmand Gmare the bulk and shear moduli of the matrix,respectively.
Calculations have shown that at φmb=15%,the hydrostatic strengths of a syntactic foam decreases by 35%-40%,and for φmb=28%by 60%as compared to the matrix.Note that for practical applications the hydrostatic strength of a syntactic foam does not depend on scale factors(between 1 and 100)nor on the shape of the final article[13].
It does not appear that the approach of either changing the wall thickness or volume fraction has a more prominent effect on the compressive strength.It is known that some of the HGMs fracture during syntactic foam synthesis and the broken particles act like solid fillers in the resin and increase the density of the syntactic foam.In addition,mechanical mixing process of syntactic foam causes entrapment of air voids in the resin,which can be up to 5-10 vol%.Such voids result in decrease in density of syntactic foams.Despite the possibility of having defects in syntactic foams,the compressive strength does not seem to show any strong dependence on one parameter and stays on course with respect to the foam density[16].
It is observed that the modulus of syntactic foams increases with increasing volume fraction of thick-walled particles,whereas modulus decreases with the increasing volume fraction of thin walled particles.The ratio of modulus of the particle material to the matrix material de-fines the limit between the thick-and thin-walled particles for a given particle-matrix system.Extensive theoretical and simulation studies have been conducted on determining the failure mode of particles in syntactic foams.It is found that shear failure and particle crushing may occur before particle wall buckling in syntactic foams[49].
2 Special issues and potential solutions related to syntactic foams for FOD manned submersibles
2.1 Design standard
If we go through all the existing design rules from major classification societies such as ABS[50]and CCS[51],there is no mention for the requirement of safety factor for buoyancy materials in most of the classification society rules except the latest version of CCS[51]in which a safety factor of 1.5 is required.

Fig.12 Syntactic foam taken to crush depth[52]
Therefore,in the past,the safety factor is totally left to be decided by the chief designer of the submersibles.For the unmanned submersibles,the crush depth of the buoyancy material is usually 1.1 times above the service depth.However,this is certainly too low for the manned submersibles.For the famous manned submersible Alvin,in the process of updating it from 4 500 m to 6 500 m,a decision of using 1.5 as the safety factor has been made[52].The latest Alvin specification can readily be attained online.Fig.12 shows a typical high-performance material,rated to approximately 6 500 m(67 MPa),that went well beyond its test depth.As can be seen,the material,designed(with safety factor)for 99.974 6 MPa(SFdesign=1.49),eventually crushed at a pressure of 110.316 8 MPa(SFactual=1.65).
2.2 Current Status for the selection of buoyancy materials
Currently only few companies are claimed to be able to provide the buoyancy material for the full ocean depth.These are:(1)Trelleborg Offshore[53]:DS-39,its density is 0.609 g/cm3,the crushing pressure is 137.8 MPa;(2)Engineered Syntactic Systems[54]:HZ-42,its density is 0.67±0.03 g/cm3,the crushing pressure is 165.0 MPa;HZ-45,its density is 0.72±0.03 g/cm3,the crushing pressure is 165.0 MPa;(3)Ron Allum Deepsea Services(RADS)[55],DR11,its density is 0.69±0.01 g/cm3,the crushing pressure is 152.0 MPa.
In China,Marine Chemical Research Institute Co.Ltd in Qingdao can produce a buoyancy material with a density of 0.676~0.70 g/cm3,the crushing pressure is about 142 MPa[56].Technical Institute of Physics and Chemistry of Chinese Academy of Sciences in Beijing can produce two types of buoyancy materials for full ocean depth,one is with a density of 0.64 g/cm3and a crushing pressure of above 140 MPa and the other is with a density of 0.68 g/cm3and a crushing pressure of above 155 MPa[57].
2.3 Issues to be considered for a lower Safety Factor design
The maximum ocean depth currently measured is 11 034 m and it is located at the Challenger Deep in the southern Mariana Trench in the western Pacific Ocean.The maximum hydrostatic pressure there is a function of depth,salinity and temperature.Based on a wellknown equation,the maximum pressure at the Challenger Deep can be calculated as 113.8 MPa.Thus,if a safety factor of 1.5 is used,the crushing pressure for the buoyancy material should be greater than 170.7 MPa.From previous section one can see that none of the companies can provide such a high strength buoyancy material.There are generally two solutions to this situation.
One is to ask one manufacturer to produce a new type of syntactic foam to satisfy the strength requirement.As one is aware,in that case,the strength of the glass microballons may need to be increased.The density of the syntactic foam may be further increased.This will cost much time and money.The second solution is to choose one of the existing syntactic foam and measure its water absorption property thoroughly and then shorten the safe life period for the buoyancy material.For example,if the safety factor is 1.5,the design life specified in the design rule is 20 years[51].Then if we lower the safety factor to 1.4,we may specify the safe life for this buoyancy material as 10 years or less.How to define the safe life depends on the water absorption performance under hydrostatic pressure and prolonged time.
In general,the HGM/epoxy syntactic foam has the following water absorption property.As the pressure increases,the water absorption rate increases.When the pressure is kept to be constant,the water absorption rate increases as the time prolongs,see Fig.13.The water absorption rate will be very low and almost constant for a large range of pressure or time.However,when it starts to increase,it will increase quite quickly either with time and pressure.Therefore,we suggest the following engineering criterion for the safe use of a buoyancy block.When the water absorption rate is greater than 1%for a particular block,it must be replaced by a new block.Therefore,the maximum time for a block to reach the 1%water absorption rate is defined as the safe life period for the block.
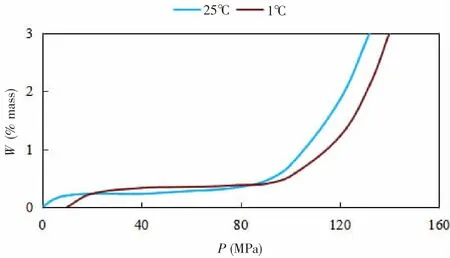
Fig.13 Water absorption(W)versus hydrostatic pressure(P)at two different water temperatures,for an epoxy syntactic foam with glass microspheres as filler[13]
From Fig.13,one can see that if the collapse pressure is 140 MPa,the maximum pressure for a constant water absorption rate is about 100 MPa.If we assume this is a general behavior for HGM/epoxy buoyancy material,then if we need the buoyancy material to have a constant water absorption rate at 115 MPa,the minimum collapse pressure should be 115×140/100=161 MPa,the minimum safety factor for the buoyancy material is 161/115=1.4.This assumption needs to be experimentally confirmed before the final decision to lower the safety factor from 1.5 to 1.4 is taken.
2.4 Technical status in China
In comparing with those deep submergence technology of developed countries such as the United States,Japan,Russia and others,the research and development of solid buoyancy materials used in deep submergence vehicles in China started quite late.The early developed buoyancy materials are mostly foamed plastic made from polyurethane or epoxy resin.In comparing with the similar buoyancy materials available from abroad,the domestically made buoyancy material is much cheaper,but with a poor reliability.After a period of immersing in the water,the buoyancy will gradually decrease due to the water absorption of the buoyancy material.The maximum working depth is only about 400 m.
Harbin Shipbuilding Engineering Institute successfully developed the first generation of the solid buoyancy material in China in 1984.It mainly consisted of the hollow polymer microballoons,hollow glass microballoons and epoxy resin.The density is 0.5 g/cm3,and its compressive strength under hydrostatic pressure is 28.87 MPa[58].In 1995,Marine Chemical Engineering Institute in Qingdao developed a lightweight buoyancy material using the chemical foaming method.Its density is 0.33 g/cm3,and it can be used in a submersible of 500 m diving depth.It has been successfully used in underwater robot,the diving bell and the towed antenna.Later,she has developed another type of lightweight buoyancy material with a density of 0.55 g/cm3and the compressive collapse strength of 50 MPa.This can be used in deeper submersibles up to 4 000 m diving depth[59].
Chen et al[59]have provided a detailed introduction to the domestic development history of buoyancy materials.In general,in 2002 when the Project to develop a 7 000 m deep manned submersible‘JIAOLONG’ was launched,the technical difference between China and the international advanced level is very large and China could not produce the required buoyancy materials at that time.Faced with this situation,the Ministry of Science and Technology adopted an encouraging policy and since 2000 many research institutes and universities started the research and development of solid buoyancy materials.They used either hollow glass microballoons or hollow ceramic microballoons as the filler.Various types of solid buoyancy materials have been developed.At present,the following organizations can produce some sort of solid buoyancy materials.They include Harbin Engineering University,Marine Chemical Engineering Institute,Beihang University,Beijing University of Science and Technology,Tianjin University,Ocean University of China,Zhejiang University,Central South University,Dalian University of Technology,Wuhan University of Technology,National Ocean Technology Center,Northwestern Polytechnical University,Technical Institute of Chemistry and Physics of the Chinese Academy of Sciences,No.710 and No.725 research institutes of China Shipbuilding Industry Cooperation.However,most of them are still on the stage of laboratory research and they can produce the buoyancy material for 5 000 m or less diving depth.
At present,only the Marine Chemical Engineering Institute[56]and Technical Institute of Chemistry and Physics of the Chinese Academy of Sciences[57]have the capability to produce solid buoyancy materials for full ocean depth in China.Their data have been given in section 2.2 and one can find that neither organization can produce a buoyancy material with a density of less than 0.70 g/cm3and the collapse strength of larger than 161 MPa.Their buoyancy materials have not reached the advanced international level as compared with the products from three international companies[53-55].An in-depth study on their water absorption characteristics is needed to make the decision whether they can be used in the full ocean depth manned submersibles or not.
2.5 A potential method to reduce the density for large blocks
From above discussion,one can see that for full ocean depth manned submersible,the minimum collapse strength is around 160 MPa and the density of HGM/epoxy syntactic foam is around 0.67-0.72 g/cm3.This density is relatively too high for the submersible use.If we want to further reduce the density while keeping the collapsing strength,we may cast the buoyancy block by adding some ceramic macrospheres.As a matter of the fact,Trelleborg has already applied the concept[53].Trelleborg produces four different types(EL,TG,DS and VF)of syntactic foams to meet the broad range of requirements of its customers.VF Grade epoxy syntactic foams combine microballoons and macrospheres for ultra-light weight applications such as filling control surfaces on 688 class U.S.nuclear submarines.The Grade A shock-qualified castable syntactic foams are sold as kits for on-site/field use.However,VF Grade can only be used for about 1 000 m diving depth.
If we assume a solid buoyancy block of 1 m3,with the original density of 0.70 g/cm3.On each side leaves a free space of 200 mm for machining or bolting purposes.Inside the middle,the uniformly ceramic macrospheres can be added.If we assume the outer diameter of each macrosphere is 92 mm,its wall thickness is 1.6 mm,its density is 0.35 g/cm3[60].The arrangement of macrospheres is shown in Fig.14.Then the average density of the buoyancy block can be calculated as follows:V=109mm3,V=216×4/3×=904.778×
blocksphere463=8.806 7×107mm3,φ=V/V=0.088 067,ρ=
sphereblockblock0.7×(1-0.088 067)+0.35×0.088 067=0.67 g/cm3.If only 100 mm is left on each side,then V=51.2×107mm3,φ=
block8.806×7/51.2=0.172,then the density of the block is ρblock=0.7×(1-0.172)+0.35×0.172=0.64 g/cm3.Therefore,through this activity,the density of the big buoyancy block can be reduced from 0.7 g/cm3to 0.64-0.67 g/cm3.

Fig.14 Schematic arrangement of ceramic macospheres in a buoyancy block
3 Summary and conclusions
In the design of the full ocean depth(FOD)human occupied vehicle(HOV),the issue how to select the buoyancy material is one of the main key issues.In this paper,a preliminary study has been carried out on the various issues related to the selection of buoyancy materials for the use in FOD manned submersibles.The basic concepts and development history of HGM/epoxy syntactic foams have been overviewed.Theoretical and numerical models to predict the properties of HGMs and syntactic foams are presented.Special issues and potential solutions related to syntactic foams for FOD manned submersibles have been discussed.Through this preliminary study,the following conclusions can be drawn:
(1)Hollow glass microballoons/epoxy syntactic foam is the only commercially available buoyancy material for the FOD manned submersibles,but carbon microballoons may provide better performance.
(2)Currently,only China Classification Society has required the buoyancy material to have a safety factor of 1.5 while other classification societies leave this issue to designers.Whether this safety factor is adequate or over-conservative needs to be further studied.
(3)It is found that no existing companies can produce buoyancy materials with a safety factor of 1.5.Based on an extrapolation from limited experimental data,the minimum safety factor may be reduced to 1.4,but this needs to be confirmed by experiments.
(4)The density of the currently available buoyancy materials is a bit high and a new method to add ceramic macrospheres to a large buoyancy block is proposed and it may reduce the density from 0.7 g/cm3to 0.64 g/cm3.
(5)It is expected that this study can lay a foundation for the further study based on existing available buoyancy materials rather than producing another new brand which costs both time and money.
[1]Jamieson,Alan.The Hadal Zone:Life in the deepest oceans[M].Cambridge:Cambridge University Press,2015
[2]Jamieson A J,Fujii T,Solan M,Priede I G.HADEEP:Free-falling landers to the deepest places on earth[J].Marine Technology Society Journal,2009,43(5):151-160.
[3]Fletcher B,Bowen A,Yoerger D R,Whitcomb L L.Journey to the challenger deep:50 years later with the nereus hybrid remotely operated vehicle[J].Marine Technology Society Journal,2009,43(5):65-76.
[4]Kohnen W.Human exploration of the deep seas:Fifty years and the inspiration continues[J].Marine Technology Society Journal,2009,43(5):42-62.
[5]Cui W C.Development of the JIAOLONG deep manned submersible[J].Marine Technology Society Journal,2013,47(3):37-54.
[6]Cui W C,Liu F,Hu Z,Zhu M,Guo W,Liu C G.On 7 000 m sea trials of the manned submersible ‘JIAOLONG’[J].Marine Technology Society Journal,2013,47(1):67-82.
[7]Cui W C,Hu Y,Guo W,Pan B B,Wang F.A preliminary design of a movable laboratory for hadal trenches[J].Methods in Oceanography,2014,9:1-16.
[8]Cui W C,Wang F,Pan B B,Hu Y,Du Q H.Chapter 1:Issues to be solved in the design,manufacture and maintenance of a full ocean depth manned cabin,in advances in engineering research[M].Volume 11,Editors:Petrova V M.2015-3rd Quarter,Nova Science Publishers,2015.
[9]Cyranoski D.What China’s latest five-year plan means for science[J].Nature,2016,531:524-525.
[10]Allen W.Syntactic floatation material for deep-submergence vehicles[J].J Cell.Plast.,1955,2:157-161.
[11]Hobaica E C,Cook S D.The characteristics of syntactic foams used for buoyancy[J].J Cell.Plast.,1968,4(4):143-148.
[12]Kallas D H,Chatten C K.Buoyancy materials for deep submergence[J].Ocean Engineering,1969,1(4):421-424.
[13]Shutov F A.Syntactic polymer foams[J].Advances in Polymer Science,1986,73/74:63-123.
[14]John B,Nair C P R.Chapter 13:Syntactic foams[K].in Dodiuk H and Goodman S H.(Ed.),Handbook of Thermoset Plastics(Third Edition).Elsevier Inc.,2014:511-554.
[15]Luxmoore A R,Owen D R J.Syntactic foams[M].In:Hilyard,N.C.(Ed.),Mechanics of Cellular Plastics.Applied Science Publishers,London,1982:359-391.
[16]Pinisetty D,Shunmugasamy V C,Gupta N.Chapter 6:Hollow glass microspheres in thermosets-Epoxy syntactic foams[M].in Amos S E,and Yalcin B(Ed.).Hollow Glass Microspheres for Plastics,Elastomers,and Adhesives Compounds,A volume in Plastics Design Library,Elsevier Inc.,2015:147-174.
[17]Christ R D,Wernli R L.Chapter 5:Vehicle design and stability[M].in The ROV Manual(Second Edition),A User Guide for Remotely Operated Vehicles.Elsevier Ltd.,2014:107-120.
[18]Carlisle K B,Chawla K K,Gladysz G M,Koopman M.Structure and mechanical properties of micro and macro balloons:An overview of test techniques[J].J Mater Sci.,2006a,41(13):3961-3972.
[19]Bratt P W,Cunnion J P,Spivack B D.Mechanical testing of glass hollow microspheres[J].Materials Science Research,1983,15:441-447.
[20]Gupta N,Nagorny R.Tensile properties of glass microballoon-epoxy resin syntactic foams[J].J Appl Polym Sci.,2006,102(2):1254-1261.
[21]Pan B B,Cui W C.An overview of buckling and ultimate strength of spherical pressure hull under external pressure[J].Marine Structures,2010,23(3):227-240.
[22]Carlisle K B,Brito V,Gladysz G M,Ricci W,Koopman M.Fabrication and finite element modeling of ellipsoidal macroshells[J].J Mater Sci.,2009,44:1449-1455.
[23]Gall M L,Choqueuse D,Gac P Y L,Davies P,Perreux D.Novel mechanical characterization method for deep sea buoyancy material under hydrostatic pressure[J].Polymer Testing,2014,39:36-44.
[24]Carlisle K,Koopman M,Chawla K,Kulkarni R,Gladysz G,Lewis M.Microstructure and compressive properties of carbon microspheres[J].J Mater.Sci.,2006b,41(13):3987-3997.
[25]Zhang L,Ma J.Effect of coupling agent on mechanical properties of hollow carbon microsphere/phenolic resin syntactic foam[J].Compos.Sci.Technol.,2010,70(8):1265-1271.
[26]Kenyon A S.Role of the interface in glass-epoxy composites[J].J Coll.Interface Sci.,1968,27(4):761-771.
[27]Rizzi E,Papa E,Corigliano A.Mechanical behavior of a syntactic foam:Experiments and modeling[J].Int J Solids Struct,2000,37(40):5773-5794.
[28]DeRuntz J A,Hoffman O.The static strength of syntactic foams[J].Journal of Applied Mechanics,Transactions of the ASME,1969,36(3):551-557.
[29]Dementyev A G,Tarakanov O G.Effect of cellular structure on the mechanical properties of plastic foams[J].Polymer Mechanics,1970,6(4):519-525.
[30]Gupta N,Woldesenbet E,Kishore.Compressive fracture features of syntactic foams-microscopic examination[J].J Mater Sci,2002,37(15):3199-3209.
[31]Sankaran S,Ravisekhar K,Raju G,Jagdishkumar M N.Characterization of epoxy syntactic foams by dynamic mechanical analysis[J].J MATER SCI,2006,41:4041-4046.
[32]L’Hostis G,Devries F.Characterization of the thermoelastic behavior of syntactic foams[J].Composites Part B,1998,29B:351-361.
[33]Marur P R.Numerical estimation of effective elastic moduli of syntactic foams[J].Finite Elements in Analysis and Design,2010,46:1001-1007.
[34]Antunes F V,Ferreira J A M,Capela C.Numerical modelling of the Young’s modulus of syntactic foams[J].Finite Elements in Analysis and Design,2011,47:78-84.
[35]Nian G D,Shan Y J,Xu Q,Qu S X,Yang Q D.Failure analysis of syntactic foams:A computational model with cohesive law and XFEM[J].Composites,Part B,2016,89:18-26.
[36]Yu M,Zhu P,Ma Y Q.Identification of the interface properties of hollow spheres filled syntactic foams:An inverse strategy combining microstructural modeling with Kriging meta model[J].Composites Science and Technology,2013,74:179-185.
[37]Bardella L,Alessandro Sfreddo A,Ventura C,Porfiri M,Gupta N.A critical evaluation of micromechanical models for syntactic foams[J].Mechanics of Materials,2012,50:53-69.
[38]Bardella L,Malanca F,Ponzo P,Panteghini A,Porfiri M.A micromechanical model for quasi-brittle compressive failure of glass-microballoons/thermoset-matrix syntactic foams[J].Journal of the European Ceramic Society,2014,34:2605-2616.
[39]Panteghini A,Bardella L.On the compressive strength of glass microballoons-based syntactic foams[J].Mechanics of Materials,2015,82:63-77.
[40]Gupta N,Ricci W.Comparison of compressive properties of layered syntactic foams having gradient in microballoon volume fraction and wall thickness[J].Mater Sci Eng A,2006,427(1):331-342.
[41]Mae H.Effects of local strain rate and micro-porous morphology on tensile mechanical properties in PP/EPR blend syntactic foams[J].Materials Science and Engineering A,2008,496:455-463.
[42]Mae H,Omiya M,Kishimoto K.Effects of strain rate and density on tensile behavior of polypropylene syntactic foam with polymer microballoons[J].Materials Science and Engineering A,2008,477:168-178.
[43]Tagliavia G,Porfiri M,Gupta N.Influence of moisture absorption on flexural properties of syntactic foams[J].Composites:Part B,2012,43:115-123.
[44]Zhang X,Wang P F,Zhou Y H,Li X T,Yang E H,Yu T X,Yang J L.The effect of strain rate and filler volume fraction on the mechanical properties of hollow glass microsphere modified polymer[J].Composites Part B,2016,101:53-63.
[45]Gupta N,Zeltmann S E,Shunmugasamy V C,Pinisetty D.Applications of polymer matrix syntactic foams[J].JOM,2014,66(2):245-254.
[46]Islam M M,Kim H S.Pre-mould processing technique for syntactic foams:generalised modelling,theory and experiment[J].Journal of Materials Processing Technology,2011,211:708-716.
[47]Woldesenbet E,Peter S.Radius ratio effect on high-strain rate properties of syntactic foam composites[J].J Mater Sci,2009,44:1551-1559.
[48]Turcsanyi B,Pukanszky B,Tudos F.Composition dependence of tensile yield stress in filled polymers[J].Mater Sci Lett,1988,7:160-162.
[49]Shams A,Aureli M,Porfiri M.Nonlinear buckling of a spherical shell embedded in an elastic medium with imperfect interface[J],Int.J Solids Struct.,2013,50(14-15):2310-2327.
[50]Rules for building and classing underwater vehicles,systems and hyperbaric facilities[S].Published by American Bureau of Shipping(ABS)in 2017.
[51]Rules for the classification and construction of diving systems and submersibles[S].Published by China Classification Society(CCS)in 2017.
[52]Walden B,Tessier N,Popenoe H.Newly developed specification and test Methods for syntactic foam buoyancy material for deep ocean manned submersible vehicles[J].Oceans,2010,52(4):1-9.
[53]Trelleborg eccofloat syntactic foams,trelleborg offshore boston[EB/OL].Inc.www.Trelleborg.com/AEM.
[54]Technical Data Sheet for HZ Grade Microsphere syntactic foam.Engineered Syntactic Systems[EB/OL].www.esyntactic.com.
[55]Structural Syntactic Foam Brochure.Ron Allum Deepsea Services(RADS)[EB/OL].www.ronallum.com.
[56]Liang X J,Liang Z X,Zhou Y,Wang J,Wang Q Y,Chen X.Properties of syntactic foams under full ocean depth[J].Thermosetting Resin,2016,31(5):38-41.(in Chinese)
[57]Wang P,Yan K Q,Pan S L,Zhang J J.Research development on solid buoyancy material for deep-sea application[J].Journal of Engineering Studies,2016,8(2):223-229.(in Chinese)
[58]Cheng P,Wang Y C.Research on improving property of buoyant material[J].Journal of Harbin Shipbuilding Engineering Institute,1993,14(4):64-69.(in Chinese)
[59]Chen X,Zhou Y,Lu W.Solid buoyancy materials[M].Beijing:Chemical Industry Press,2011.(in Chinese)
[60]Weston S,Olsson M,Merewether R,Sanderson J.Flotation in ocean trenches using hollow ceramic spheres[J].Marine Technology Society Journal,2009,43(5):110-114.
- 船舶力学的其它文章
- Numerical Simulations of the Effects of Marine Fouling on the Hydrodynamic Performance of Blade Sections
- Analysis of Effective Height of Green Water Loading for Tension Leg Platform Based on Statistical Process Control
- Seakeeping Prediction of SWATH with Three Dimensional Translating-pulsating Source Green Function
- Experimental Investigation on Motion Responses and Sectional Wave Loads of a Moored Semi-submersible in Deepwater
- Low-Cycle Dwell-Fatigue Life and Failure Mode of a Candidate Titanium Alloy Material TB19 for Full-Ocean-Depth Manned Cabin
- A Probability Distribution Model for SCFs in Multi-planar Circular Hollow Section DT-joints Subjected to Axial Loading

