基于砂滤层内水体积分数瞬态模拟的反冲洗速度优选
李景海,蔡九茂,翟国亮,刘清霞,张文正
基于砂滤层内水体积分数瞬态模拟的反冲洗速度优选
李景海1,2,蔡九茂3,翟国亮3※,刘清霞1,张文正3
(1. 安阳工学院土木与建筑工程学院,安阳 455000; 2. 安阳市水资源管理委员会办公室,安阳 455000; 3. 中国农业科学院农田灌溉研究所,新乡 453002)
为了对石英砂滤层反冲洗过程水的体积分数波动规律进行分析,并确定合理的反冲洗速度范围,该文采用数值模拟手段对滤层反冲洗过程水的体积分数进行三维动态模拟,采用Gambit软件建立了石英砂过滤器的几何模型,并对几何模型进行了网格划分,以Mixture模型做为反冲洗过程水的体积分数的数值模拟模型。以当量粒径分别为1.06、1.2和1.5 mm的3种石英砂滤层为研究对象进行动态模型。为了验证模拟结果的准确性,开展了室内模型试验,并将模拟结果与试验结果进行对比,结果显示,水的体积分数的最大模拟误差为5.64%,说明数值模拟结果是可信的。在使用模拟数据进行流场分析时,为了得出更具普遍性的结论,引入了反冲洗流化倍数的概念,最小反冲洗流化速度的倍数称为反冲洗流化倍数。在此基础上,分别分析了反冲洗流化倍数为1.1、1.3、1.5、1.7和1.9时,滤层高度分别为15、25和35 cm共3个横截面上,反冲洗过程水的体积分数随时间的变化规律。计算了水的体积分数的均值和标准偏差,分析了水的体积分数的均值和标准偏差随随反冲洗流化倍数的变化规律。在3个截面上水的体积分数均值基本相同的情况下,根据标准偏差的大小,判定滤层反冲洗的稳定性。由此得出,使反冲洗水的体积分数波动保持稳定的反冲洗流化倍数的临界值为1.7。当反冲洗流化倍数范围为1~1.7时,标准偏差适中,反冲洗效果理想。结果表明,对于均质石英砂滤层,反冲洗效果是否理想,决定因素是反冲洗流化倍数。该文可为砂过滤器的反冲洗运行机理提供参考。
灌溉;模型;计算机仿真;石英砂滤层;反冲洗;多相流
0 引 言
微灌技术是一项重要的节水灌溉技术[1-2],发展微灌技术是缓解水资源短缺的有效途径[3]。微灌砂过滤器做为微灌系统的重要组成部分,对于微灌装置的正常运行起着至关重要的作用。中国对微灌石英砂过滤器的研究始于20世纪90年代[4-7],迄今为止,在砂过滤器的过滤和反冲洗方面都开展了大量的试验研究[8-11]。近几年,基于计算流体动力学的数值模拟方法迅速发展[12-13],并逐步应用于旋流式过滤器[14]和网式过滤器的研究[15-17]。但对于砂过滤器的数值模拟较少,仅有个别文献进行了二维模拟[18]。
数值模拟的方法可以大幅减少试验量,还可以从微观结构研究砂过滤器的运行机理,笔者采用分形理论[19-20]、多孔介质模型[21]和数值模拟方法[20,22]开展了一系列前期研究。为了减少模拟计算量,同时增加模拟的稳定性,并得出更具普遍性的结论,本文采用Eulerian-Eulerian模型的简化形式Mixture两相流模型,对石英砂滤层反冲洗过程中水的体积分数随时间的变化过程进行了三维动态模拟,引入了反冲洗流化倍数的概念,根据水的体积分数波动特性,确定了保持水的体积分数稳定变化的临界反冲洗流化倍数,为砂过滤器的反冲洗研究提供了技术支撑,为反冲洗性能参数的确定提供了参考。
1 石英砂滤层反冲洗试验
试验在中国农业科学院农田灌溉研究所进行。试验用材料为石英砂滤层,采用粒径范围为1.0~1.18、1.18~1.4和1.4~1.7,当量粒径分别为1.06、1.2和1.5 mm的3种滤层。试验用模型装置如图1所示,过滤器采用透明有机玻璃管制作,有机玻管内径200 mm、高1 200 mm,在其上每隔100 mm高度打孔,设为测压取料孔,有机玻管下端安装3个滤帽。石英砂滤料放置于过滤器内部,滤层孔隙率0.44,厚400 mm。试验时,使用水池供水,采用涡轮流量计(LWGY-25)测流量,采用U型压差计测量滤层内部压差。
反冲洗试验时,利用水泵将清水从反冲洗进水口注入过滤器模型,通过砂过滤器底部滤帽将水流分散并均匀作用于石英砂滤料,试验过程中,记录下每一个反冲洗速度对应的滤层膨胀高度,由膨胀高度计算出滤层水的体积分数。水的体积分数指在水与石英砂的混合物中,水的体积占混合物总体积的占比,计算如下[23-25]。

式中为水的体积分数;为石英砂的净体积,可以由质量与密度的比值得到,m3;为滤层高度,m;为滤层截面面积,m2。
由式(1)得

2 石英砂滤层反冲洗数值模拟
2.1 砂滤层反冲洗模拟模型
微灌石英砂滤层的反冲洗过程属于复杂的固液多相流系统,因此,滤层反冲洗过程模拟需采用多相流模型。Mixture模型是Eulerian-Eulerian模型的简化形式[26-28],该模型的收敛性和稳定性要优于Eulerian-Eulerian模型,且适用于颗粒相分布范围比较广泛的情况。而微灌石英砂滤层反冲洗过程与Mixture模型的适用条件十分吻合,因此采用Mixture模型模拟水与石英砂组成的固液两相流,其中,水为连续相,石英砂为离散相。Mixture模型连续性方程为
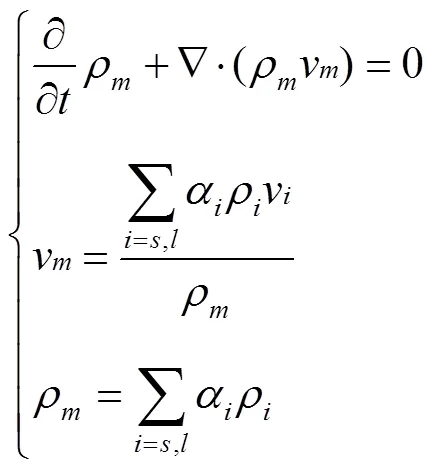
式中分别代表固相与液相;为模拟时间,s;v为平均速度,m/s;ρ为混合相密度,m3/s;为相数;α(下同)为第相体积分数,无量纲量;v为第相速度,m/s;ρ为第相密度,m3/s。
Mixture模型两相流的动量方程为
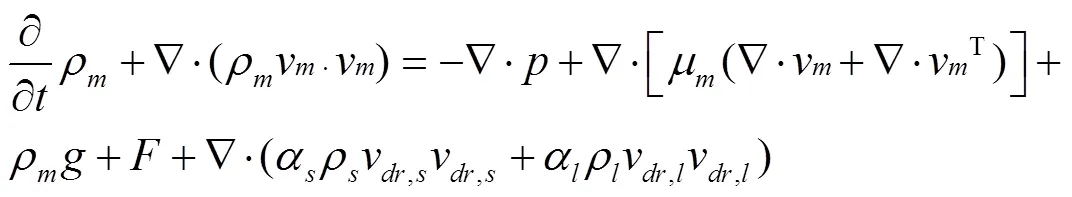
其中

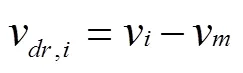
式中▽为拉普拉斯算子;为压力,Pa;为体积力,Pa;μ为混合黏度,Pa·s;v,i为相的漂移速度,m/s。
固相与液相的相对速度v为

Manninen等[29]给出了驰豫时间τ的表达式

Schiller等[30]提出了曳力函数drag的表达式
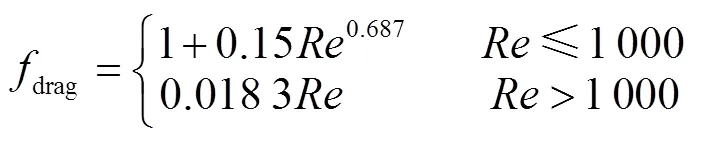
式中为雷诺数。
固相的体积分数方程为

2.2 几何模型及算法设置
采用Gambit软件建立几何模型,过滤器几何模型与细部结构见图2。
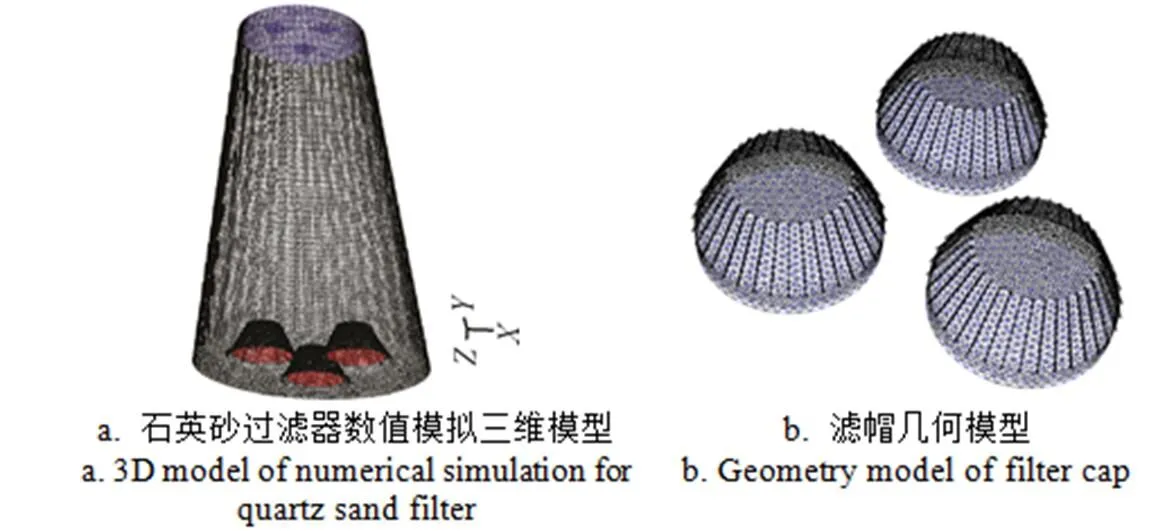
图2 过滤器几何模型
采用时间的二阶隐式控制方程和瞬态求解器计算。采用Mixture多相流模拟模型,采用PC-SIMPLE算法求解压力速度耦合方程,采用基于Green-Gauss的梯度方程进行空间离散化,动量、湍动能、湍流耗散率和体积分数方程均采用一阶迎风格式,进口边界设为速度进口,出口边界设为压力出口,并以速度进口对流场进行初始化。采用模拟软件Fluent14.5进行数值计算,参数设置如表1。
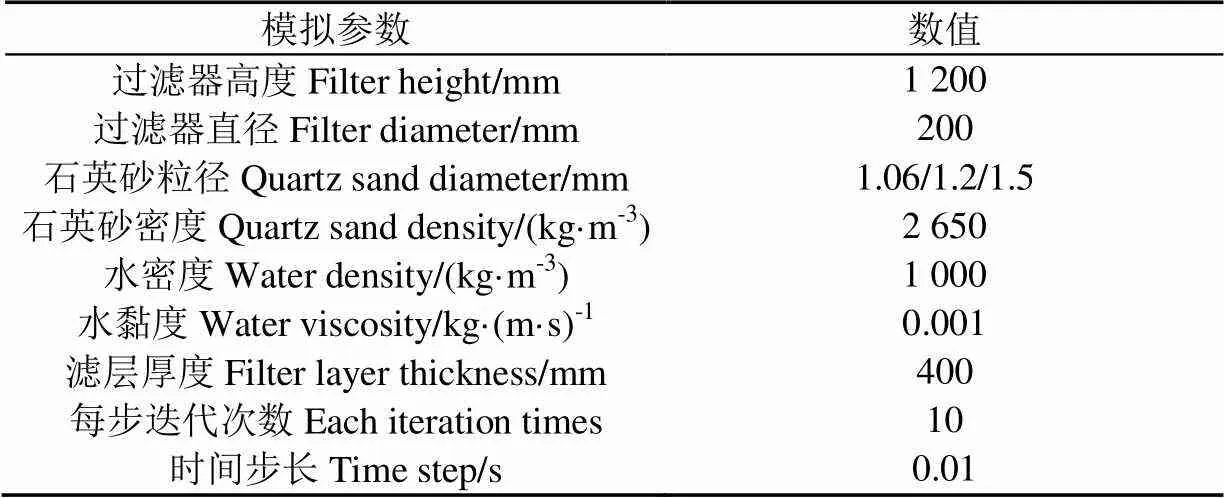
表1 数值模拟参数
3 数值计算结果与分析
3.1 滤层水的体积分数的试验验证
根据入口的反冲洗流速,由CFD软件计算出滤层水的体积分数,绘出水的体积分数随反冲洗速度的变化关系图并与试验值进行对比,如图3所示。由图3可知,当滤层当量粒径为1.06 mm时,滤层水的体积分数的最大误差为4.62%;当滤层当量粒径为1.2 mm时,滤层水的体积分数的最大误差为5.38%;当滤层当量粒径为1.5 mm时,滤层水的体积分数的最大误差为5.64%。对比结果说明,滤层水的体积分数的试验值与模拟值能够较好地吻合,模拟结果准确可信。

图3 滤层水的体积分数模拟值与试验值对比
以v表示滤层最小反冲洗流化速度,最小反冲洗流化速度的倍数称为反冲洗流化倍数。对于当量粒径为1.06、1.2和1.5 mm的滤层,选取1.1v、1.3v、1.5v、1.7v、1.9v5个反冲洗速度对滤层水的体积分数的变化规律进行分析。
3.2 滤层横截面水的体积分数的波动规律分析
在滤层中由低到高依次选取高度为15、25和35 cm的3个横截面,绘制3种滤层,5个反冲洗速度对应的水的体积分数随时间的变化关系曲线,如图4~图6所示。
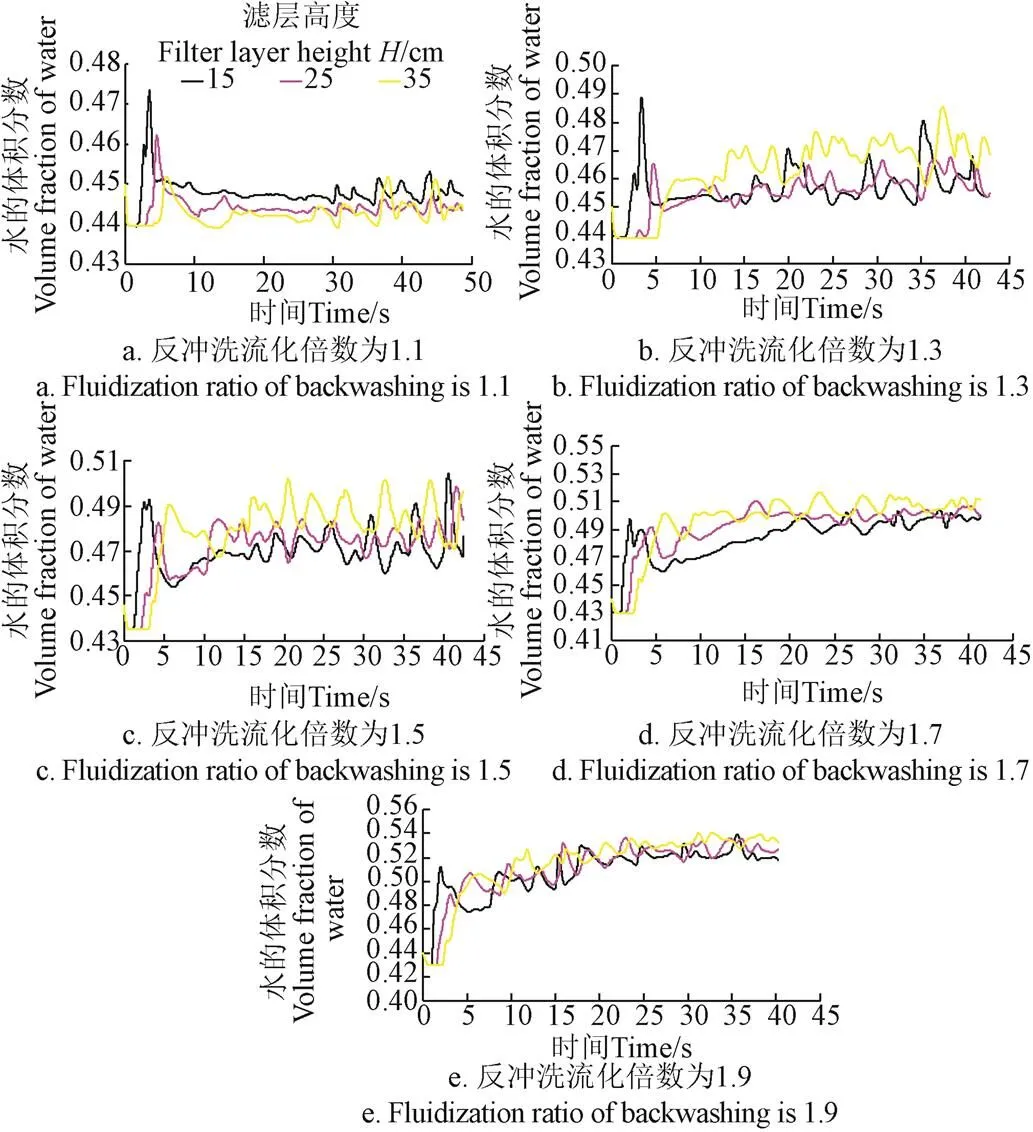
注:最小反冲洗流化速度的倍数称为反冲洗流化倍数。

图5 不同滤层高度水的体积分数随时间变化关系曲线(当量粒径为1.2 mm)
由图4-图6可知,在反冲洗的初始阶段,水流刚进入滤层,水的体积分数在滤层的自然堆积状态做短暂停留,然后由自然堆积状态迅速提高到最高点,之后又在极短时间内下降,经过几个周期的波动逐渐稳定至某一固定值,并围绕这一固定值上下波动。造成这种现象的原因是,在反冲洗的初期,石英砂滤层处于自然堆积状态,水的体积分数相应较小,水流通过滤层时,遇到较大阻力,水流的冲击导致滤层迅速膨胀,从而水的体积分数迅速增大,由于水流空隙的增加,水流速度则随之减小,水流对石英砂颗粒的携带作用随之减小,石英砂由上升迅速回落,滤层水的体积分数又达到最小值。经过这个短暂的突变过程后,滤层水的体积分数与水流速度逐渐相适应并稳定下来。

图6 不同滤层高度水的体积分数随时间变化关系曲线(当量粒径为1.5 mm)
为了对滤层水的体积分数波动规律进行深入分析,计算每种滤层每个截面水的体积分数的平均值,绘出水的体积分数均值随反冲洗速度的变化关系图(图7)。由图7可知,随着反冲洗速度的增加,水的体积分数均值呈增加趋势。但对于同一反冲洗速度,不同的滤层高度上水的体积分数均值基本相同,这说明,单纯从均值看,水的体积分数在整个滤层内分布比较均匀。
计算每种滤层每个截面水的体积分数的标准偏差,绘出水的体积分数的标准偏差随反冲洗速度的变化关系图(图8)。由图8可知,随着反冲洗速度的增加,水的体积分数的标准偏差呈增加趋势,说明在均值稳定的情况下,水的体积分数的波动幅度呈增加趋势。当滤层反冲洗流化倍数达到1.7时,标准偏差急剧变大,说明滤层开始变得不稳定。
由此可知,石英砂滤层临界反冲洗流化倍数为1.7。当反冲洗流化倍数大于1.7时,水的体积分数的均值虽然仍然稳定,但由于标准偏差增大,水的体积分数出现极大值和极小值的情形增多,水的体积分数的极小值出现表明石英砂滤层出现局部堆积,对于反冲洗是不利的。当反冲洗流化倍数范围为1~1.7时,反冲洗效果是理想的。

图7 不同滤层高度水的体积分数均值随反冲洗流化倍数的变化关系曲线

图8 不同滤层高度水的体积分数标准偏差随反冲洗流化倍数的变化关系曲线
4 结论与讨论
1)采用Mixture模型作为反冲洗模拟模型,建立了以水为液相、以石英砂为固相的Mixture两相流模型的控制方程、驰豫时间方程和曳力函数。
2)对滤层反冲洗过程水的体积分数的变化进行了瞬态模拟,并且通过室内试验对模拟结果进行了验证,水的体积分数的最大模拟误差为5.64%,表明数值模拟结果准确可信。
3)分析了反冲洗过程水的体积分数的变化规律,通过对水的体积分数均值和标准偏差的分析,确定了使水的体积分数波动保持稳定的临界反冲洗流化倍数。结果表明,石英砂滤层临界反冲洗流化倍数为1.7,当反冲洗流化倍数范围为1~1.7时,反冲洗效果是理想的。反冲洗流化倍数与滤层粒径无关,反冲洗效果是否理想,决定因素是临界反冲洗流化倍数。
多相流动态数值模拟对于计算工具的要求非常高,为了将模拟计算的工作量控制在一定范围,笔者在对石英砂滤层反冲洗过程进行模拟时,设定的时间步长为0.01 s,每步迭代次数为10次,保证了计算过程能够收敛,并能反映出滤层反冲洗的大致规律,但模拟结果精度较低。下一步的研究中,应采用计算速度更快的计算工具,设定小于0.001 s的时间步长,每步迭代次数不少于20次,从而增加模拟精度,得出水的体积分数更准确的波动规律、动态云图和临界反冲洗速度等模拟结果。
[1] 黄修桥,高峰,王景雷,等. 节水灌溉发展研究[M]. 北京:科学出版社,2014:5-7.
[2] 谢新民,张海庆,尹明万,等. 水资源评价及可持续利用规划理论与实践[M]. 郑州:黄河水利出版社,2003:231-234.
[3] 李久生,王迪,栗岩峰. 现代灌溉水肥管理原理与应用[M].郑州:黄河水利出版社,2008:161-165.
[4] 董文楚. 微灌用砂过滤器的过滤与反冲洗[J]. 中国农村水利水电,1996(12):15-20.
Dong Wenchu. On filtering and inverse washing of sandy filter in micro-irrigation[J]. China Rural Water and Hydropower, 1996(12): 15-20. (in Chinese w ith English abstract)
[5] 董文楚. 微灌用过滤砂料选择与参数测定[J]. 喷灌技术,1995(2):42-46.
Dong Wenchu. The material selection and parameter determination of sand filter in micro irrigation[J]. Sprinkler Irrigation Technology, 1995(2): 42-46. (in Chinese w ith English abstract)
[6] 翟国亮,陈刚,赵武,等. 微灌用石英砂滤料的过滤与反冲洗试验[J]. 农业工程学报,2007,23(12):46-50.
Zhai Guoliang, Chen Gang, Zhao Wu, et al. Experimental study on filtrating and backwashing of quartz sand media in micro-irrigation filter [J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2007, 23(12): 46-50. (in Chinese w ith English abstract)
[7] 翟国亮,冯俊杰,邓忠,等. 微灌用砂石过滤器反冲洗参数试验[J]. 水资源与水工程学报,2007,18(1):24-28.
Zhai Guoliang, Feng Junjie, Deng Zhong, et al. Parameters experiment of backwashing on sandy filter in micro- irrigation[J]. Journal of Water Resources & Water Engineering, 2007, 18(1): 24-28. (in Chinese w ith English abstract)
[8] 冯俊杰,翟国亮,邓忠,等. 微灌过滤器用水压驱动反冲洗阀启闭机构的力学计算[J]. 农业机械学报,2007,38(12):212-214.
Feng Junjie, Zhai Guoliang, Deng Zhong, et al. Mechanical calculation of opening and closing mechanism of back flushing valve driven by hydraulic pressure[J]. Transactions of the Chinese Society of Agricultural Machinery, 2007, 38(12): 212-214. (in Chinese w ith English abstract)
[9] 邓忠,翟国亮,仵峰,等. 微灌过滤器石英砂滤料过滤与反冲洗研究[J]. 水资源与水工程学报,2008,19(2):34-37.
Deng Zhong, Zhai Guoliang, Wu Feng, et al. Study on the filtration and backwashing for the quartz filter in micro- irrigation[J]. Journal of Water Resources & Water Engineering, 2008, 19(2): 34-37. (in Chinese w ith English abstract)
[10] 赵红书. 微灌用石英砂滤料的过滤与反冲洗性能研究[D].北京:中国农业科学院,2010.
Zhao Hongshu. Performance of Filtration and Flushing of Quartz Sand Media for Micro-irragation[D]. Beijing: Chinese Academy of Agricultural Sciences, 2010. (in Chinese with English abstract)
[11] 张文正. 微灌砂滤层气水反冲洗与过滤的试验研究[D]. 北京:中国农业科学院,2013:1-5.
ZhangWenzheng. Experiment Research of Air Water Backwashing and Filtration of Sand Layer in Micro- irrigation[D]. Beijing: Chinese Academy of Agricultural Sciences, 2013: 1-5. (in Chinese with English abstract)
[12] ANSYS Inc. ANSYS FLUENT User’s Guide[M]. Pittsburgh: ANSYS Inc, 2011: 301-309.
[13] ANSYS Inc. ANSYS FLUENT Theory Guide[M]. Pittsburgh: ANSYS Inc, 2011: 486-192.
[14] 宋辉智,塔娜,王全喜,等. 溢流管插入深度及圆柱段高度对旋流式过滤器沉沙效果的影响[D]. 内蒙古:内蒙古农业大学,2012:3-7.
Song Huizhi, Ta Na, Wang Quanxi, et al. Overflow Pipe Insertion Depth and the Cylindrical Section Height of Cyclone Filter Settling Effect[D]. Inner Mongolia: Inner Mongolia Agricultural University, 2012: 3-7. (in Chinese with English abstract)
[15] 王新坤,高世凯,夏立平,等. 微灌用网式过滤器数值模拟与结构优化[J]. 排灌机械工程学报,2013,31(8):719-723.
Wang Xinkun, Gao Shikai, Xia Liping, et al. Numerical simulation and structure optimization of screen filter in micro-irrigation[J]. Journal of Drainage and Irrigation Machinery Engineering, 2013, 31(8): 719-723. (in Chinese with English abstract)
[16] 王栋蕾,宗全利,刘建军,等. 微灌用自清洗网式过滤器自清洗结构流场分析与优化研究[J]. 节水灌溉,2011,12:5-8.
Wang Donglei, Zong Quanli, Liu Jianjun, et al. Flow analysis and structure optimization of Self cleaning nets filter for micro-irrigation[J]. Water Saving Irrigation, 2011, 12: 5-8. (in Chinese with English abstract)
[17] 宗全利,郑铁刚,刘焕芳,等. 滴灌自清洗网式过滤器全流场数值模拟与分析[J]. 农业工程学报,2013,29(16):57-65.
Zong Quanli, Zheng Tiegang, Liu Huanfang, et al. Numerical simulation and analysis on whole flow field for drip self-cleaning screen filter[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2013, 29(16): 57-65. (in Chinese with English abstract)
[18] 刘文娟. 石英砂过滤器过滤及反冲洗特性的实验研究与数值模拟[D]. 北京:中国农业科学院,2014:35-38.
Liu Wenjuan. Experimental Study and Numerical Simulation of Filtration and Backwashing Characteristics of Quartz Sand Filter[D]. Beijing: Chinese Academy of Agricultural Sciences, 2014: 35-38. (in Chinese with English abstract)
[19] 李景海,刘清霞,黄修桥,等. 微灌石英砂滤层流态特性与分形阻力模型参数确定[J]. 农业工程学报,2015,31(13):113-119.
Li Jinghai, Liu Qingxia, Huang Xiuqiao, et al. Flow state characteristics and fractal model parameters determination of quartz sand filter layer used in micro-irrigation[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2015, 31(13): 113-119. (in Chinese with English abstract)
[20] 李景海. 微灌石英砂滤层清洁压降分形阻力模型与反冲洗数值模拟[D]. 北京:中国农业科学院,2016:70-81.
Li Jinghai. Fractal resistance Model of Clean Pressure Drop and Numerical Simulation of Backwashing Process of Quartz Sand Filter Layer in Micro-irrigation[D]. Beijing: Chinese Academy of Agricultural Sciences, 2016: 70-81. (in Chinese with English abstract).
[21] 李景海,刘清霞,黄修桥,等. 微灌石英砂滤层清洁压降计算参数确定与分析[J]. 灌溉排水学报,2016,35(11):24-28.
Li Jinghai, Liu Qingxia, Huang Xiuqiao, et al. Determination and analysis of the calculation parameters for the cleaning pressure drop of quartz sand filter layer used in Micro-Irrigation[J]. Journal of Irrigation and Drainage, 2016, 35(11): 24-28. (in Chinese with English abstract)
[22] 李景海,翟国亮,黄修桥,等. 微灌石英砂过滤器反冲洗数值模拟与流场分析[J]. 农业工程学报,2016,32(9):74-82.
Li Jinghai, Zhai Guoliang, Huang Xiuqiao, et al. Numerical simulation and flow field analysis of backwashing of quartz sand filter in micro irrigation[J]. Transactions of the Chinese Society of Agricultural Engineering, 2016, 32(9): 74-82. (in Chinese with English abstract).
[23] 董文楚. 微灌用砂过滤器水力性能研究[J]. 喷灌技术,1996(1):7-14.
Dong Wenchu. Study on the hydraulic performance of sand filter in micro irrigation[J]. Sprinkler Irrigation Technology, 1996(1):7-14. (in Chinese with English abstract)
[24] 董文楚. 微灌用砂过滤器堵塞与反冲洗效果研究[J]. 武汉水利电力大学学报,1996,29(6):30-34.
Dong Wenchu. Study on the clogging and backflushing coefficieng of the sand filters for micro-irrigation[J]. J. Wuhan Univ. of Hydr. & Elec.Eng., 1996, 29(6): 30-34. (in Chinese with English abstract)
[25] 马俊. 球床多孔介质通道高速区流动特性研究[D]. 黑龙江:哈尔滨工程大学,2010:19-20.
Ma Jun. Research on the High Speed Fluid Flow in Pebble-Bed Porous Channel[D]. Heilongjiang: Harbin Engineering University, 2010: 19-20. (in Chinese with English abstract)
[26] 贺靖峰. 基于欧拉-欧拉模型的空气重介质流化床多相流体动力学的数值模拟[D]. 北京:中国矿业大学,2012:25-26.
He Jingfeng. Numerical Simulation of Multiphase Fluid Dynamic in Air Dense Medium Fluidized Bed Based on Euler-Euler Model[D]. Beijing: China University of Mining and Technology, 2012:25-26. (in Chinese with English abstract)
[27] 贺靖峰,赵跃民,何亚群,等. 基于Euler-Euler模型的空气重介质流化床密度分布特性[J]. 煤炭学报,2013,38(7):1277-1282.
He Jingfeng, Zhao Yuemin, He Yaqun, et al. Distribution characteristic of bed density in air dense medium fluidized bed based on the Euler-Euler model[J]. Journal of Coal Science & Engineering,2013, 38(7): 1277-1282. (in Chinese with English abstract)
[28] He Jingfeng, He Yaqun, Zhao Yuemin, et al. Numerical simulation of the pulsing air separation field based on CFD[J]. International Journal of Mining Science and Technology, 2012, 22(2): 201-207.
[29] Manninen M, Taivassalo V, Kallio S. On the Mixture Model for Multiphase Flow[M]. VTT publications 288:Technical Research Centre of Finland,1996: 52-60.
[30] Schiller L, Naumann Z. A drag coefficient correlation[J]. Z.Ver.Deutsch Ing.. 1935, 77: 318-320.
Optimization of backwashing speed based on transient simulation of water volume fraction in sand filter layer
Li Jinghai1,2, Cai Jiumao3, Zhai Guoliang3※, Liu Qingxia1, Zhang Wenzheng3
(1.,455000,; 2.455000; 3.,,453002)
The volume fraction of water is an important parameter which affects the backwashing effect of quartz sand filter layer. In order to analyze flow field of the volume fraction of water and to determine the reasonable range of backwashing speed in the backwashing process of quartz sand filter layer, numerical simulation method was used in this paper to simulate the dynamic process of the volume fraction of water in the filter layer. For this, the geometric model of quartz sand filter was established and the mesh division of the geometric model was carried out through Gambit software. Because the backwashing process of quartz sand filter layer is a solid-liquid multiphase flow system composed of water and quartz sand, we can conclude that the mixture model is suitable for the numerical simulation of the volume fraction of water by comparing the applicability of the current multiphase flow numerical simulation models such as Eulerian model, mixture model and VOF (volume of fluid ) model. At the same time, because the backwashing process of quartz sand filter layer is both a dynamic and a stable process, the transient simulation solver was adopted. The simulation objects were 3 kinds of quartz sand filter layers whose thickness was all 400 mm, and the equivalent particle diameter was 1.06, 1.2 and 1.5 mm respectively. In order to verify the reliability of simulation results, laboratory experiments of backwashing were conducted with the 3 different quartz sand filter layers in Farmland Irrigation Research Institute, Chinese Academy of Agricultural Sciences, which is located in Xinxiang City, Henan Province, China. The parameters such as the backwashing speed and the total height of the filter layers were measured during the experiments. And the simulation results were compared with the experimental results. Comparison results showed that the maximum simulation error of the volume fraction of water was 5.64%. It was proved that the numerical simulation results were reliable. When the flow field of the volume fraction of water was analyzed with the simulation data, in order to draw a more general conclusion, the concept of fluidization ratio of backwashing was introduced. On this basis, 3 cross-sections, whose heights were 15, 25 and 35 cm respectively, were selected in each filter layer and the fluctuation rule of the volume fraction of water on the sections with time was analyzed when the fluidization ratio of backwashing was 1.1, 1.3, 1.5, 1.7 and 1.9 respectively. Then the mean and the standard deviation of the volume fraction of water were calculated. And their variation trend with the backwashing speed of quartz sand filter layer was analyzed. In the condition that the volume fraction of water in the 3 cross-sections is basically the same, the stability of filter layer can be determined according to the standard deviation. Therefore, it was concluded that the critical value of the fluidization ratio of backwashing was 1.7 for these 3 filter layers. It is said that the standard deviation is modest and the backwashing effect is ideal when the range of the fluidization ratio of backwashing is 1-1.7. The results showed that the fluidization ratio of backwashing decided whether the backwashing effect was ideal. The research results above provide not only a theoretical basis but also a technical support for the operation of the sand filter in the process of backwashing.
irrigation; models; computersimulation; quartz sand filter layer; backwashing; multiphase flow
10.11975/j.issn.1002-6819.2018.02.011
S275.6
A
1002-6819(2018)-02-0083-07
2017-08-14
2017-11-04
“十三五”国家重点研发计划(2016YFC0400202)
李景海,博士,高级工程师,主要从事微灌过滤器及水资源配置研究。Email:649923670@qq.com
翟国亮,研究员,博导,主要从事节水灌溉设备研究。 Email:275580557@qq.com
李景海,蔡九茂,翟国亮,刘清霞,张文正. 基于砂滤层内水体积分数瞬态模拟的反冲洗速度优选[J]. 农业工程学报,2018,34(2):83-89. doi:10.11975/j.issn.1002-6819.2018.02.011 http://www.tcsae.org
Li Jinghai, Cai Jiumao, Zhai Guoliang, Liu Qingxia, Zhang Wenzheng. Optimization of backwashing speed based on transient simulation of water volume fraction in sand filter layer[J]. Transactions of the Chinese Society of Agricultural Engineering (Transactions of the CSAE), 2018, 34(2): 83-89. (in Chinese with English abstract) doi:10.11975/j.issn.1002-6819.2018.02.011 http://www.tcsae.org

