p-Laplacian椭圆型方程解的不存在性问题研究
朱胜兰,李涛会
(无锡城市职业技术学院 无锡环境科学与工程研究中心, 江苏 无锡 214153)
p-Laplacian椭圆型方程解的不存在性问题研究
朱胜兰,李涛会
(无锡城市职业技术学院 无锡环境科学与工程研究中心, 江苏 无锡 214153)
p-Laplacian椭圆型方程;Pohozaev恒等式;解的不存在性

Keywords: p-Laplacian elliptic equations; Pohozaev constant equation; nonexistence of solutions
利用Pohozaev恒等式来证明偏微分方程没有非平凡解始于1965年Pohozaev在文献[1]中关于二阶半线性椭圆型方程齐次Dirichlet问题的正则解建立的积分恒等式。由于Pohozaev恒等式在研究解的不存在性的同时也就得到了解存在的必要条件,从而引起了国内外学者的重视,并被积极地推广。
本文我们主要讨论下面的p-Laplacian椭圆型问题
(1)
不存在非平凡解。
关于问题(1)解的存在性和非存在性及其多解性的研究已经取得了丰富的成果。
2008年, Lari在文献[2]中又研究了问题(1)在f(x,u)=p(x)uα+q(x)uβ时,即
Δu=p(x)uα+q(x)uβ
(2)
整体解的存在性和不存在性。这里0<α≤β,p,q是非负连续函数。笔者通过研究得到如下结果:
1) 假设0<α≤1<β且函数p,q满足条件

(3)
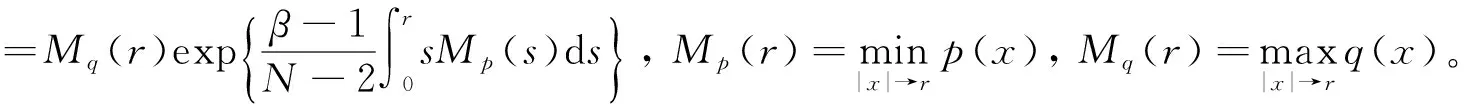
2) 若方程Δv=q(x)vβ(β>1)没有非负整体大解,那么半线性椭圆方程(2)也没有非负整体大解。
另外,在文中 Lari还证明了方程
Δu=u+e-|x|uβ(β>2)
(4)
也没有正的整体大解。
最近, Wu在文献[3]中对式(1)中的
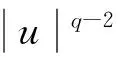
(5)
解的存在性问题进行了探索,笔者通过变分方法发现其在满足一定条件时在RN上存在多个正解。这里1 与上面文献不同的是:本文主要是利用Pohozaev恒等式来证明几类p=2的p-Laplacian椭圆型方程解的不存在性。 (6) (7) (8) 注意到 (9) (10) 那么对于等式(8)有 (11) (12) 注意到 (13) 所以 (14) (15) 即存在rn→∞使得 (16) 下面用反正法来证明结论式(15)。 (17) 那么就可以取rn=r0+n,n=1,2,3,…,当所有n→∞时,rn→∞且r0 (18) 所以有 (19) 在式(14)中取r→∞得 (20) 结论得证,到此,定理1的证明全部结束。 定理2 假设定理1中的条件满足,且f(x,u)∈L1(R1),若存在α∈R1,u≠0,使得 (21) 则方程 -Δu+f(x,u)=0x∈R (22) 没有非平凡解。 下面用一个具体的例子进行说明。 例如:在全空间上考虑方程 (23) 这里,1 证明:由式(23)知此时式(6)中 所以 (24) (25) 则可以得到 (26) 所以 (27) 从而可得 (28) 那么根据所给的条件,方程(23)在下列3种情况下于空间εr,s内均无平凡解: 1)r<2*,s<2*,λ1,λ2≥0; 2)r>2*,s>2*,λ1,λ2≥0; 3)r<2*,s>2*,λ1,λ2≤0。 本文首先介绍了由Pohozaev 提出的恒等式,然后运用它证明相关的p-Laplacian椭圆型方程解的不存在性,得到更广泛的解存在的必要条件,并给出了一些具体的实例来验证结论。 [1] POHOZAEV S.Eigenfunctions of the equation Δu+λf(u)=0[J].Soviet Math Dokl,1965(6):1408-1411. [2] LAIR A V.Large solutions of mixed sublinear-superlinear elliptic equations[J].Math Anal Appl,2008,346:99-106. [3] WU T F.Multiple positive solutions for a class of concave-convex elliptic problems in RN involving sign-changing weight[J].Funct.Anal.,2010,258:99-131. [4] MOSCHINI L,POHOZAEV S I,TESEI A.Existence and nonexistence of solutions of nonlinear Dirichlet problems with first order terms[J].Journal of Functional Analysis,2000,177:365-382. [5] OTANI M.Existence and nonexistence of nontrivial solution of some nonlinear degenerate elliptic equations[ J].Funct Anal,1988,76:140-159. [6] STRUWE M.Variational Methods[M].Berlin:Springer-Verlag,1996. [7] WAGNER A.Pohozaev identity from variational viewpoint[J].Math.Anal.Appl.,2002,266:149-159. [8] AN T Q.Non-existence of positive solutions of some elliptic equations in positive-type domains[J].Applied Mathematics Letters,2007,20:681-685. [9] DE PAIVA F O.Nonnegative solutions of elliptic problems with sublinear indefinite nonlinearity[J].Journal of Functional Analysis,2011,261:2569-2586. [10] 朱胜兰.P-Laplacian 椭圆型方程的整体解[J].重庆理工大学学报(自然科学),2013,27(4):138-142. [11] LARI A V,WOOD A W.Large solutions of sublinear elliptic equations[J].Nonlinear Anal,2000,39:745-753. [12] LARI A V,WOOD A W.Existence of entire large positive solutions of semilinear elliptic systems[J].Diff.Equat,2000,164:380-394. (责任编辑何杰玲) StudyontheNonexistenceofSolutionsforP-LaplacianEllipticEquation ZHU Shenglan, LI Taohui (Wuxi Environmental Science and Engineering Research Center, Wuxi City College of Vocational Technology, Wuxi 214153, China) 2016-12-14 国家自然科学基金资助项目(11201115); 江苏省科技厅基础研究计划(自然科学基金)——青年基金项目(BK20140126) 朱胜兰(1984—),女,博士,讲师,主要从事偏微分方程研究,E-mail:zhushenglan2164@126.com。 朱胜兰,李涛会.p-Laplacian椭圆型方程解的不存在性问题研究[J].重庆理工大学学报(自然科学),2017(9):200-204. formatZHU Shenglan, LI Taohui.Study on the Nonexistence of Solutions for P-Laplacian Elliptic Equation[J].Journal of Chongqing University of Technology(Natural Science),2017(9):200-204. 10.3969/j.issn.1674-8425(z).2017.09.031 O29 A 1674-8425(2017)09-0200-051 主要结论










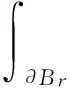
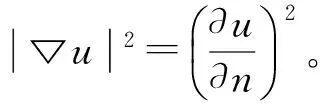




2 实例认证

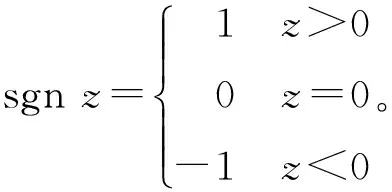

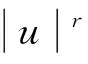


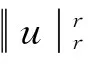


3 结束语

