严格半正张量特征值互补问题的Pareto-谱估计
凌莉芸,凌 晨
(杭州电子科技大学理学院,浙江 杭州 310018)
严格半正张量特征值互补问题的Pareto-谱估计
凌莉芸,凌 晨
(杭州电子科技大学理学院,浙江 杭州 310018)
针对一类严格半正张量特征值互补问题,研究了其Pareto-特征值的符号特征.在此基础上,利用严格半正张量的常量定义和算子定义,得到了严格半正张量特征值互补问题的Pareto-特征值的上下界估计.
张量;严格半正张量;Pareto-特征值;Pareto-谱
0 引 言
张量特征值互补问题[1]是矩阵特征值互补问题[2-3]和张量特征值问题[4-5]的自然推广,它与矩阵特征值互补问题类似,在许多工程领域有广泛应用[1].但与矩阵特征值互补和张量特征值问题比较,张量特征值互补问题的计算更难[6].例如,求最大Pareto-特征值是NP-难问题,因此需要进行相关估计.本文研究了严格半正张量特征值互补问题的Pareto-谱估计.结合算子范数的性质,刻画严格半正张量特征值互补问题的Pareto-特征值的符号特征和上下界估计,对进一步研究结构性张量特征值互补问题具有重要的理论意义.
1 问题描述及相关预备知识
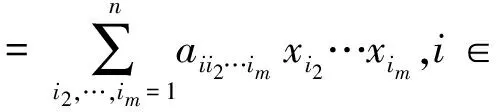

本文考虑张量特征值互补问题,即求λ∈R和x∈Rn{0},使得:

(1)

下面首先给出有关定义和引理.
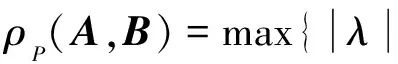
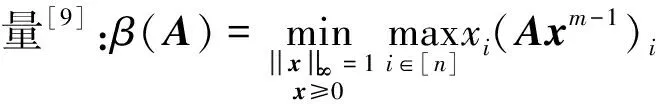
引理1[9]设A∈Tm,n.则A是严格半正张量,当且仅当β(A)>0.
命题1 设A∈Tm,n.若A为严格协正,则A必为严格半正.
下面的例子表明,严格半正张量未必是严格协正张量.
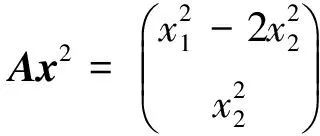
针对给定的A∈Tm,n,现在定义连续正齐次算子TA∶Rn→Rn如下:
特别地,若m是偶数,还可定义另一连续正齐次算子FA∶Rn→Rn:
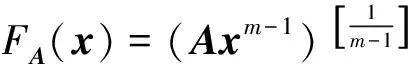
(2)
引理2[10]设A∈Tm,n,则有:

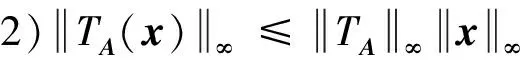
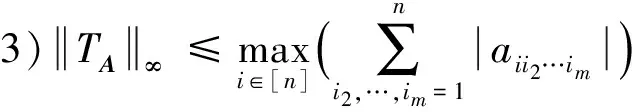
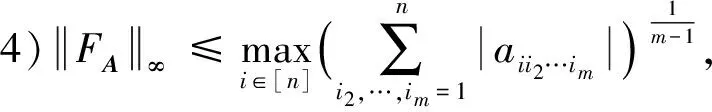
引理3[10]设A∈Tm,n,且A≥0(即A中任一元均非负),则有:
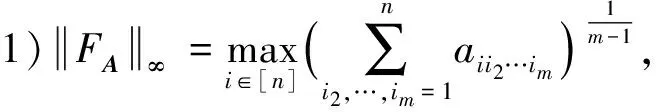
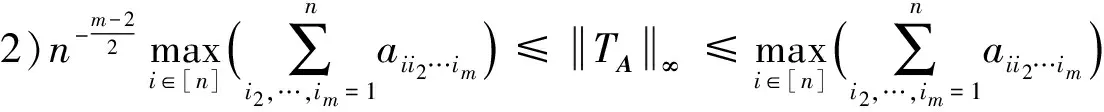
2 主要结果
在文献[1]中所需条件更弱的假设下,考虑式(1)的Pareto-谱估计.首先,讨论A为严格半正且B=-I情形时A的Pareto-特征值符号.
命题2 设A,B∈Tm,n.若A为严格半正且B=-I,则(A,-I)的任一Pareto-特征值均是正的.

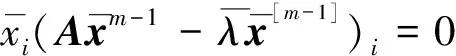
(3)
下面的定理进一步刻画A的Pareto-谱的上下界.
定理1 设A,B∈Tm,n.若A为严格半正且B=-I,则:
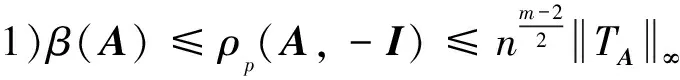
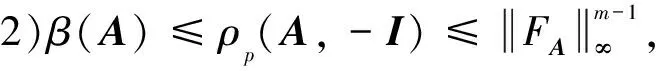
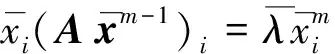
(4)

(5)

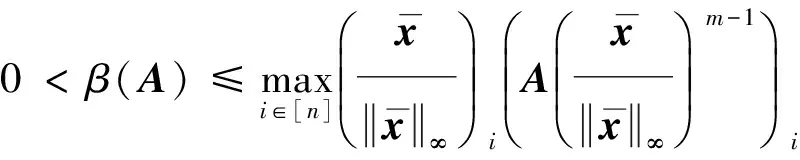
(6)
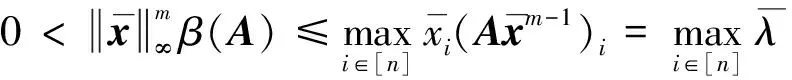
由引理2和定理1,得到下面的结论.
推论1 设A,B∈Tm,n.若A为严格半正,且B=-I,则:
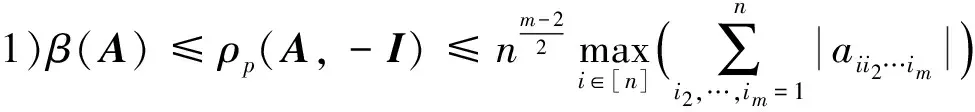
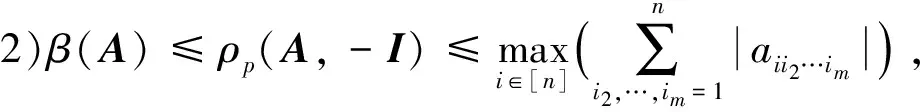
下面讨论A为严格半正且B≥0情形时(A,B)的Pareto-特征值符号和上下界.
命题3 设A,B∈Tm,n.若A为严格半正且B≥0,则(A,B)的任一Pareto-特征值均是负的.
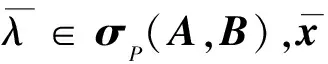
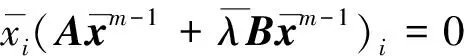
(7)
下面的定理进一步刻画(A,B)的Pareto-特征值的上下界.
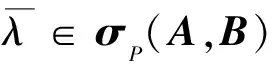

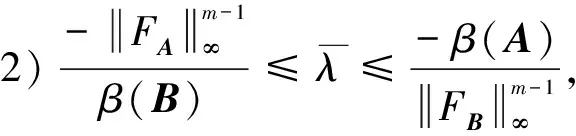
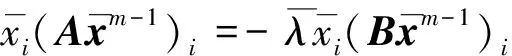
(8)
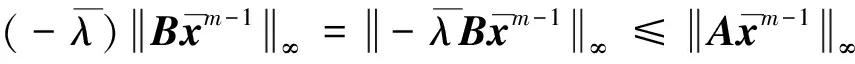
(9)

(10)
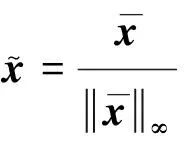
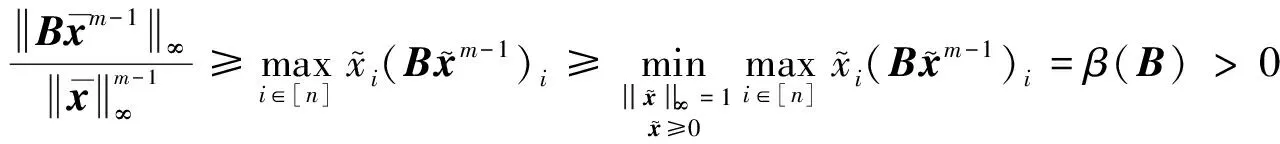
由上式和式(10)知,定理2的结论1中第一个不等式成立.
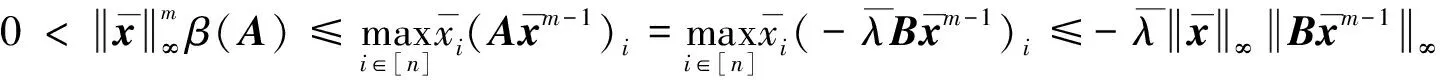
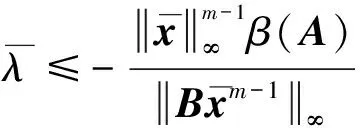
(11)
进一步,由于
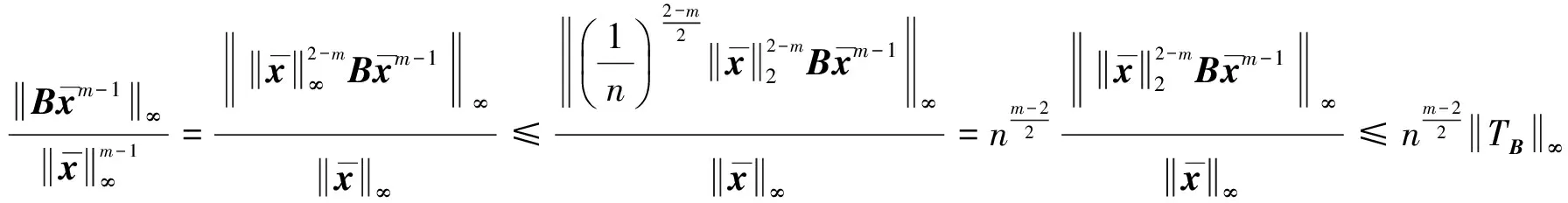
由上式和式(11)知,定理2的结论1中第二个不等式成立.从而定理2的结论1成立.
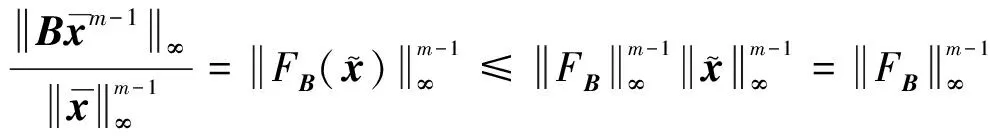
由命题3和定理2,可以得到下述推论.
推论2 设A,B∈Tm,n为严格半正,且B≥0,则:
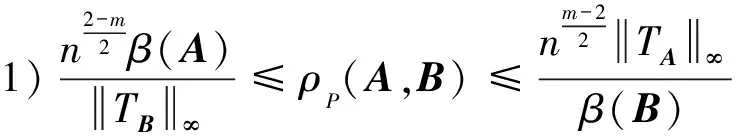
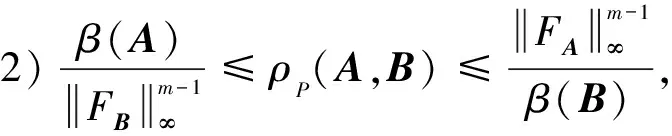
由引理2、引理3和推论2,进一步得到:
推论3 设A,B∈Tm,n为严格半正,且B≥0,则:
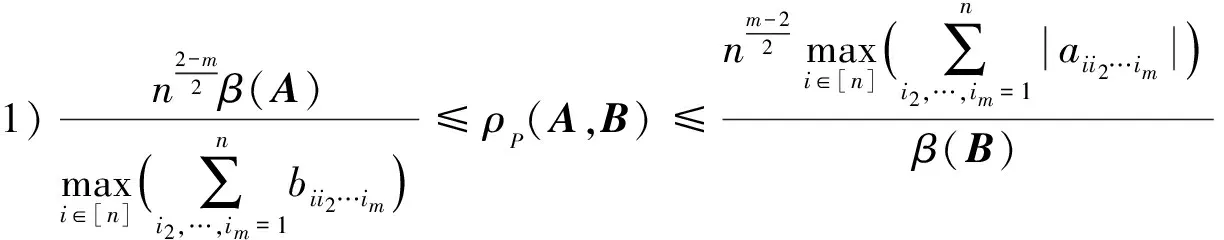

3 结束语
本文首先针对严格半正张量特征值互补问题,分别在B为负单位张量和非负严格半正张量的条件下,讨论了(A,B)的Pareto-特征值的符号特征.在此基础上,进一步得到了(A,B)的Pareto-特征值的上下界估计.这些结果为今后研究一般的(B无限制条件)严格半正张量特征值互补问题的Pareto-特征值的估计提供了新途径.
[1]LING C, HE H, QI L. On the cone eigenvalue complementarity problem for higher-order tensors[J]. Computational Optimization and Applications, 2016, 63(1): 143-168.
[4]QI L. Eigenvalues of a real supersymmetric tensor[J]. Journal of Symbolic Computation, 2005, 40(6): 1302-1324.
[5]LIM L H. Singular values and eigenvalues of tensors: a variational approach[C]// Proceedings of the 1st IEEE International Workshop on Computational Advances in Multi-Sensor Adaptive Processing,2005,1:129-132.
[6]HILLAR C J, LIM L H. Most tensor problems are NP-hard[J]. Journal of the ACM (JACM), 2013, 60(6): 45.
[7]SONG Y, QI L. Properties of tensor complementarity problem and some classes of structured tensors[J/OL]. Eprint Arxiv,2014, [2016-03-23]. http://arxiv.org/abs/1412.0113.
[8]QI L Q.Symmetric nonnegative tensors and copositive tensors[J].Linear Algebra and its Applications, 2013, 439 (1):228-238.
[9]SONG Y, QI L. On strictly semi-positive tensors[J/OL]. Eprint Arxiv,2015, [2016-03-23]. http://arxiv.org/abs/1509.01327.
[10]SONG Y, QI L. Spectral properties of positively homogeneous operators induced by higher order tensors[J]. Siam Journal on Matrix Analysis and Applications,2013, 34(4): 1581-1595.
Pareto-spectrum Estimations of Eigenvalue Complementarity Problem with Strictly Semi-positive Tensors
LING Liyun, LING Chen
(SchoolofScience,HangzhouDianziUniversity,HangzhouZhejiang310018,China)
For a class of eigenvalue complementarity problem with strictly semi-positive tensors, we study the symbolic features of Pareto-eigenvalue. On this based, we obtain the upper and lower bounds of Pareto-eigenvalue for eigenvalue complementarity problem with strictly semi-positive tensors by using the constant definition and operator definition of strictly semi-positive tensors.
tensor; strictly semi-positive tensor; Pareto-eigenvalue; Pareto-spectrum
10.13954/j.cnki.hdu.2016.06.017
2016-04-19
国家自然科学基金资助项目(11571087)
凌莉芸(1991-),女,河南洛阳人,硕士研究生,非线性优化.通信作者:凌晨教授,E-mail: macling@hdu.edu.cn.
O221.2
A
1001-9146(2016)06-0081-05

